ACT Math For Dummies (2011)

Part IV. Visualizing Plane Geometry and Trigonometry

Chapter 11. Trig and Beyond: ACT Advanced Math Topics

In This Chapter

Getting a refresher on trigonometry

Understanding basic matrix operations

Unraveling logarithms

The ACT includes some advanced math topics not found on the SAT. Don’t fret, though. In this chapter, I get you up to speed. I introduce you to some basic concepts in trigonometry, including trig ratios, radian measure, sine and cosine graphs, and trig identities. I also show you how to apply basic math operations to matrices and how to calculate the determinant of a 2 × 2 matrix. Finally, I help you become comfortable working with both logarithms and imaginary and complex numbers.

I discuss these topics in order of their importance on the ACT: You’ll most likely face three or four trigonometry questions, one matrix question, and one question testing you on either logarithms or imaginary numbers. So if you’re pressed for time and need to choose among them, don’t skip the trig!

Trigonometry: Watching the Sines and Taking a Few Tangents

Trigonometry — which literally means “the study of triangles” — builds on the basic geometry of triangles that you discover in Chapter 10. In that chapter, I discuss two important triangles: the 45-45-90 triangle and the 30-60-90 triangle, which are both shown in Figure 11-1.

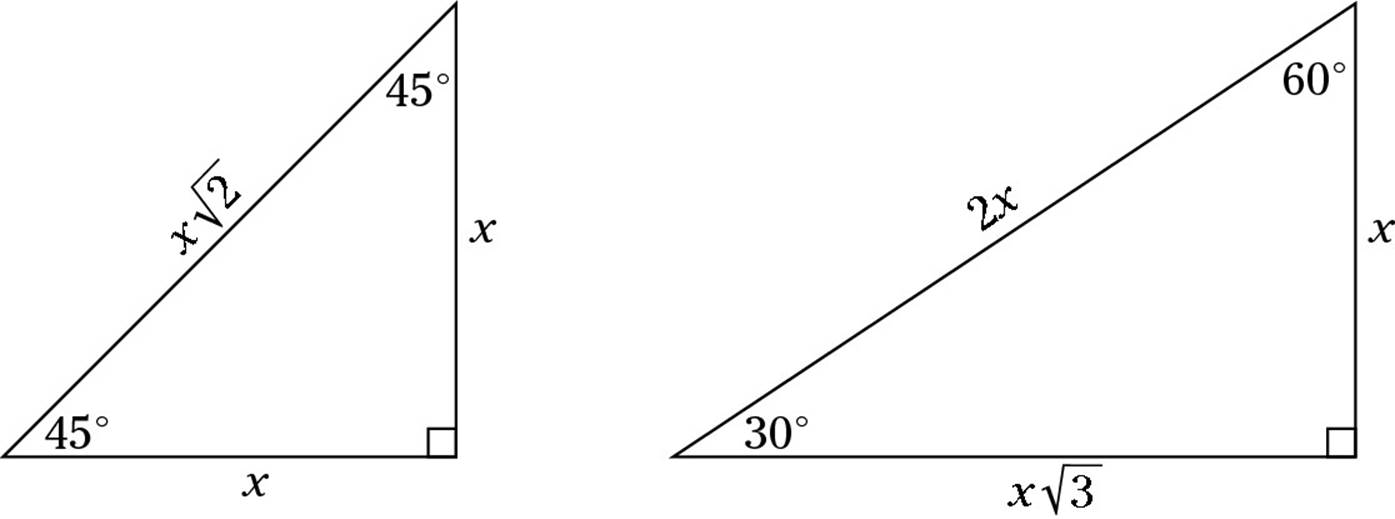
Any two triangles that have all congruent angles and sides in proportion are called similar triangles. The concept of similar triangles plays a vital role in trigonometry.

For example, all 30-60-90 triangles have three sides that are in a ratio of . The additional fact that all 30-60-90 triangles are similar has an important implication: The ratio between any two corresponding sides of a 30-60-90 triangle is always the same, regardless of the size of the triangle. Figure 11-2 shows such triangles.

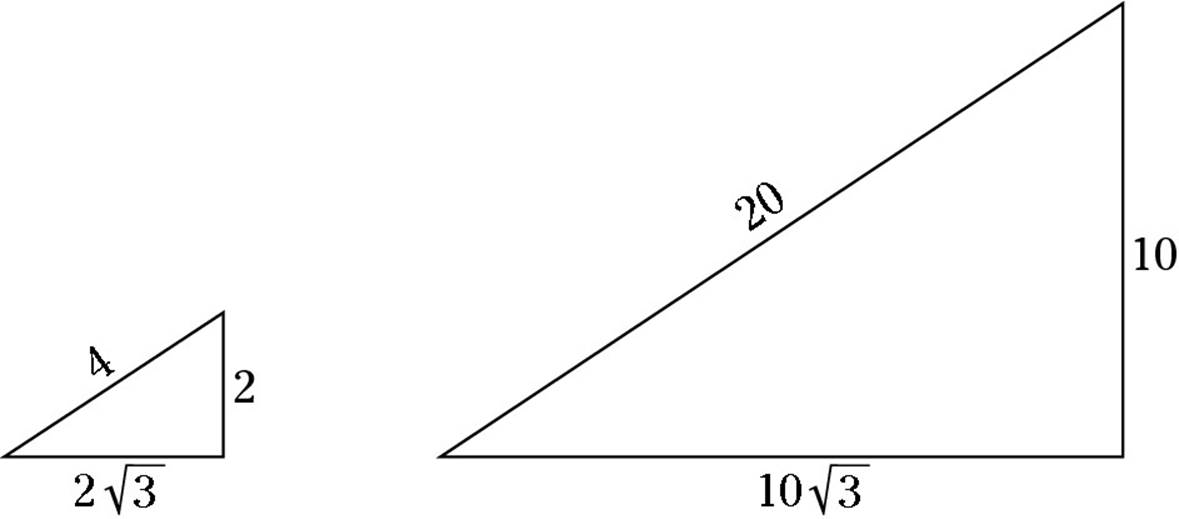
For each triangle in the figure, here’s the ratio of the short leg to the hypotenuse:

Smaller triangle:

Larger triangle:

As you can see, the ratio of the short leg to the hypotenuse of a 30-60-90 triangle is always , regardless of the size of the triangle. This result occurs because all 30-60-90 triangles are similar to each other.

Picking out the opposite, the adjacent, and the hypotenuse

What’s great about similar triangles is that if you know the measure of one angle in any righttriangle, you can find the ratio of any two sides even if you don’t know the length of any of the sides. The first step is to name the three sides of a triangle in relation to the angle that you do know.

The hypotenuse is the longest side, and it’s always directly across from the right angle.

The opposite is the leg of the triangle that isn’t part of the angle.

The adjacent is the leg of the triangle that is part of the angle.

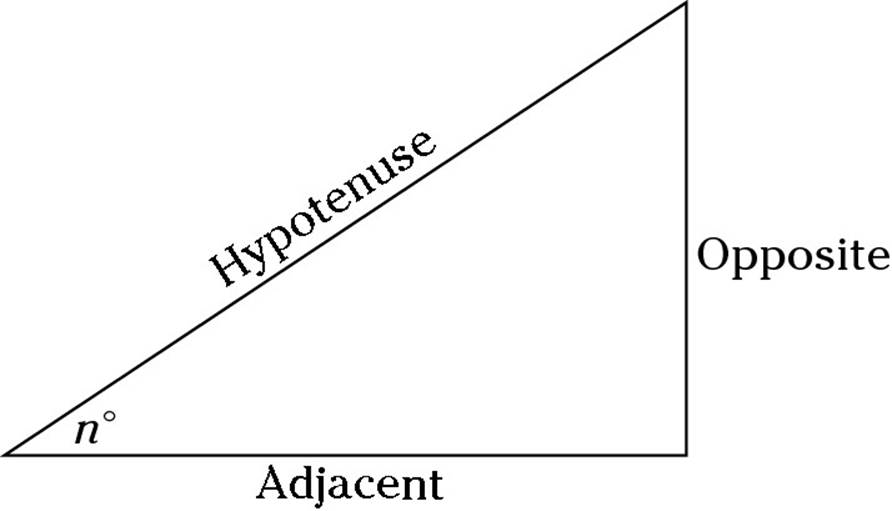
In the triangle in Figure 11-3, you’re working with angle n, which allows you to distinguish the two legs of the triangle in relation to this angle as the opposite (O) and the adjacent (A). The hypotenuse (H) of the triangle is always its longest side.

Knowing how to SOH CAH TOA

Every triangle has three distinct sides — the opposite, the adjacent, and the hypotenuse — giving rise to six distinct ratios among these sides. Table 11-1 lists these ratios and their formulas.

If you have trouble remembering the six trig ratios, the famous (or infamous) mnemonic SOH CAH TOA may help you. This mnemonic stands for:

Sine is Opposite over Hypotenuese.

Cosine is Adjacent over Hypotenuese.

Tan is Opposite over Adjacent.

Knowing these three ratios can help you remember the other three, which are simply reciprocals of the first three:

Sin x is the reciprocal of csc x .

Cos x is the reciprocal of sec x .

Tan x is the reciprocal of cot x .

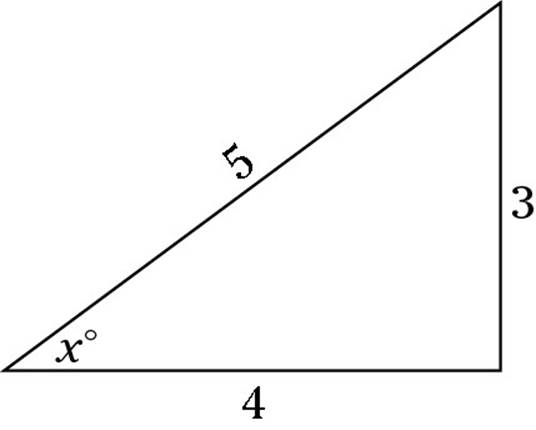
In the following figure, what is the value of cot x?

(A)

(B)

(C)

(D)

(E)

The cotangent of an angle is the ratio of the adjacent side and the opposite side — that is:

Thus, the correct answer is Choice (D).

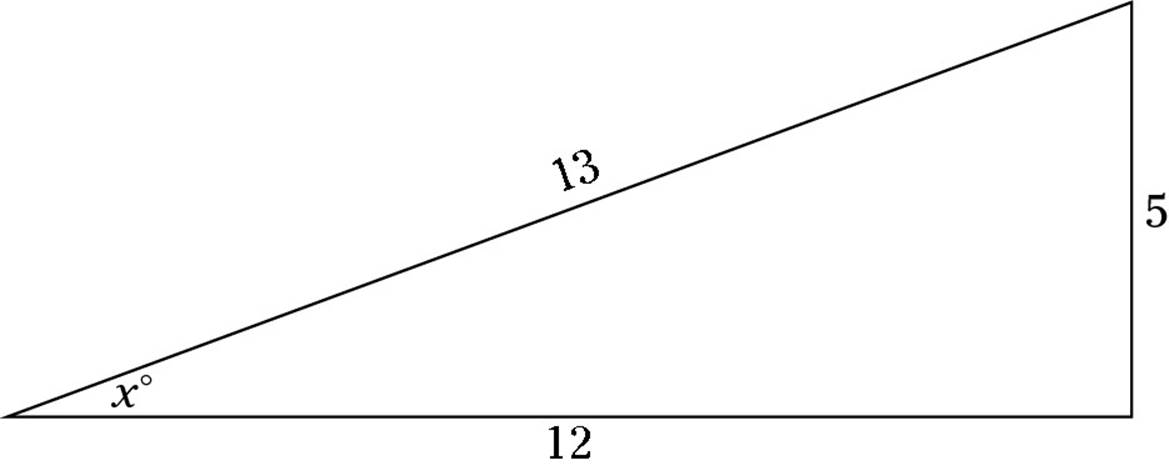
In the following figure, which of the answer choices is equal to ?

(F) sin x

(G) cos x

(H) tan x

(J) cot x

(K) sec x

With respect to angle x in the triangle in the preceding figure:

Therefore, the correct answer is Choice (H).

Feeling radiant with radian measure

Radian measure is an alternative way to measure angles. Instead of dividing the angle measurement of a circle into 360 degrees, a circle is divided into 2π radians. So

360° = 2π radians

Unlike the symbol for degrees (°), the symbol for radians (rad) is rarely used in higher math: An angle without units of degrees is assumed to be expressed in radians. (Also, higher math uses radians almost exclusively.) Table 11-2 shows some conversions for the most commonly used angles.

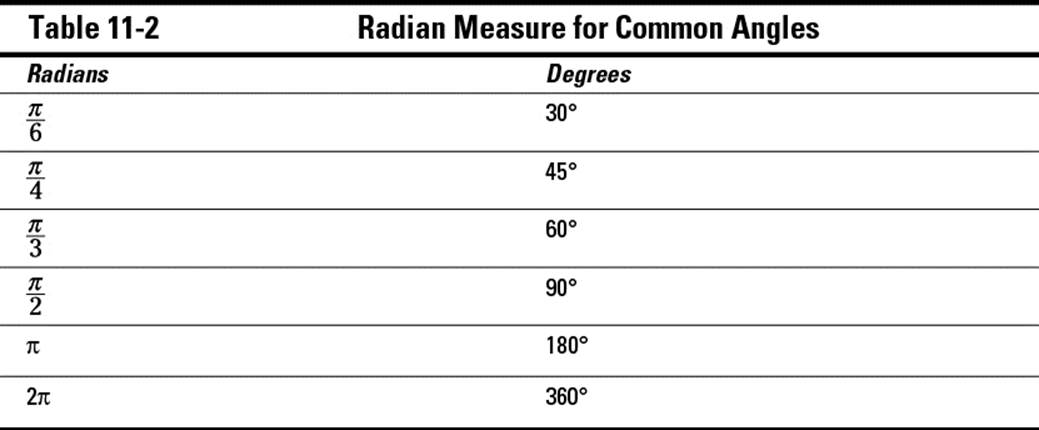
You can use the following proportion for converting other angles from radians to degrees (or vice versa):

What is the value of 75 degrees expressed as radians?

(A)

(B)

(C)

(D)

(E)

Use the formula, substituting 75 for degrees and r for radians:

Solve for r:

So the correct answer is Choice (D).

Graphing trig functions

Although all six trig functions can be graphed, the ACT mostly requires you to work with graphs of sines and cosines. Both of these ratios have similar wave-shaped curves, as shown in Figure 11-4.

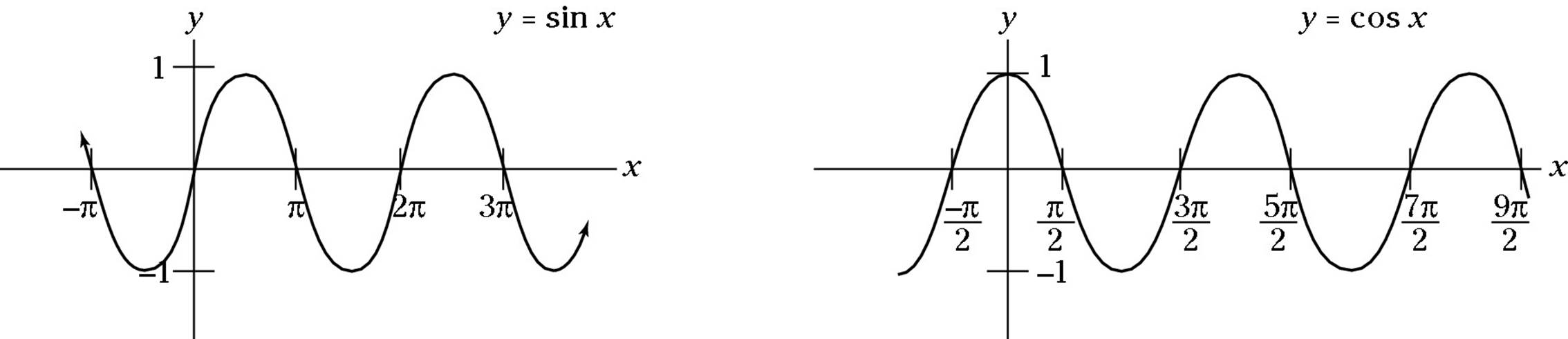
Radian measure (which I introduce in the earlier section, “Feeling radiant with radian measure”) is typically used for graphing trig functions. Here are a few important words for describing waves, some of which may show up on your ACT:

Peak: A peak (or crest) of the function is the highest point on the wave. For example, the function sin x in the figure has peaks at , , , and so forth.

Trough: A trough is the lowest point on the wave. For example, the function sin x has troughs at , , , and so on.

Period: The period of the function is the horizontal distance between two adjacent crests. For example, the period of both sin x and cos x in the figure is 2π.

Amplitude: The amplitude of the function is the vertical distance from the vertical midpoint of the function to the highest point on the function — that is, half the vertical distance from crest to trough. For example, the amplitude of both sin x and cos x is 1.

Use the following graph for the next two example questions:

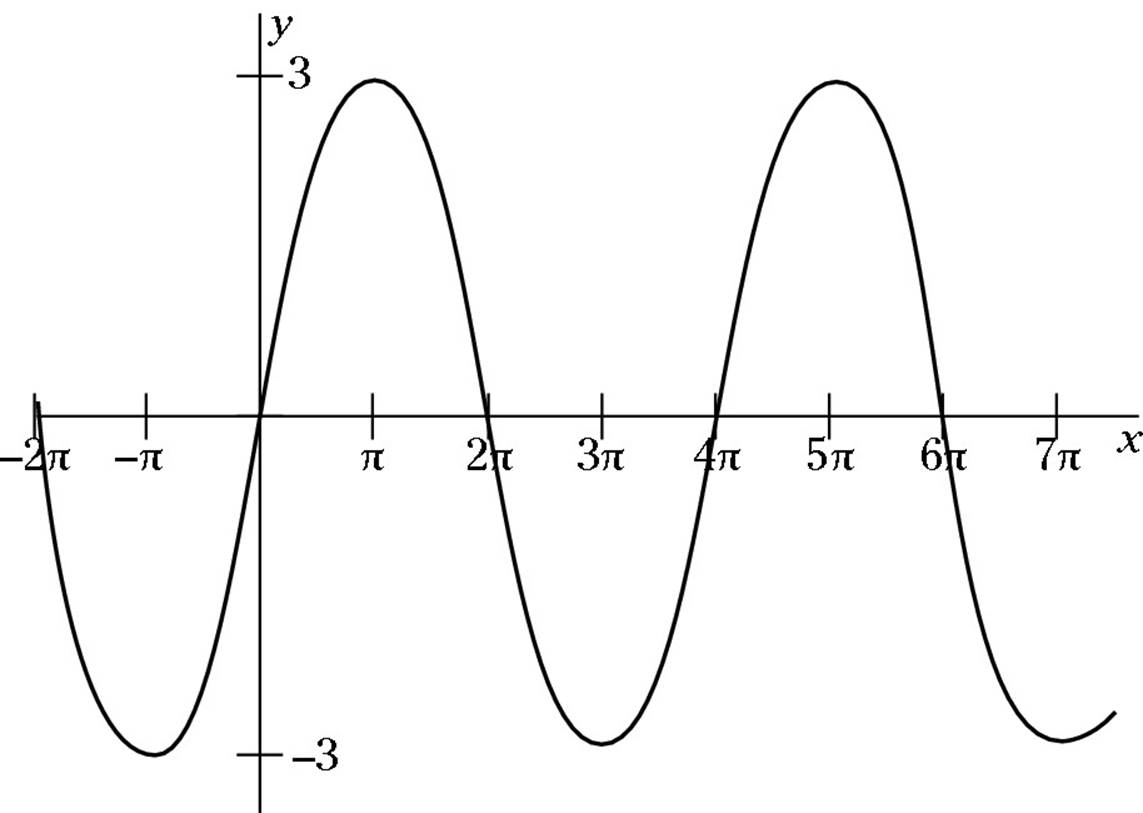
What is the period of this function?

(A) 2

(B) 3

(C) 6

(D) 2π

(E) 4π

The period is the distance from crest to crest, so this distance in the figure is 4π. Therefore, the correct answer is Choice (E).

What is the amplitude of this function?

(F) 2

(G) 3

(H) 6

(J) 2π

(K) 4π

The amplitude is half the vertical distance from crest to trough. This distance in the preceding figure is 3, making the correct answer Choice (G).

A more difficult problem may give you an equation and ask you to identify characteristics of the resulting graph.

Which of the following is the period of the function y = 2 cos x?

(A)

(B)

(C) π

(D) 2π

(E) 4π

This is a transformation of the basic function y = cos x, shown in Figure 11-4. You can use this basic function to sketch the graph you’re looking for by plotting a few points. Either build a table on your calculator or just input a few point values for 2 sin x to create a table like this one:

This should be enough points to sketch the following graph:

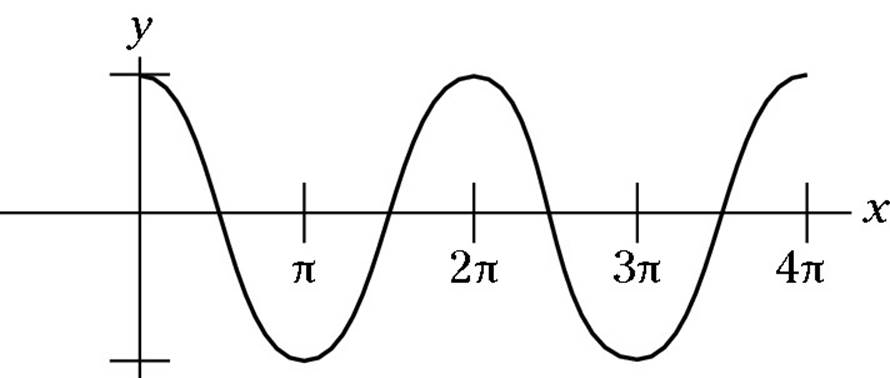
The distance between the two peaks is 2π, so the correct answer is Choice (D).

Identifying trig identities

Recall from the earlier section “Knowing how to SOH CAH TOA” that each of the six trig functions is a ratio of two of the three sides of a right triangle (the opposite, the adjacent, and the hypotenuse). These ratios create connections among the trig functions called identities. These identities are equations using the trig functions that are always true no matter what the value of the variable is. In a high school trig course, you may solve tricky problems that use more than a dozen trig identities. On the ACT, however, you’ll probably need just five of them.

Here are the three reciprocal identities (so called because they show you that three pairs of trig function are reciprocals of each other):

To see why these identities are true, refer to the first identity in the preceding list and remember the SOH CAH TOA rule I introduce earlier in this chapter. This rule tells you that and . So sin x and csc x are reciprocals of each other. You can make similar connections for the other two reciprocal identities.

The next two identities express the tangent and cotangent functions in terms of sines and cosines:

Again, the SOH CAH TOA rule can help you make sense of why these are true. For example, here’s how you use these rules to show why tan x is equal to :

The remaining identity follows easily, because tan x and cot x are reciprocals of each other, so cot x must equal .

When you know these five trig identities, you can use them to answer ACT questions.

If sin x = 2a and cos x = 6b, what is the value of cot x?

(A) 3ab

(B) 12ab

(C)

(D)

(E)

The problem gives you the value of sin x and cos x and asks you to find the value of cot x. Use the identity , and then plug in the values for sin x and cos x:

Therefore, the correct answer is Choice (E).

Reloading the Matrix

A matrix is a grid of numbers or variables arranged in horizontal rows and vertical columns. Figure 11-5 shows a variety of matrices.

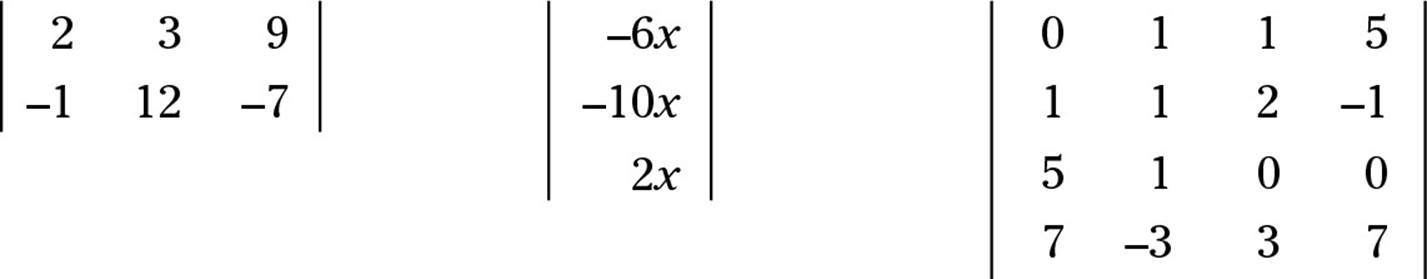
The dimensions of the matrices (that is, the number of rows and columns) in the figure are 2 × 3, 3 × 1, and 4 × 4, respectively. By convention, the number of rows is stated first and the number of columns second. (This convention becomes important when multiplying two matrices, as you discover later in this section.)

Matrices have a variety of uses in math beyond the scope of the ACT. For the test, you only need to know a few basics. I tell you exactly what to focus on in this section.

Adding and subtracting matrices

For the ACT, you definitely want to know how to add and subtract matrices. You’re bound to encounter problems with these simple operations. Luckily, adding matrices is easy: Just add pairs of corresponding numbers in the matrix and place the results in a new matrix.

The two matrices you’re adding must have identical horizontal and vertical dimensions. As a result, the new matrix always has the same dimensions as the two matrices you’re adding.

Which of the following is the result when you add and ?

(A) 4

(B)

(C)

(D)

(E) Cannot be determined from the information given.

To find the correct answer, simply add each pair of corresponding numbers (3 + 5 = 8, 4 + (–6) = –2, –3 + 2 = –1, and –1 + 0 = –1) and then place them in the same positions in a new matrix. The correct answer is Choice (C).

Subtracting matrices is similar to adding them: For each pair of corresponding numbers in the matrix, subtract the second number from the first and then place the results in a new matrix. As with addition, you can only subtract one matrix from another if both matrices have identical horizontal and vertical dimensions.

Which of the following is the result when you perform this matrix subtraction:

– ?

(F)

(G)

(H)

(J)

(K)

Subtract each pair of corresponding numbers in order (7 – 2 = 5, 1 – (–6) = 7, –2 – 0 = –2, and 0 – (–3) = 3) and then place them in the same positions in a new matrix. The correct answer is Choice (H).

Making sense of matrix multiplication

You may encounter ACT questions that ask you to use multiplication with matrices. You may have to multiply an entire matrix by a number (very simple), or you may have to multiply two matrices by one another (not so simple). I show you how to do both in this section.

Multiplying a matrix by a real number

Multiplying a matrix by a real number is straightforward: Simply multiply the integer by every element in the matrix. Doing so is similar to using the distributive property to multiply a number by each term inside a set of parentheses. Yeah, it’s that easy.

Which of the following is the result when you multiply the matrix by 3?

(A) –3 + 3x

(B)

(C)

(D)

(E) Cannot be determined from the information given.

To find the answer, you simply multiply each element of the matrix by 3. Place these three answers into a new matrix of the same dimensions:

Therefore, the correct answer is Choice (D).

Multiplying two matrices

Multiplying two matrices by one another can be tricky, especially when the matrices themselves are lengthy. However, on the ACT, you’ll probably just have to multiply pairs of matrices that have either one row or one column.

Matrix multiplication is not commutative, because the order in which you multiply two matrices can change the result. In other words, if P and Q are matrices, P × Q doesn’t necessarily equal Q × P.

In this section, I show you first how to multiply a matrix that has one column by a matrix that has one row. Then, I show you how to reverse this process and multiply a matrix that has one row by a matrix that has one column.

Multiplying a vertical matrix by a horizontal matrix

An easy way to multiply a vertical matrix by a horizontal matrix is to set up a small grid that resembles a multiplication table. This method allows you to fill in the numbers to get the right answer.

If 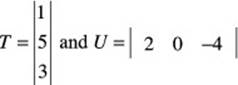, which of the following is the result when you multiply T × U?

(A)

(B)

(C)

(D)

(E)

Note that T has one column and U has one row, so you can multiply these two matrices in this order. So set up a grid with the first matrix in the left-hand column and the second matrix in the top row:

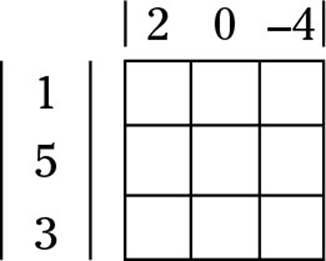
For each box in the grid, multiply the two numbers in the corresponding row and column, like this:

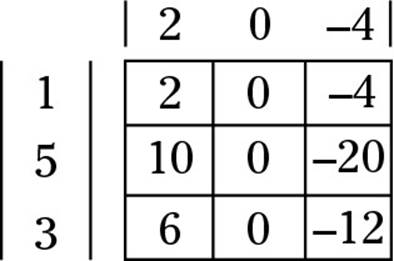
The contents of the grid is the result of the matrix multiplication:

So the correct answer is Choice (D).

Multiplying a horizontal matrix by a vertical matrix

The process for multiplying a horizontal matrix by a vertical matrix is different from multiplying vertical ones by horizontal ones. This fact illustrates the idea that matrix multiplication is not commutative — when you reverse the order of the multiplication, the result is usually a different matrix. In the following example, you multiply the two matrices from the previous section in reverse order to see how this happens.

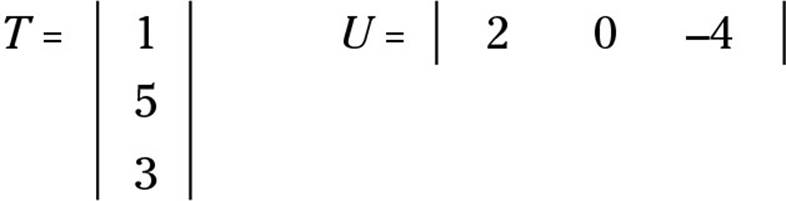
Which of the following is the result when you multiply U × T?

(A)

(B)

(C)

(D)

(E)

Notice that U has three columns and T has three rows, so you can multiply these two matrices in this order. However, the result will be different. To start, make another grid, this time with U in the left-hand column and T in the top row:

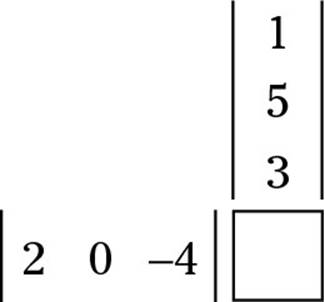
This time, the resulting grid has only one box. To fill it in, multiply the three pairs of corresponding numbers in the two matrices, and then add the results:

(2 × 1) + (0 × 5) + (–4 × 3) = 2 + 0 + (–12) = –10

Place this result into the box:

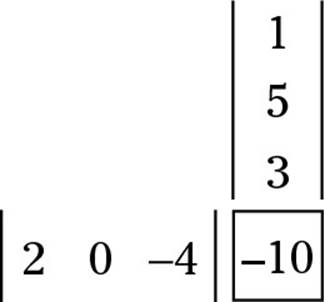
The contents of the grid become the result of the matrix multiplication:

So the correct answer is Choice (A).

Determining the meaning of determinants

A determinant is a common operation performed on a square matrix. On the ACT, the only determinant formula you need to be familiar with is for a 2 x 2 matrix. Here’s the formula for

the determinant of M = :

Det(M) = ad – bc

Note that the determinant of a matrix is simply a number, not a matrix. To solve a problem with a determinant, you simply plug the numbers from the matrix into the formula and solve.

What is the determinant of the matrix ?

(A) –22

(B) –2

(C) 0

(D) 2

(E) 22

First, apply the determinant formula:

ad – bc = (4)(7) – (5)(6)

Now evaluate to solve the problem:

= 28 – 30 = –2

Therefore, the correct answer is Choice (B).

What is the value of k if the determinant of the matrix equals 16?

(F) –2

(G) –1

(H) 0

(J) 1

(K) 2

Start by applying the determinant formula and then evaluate:

ad – bc = (k)(0) – (2k)(k²) = 0 – 2k³ = –2k³

This value equals 16, so create your equation and solve:

–2k³ = 16

k³ = –8

k = –2

So the correct answer is Choice (F).

Logging Some Hours with Logarithms

A logarithm (log for short) is an alternative way of working with powers (exponents). The logs you’re likely to see on the ACT won’t be as difficult as those you may have worked with in your math classes. Still, logs can be a little confusing. To help you out, in this section, I give you a useful way to think about logarithms and then show you how to apply this idea.

Like a root (or radical), a logarithm is an inverse operation because it will undo a power. Recall from Chapter 4 that a power has two parts: a base (bottom number) and an exponent (top number). A root will undo the exponent and give you back the base you started with. For example:

5³ = 125 

In this example, I started with a base of 5 and an exponent of 3. The power operates on these two numbers, giving you 125. The root undoes this operation, eliminating the exponent of 3 and giving you back the base you started with — that is, 5. (I know “undoes” isn’t exactly great word choice, but work with me here.)

In contrast, a logarithm will undo the base and give you back the exponent you started with:

5³ = 125 log₅ 125 = 3

This time I started with the same base of 5 and exponent of 3 but used a logarithm to reverse the operation in a different way, eliminating the base of 5 and giving you back the exponent you started with — that is, 3.

On the ACT, your main task with logarithms is to remove them as quickly as possible. The best way to do that is to turn any equation that has a messy logarithm into one that has a nice, clean power. When you do this, you’ll usually find that the problem looks a lot easier, and you can take it from there without much trouble.

A good way to avoid the confusion of logarithms is to memorize this fact:

log₁₀ 100 = 2 means 10² = 100

When I solve a logarithm problem, I always write this note down so I can refer to it. Believe me, it helps reduce the confusion.

What is the value of n if log₄ n = 3?

(A) 64

(B) 81

(C)

(D)

(E) Cannot be determined from the information given.

The only thing really difficult about this problem is the fact that it includes a logarithm. So your best bet is to get rid of it. To do this, use the tip I just provided to turn this log equation into a power equation:

log₄ n = 3 means 4³ = n

Now the problem practically solves itself:

n = 4³ = 64

Therefore, the correct answer is Choice (A).

Sometimes, you may have a little more work ahead of you, even after you remove the log. But in almost every case, changing the log to a power turns the problem into something you know how to handle.

If log_p 4 = 2, what is the value of p⁴?

(F) 4

(G) 16

(H) 256

(J)

(K)

Turn the log equation into a power equation:

log_p 4 = 2 means p² = 4

The equivalent equation p² = 4 is much more intuitive to work with, and you can solve it easily:

p² = 4

p = ±2

Thus, p is either 2 or –2. In either case, p⁴ = 16, so the correct answer is Choice (G).

Imagining the Possibilities with Imaginary Numbers

When you take the square root of a negative number, the result is an imaginary number. Imaginary numbers are ruled out of many math problems for a variety of reasons. On the ACT, however, you may face a question (though probably not more than one) that requires you to understand imaginary numbers.

The creation of imaginary numbers begins with the leap of faith that they exist in the first place. The variable i is made to equal the square root of –1:

Note why this equation is a leap of faith: It says that when you multiply i by itself, the result is –1. But when you multiply any real number by itself, the result is never less than 0. So i isn’t a real number.

Despite the uncomfortable fact that i isn’t a real number, you can perform the usual operations on it as you would with a variable. For example, you can add i to itself:

i + i = 2i i + i + i = 3i i + i + i + i = 4i

Because you can add i to itself, you can multiply i by a constant using the normal rules of multiplication:

2 × i = 2i –5 × i = –5i 0.1 × i = 0.1i

You also can apply an exponent to i:

i² = –1 by definition

i³ = –i because i³ = i² × i = –1 × i = –i

i⁴ = 1 because i⁴ = i² × i² = –1 × –1 = 1

Finally, you can add a real number to (or subtract a real number from) i:

i + 1 i – 8 6 + 3i –11 – 4i

As when adding a constant to a variable in algebra, you can’t combine a term with i and a term without i. An expression that contains an imaginary number plus or minus a constant is called a complex number. Working with complex numbers is similar to working with algebraic expressions (turn to Chapter 5 for more details). The two examples that follow apply what you already know about math to complex numbers.

Given that i² = –1, what is the result when you multiply 4 + 3i by –6 + 2i?

(A) –6 + 10i

(B) 24 + 10i

(C) 30 + 10i

(D) –24 – 10i

(E) –30 – 10i

Multiply these two expressions using the FOIL rule (see Chapter 5):

(4 + 3i)(–6 + 2i) = –24 + 8i – 18i + 6i²

Combine the two terms containing i (–18i and 8i):

= –24 – 10i + 6i²

Remember that i² = –1, so you can simplify the i² term:

= –24 – 10i – 6

And, finally, combine the two constant terms (–6 and –24):

= –30 – 10i

Thus, the correct answer is Choice (E).

In complex numbers, where i² = –1, which of the following is equivalent to ?

(F) 1

(G) 2

(H) i

(J)

(K)

Add these two fractions just as you would add two rational expressions in algebra. First, multiply the denominators to find the common denominator of (1 + i)(1 – i). Then increase each fraction to adjust the denominators as needed:

Now add the fractions according to the usual rules: Add the numerators and keep the denominator the same. Your equation now looks like this:

Simplify the numerator by combining like terms:

Next simplify the denominator by FOILing and combining like terms:

Remember that i² = –1, so now you can simplify even further:

So the correct answer is Choice (F).