ACT Math For Dummies (2011)

Part III. Digging In to Intermediate Algebra and Coordinate Geometry

In this part. . .

In Part III, I focus on the skills you need to know for intermediate algebra and coordinate geometry. I discuss inequalities, systems of equations, functions, quadratic equations, and lines and parabolas on the xy-graph. I round out the part by providing some practice problems to help prepare you for the practice tests later in the book.

Chapter 7. Moving to Intermediate Algebra

In This Chapter

Working with inequalities

Using systems of equations

Reviewing direct and inverse proportions

Solving quadratic equations

Intermediate algebra takes your basic algebra skills (discussed in Chapter 5) to the next level. In this chapter, you review the subtleties and complexities of algebra that are normally covered at the end of Algebra I and in Algebra II.

You begin by working with inequalities such as >, <, ≥, and ≤, including inequalities that contain absolute value. Then you focus on systems of equations, which are sets of equations with more than one variable that must be solved as a group. Systems of equations are often useful for solving word problems. Next, you see how to work with pairs of variables that are either directly proportional or inversely proportional.

You continue with a look at solving all-important quadratic equations, using both factoring and the quadratic formula. The chapter wraps up with an introduction to functions, including modeling with functions, functional notation, and finding the domain and range of a function.

Knowing More or Less about Inequalities

An inequality is a mathematical statement that tells you two values are unequal. Most of the inequalities you’ll work with on the ACT are of four types, which I show in Table 7-1.

Table 7-1 Four Types of Inequalities

Inequalities differ from algebraic equations because usually you can’t solve for the actual value of the variable. Instead, inequalities are solved for a solution set, which is a set of values that all make the inequality true. A solution set itself is usually expressed as a much simpler version of the original inequality.

An ACT question may ask you for the solution set or for an equivalent inequality, both of which are essentially the same thing. A question also may ask you to identify which of five values is in the solution set or, conversely, which is not in the solution set.

Before you begin solving an inequality, notice whether it’s exclusive (< or >) or inclusive (≥ or ≤). The process of solving an inequality may change the direction of the inequality but not whether it’s exclusive or inclusive. This fact often allows you to easily rule out one or more wrong answers.

In this section, I show you how to use algebra to solve a variety of inequalities, including those that contain absolute value. (For a refresher on absolute value, see Chapter 5.)

Becoming a pro at inequalities

Solving inequalities is virtually identical to solving equations, with one important difference: When you multiply or divide by a negative number, you must reverse the direction of the inequality.

Which of the following is the solution set of the inequality 8 – 5p > 23?

(A) p > 3

(B) p < 3

(C) p ≥ 3

(D) p > –3

(E) p < –3

To begin, notice that Choice (C) changes the inequality from exclusive (>) to inclusive (≥), so you can rule out this answer immediately. Now your goal is to isolate p, just as you would solve an equation. Begin by subtracting 8 from both sides:

8 – 5p > 23

–5p > 15

Your next step is to divide both sides by –5. As you do this, reverse the direction of >, changing it to <:

p < –3

So the correct answer is Choice (E).

When working with inequalities, remember that the only time you need to reverse the direction of the inequality is when the actual step you’re taking is multiplication or division by a negative number. This next example shows how confusion can arise and how to avoid it.

Which of the following is equivalent to the inequality –4(1 – a) < 2a – 8?

(F) a < 0

(G) a < –1

(H) a > –1

(J) a < –2

(K) a > –2

To answer this question, isolate a as usual. Begin by distributing –4 on the left side of the inequality. Although this step involves multiplication by a negative number, you are not multiplying the entire inequality by a negative, sodon’t reverse the direction of the inequality:

–4(1 – a) < 2a – 8

–4 + 4a < 2a – 8

Next, subtract 2a from both sides (again, no need to reverse):

–4 + 2a < –8

Now add 4 to both sides:

2a < –4

Finally, divide both sides by 2. Again, even though the right side of the inequality is negative, the actual step you’re taking does not involve multiplying or dividing by a negative number, so don’t reverse the inequality:

a < –2

Thus, the correct answer is Choice (J).

Be careful when solving inequalities that have fractions. To simplify fractions out of an equation, you may need to cross-multiply. When working with inequalities, move any minus signs in front of a fraction or in the denominator (bottom number) up to the numerator (top number) so you can avoid multiplying or dividing by a negative number.

Which of the following is equivalent to ?

(A)

(B)

(C)

(D)

(E)

For starters, the inequality is ≤, so you can rule out any answer that includes either < or >; therefore, Choice (A) is wrong. To simplify this inequality, you need to cross-multiply, which involves multiplying by the denominator. To avoid the confusion of multiplying by a negative number, begin by moving the minus sign on the left side of the inequality to the numerator:

This adjustment doesn’t change the fraction, but it makes it safer to work with, because you don’t have to multiply by a negative number. Now you can cross-multiply as usual, and then solve for n:

At this point, you need to divide both sides by –5, so reverse the inequality as usual:

Therefore, the correct answer is Choice (E).

Separate but unequal: Solving inequalities with absolute value

As when solving an equation that includes an expression with absolute value, you also need to split an inequality with absolute value into two separate inequalities. However, keep in mind one twist: One of the two resulting inequalities is simply the original inequality with the bars removed. The other inequality is the original inequality with

The bars removed

The opposite side negated (as with absolute value equations)

The inequality reversed (as with inequalities when you multiply or divide by a negative number)

These rules aren’t difficult, but they’re a little complicated, so be careful to do all three parts correctly.

Which of the following values is in the solution set of |4t – 1| < 5?

(A) 0

(B) 2

(C) –2

(D) 4

(E) –4

Begin by splitting the inequality:

4t – 1 < 5 4t – 1 > –5

Notice that the second of these two inequalities has the bars removed, the right side negated, and the inequality sign reversed. You’re now ready to solve both of these inequalities for t:

4t < 6 4t > –4

t < 1.5 t > –1

To make these inequalities a little easier to read, put them in the following form:

–1 < t < 1.5

Thus, 0 falls into the range of solutions, so the right answer is Choice (A).

In some cases, the solution to an inequality with absolute value can lead to a pair of inequalities that appear to contradict each other. When this happens, both inequalities aren’t true, but at least one of them is, so link them with the word or. This concept is a little tricky, so don’t worry if it’s not making sense. The next problem provides a concrete example.

What is the solution set for

(F) n ≤ 4

(G) 1 < n < 4

(H) 1 ≤ n ≤ 4

(J) n < 1 or n > 4

(K) n ≤ 1 or n ≥ 4

Before you begin, notice that the original inequality is ≥, so no solution can include either < or >. As a result, you can rule out Choices (G) and (J). Now isolate |2n – 5| on the left side of the inequality:

You’re now ready to remove the bars and split the inequality:

2n – 5 ≥ 3 2n – 5 ≤ –3

Notice that the second of these two inequalities has the bars removed, the right side negated, and the inequality sign reversed. You’re now ready to solve the first one:

Next, solve the second inequality:

Notice that the two solutions (n ≥ 4 and n ≤ 1) seem to contradict each other: If n is greater than 4, how can it be less than 1? When this situation occurs, either solution can be true, so link the two resulting solutions with the word or:

n ≤ 1 or n ≥ 4

Thus, the correct answer is Choice (K).

Be extra careful when working with an inequality that sets an absolute value either greater than (>) or greater than or equal to (≥) another value that includes a variable. This type of inequality can sometimes produce afalse (or extraneous) solution — that is, a solution that appears correct but doesn’t work when plugged back into the problem. The next example shows you how and why this can happen.

Which of the following is the solution set for |x – 3| > 2x?

(A) x < 1

(B) x < 0

(C) x < –1

(D) x < –2

(E) x < –3

To begin, remove the absolute value bars, split the inequality, and solve each separately:

x – 3 > 2x x – 3 < –2x

–3 > x –3 < –3x

  1 > x

According to this result, x < 1 and x < –3 both appear correct, so you may be tempted to choose Choice (E). However, if this answer were correct, then x = 0 should be outside the solution set. So plugging 0 into the original inequality should give you the wrong answer:

|x – 3| > 2x

|0 – 3| > 2(0)

3 > 0 Correct!

This solution is unexpected. In fact, x = 0 is in the solution set for this inequality.

What went wrong? Take another look at the original inequality:

|x – 3| > 2x

This inequality sets an absolute value greater than 2x. So if x is any negative number, the absolute value (which can never be negative) must be in the solution set. Therefore, the solution x < –3 is false because it tells you that only certain negative values of x are in the solution set. Throwing out this false solution leaves you with the correct answer, which is x < 1; so the correct answer is Choice (A).

Beating the System: Dealing with Systems of Equations

Much of elementary algebra focuses on solving equations that have a single variable. In contrast, in intermediate algebra you’re bound to come across problems that include more than one variable. A system of equations is a set of two or more equations that include two or more variables. Systems of equations can be useful for answering tricky word problems. In this section, I show you how and when to use systems of equations.

Solving systems of equations systematically

To solve a system of equations, you need one equation for every variable in the system. In an ACT question, this usually means two equations and two variables.

You can solve a system of linear equations in two ways:

With substitution: With this technique, you solve one equation for a variable in terms of the other(s), and then you substitute this value into the second equation.

By combining equations (elimination): To use this method, you add or subtract the two equations in such a way that one variable drops out of the resulting equation.

Both of these methods are similar in that they allow you to write a single equation in one variable, which you can then solve using your usual bag of algebra tricks. After you know the value of one variable, you can substitute this value back into one of the original two equations (usually the easier one) to get the value of the remaining variable.

Finding values with substitution

Substitution is easier to use when a variable in one equation is already isolated or when it can be isolated easily.

If x + 9 = y and 7x – 2 = 2y, what is the value of xy?

(A) 48

(B) 49

(C) 50

(D) 51

(E) 52

This question gives you two equations in two variables. In the first equation, y is already isolated on one side of the equation, so substitution should work well. Substitute x + 9 for y in the second equation:

7x – 2 = 2y

7x – 2 = 2(x + 9)

Simplify and solve:

7x – 2 = 2x + 18

5x = 20

x = 4

Now that you know the value of x, substitute this value back into the equation that looks easiest to work with — in this case, the first equation — and solve for y:

x + 9 = y

4 + 9 = y

13 = y

Thus, x = 4 and y = 13, so xy = 52. The correct answer is Choice (E).

Combining equations

The technique of combining equations is easier to use when both equations contain essentially the same term. Check out the following example to see what I mean.

If 4s + 5t = 9 and 9s + 5t = –11, what is the value of s + t?

(A) 2

(B) 1

(C) 0

(D) –1

(E) –2

Answering this question using substitution would be difficult because neither variable is very easy to isolate on one side of the equations. However, both equations include the term 5t, so you can combine the two equations using subtraction.

4s + 5t = 9

– 9s + 5t = –11

–5s = 20

When you subtract one equation from the other, the t term drops out. The resulting equation is easy to solve:

–5s = 20

s = –4

As always, when you know the value of one variable, you can substitute this value back into either equation — whichever looks easiest — and solve for the other variable, like this:

4s + 5t = 9

4(–4) + 5t = 9

–16 + 5t = 9

5t = 25

t = 5

So s = –4 and t = 5, meaning s + t = 1. As a result, the correct answer is Choice (B).

Sometimes before you can combine equations, you may need to multiply one or both of them by a certain number to get two terms to match.

If 7x + 2y = –1 and 9x – 3y = 1.5, which of the following is the value of y?

(F) 0

(G) –0.25

(H) –0.5

(J) –0.75

(K) –1.25

This system would be difficult to solve using substitution. And to combine equations, you need to make two terms look similar. The y terms have smaller coefficients (2 and –3), so they should be easier to work with. You want to multiply both of these equations by different numbers so the coefficients of the y terms become the same (disregarding their signs.) I choose the numbers 3 and 2, for reasons that will make sense in a moment:

3(7x + 2y = –1) becomes 21x + 6y = –3

2(9x – 3y = 1.5) becomes 18x – 6y = 3

Notice that the two resulting equations have y terms that look very similar — the coefficients are identical except for their sign. If you add these two equations, these similar terms drop out:

21x + 6y = –3

+ 18x – 6y = 3

39x = 0

The equation is now easy to solve:

39x = 0

x = 0

Now you can substitute 0 for x in either equation:

7x + 2y = –1

7(0) + 2y = –1

2y = –1

y = –0.5

Thus, the correct answer is Choice (H).

Working word problems using a system of equations

Solving word problems is one of the most common reasons to use a system of equations. Some word problems that would be difficult to approach using a single variable are relatively easy when you use more than one variable. This section shows you what I mean.

Dorian and Micah have been saving money from their summer jobs. If Dorian had twice as much money and Micah had half as much, together they would have $2,075. And if Micah had twice as much money and Dorian had half as much, together they would have $2,300. How much money does Dorian have?

(A) $800

(B) $850

(C) $900

(D) $950

(E) $1,000

You could solve this problem using only one variable, but that approach would be tricky and would likely lead to a mistake along the way. Instead, use two variables, letting d equal Dorian’s money and m equal Micah’s money. Set up two equations as follows:

To eliminate the fractions, multiply both of these equations by 2:

4d + m = 4,150

4m + d = 4,600

This system of equations is easy to solve using substitution. Begin by isolating m in the first equation:

m = 4,150 – 4d

Now substitute 4,150 – 4d for m in the second equation, and then solve for d:

4(4,150 – 4d) + d = 4,600

16,600 – 16d + d = 4,600

16,600 – 15d = 4,600

–15d = –12,000

d = 800

Dorian has $800, so the correct answer is Choice (A).

To solve some complicated problems, the only sensible approach is to use several different variables to set up a system of equations and then solve it.

Andie, Candie, and Sandie are all selling boxes of greeting cards to help pay for new soccer equipment for their team. On Friday night, Andie had sold as many boxes as Candie and Sandie combined. Over the weekend, each girl sold 10 boxes, so at that point Andie had sold twice as many boxes as Candie. Then on Monday, Andie and Sandie sold 5 more boxes each, bringing the final total for the three girls to 120 boxes. How many boxes did Candie sell?

(F) 10

(G) 12

(H) 15

(J) 16

(K) 20

This problem is a long and complicated one, and you may do well to skip over it to save time. If you decide to solve it, use variables and build a system of three equations.

Let a, c, and s stand for the amounts that the three girls had sold before the weekend. This problem is so complicated that a chart is helpful. Set up a chart showing how many boxes each girl had sold at the three different times:

Friday Weekend Monday

a a + 10 a + 15

c c + 10 c + 10

s s + 10 s + 15

Now use the chart to begin writing equations. On Friday, Andie had sold twice as many boxes as both Candie and Sandie combined:

a = c + s

After the weekend, Andie had sold twice as many boxes as Candie:

a + 10 = 2(c + 10)

a + 10 = 2c + 20

a = 2c + 10

On Monday, the three girls had sold 120 boxes altogether:

a + 15 + c + 10 + s + 15 = 120

a + c + s + 40 = 120

a + c + s = 80

Taken together, here’s the resulting system of equations:

a = c + s

a = 2c + 10

a + c + s = 80

To begin solving, substitute c + s for a into the second and third equations:

c + s = 2c + 10

c + s + c + s = 80

Simplify both equations:

s = c + 10

2c + 2s = 80

Now substitute c + 10 for s in the second equation:

2c + 2(c + 10) = 80

2c + 2c + 20 = 80

4c + 20 = 80

4c = 60

c = 15

Candie sold 15 boxes, so the correct answer is Choice (H).

Keeping Things in Proportion: Direct and Inverse Proportionality

Proportionality refers to a connection between two variables based on either multiplication or division. Two types of proportionality exist:

Direct proportionality: When a pair of variables is directly proportional, the variables tend to rise and fall together. That is, as one increases or decreases, the other does the same.

Inverse proportionality: When a pair of variables is inversely proportional, they tend to rise and fall separately. That is, as one increases, the other decreases, and vice versa.

In this section, I show you how to answer a variety of ACT questions that involve direct and inverse proportionality.

Maintaining a balance with direct proportions

Two variables, x and y, are directly proportional when the following equation is true for some constant k:

In practical terms, direct proportionality simply means that as the value of one variable changes, the other value also must change so that any resulting fraction remains constant.

Two variables, a and b, are directly proportional. If a = 6, then b = 18. Which of the following must be true?

(A) If a = 1, then b = 6

(B) If a = 3, then b = 9

(C) If a = 12, then b = 12

(D) If a = 18, then b = 6

(E) If a = 100, then b = 200

The fraction is a constant, and

Thus, any combination of a and b must make a fraction equivalent to . The only such combination is a = 3 and b = 9, because:

So the correct answer is Choice (B).

Two variables, x and y, are directly proportional such that if x = 3, then y = 5. What is the value of x when y = 15?

(F) 1

(G) 2

(H) 6

(J) 9

(K) 13

When x = 3 and y = 5:

Thus, any value of must also produce the fraction . Substitute 15 for y into the preceding equation:

Cross-multiply and solve for x:

5x = 45

x = 9

Therefore, the correct answer is Choice (J).

Turning things around with inverse proportions

Two variables, x and y, are inversely proportional when the following equation is true for some constant k:

xy = k

Inverse proportionality means that as the value of one variable changes, the other value must also change so that any resulting product xy remains constant.

Two variables p and q are inversely proportional, such that if p = 4, then q = 8. What is the value of q when p = 16?

(A) 1

(B) 2

(C) 4

(D) 16

(E) 32

The product pq is a constant and

pq = (4)(8) = 32

Thus, pq = 32 for all possible pairings of p and q. Substitute 16 for p into this equation:

pq = 32

16q = 32

q = 2

Therefore, the correct answer is Choice (B).

If and uv = 10, which of the following must be true?

(F) t and u are inversely proportional

(G) t and v are inversely proportional

(H) t and w are directly proportional

(J) t and w are inversely proportional

(K) u and v are directly proportional

Begin by cross-multiplying:

Substitute 10 for uv:

tw = 10

Thus, tw = k for k = 10, so t and w are inversely proportional. So the correct answer is Choice (J).

Working with Quadratic Equations and the Roots of Polynomials

A quadratic equation is a second-degree polynomial — that is, it has an x² term. Here’s the typical form of the quadratic equation:

ax² + bx + c = 0

Quadratic equations differ from the equations you work with in elementary algebra for a variety of reasons. First of all, you can’t solve a typical quadratic equation using the common method of trying to isolate x.Instead, two alternative methods for solving quadratic equations are used:

Factoring: In some cases, you can factor one side of a quadratic equation into a pair of linear factors and then split it into two separate equations. This method is usually quick, but it doesn’t always work.

The quadratic formula: In every case, you can apply the quadratic formula to solve for x. The downside is that even though the formula is effective, it’s long and unwieldy to use.

In this section, I show you how and when to use both methods for solving quadratic equations.

Try factoring first whenever a quadratic equation has relatively small coefficients and an x² term with a coefficient of 1 — for example, the equation x² + 9x + 10 = 0. When a quadratic equation has larger coefficients — and especially when it has an x² term with a coefficient that isn’t 1 — you may want to use the quadratic formula. This advice goes double if you don’t feel confident with the factoring method. And in any case where factoring simply doesn’t work, you need to use the quadratic formula.

Factoring to solve quadratic equations

A quick way to solve many simple quadratic equations — especially the ones you’re likely to find on the ACT — is factoring. When you factor a quadratic equation, you break down the quadratic into the product of two linear terms. In Algebra II class, most students spend a bunch of time developing this skill. The good news is that on the ACT, most of the problems you run across are much easier than those you face in your homework assignments.

The easiest type of quadratic to solve has a value of a = 1. In other words, it’s one whose x² term has a coefficient of 1. When working with this type of problem, factor using a pair of numbers that:

Adds up to b

Multiplies to c

The following example shows you how this method of factoring works.

If x₁ and x₂ are the two solutions to the quadratic equation x² – 6x + 8 = 0, with x₁ < x₂, then x₂ – x₁ equals what?

(A) 0

(B) 2

(C) 6

(D) 7

(E) 9

In the equation x² – 6x + 8 = 0, the x² term has a coefficient of 1, so you can set up a shell for factoring it as follows:

(x )(x ) = 0

To fill in the remaining numbers, you’re looking for a pair of integers that adds up to –6 and multiplies to 8. Begin by listing all the pairs of integers that multiply to 8, including those pairs that include negative numbers:

1 × 8 2 × 4

–1 × –8 –2 × –4

Now pick out the pair that adds up to –6:

–2 + (–4) = –6

Fill these two numbers into the empty shell you made earlier:

(x – 2)(x – 4) = 0

The good news is that if either of these factors equals 0, the whole equation is correct (because 0 multiplied by anything equals 0). So you can split this equation into two separate equations and solve both:

x – 2 = 0 x – 4 = 0

x = 2  x = 4

The question asks you to subtract the smaller number from the greater one, so x₂ – x₁ = 4 – 2 = 2; therefore, the correct answer is Choice (B).

Choosing the quadratic formula when all else fails

Factoring becomes a bit more complex when the x² term has a coefficient greater than 1. In these cases, use factoring only when all the coefficients are small. Alternatively, you can try plugging in numbers to see which ones work. Or you can use the quadratic formula.

The quadratic formula gives you the value of x for any quadratic equation of the form ax² + bx + c = 0, when you input the values of a, b, and c. Here’s the quadratic formula:

This formula produces two values, because the symbol ± stands for “plus or minus.”

Although the quadratic formula always gives the correct answer, it’s complicated and easy to make a mistake with, so use it only when you’re clear that factoring doesn’t work.

What is the sum of the values of x that satisfy the equation x² + 10x + 7 = 0?

(A) 0

(B) –10

(C)

(D)

(E)

Your first thought may be to factor x² + 10x + 7, but this proves impossible. After all, only two pair of integers multiply to 7: 1 and 7, and –1 and –7. And, as you can see, neither of these pairs adds up to 10. The only way to find the value of x is to use the quadratic formula, using the values of the coefficients, as follows:

a = 1

b = 10

c = 7

Plug these values into the quadratic formula:

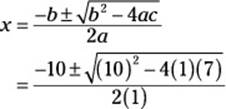
Now simplify:

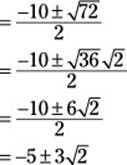
You actually have two solutions: and . Adding these two solutions together gives you:

So the correct answer is Choice (B).

Making Connections with Functions

A function is a mathematical connection between two numbers: an input (or independent variable) and an output (or dependent variable). Most commonly, a function links an input of x with an output of y. For example:

y = 3x

You can draw an input-output table to show how any input value of x determines the output value of y:

x 0 1 2 3 4 5 . . . 10 . . . 100 . . .

y 0 3 6 9 12 15 . . . 30 . . . 300 . . .

One key feature of a function is that each input value of x results in exactly one output value of y. That is, in an input-output chart, the x-values cannot be repeated, but the y-values can.

Often, the y variable is set equal to f(x). For example:

f(x) = 3x

The use of f(x) to describe a function is called functional notation, which you work with later in this chapter. The variables y and f(x) are essentially interchangeable, but y generally is used for graphing specific functions in coordinate geometry (see Chapter 8) and f(x) is more common for practical uses of functions, such as modeling real-world situations.

In this section, I provide a variety of common skills to help you answer ACT function questions quickly and correctly.

Using functions as models

Functions typically are used to model the real world — that is, to create an equation that takes the place of a complex table of numbers. A typical situation asks you to model the cost over time of an initial amount of money plus another amount paid at regular intervals.

For example, suppose you open a savings account with $1,000 and begin saving $100 a week. You could use the following table to keep track of how much money you will have saved in any particular week:

x = Week number 1 2 3 4 5 6 7

y = Dollars saved 1,100 1,200 1,300 1,400 1,500 1,600 1,700

This table is useful but limited. For example, if you want to find out how much money you’ll have after two years (104 weeks), the table is no longer practical. A more compact way to express this relationship between input value x (the week number) and the output value y (the number of dollars saved) is with the following function:

y = 100x + 1,000

Now just plug in 104 as your input value for x and solve for y:

y = 100(104) + 1,000 = 10,400 + 1,000 = 11,400

ACT questions often ask you to use a function to model a real-world situation like this one and then use it to find specific information. You’ll know you’ve come across one of these questions when you see the following type of setup for a series of questions.

A health club has a sign-up fee of $250 and a monthly charge of $40 per month. Use this information to answer the next two questions.

Which of the following functions models the cost of the health club, outputting a dollar amount (y) when you input a number of months (x)?

(A) y = 40x

(B) y = 250x

(C) y = 40x + 250

(D) y = 40x – 250

(E) y = 250x + 40

When modeling with a function, drawing a quick input-output table like the following can be helpful:

x = Number of months 1 2 3 4 5 6 7

y = Number of dollars 290 330 370 410 450 490 530

Setting up the table clarifies how to proceed in writing the function. Multiply the number of months (x) by 40 and then add 250:

y = 40x + 250

So the correct answer is Choice (C).

How much money will a 36-month membership cost?

(F) $940

(G) $1,200

(H) $1,440

(J) $1,690

(K) $9,400

Use the function y = 40x + 250, which you created in the previous example question, to answer this one. Substitute 36 for x and solve for y:

y = 40(36) + 250 = 1,440 + 250 = 1,690

Therefore, the correct answer is Choice (J).

Be careful when an initial cost helps to cover one or more payments — you may have to subtract a number from x before multiplying. The next example shows you how this type of problem works.

A personal storage facility is offering a special deal for new customers: The first three months costs a total of $10, and then each subsequent month costs $50. Which of the following functions models the monthly cost of this facility for all input values of three or more months?

(A) f(x) = 10x + 50

(B) f(x) = 50x + 10

(C) f(x) = 50x + 30

(D) f(x) = 50x – 140

(E) f(x) = 50x – 150

Notice that this example uses f(x) rather than y, but the meaning is the same. In this case, the input variable x is the number of months, and the output variable f(x) is the dollar amount. You may be tempted to write this answer:

f(x) = 50x + 10 Wrong!

Unfortunately, this answer is too simple, because it doesn’t account for the fact that the initial cost of $10 covers the first three months. So you can subtract 3 months from the input value before you multiply this number by 50:

f(x) = 50(x – 3) + 10

Simplify:

f(x) = 50x – 150 + 10

f(x) = 50x – 140

This answer makes sense when you think about it: Instead of paying the normal rate of $50 a month for the first three months, which would be $150, you only pay $10 total. So you’re saving $140, which you deduct from whatever you end up paying. Therefore, the correct answer is Choice (D).

Defining relationships with functional notation and evaluation

Functional notation is a compact way to define the relationship between an input variable and an output variable. Typically, the input variable in a function is x and the output variable is f(x). For example:

f(x) = x² + 1

You can substitute any number for x and then evaluate the right side of the function as a value. For example, here’s how you find the value f(5) and f(10):

f(5) = 5² + 1 = 25 + 1 = 26

f(10) = 10² + 1 = 100 + 1 = 101

Thus, f(5) = 26 and f(10) = 101.

What is the value of f(8) – f(3) for the function f(x) = x² – 6?

(A) 55

(B) 58

(C) 64

(D) 72

(E) 73

First find the value of f(8) and f(3):

f(8) = 8² – 6 = 64 – 6 = 58

f(3) = 3² – 6 = 9 – 6 = 3

After you discover that f(8) = 58 and f(3) = 3, you have to do the subtraction:

f(8) – f(3) = 58 – 3 = 55

Therefore, the correct answer is Choice (A).

In some cases, an ACT question may include more than one function to evaluate. Consider the following example:

If f(x) = 5x + 4 and g(x) = 2x², what is the value of ?

(F) 2

(G) 3

(H) 4

(J) 6

(K) 8

First evaluate f(10) and g(3):

f(10) = 5(10) + 4 = 54

g(3) = 2x² = 2(3)² = 2(9) = 18

Now that you know that f(10) = 54 and g(3) = 18, you can simplify:

So the correct answer is Choice (G).

Getting to know domain and range

The domain of a function is the set of values that can be inputted into that function (that is, the x values). The range of a function is the set of values that the function can output (that is, the y values). In this section, you discover how to find the domain and range for a variety of functions.

Arriving at the domain event

On the ACT, questions involving the domain of a function usually require you to rule out input values of x that would cause problems. The two main problems to watch out for are the following:

The denominator of a fraction can’t equal 0.

The value inside a square root (radical) can’t be a negative number.

If any value of x causes either of these two problems, that value can’t be in the domain. When working with fractions, usually only a limited number of values are left out of the domain, as in the following example.

Which of the following values of x is NOT in the domain of ?

(A) 0

(B) –1

(C) 2

(D) –4

(E) 5

Any value of x that causes the denominator of the fraction to equal 0 can’t be in the domain. To find any problematic values, set the denominator equal to 0 and solve for x:

2x – 10 = 0

2x = 10

x = 5

Because 5 can’t be in the domain, the correct answer is Choice (E).

The domain of a function must exclude all values in which a negative number appears inside a square root. (However, the value 0 inside a square root is acceptable as long as it’s not the denominator of a fraction.) Typically, the domain of a function that includes a square root is expressed as an inequality.

Which of the following is the domain of the function ?

(F) x > 3

(G) x ≥ 3

(H) x ≤ 3

(J) x < –3

(K) x ≥ –3

The domain of the function can’t include a negative number inside the square root. Therefore, the value inside the square root is either greater than or equal to 0. You can set up an inequality that looks like this:

3x – 9 ≥ 0

Now simplify:

3x ≥ 9

x ≥ 3

The correct answer is Choice (G).

Feeling at home with the range

ACT questions involving the range usually focus on functions that input non-negative values. These fall into two main categories:

Squares (and even-numbered powers): For example, no matter what value of x you input, the function f(x) = x² is never negative. So the range of f(x) ≥ 0.

Absolute value: For example, the function f(x) = |x| can’t be negative either, so the range of f(x) ≥ 0.

Use these basic principles to think through an ACT question about the range of a function. For example, the range of f(x) = –x² is f(x) ≤ 0, because x² is always non-negative, and then the minus sign negates the function. Similarly, the range of f(x) = |x| + 3 is f(x) ≥ 3, because the minimum value of |x| is 0, and then 3 is added. However, the range of f(x) = |x + 3| is f(x) ≥ 0, because the entire function is an absolute value, whose value can never fall below 0.

Which of the following has a range of f(x) ≥ –2?

(A) f(x) = |x – 2|

(B) f(x) = (x + 2)²

(C) f(x) = |x| + 2

(D) f(x) = |x| – 2

(E) f(x) = 2 – |x|

First, because any function with range of f(x) ≥ –2 produces some negative values, you can rule out a few answers. A function that’s entirely an absolute value can never produce a negative value, so Choice (A) is out. Similarly, a function that’s entirely squared can never produce a negative value, so Choice (B) is also ruled out.

The lowest possible value for |x| is 0, so the lowest possible value for both f(x) = |x| + 2 and f(x) = 2 – |x| is 2; therefore, you can rule out Choices (C) and (E). By process of elimination, you know that the correct answer is Choice (D). To check, consider this: If x = 0, then f(x) = |x| – 2 = 0 – 2 = –2, and this is the lowest possible value for this function.