High School Algebra I Unlocked (2016)

Lesson 6.3. Quadratic Inequalities

Thankfully, quadratic inequalities are tackled in much the same way as quadratic equations: you can determine the solutions to a quadratic inequality by factoring, completing the square, graphing, or using the quadratic formula. The main difference, however, is that you will use the skills that you learned in Chapter 5 to solve linear inequalities. More specifically, when graphing a quadratic inequality, you will need to shade the appropriate side of the inequality line in the coordinate plane. Additionally, when solving quadratic inequalities, replace the inequality sign with an equals sign, identify the solutions, and then test numbers in the original inequality. Let’s see how this works.

Remember inequalities?
We covered linear
equalities in Chapter 5.
Turn back to Lesson 5.3
if you need a refresher.

This question is slightly different than the other questions we’ve looked at in this chapter. Instead of looking for the roots of the quadratic expression, the question asks you to find a possible value of k in the expression x² + kx + 12, given that k is negative.

Since you’re told that k is negative, you can eliminate (D), 7, as it’s a positive number. Furthermore, based on the rules of quadratics, you should know that both factors of the quadratic x² + kx + 12 will be negative, as the c-term is positive and the b-term is negative. Therefore, make your factor chart as follows:

Thus, based on the factor chart, the value of k could be −7, −8, or −13. However, the only value of k that appears in the answer choices is −13. The correct answer is (A).

EXAMPLE

Solve and graph the quadratic inequality x² − 7x + 12 ≥ y.

We’re given the inequality x² − 7x + 12 ≥ y. Start by replacing the inequality sign with an equals sign, and solve for x by factoring.

x² − 7x + 12 ≥ y

x² − 7x + 12 = 0

Next, find factors of the c-term, 12, that add up to the b-term, which is −7. In this scenario, the b-term is negative and the c-term is positive, so both factors will be negative. Make a chart like the following:

In the chart, the only factors of the c-term, 12, that add up to the b-term, −7, are −3 and −4. Thus, you can factor the quadratic x² − 7x + 12 as (x − 3)(x − 4). So, based on the zero product property, the roots of the quadratic inequality x² − 7x + 12 ≥ y are x = 4 and x = 3.

You can also find the vertex (h, k) for any quadratic inequality in the form ax² + bx + c with the formula (h, k) = 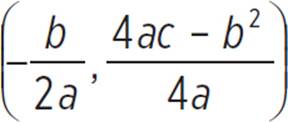. Therefore, the vertex (h, k) of the inequality x² − 7x + 12 ≥ y, where a = 1, b = −7, and c = 12 is as follows:

Note: If you find
coordinates that are not
whole numbers, it is
acceptable to express your
answer in either fraction
or decimal form, unless
the question specifies
otherwise. When graphing
such coordinates, you
may find it easier to
use the decimal form
to better visualize the
points you’re plotting.

Next, graph the inequality:

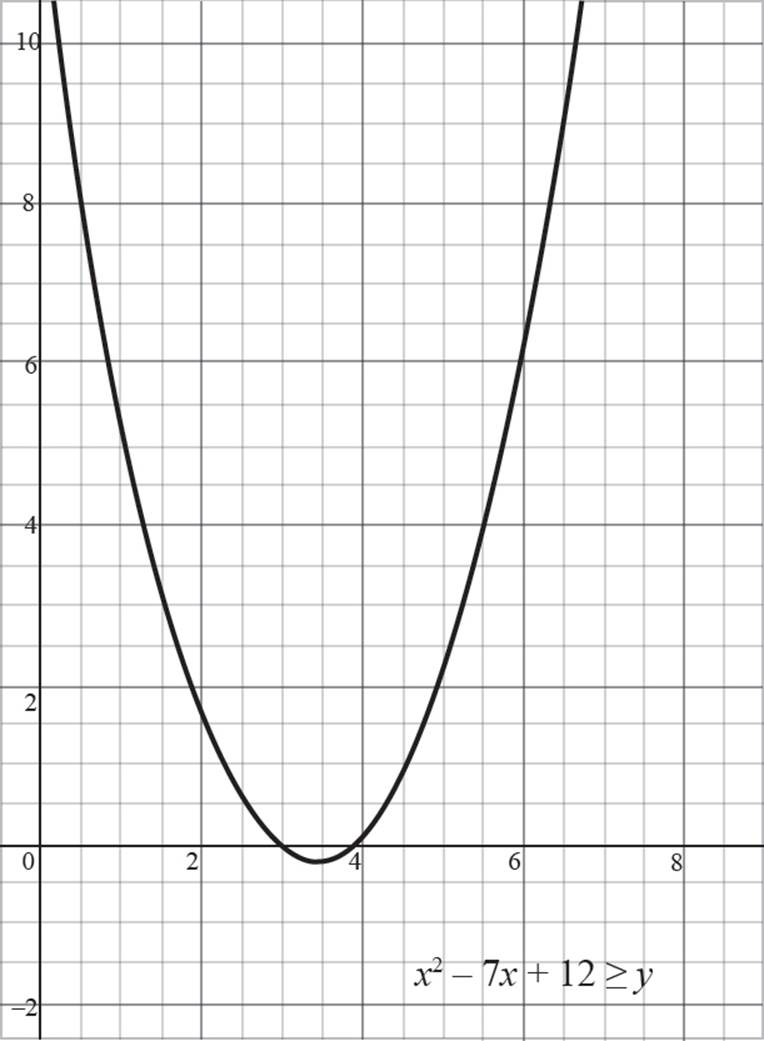
Finally, determine which area of the graph to shade. Select a test point that falls either inside or outside of the parabola and see if the original inequality holds true. Since the origin does not fall on the parabola, test the point (0, 0) in the original inequality, x² − 7x + 12 ≥ y.

0² − 7(0) + 12 ≥ 0

 0 − 0 + 12 ≥ 0

   12 ≥ 0

Since 12 ≥ 0, the statement is true, and the area containing the origin should be shaded as follows:

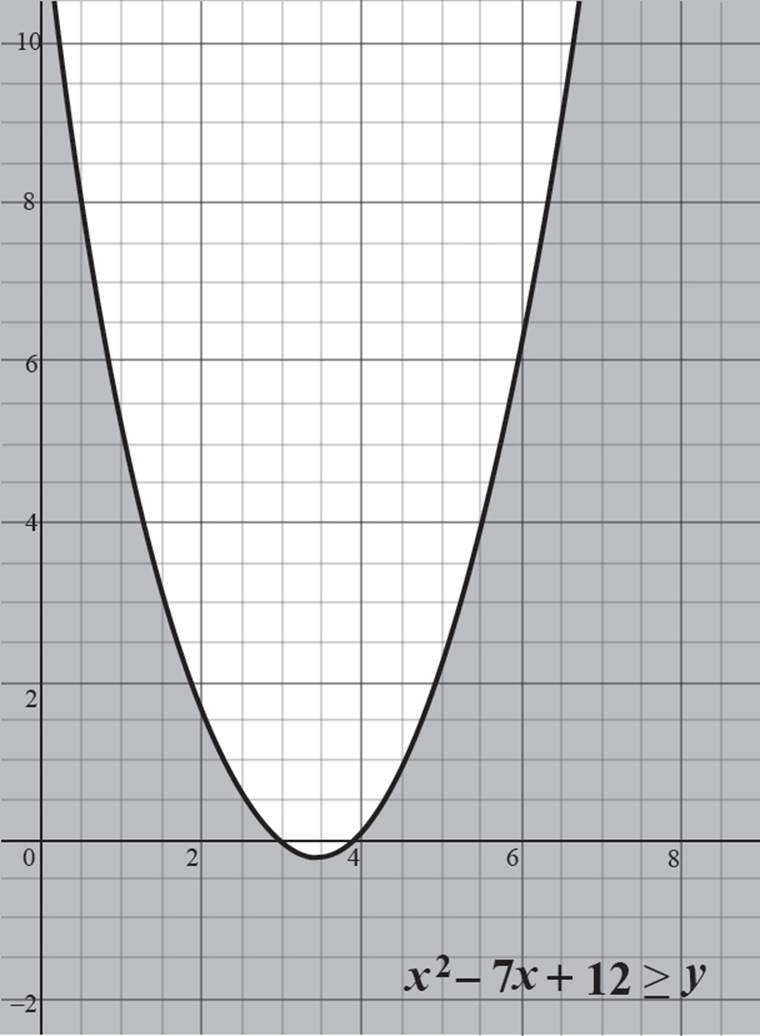
Let’s try one more quadratic inequality question before moving on to the next topic.

EXAMPLE

Solve and graph the quadratic inequality y < −x² + 5x − 6.

In this question, you’re given the inequality y < −x² + 5x − 6, so replace the inequality sign with an equals sign and solve for x.

y < −x² + 5x − 6

y = −x² + 5x − 6

Because the standard form of a quadratic expression is ax² + bx + c, we know that a = −1, b = 5, and c = −6. Plug these values into the quadratic equation and simplify:

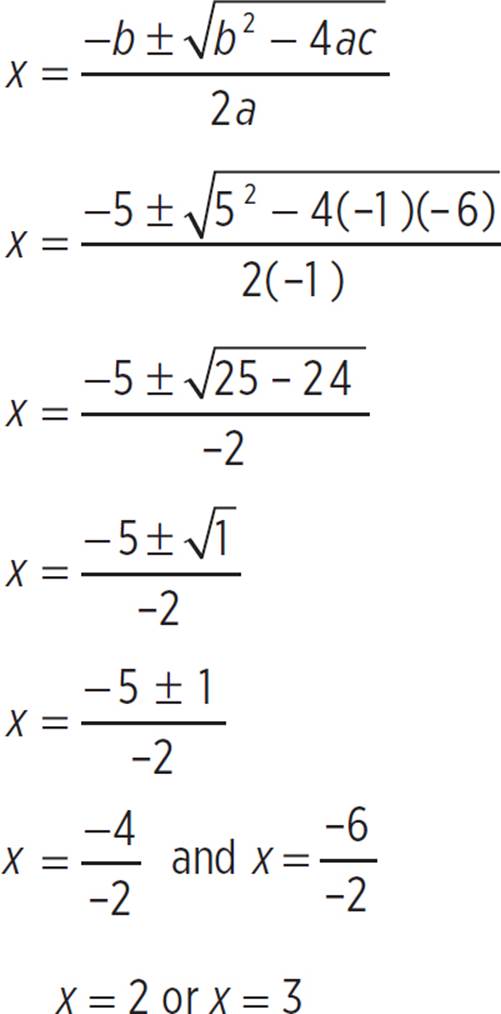
Now that you’ve found that the roots of the quadratic inequality y < −x² + 5x − 6 are x = 2 or x = 3, find the vertex of the quadratic with the formula (h, k) = 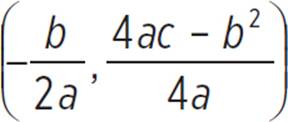. Thus, the vertex (h, k) of the inequality y < −x² + 5x − 6, where a = −1, b = 5, and c = −6:

Now that you have the roots and the vertex, graph the inequality, using a dashed boundary line because of the less than inequality sign.

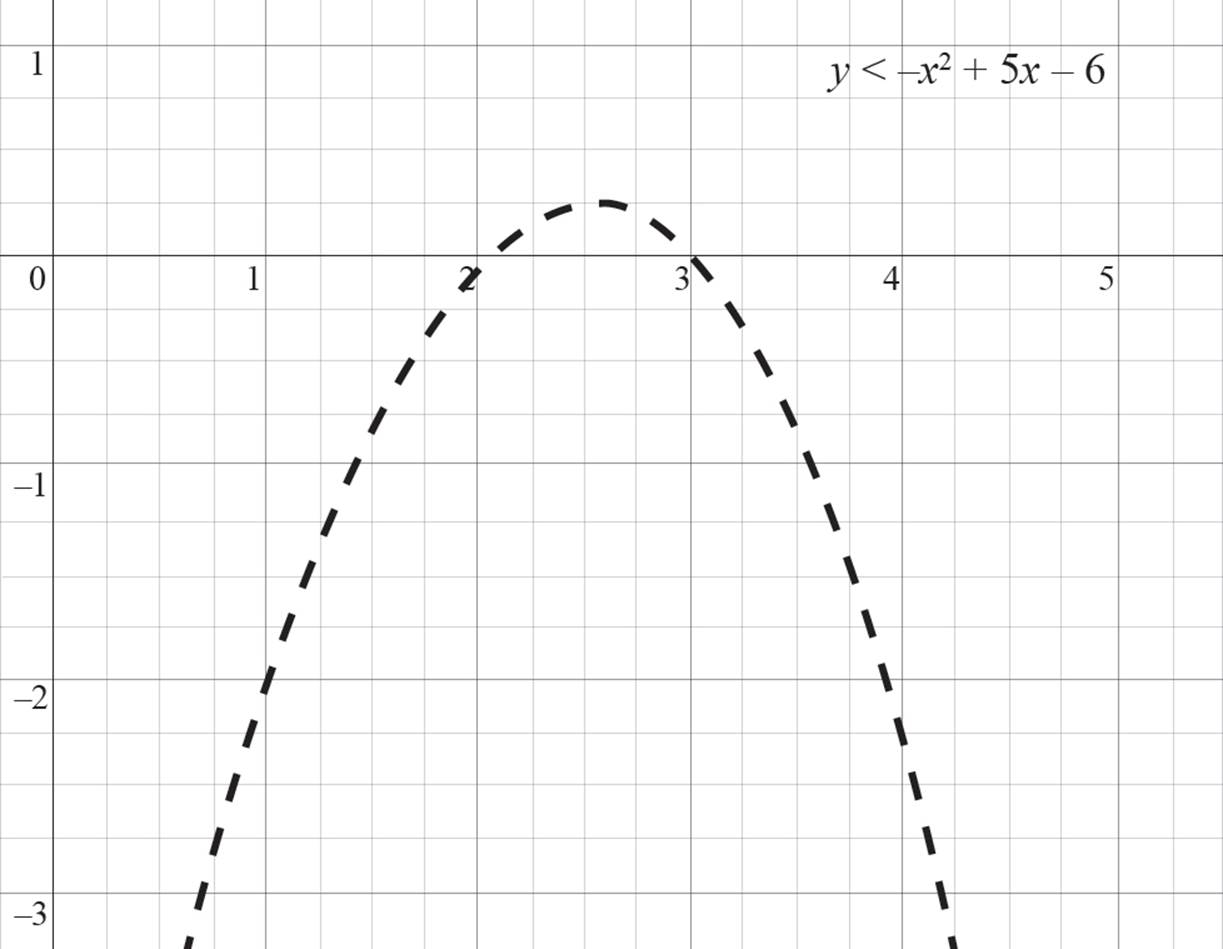
Finally, you need to determine which side of the parabola to shade by selecting a test point that falls either inside or outside of the parabola and determining if the original inequality holds true. Since the origin does not fall on the parabola, test the point (0, 0) in the original inequality, y < −x² + 5x − 6.

y < −x² + 5x − 6

0 < −0² + 5(0) − 6

0 < 0 + 0 − 6 

0 < −6   

Since 0 is not less than −6, the statement is false, and the area on the side of the boundary line that does not contain the origin should be shaded:

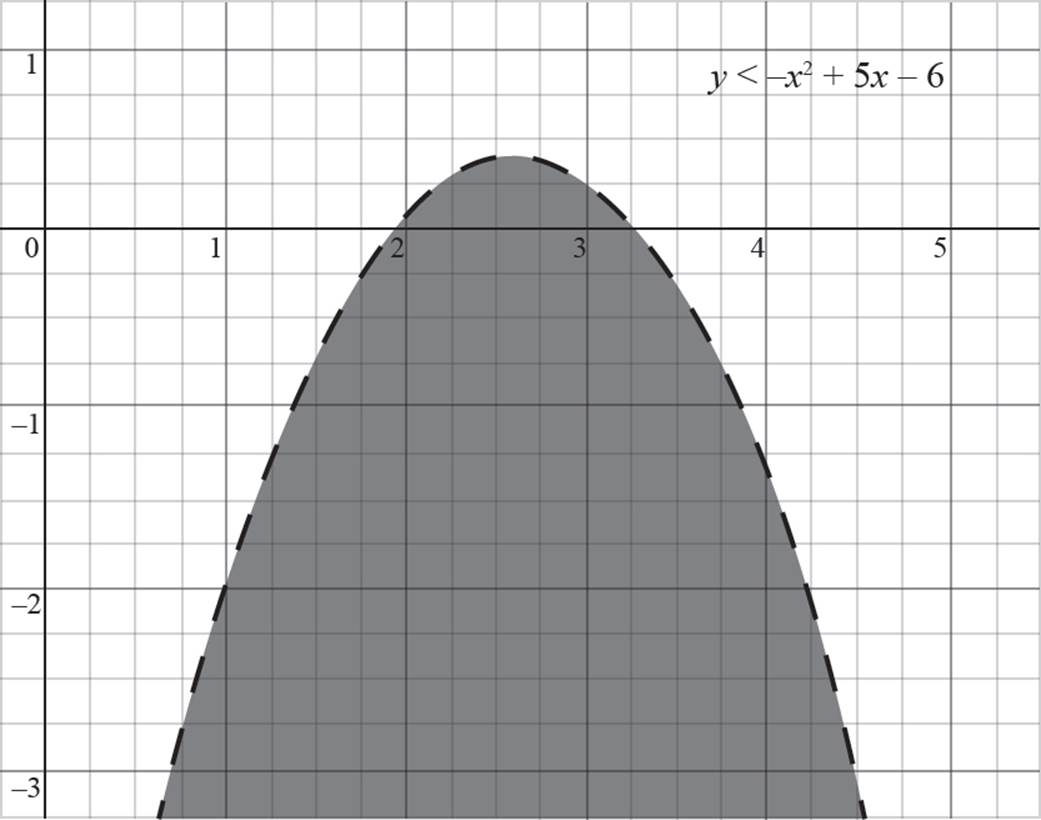
And you’re done! You’ve successfully solved the quadratic inequality!

Success! You’ve now applied what you know about linear inequalities in order to solve quadratic inequalities. Now let’s see how we handle systems of quadratic equations.