High School Algebra I Unlocked (2016)

Lesson 1.2. Mathematical Properties

Think back to our discussion about language development. In the same way that there are rules for spoken language, there are rules when working with the language of math. The correct way to perform mathematical operations can be summed up with a series of mathematical properties.

While you won’t need to memorize the precise definitions that follow, you should be familiar with how each property works. Once you have a solid grasp of these properties, you’ll be able to tackle many basic mathematical operations with ease. Perhaps the three most important number properties to remember are the associative, commutative, and distributive properties.

The Associative Property

The associative property focuses on groups. In math, you can add or multiply terms regardless of the way they are grouped within parentheses—this is the gist of the associative property.

This ACT question requires you to evaluate the five expressions given in the answer choices. The question states that p is an odd integer, or a whole number, and asks you to identify the expression that will also result in an odd integer. In order to determine if the expressions are odd, let’s set p = 3, and substitute p = 3 into the answer choices. When we do this for (A), we find that p + 1 = 3 + 1 = 4; this is even, so eliminate (A). Substituting p = 3 for (B), we find that p/2 = 3/2; this is not an integer, so eliminate (B). For (C), we find that p + 2 = 3 + 2 = 5; this is odd, so keep (C). Choice (D), 2p, becomes 2(3) = 6; this is even, so eliminate (D). Finally, substituting into (E), p − 1 results in 3 − 1 = 2; this is also even and can be eliminated. Thus, the only expression that results in an odd integer is (C).

Let’s see how the associative property works with both addition and multiplication.

In these examples, you should notice that the grouping of terms within parentheses does not affect the answer. Whenever you add or multiply a string of numbers together, the solution will be identical regardless of the placement of parentheses. This is the associative property in action!

The Commutative Property

Maybe you’ve heard someone complain about his or her long commute to work or school. Just as a commute involves movement, the commutative property in math involves the movement of terms.

The commutative property works ONLY with addition and multiplication. Don’t try to make it work with subtraction or division—it’s impossible!

If you an encounter a question that refers to the commutative property, chances are you’ll need to move, or rearrange, the terms in an equation. In short, the commutative property states that you can add or multiply a string of numbers in any order you wish.

According to the commutative property, order of the terms does not matter when adding or multiplying a series of numbers. Notice that there are five other ways to rearrange the expressions a + b + c and a × b × c, but all of the new arrangements are equal to the original expression. Pretty cool, right? Let’s see how this works with numbers.

As you probably noticed, we get the same result with numbers as we did with variables; the order of terms does not affect the solution when adding or multiplying a string of numbers together.

On a test, it’s unlikely you’ll be asked to list out all of the possible arrangements—especially if you’re dealing with more than three terms. Still, you should be familiar with how the property works: When multiplying or adding numbers, order doesn’t matter.

The Distributive Property

In math, the distributive property focuses on the way a term is either applied to, or factored out of, an expression. This property describes the methodical process of applying or factoring a term to all items in an expression.

For example, if you’ve ever handed out invitations to a party or some other event, you know that you distribute an invitation to each guest in some kind of methodical order. You would probably have a list of guests, and you would give each of them a single invitation. You wouldn’t give one guest three invitations and another guest zero invitations. This idea of “handing out to all equally” is the basic principle behind the distributive property. Distributing terms in an expression is a lot like handing out invitations; both are done in a methodical order.

Distributive Multiplication

When working with multiplication problems, you apply a term outside of a parenthetical expression in a methodical order. Let’s see how you perform distributive multiplication using the expression a(b + c). Here’s the step-by-step breakdown:

Distributing numbers works in the exact same way as distributing variables. In the following example, we distribute the number 2 to both terms, 3 and 4, within the parenthetical expression.

2(3 + 4) = (2 × 3) + (2 × 4)
2 × 7 = 6 + 8
14 = 14

As long as you distribute terms in a logical, methodical order, you will be in good shape.

Let’s see how we might use the distributive property in a real-world situation. Imagine that you want to purchase a video game that costs $50 and wireless controller that costs $45. When you go to pay the cashier, there is a sales tax of 7% applied. What is the total sales tax collected? The following table will show you the two different ways you can find the total sales tax: either by distributing the tax amount to each item and then finding the sum of the items, or by finding the sum of the items and then distributing the tax amount.

Again, regardless of how we distribute the tax, the answer is the same.

Now, what if you want to find out how much money, including tax, you owe the cashier? Again, use the distributive property to determine the total amount of money you need, including tax, to purchase the video game and controller.

Same result here: We find the same total, regardless of whether we distribute first and sum the items second, or if we sum the items first and distribute second.

Distributive Factoring

Distributive factoring is the reverse process of distributive multiplication. Instead of multiplying a term by those within parentheses, you factor out a common factor and create a parenthetical expression. Take a look at the following example.

a² − 4a =

Let’s try another example.

4a + 12 =

The Associative, Commutative, and Distributive Properties in Action

At some point in your educational career, you will probably be asked to construct a proof. In math, a proof is a deductive argument for a mathematical statement that uses established statements and theorems to support a logical conclusion. That definition might sound complicated and intimidating, but in practice, proofs are actually quite simple. Let’s try one together.

Simplify 10x + 4 + 4y − 2x + 12 + y. Justify your steps.

Set up a table to list out each step and the justification for each one:

By using mathematical properties, you can simplify mathematical expressions—and provide proof that you are doing the math correctly!

Here is how you may see distributive factoring on the SAT.

Which of the following is equivalent to 2x² − 6x − 8 ?

A) 2(x − 4)(x + 1)

B) 3(x + 4)(x − 1)

C) 2(x − 3)(x + 2)

D) 3(x − 4)(x − 1)

Other Mathematical Properties

If we were to get into the nitty-gritty of math, there would be more mathematical number properties than could fill this book. Luckily, however, you don’t need to know every math rule ever invented. Some of these more obscure properties are defined in the following table. Don’t worry about memorizing them. Rather, review them, make sure you understand the implications of the rules, and be able to apply the rules to the math questions you encounter.

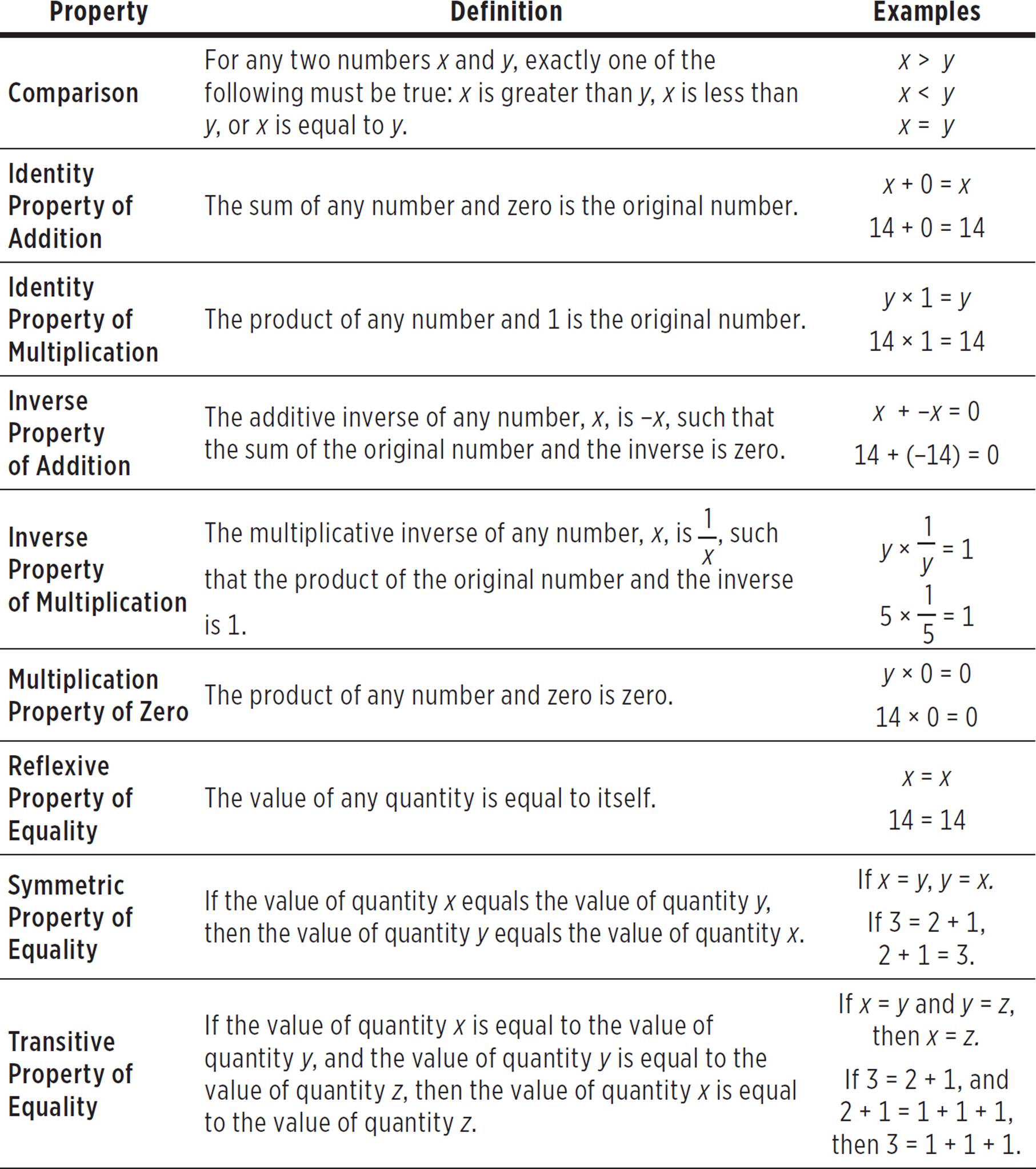
If these properties are a bit overwhelming, don’t worry. It’s actually very likely that you’ve used them before without knowing it. That said, if you encounter a question that refers to a specific property and you don’t remember what the property does, you can always refer to the previous table for help.