Master AP Calculus AB & BC
Part II. AP CALCULUS AB & BC REVIEW
CHAPTER 4. Differentiating
OVERVIEW
• Derivative as a rate of change
• The power rule
• Derivatives to memorize
• The chain rule
• The product rule
• The quotient rule
• A word about respecting variables
• Implicit differentiation
• Hands-On Activity 4.1: Approximating derivatives
• Technology: Finding numerical derivatives with the graphing calculator
• Summing it up
The study of limits and continuity only sets the stage for the first meaty topic of calculus: derivatives and differentiation. In fact, more than half of the questions on the AP test will involve derivatives in some way or another. Unlike limits, however, finding derivatives is an almost mechanical process full of rules and guidelines, which some students find a relief. Other students don’t find any relief in any topic of calculus; these people have awful nightmares in which monsters corner them and force them to evaluate limits while poking them with spears.
DERIVATIVE AS A RATE OF CHANGE
The derivative of a function describes how fast and in what capacity a function is changing at any instant. You already know a bit about derivatives, though you may not know it. Consider the graph of y = 2x — 1. At every point on the line, the graph is changing at a rate of 2. This is such an important characteristic of a line that it has its own nifty term—slope. Because a slope describes the rate of change of a linear equation, the derivative of any linear equation is its slope. In the case of y = 2x — 1, we write y' = 2.
NOTE. Differentiation is the process of taking derivatives. If a function is differentiable, then it has derivatives.
Not all derivatives are so easy, however. You’ll need to know the definition of the derivative, also called the difference quotient. The derivative of a function f(x) is Differentiation is the process defined as this limit:
![]()
Example 1: Use the difference quotient to verify that dy/dx = 2 if y = 2x — 1.
NOTE. The notations y', dy/dx’, and Dx all mean the derivative of y with respect to x. Don’t concern yourself too much with what “with respect to x” actually means yet.
Solution: Set f(x) = 2x — 1, and apply the difference quotient. You need to substitute (x + ∆x) into the function, subtract f(x), and then divide the whole thing by ∆x.
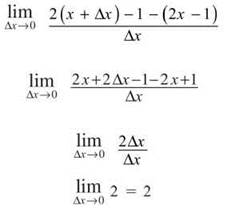
That wasn’t so bad, now was it? Well, bad news—it gets worse. What about the graph y = x2 + 2? That graph changes throughout its domain at different rates. In fact, when x < 0, the graph is decreasing, so its rate of change will have to be negative. However, when x > 0, the graph increases, meaning that its rate of change will need to be positive. Furthermore, can you discuss “slope” if the graph in question isn’t a line? Yes. Derivatives allow us to discuss the slopes of curves, and we do so by examining tangent lines to those curves. The diagram below shows y = x2 + 2 and three of its tangent lines.
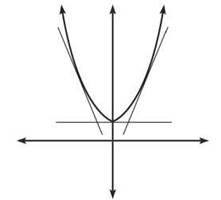
The graph of y = x2 + 1 and some tangent lines.
TIP. The difference quotient has an alternative form for finding derivatives at a specific value x = c: f'(c) = ![]()
Geometrically, the derivative of a curve at a point is the slope of its tangent line there. In the diagram above, it appears that the tangent line is horizontal when x = 0 and thus has slope zero. Is it true, then, that y'(0) = 0? You can use the alternate form of the difference quotient (given in the preceding margin note) to find out.
Example 2: Prove that y'(0) = 0 if y = x2 + 1 using the difference quotient.
Solution: Set f(x) = x2 + 1. In this problem, you are finding y'(0), so c = 0; therefore, f(c) = f(0) = 1:
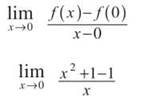
![]() (by substitution)
(by substitution)
Sometimes, the AP test will ask you to find the average rate of change for a function. This is not the same thing as a derivative. The derivative is the instantaneous rate of change of a function and is represented by the slope of the tangent line. Average rate of change gives a rate over a period of time and is represented geometrically by the slope of a secant line. The next example demonstrates the difference.
Example 3: Given the function f(x) defined by the graph below is continuous on [a,b], put the following values in order from least to greatest: f'(d), f’(e), f'(h), average rate of change off on [a,b].
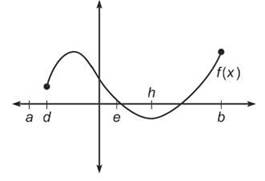
Solution: The function is not given, so you cannot use the difference quotient. Remember that derivatives are represented by slopes of tangent lines to the graph (drawn below), and average rate of change is given by the slope of the secant line (drawn below as a dotted line).

The tangent line at x = e has the only negative slope, so it is ranked first. Of the remaining lines, you can tell which slope is greatest according to which line is the steepest. Thus, the final ranked order is f'(e), f'(h), average rate of change, f'(d).
By now, you have an idea of what a derivative is (a rate of change), how it gets its value (the difference quotient), and what it looks like (the slope of a tangent line). As was the case with limits, there are times when a derivative does not exist. No derivative will exist on f(x) at x = c when any of the following three things happen:
• The graph of/has a sharp point (cusp) at x = c;
• f is discontinuous at x = c;
• The tangent line to f at x = c is vertical.
Conditions under which f'(c) does not exist.

A sharp point exists at x= c.
In the first graph above, two linear segments meet at a sharp point at x = c. The slope of the left segment is positive, while the slope of the second is negative. The derivative will change suddenly and without warning (“Stand back, my derivative is about to change! Seek cover!”) at x = c, and thus, it is said that no derivative exists. The same is true of the case of discontinuity at x = c in the diagram. You will justify the nonexistence of a derivative at a vertical tangent line in the problem set.
The final fact you need to know before we get our feet wet finding actual derivatives without the big, bulky difference quotient is this important fact: If f(x) is a differentiable function (in other words, f has derivatives everywhere), then f is a continuous function. (Remember from the discussion above that if a function is discontinuous, then it does not have a derivative.) However, the converse is not true: continuous functions do not necessarily have derivatives! Explore this further in the proceeding exercises.
EXERCISE 1
Directions: Solve each of the following problems. Decide which is the best of the choices given and indicate your responses in the book.
DO NOT USE A GRAPHING CALCULATOR FOR ANY OF THESE PROBLEMS.
1. Which function, among those you are to have memorized, is continuous but not differentiable on (—∞,∞)?
2. What is the average rate of change of g(x) = sin x on ![]()
3. Knowing that the derivative is the slope of a tangent line to a graph, answer the following questions about p(x) based on its graph.
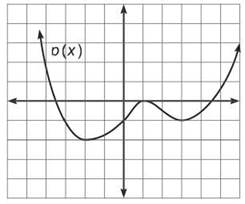
(a) Where is p' (x) = 0?
(b) Where is p' (x) > 0?
(c) Where is p' (x) < 0?
(d) Where is p' (x) undefined?
4. Why does no derivative exist when the corresponding tangent line is vertical?
5. Set up, but do not evaluate, an expression that represents the derivative of g(x) = esc x if x = π/4.
ANSWERS AND EXPLANATIONS
1. y = lxl is continuous but not differentiable at x = 0, because its graph makes a sharp point there.
2. The average rate of change is the slope of the line segment from ![]() to
to ![]() as shown in the diagram below.
as shown in the diagram below.

To find slope, calculate the change in y divided by the change in x:
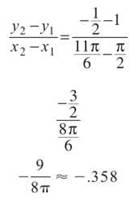
TIP. Notice in 3(b) and 3(c) that f'(x) is positive when f(x) is increasing, and f'(x) is negative when f(x) is decreasing. More on this later—stay tuned.
3. (a) The derivative is zero when the tangent line is horizontal. This happens at x = —2, 1, and 3.
(b) If you draw tangent lines throughout the interval, their slopes will be positive on the intervals (—2,1) and (3,∞).
(c) Again, drawing tangent lines on the graph shows tangents with negative slope on (—∞,—2) and (1,3).
(d) There are no cusps, discontinuities, or vertical tangent lines, so the derivative is defined everywhere.
4. The slope of a vertical line does not exist, and since the derivative is equal to the slope of a tangent line, the derivative cannot exist either.
5. Use either form of the difference quotient to get one of the following:
