Master AP Calculus AB & BC
Part II. AP CALCULUS AB & BC REVIEW
CHAPTER 4. Differentiating
TECHNOLOGY: FINDING NUMERICAL DERIVATIVES WITH THE GRAPHING CALCULATOR
If you are a studious person and a friend of the environment, you have already read Chapter 1, which discusses when you may and may not use a graphing calculator to justify an answer. You should already know how to graph using the calculator, and you learned how to solve equations using the calculator in Chapter 2. That leaves two topics, and one of these is calculating a numerical derivative.
The TI-83 can evaluate a derivative at any point on its domain (cue bugle fanfare). Unfortunately, it will not actually take the symbolic derivative (cue booing from studio audience). For example, you cannot type d/dx (tan x) and expect the calculator to respond “sec2x” (although some calculators, such as the TI-89, do have this capability). The numerical differentiation function is called “nDeriv” and is found under the [Math] button, option Number 8. To learn this technique, let’s revisit an old friend: problem 1 in the Quotient Rule section.
Example 19: Find f'(1) if 
Solution: The correct syntax for a numerical derivative is
nDeriv (function of x, x, number at which to find derivative).
Be careful to type the function correctly; this one will require a whole bunch of parentheses.
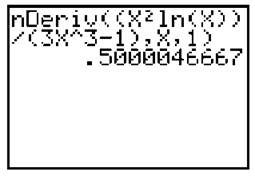
When you did this problem by hand, you got an answer of 1/2. Note that the calculator’s nDeriv result is not the exact answer, as the calculator only approximates it, although it is a pretty darn good approximation. Since the AP test only requires three-decimal accuracy, a truncated or rounded answer of .500 would have been fine, but the complete calculator answer may have been marked incorrect, since the actual answer is 1/2 and not .5000046667. Always take your calculator’s solutions with a grain of salt; they’re usually close but not always right.
NOTE. The cube root symbol is found in the [Math] menu on the TI-83, and the absolute value notation “abs” is found in the [Math] → “Num” menu.
Example 20: Use the calculator to find the following numerical derivatives:
(a) h’(0) if 
When you type the nDeriv command, you get a very bizzare result. I got 300, as shown below.
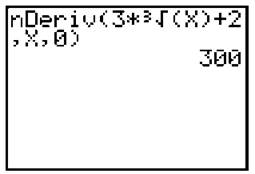
You should check this by hand, because it just feels wrong. You can rewrite h as h(x) =3x1/3 + 2, so 
Clearly, then, h'(0) is undefined, and the derivative does not exist. (This is because h has a vertical tangent line at x = 0, and no derivatives exist at vertical tangent lines.) The calculator has unintentionally lied.
(b) g'(3) if g(x) = lx — 3l — 1.
Below is the graph of g and the nDeriv command required to find g'(0).
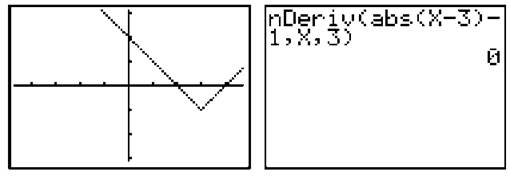
Does something about these images make you uncomfortable? g has a sharp point at (3,-1), but the calculator reports that the derivative is 0. You know better than that—no derivative exists at a sharp point, so the correct answer is g'(3) does not exist.
These examples illustrate a very important point. The calculator is a great estimation tool, but it cannot always replace good knowledge of where derivatives exist and how to compute them. Be aware of the calculator’s limitations, and don’t accept the calculator outputs as gospel truth.
EXERCISE 10
Directions: Solve each of the following problems. Decide which is the best of the choices given and indicate your responses in the book.
YOU MAY USE A GRAPHING CALCULATOR ONLY FOR PROBLEMS 5 THROUGH 12.
1. Find dy/dx: y = sin x cos √x.
2. Find d/dx (sec 3x ln(tan x)).
3. Determine the value of dy/dx if x = 0 for exy + cos y = 1.
4. Write the equation of the tangent line to 
at x = 0.
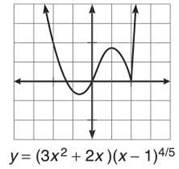
5. At what x-values does (3x2 + 2x)(x — 1)4/5 have horizontal tangent lines? On what intervals is the function differentiable?
6. Describe t(x) as completely as you can based on its graph, shown below.
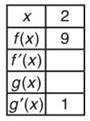
7. If n(x) = (g(x))2 and p(x) = f(x)g(x), complete the chart below knowing that n'(2) = —6 and p’(2) = —3.
8. If b(x) has the graph shown below with points of interest x1 and x2,
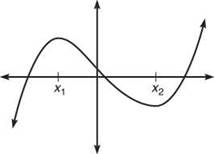
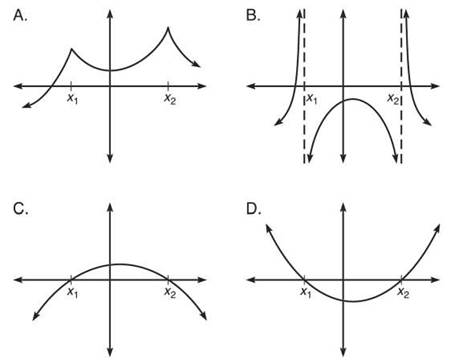
which of the following could be the graph of b'(x)?
9. If f(1) = 1, f'(1) = -3, g(1) = 4, and g'(1) = -1/2, find
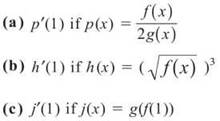
10. If m(x) is an even function differentiable at x = 4 and m'(4) = 0, determine which of the following statements must be true and briefly justify your answers.
(a) m is continuous at x = 4.
(b) 
exists.
(c) x = 4 is a root of m(x).
(d) m cannot have a point discontinuity at x = 4.
(e) m’(0) = 4.
(f) m'(4) = m'(—4).
(g) The average rate of change of m(x) on [—4,4] = 0.
11. Given that the following graph represents an audience member’s heart rate (in beats per minute) for the last 16 minutes of the new horror flick The Bloodening (starring Whoopi Goldberg and Lassie), approximate the following values and explain what they represent.
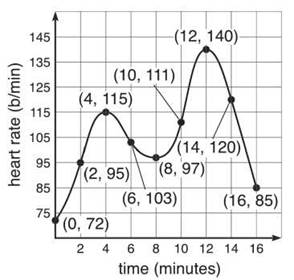
(a) h(9)
(b) h’(6)
(c) 
12. James’ Diabolical Challenge: A particle moves along the x-axis such that its distance away from the origin is given by s(t) = t3 — 9t2 + 24t — 7 (where t is in seconds and s(t) is in millimeters).
(a) Find the average velocity of the particle on [1,3].
(b) How quickly is the particle moving at t = 3, and in what direction is it moving?
(c) When is the particle at rest?
(d) Graph s'(t) and give the intervals of time during which the particle is moving to the right.
ANSWERS AND EXPLANATIONS
1. The Product Rule should be your first plan of attack (the Chain Rule will follow with cos √x):
2. This problem is very similar to the last:
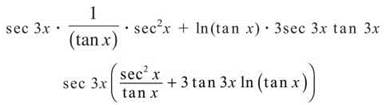
3. First, find the y that corresponds to x = 0:
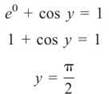
Now, use implicit differentiation, but remember to apply the Product Rule to xy:
Finally, plug in (0,π/2) and solve for dy/dx:
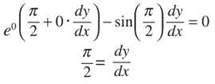
4. Clearly, the tangent line will intersect the curve at (0,f(0)), or (0,—2/5). Use the Quotient Rule to find the slope of the tangent line:
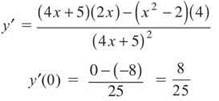
Therefore, the equation of the tangent line is 
5. First, find the derivative using the Product Rule:
Clearly, the derivative will not exist at x = 1, because (x — 1)-1/5 would cause a zero in the denominator. The function will have a horizontal tangent line when its derivative is 0, so use the calculator to find when the derivative is 0.
Horizontal tangent lines should occur when x = —.366 and x = .651; these results are supported by the graph of the function below:
6. This is a large list of characteristics of t(x), although there are probably more correct answers: t has jump discontinuities at x = —3 and 3; t has no derivative at x = —3, 3, —1, and 1 (remember that no derivative exists at discontinuities or sharp points); t'(x) = 0 on (—1,1); t'(x) = 1 on (—3,—1); t'(x) = —1 on (1,3); t appears to be an even function; t' is negative on (—∞,—3) ∪ (1,3); t' is positive on (—3,—1) ∪ (3,∞); sketch tangent lines and examine the slopes to see that this is true; t is decreasing at a decreasing rate on (—∞,3), increasing at a constant rate on (—3,—1), constant on (—1,1), decreasing at a constant rate on (1,3), and increasing at an increasing rate on (3,∞).
7. First, find the derivative of n(x) and use the fact that n'(2) = —6:
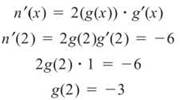
Now, you only need to find f'(2) to complete the chart. Use the fact that p’(2) = —3:
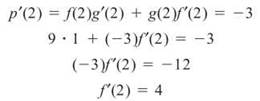
8. Draw a succession of tangent lines to the graph, and examine the slope of the tangent lines, as pictured here:
The slopes are positive on (—∞,x1) ∪ (x2,∞), so the derivative is positive there also. Similarly, b'(x) will be negative on (x1,x2). Furthermore, b(x) will have horizontal tangent lines at x1 and x2, so derivatives there will be 0. All these characteristics apply only to graph D.
9. (a) Use the Quotient Rule to find p’(1):
(b) Rewrite the function as h(x) = f(x)3/2, and then apply the Power Rule:
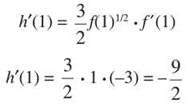
(c) Use the Chain Rule to find j’(1):
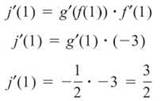
10. (a) True: Differentiability implies continuity.
(b) True: If continuous at x = 4, the limit must exist.
(c) False: m’(4) = 0; it doesn’t say that m (4) = 0.
(d) True: If m is continuous, no discontinuity exists.
(e) False: This is wrong for so many reasons
(f) True: It is also true that m'(4) = —m'(—4) since m is y-symmetric (draw a picture to convince yourself).
(g) True: m’(4) = m'(—4) = 0 since the graph is y-symmetric.
NOTE. You may have noticed that when a function increases, its derivative is positive, and when a function decreases, its derivative is negative. This is a very important observation, and it is discussed in depth in Chapter 5.
11. (a) h(9) ≈ 100 bt/min, which is the audience member’s heart rate at t = 9 minutes.
(b) h'(6) is the rate of change of the heart rate at t = 6. The rate of change of a rate is more commonly known as the acceleration. There is no formula in evidence, so you ’ll need to approximate the slope of the tangent line. Do so by finding the slope of a nearby secant line. One good approximator is the secant line connecting (4,115) and (6,103) (although it is not the only viable approximation). That slope is given by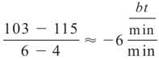 
or — 6 bt/min2 (the heart rate is decelerating).
(c) This is the formula for average rate of change on [8,12], or the average rate of change of the heart rate (average heart acceleration). There is no approximation here; your answer will be exact, as all necessary values are given exactly:
12. (a) In order to find velocity, you need to realize that velocity is the rate of change of position. This makes sense; the rate at which an object’s position changes tells you how fast that object is moving. So, you are trying to find the average rate of change of the position:
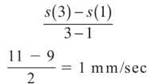
(b) Here, you are trying to find the velocity (rate of change, or derivative, of position). Thus, the velocity equation will be v(t) = 3t2 — 18t + 24, and v(3) = 27 — 54 + 24 = —3 mm/sec. Because velocity is negative, the particle is moving to the left instead of the right.
(c) The particle is not moving when its velocity is 0. So, set v(t) = 3t2 — 18t + 24 equal to 0 and use the calculator to solve the equation. The particle will be at rest when t = 2 and t = 4 sec. Factoring or using the quadratic formula would also have worked.
(d) s'(t) is the velocity of the particle, and a positive velocity implies that the particle is moving to the right. Thus, you are simply reporting the intervals on which s'(t) is positive, according to the graph.
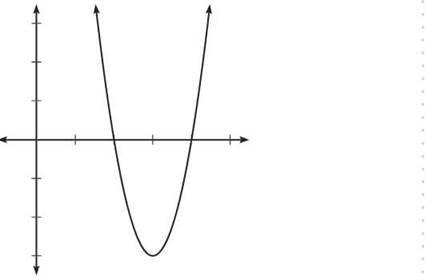
The t-intercepts appear to be t = 2 and 4, and that is verified by your work in 12(c), so the particle is moving right on (—∞,2) ∪ (4,∞).
SUMMING IT UP
• The study of limits and continuity only sets the stage for derivatives and differentiation. More than half of the questions on the test will involve derivatives in some way or another
• The derivative of a function describes how fast and in what capacity a function is changing at any instant.
• Trigonometric functions and their derivatives are all over the AP test.