Master AP Calculus AB & BC
Part II. AP CALCULUS AB & BC REVIEW
CHAPTER 5. Advanced Topics in Differentiation
PARAMETRIC DERIVATIVES (BC TOPIC ONLY)
If you are not yet convinced that derivatives are not only useful but also more fun than your cousin last Thanksgiving when he had the stomach flu, you need only wait until the next chapter. At that point, you will learn that derivatives can serve all kinds of purposes, most of which even have applications in the real world. Because of the extreme handiness of derivatives, it is important that you, the BC student, can differentiate all kinds of equations; specifically, you should be able to find derivatives of polar, parametric, and vector equations and be able to interpret these derivatives. However, one thing at a time; we will start with parametric equations.
In order to find the derivative, dy/dx, of a parametrically defined relation, we find the derivatives of the x and y components separately with respect to t and write them as a quotient as follows:
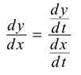
If it’s a second derivative you’re looking for, the formula is a little different. The second derivative is the derivative of the first derivative with respect to t divided by the derivative of the x component only with respect to t:
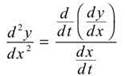
More simply,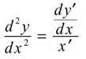 
Example 7: Find the derivative, dy/dx, and the second derivative, 
of the parametric equations x = 3 cos θ, y = 2 sin θ.
Solution: The derivatives, 
and 
are pretty simple to find, and it’s only a matter of one more step to get dy/dx.
In order to find the second derivative, find the derivative of the expression above, with respect to θ and divide it by dx/dθ:
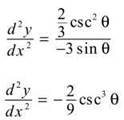
Example 8: Find the derivative of the parametric equations in Example 7 by a different method.
Solution: You can rewrite this set of parametric equations in rectangular form using the method described in Chapter 2. Because of the Mamma Theorem (cos2x + sin2x = 1), the equivalent rectangular form is 
In order to find dy/dx in this equation, you’ll have to use implicit differentiation:
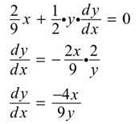
NOTE. We converted the parametric equations in Example 8 to rectangular form in the problem set for parametric equations in Chapter 2. Check back if you cannot remember how to convert.
Because you know the values of x and y, substitute them into dy/dx:
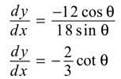
which matches the answer we got in Example 7.
Example 9: Find the equation of the tangent line to the parametric curve defined by x = arcsin t, y = e3t, when 
Solution: As always, we need a point and a slope in order to find the equation of a line. When 
we get the corresponding point (arcsin 
or 
) (You may use your calculator to find the corresponding decimals, but remember not to round anything until the problem is completely over.) To find the slope of the tangent line, you need to calculate dy/dx:
When the derivative is approximately 17.696, which leads to the tangent line equation of
TIP. 1/√2 is equivalent to √2/2; the latter is rationalized.
Vector-valued equations are very similar to parametric equations. Because vector equations are already defined in terms of x and y components, we follow the same procedure to find dy/dx. However, vector problems sometimes require us to find vectors that represent rate of change. This topic is discussed in detail in the next chapter in the section entitled “Motion in the Plane.”
EXERCISE 4
Directions: Solve each of the following problems. Decide which is the best of the choices given and indicate your responses in the book.
YOU MAY USE YOUR GRAPHING CALCULATOR ONLY FOR PROBLEM 5.
1. What is the derivative of the parametric curve defined by x = tant, y = cot2t?
2. Find the derivative of the parametrically defined curve x = 1 + 2t, y = 2 — t2 using two different methods.
TIP. Your calculator can find points on a parametric graph based on t and calculate dy/dt, dx/dt, and dy/dx. Instructions are given in the technology section at the end of the chapter.
3. Find 
when t = 2 for the parametric curve defined by the equations x = e3t, y = t2e3t.
4. If a parametric curve is defined by
and 
(a) At what point(s) does the curve have horizontal tangent lines?
(b) At what point(s) is the curve nondifferentiable?
TIP. Even though problems 1 through 4 would be calculator-inactive questions, you may want to use your calculator to check your work as you progress. Make sure you don’t rely on the calculator to complete these.
5. The position of a crazed lizard, as it runs left and right along the top of a hot brick wall, is defined parametrically as x = 2et, y = t3 - 4t + 7 for t ≥ 0 (t is in seconds). Rank the following values of t from least to greatest in terms of how fast the lizard was moving at those moments: t = 0, .5, 1, 2, 3.
ANSWERS AND EXPLANATIONS
1. Because 
and 
2. Method One: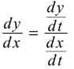 
Method Two: Convert to rectangular form first
Begin by solving either x or y for t and substituting it into the other equation.
Because x = 1 + 2t, 
and you can substitute:
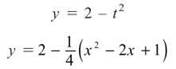
Now, take the derivative with respect to x:
This answer doesn’t seem to match the one above, but it actually does; the difference is that this solution is in terms of x, whereas the preceding solution was in terms of t. To see that they are equal, remember that x = 1 + 2t and substitute:
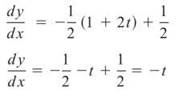
3. First, you need to find dy/dx. Don’t forget to use the Product Rule when differentiating t2e3t:
Now, to find the second derivative, divide 
by dy/dt:
The final answer is the derivative evaluated at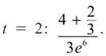 
It’s ugly, but it’s right. More simplification can be done, but it’s not necessary and not recommended.
4. (a) First find dy/dx as you have done numerous times already:
Horizontal tangent lines have a slope of 0; the derivative will only have a value of 0 when t = 2. The point that corresponds with t = 2 is 
(b) dy/dx does not exist when t = -1 or -5, because both values cause a 0 in the denominator. While the graphing calculator shows a definite sharp point when t = -1,
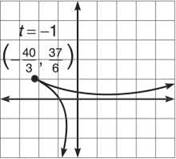
the target line is vertical when t = —5. The points corresponding to these values are 
(for t = -1) and 
(for t = -5).
5. The derivative of the parametric curve will be
NOTE. Speed is defined as the absolute value of velocity.
Rather than substitute each t value in by hand, use your calculator to evaluate each. You should get the following values: 
Remember that the derivative is the rate of change, so these numbers are the rate of change of the position of the lizard, or its velocity. Negative velocity indicates that the lizard is running to the left, positive velocity indicates running to the right. However, when speed is the issue, direction does not matter, so the lizard was moving the fastest at t = 0. The correct order is: t = 0, t = .5, t = 3, t = 2, t = 1.