Master AP Calculus AB & BC
Part II. AP CALCULUS AB & BC REVIEW
CHAPTER 6. Applications of the Derivative
MOTION
Calculus has its long, threatening talons in just about every aspect of day-to-day life; luckily, most of us are blissfully ignorant of it and unaware of it stalking us, waiting until we go to sleep, and then messing with our stuff—like putting CDs in the wrong cases and breaking all the points off your pencils on test day. But not even calculus can hide its influence in the topic of motion. Because a derivative describes a rate of change, we have already seen its influence many times and alluded to this very moment: describing how a derivative affects a position equation.
NOTE. A position equation describes an object’s motion by giving its position at any time.
Important Facts About Position Equations
A position equation is typically denoted as s(t) or x(t); for any time t, its output is the object’s position relative to something else. For example, output may represent how far a projectile is off the ground or how far away a particle is from the origin.
The derivative of position, s'(t) or v(t), gives the velocity of the object. In other words, v(t) tells how fast the object is moving and in what direction. For example, if we are discussing a ball thrown into the air and v(3 seconds) = —4 ft/sec, when time equals 3, the ball is traveling at a rate of 4 ft/sec downward.
The derivative of velocity, v\t) or a(t), gives the acceleration of the object. This ties in directly to the section you just completed. If an object has positive acceleration, then the position equation (two derivatives “above” a(t)) must be concave up, and the velocity equation (one derivative “above” a(t)) must be increasing.
The most common motion questions on the AP test focus on the motion of a particle on a line, usually horizontal (although the direction of the line doesn’t matter). For example, consider a particle moving along the x-axis whose position at any time t is given by s(t) = t3 — 10t2 + 25t — 1, t > 0. The graph of the position equation looks like
but the particle itself never leaves the v-axis. Let’s look at this problem in depth to better understand a typical particle motion problem.
Example 6: If the position (in feet) of a particle moving horizontally along the x-axis is given by the equation s(t) = t3 — 10t2 + 25t, t > 0 seconds, answer the following:
(a) Evaluate s(1), s(4), and s(5), and interpret your results.
By simple substitution, s(1) = 16, s(4) = 4, and s(5) = 0. In other words, when 1 second has elapsed, the particle is 16 feet to the right of the origin, but 3 seconds later at t = 5, the particle is back to the origin.
(b) At what time(s) is the particle temporarily not moving, and why?
The particle will be temporarily stopped when its velocity equals 0—this makes a lot of sense, doesn’t it? Since the derivative of position is velocity, take the derivative and set it equal to 0:
v(t) = s'(t) = 3t2 - 20t + 25 = 0
ALERT! Same students don’t believe me when I say that it is necessary to briefly stop when changing direction, and in demonstrating their point, many of these students end up with neck injuries.
Now, factor the quadratic equation to complete the solution:
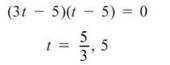
Therefore, at these two moments, the particle is stopped because it is in the process of changing direction. (Remember, part (a) showed you that it changed direction between t = 1 and t = 4.)
(c) On what interval of time is the particle moving backward?
The particle moves backward when it has negative velocity. Therefore, we will draw a velocity (first derivative) wiggle graph. We already know the critical numbers from part (b), so all that remains is to choose some test points from among the intervals. Because v(.5) is positive, v(3) is negative, and v(6) is positive (of course you wouldn’t have to pick the same test points), you get the following wiggle graph:
Therefore, the particle is moving backward on (5/3,5). This makes sense if you consider the graph of s(t). Remember, this position graph tells how far the particle is away from the origin. On the interval (5/3,5), the particle’s distance from the origin is decreasing, indicating backward movement. It should be no surprise that the velocity is negative then, since velocity is the derivative of that graph, and derivatives have a nasty habit of describing the direction of things.
(d) How far does the particle travel in its first 4 seconds of motion?
You may be tempted to answer 4 feet, since s(4) = 4; however, that is what’s called the displacement of the particle. The displacement is the net change in position. Because s(0) = 0 and s(4) = 4, no matter what happened in between, the particle ended up a total of 4 units from where it started. However, the problem doesn’t ask for displacement—it asks for total distance traveled. We need to measure how far it swung out to the right of the origin when it changed direction at t = 5/3 and then how far back toward the origin it came. We already know s(0) = 0, but it is essential to know that s(5/3) ≈ 18.518518518, because it tells us that the particle traveled 18.518518518 feet in the first 
seconds. At this point, the particle changes direction and ends up 4 feet from the origin. In the return trip, then, it traveled 18.518518518 — 4 = 14.518518518 feet. The total distance it traveled was 18.518518518 + 14.518518518 ≈ 33.037 feet.
NOTE. Air resistance has been neglected torso long in theoretical mathematics that it is rumored to have joined a 12-step program.
The other type of motion problem the AP test enjoys inflicting upon you is the dreaded trajectory problem. Did you know that anything thrown, kicked, fired, or otherwise similarly propelled follows a predetermined position equation on the earth? It’s true, neglecting air resistance of course. The generic projectile position equation is
where g is the gravitational acceleration constant (32 ft/sec2 in the English system and 9.8 m/sec2 in the metric), v0 is the object’s initial velocity, and h0 is the object’s initial height. It is probably a good idea to memorize this equation in case you ever need it, although the questions typically asked for this sort of problem are extremely similar to those asked in Example 6.
EXERCISE 5
Directions: Solve each of the following problems. Decide which is the best of the choices given and indicate your responses in the book.
YOU MAY USE A GRAPHING CALCULATOR FOR BOTH OF THESE PROBLEMS.
1. A very neurotic particle moves up and down the y-axis according to the position equation y = (t2 — 6t + 8) ∙ sin t, t > 0, where position is in centimeters and time is in seconds. Knowing this, answer the following questions:
(a) When is the particle moving down on the interval [0,5]?
(b) At what values of t is the particle moving at a rate equal to the average rate of change for the particle on the interval [0,5]?
(c) At what time(s) is the particle exactly 2 cm away from the origin on the interval [0,5]?
(d) What is the acceleration of the particle the first time it comes to rest?
2. The practice of shooting bullets into the air—for whatever purpose—is extremely dangerous. Assuming that a hunting rifle discharges a bullet with an initial velocity of 3,000 ft/sec from a height of 6 feet, answer the following questions (neglecting wind resistance):
(a) How high will the bullet travel at its peak?
(b) How long will it take the bullet to hit the ground?
(c) At what speed will the bullet be traveling when it slams into the ground, assuming that it hits nothing in its path?
(d) What vertical distance does the bullet travel in the first 100 seconds?
ANSWERS AND EXPLANATIONS
1. (a) The particle is moving down when its position equation is decreasing—when the velocity is negative. You should make a first derivative wiggle graph, so begin by finding the critical numbers of the derivative:
v(t) = (t2 — 6t + 8)(cos t) + (2t — 6)(sin t) = 0
It’s best to solve this using your graphing calculator. The solutions are t = .738, 2.499, and 3.613. The wiggle graph is
Therefore, the particle is moving down on (.738,2.499) ∪ (3.613,5).
(b) The average rate of change of the particle will be 
cm/sec. To determine when the particle travels this speed, set the velocity equal to this value and solve with your calculator. This is actually the Mean Value Theorem in disguise; we know at least one t will satisfy the requirements in the question, but it turns out that the instantaneous rate of change equals the average rate of change three times, when t = .813, 2.335, and 3.770 sec.
(c) The particle will be two cm away from the origin when its position is 2 (two cm above) or —2 (2 cm below). So, you need to solve both the equations (t2 — 6t + 8)sin t = 2 and (t2 — 6t + 8)sin t = —2 with your calculator. The solutions are t = .333, 1.234, and 4.732 sec.
(d) The particle first comes to rest at its first critical number, t = .73821769. To find acceleration, you need to differentiate the velocity and substitute in the critical number. Rather than doing this by hand, why not use the graphing calculator and the nDeriv function? You would type the following on your TI-83: nDeriv((x2 — 6x + 8)(cos (x)) + (2x — 6)(sin (x)),x,.73821769). The resulting acceleration is —8.116 cm/sec2.
2. (a) You will need to apply the projectile position equation. The initial height and velocity are stated by the problem, and since the question uses English system units (feet), you should use g = 32 ft/sec2 as the acceleration due to gravity. You put your left foot in, you take your left foot out, you put your left foot in, shake it all about, and the position equation is
s(t) = —16t2 + 3,000t + 6
The bullet will reach its peak at the maximum of the position equation (since it is an upside-down parabola, there will be only one extrema point). To find the t value at which the peak occurs, find the derivative and set it equal to 0 (since the bullet will have a velocity of 0 at its highest point before it begins to fall toward the ground):
v(t) = s'(t) = -32t + 3,000 = 0
t = 93.75 seconds
This, however, is not the answer. The height the bullet reaches at this point is the solution: 140,631 feet, or 26.635 miles.
(b) The bullet will hit the ground when s(t) = 0—literally, when the bullet is 0 feet off of the ground. So, set the position equation equal to 0, and solve (if you use the calculator, you’ll have to ZoomOut a few times before the graph appears—these are big numbers). The bullet will remain in the air 187.502 seconds, or 3.125 minutes.
(c) |s'(187.502)| = 3,000.064 ft/sec (since speed is the absolute value of velocity, the answer is not negative). The bullet will hit at a speed slightly greater than that at which it was fired. Therefore, being hit by a bullet that was fired into the air will have the same impact as being hit by a bullet at point-blank range.
(d) You already know that the bullet travels 140,631 feet in the first 93.75 seconds. Because s(100) = 140,006, the bullet falls 140,631 — 140,006 = 625 feet between t = 93.75 and t = 100. Therefore, the bullet travels a total vertical distance of 140,631 feet up + 625 feet down = 141,256 feet.