Master AP Calculus AB & BC

Part II. AP CALCULUS AB & BC REVIEW

CHAPTER 7. Integration

THE FUNDAMENTAL THEOREM OF CALCULUS

I once had a Korean professor in college named Dr. Oh. He once said something I remember to this day: “Fundamental theorems are like the beginning of the world. Yesterday, not very interesting. Today, interesting.” This is quite accurate, if not a little understated. In this theorem lies the fabled connection between the antiderivative and the area beneath a curve. In fact, the fundamental theorem has two major parts. Mathematicians can’t seem to agree which is the more important part and, therefore, number them differently. Some even refer to one as the Fundamental Theorem and the other as the Second Fundamental Theorem. I love them both equally, as I would my own children. The first part of the Fundamental Theorem deals with definite integrals. These are slightly different from the integrals we’ve been dealing with for two reasons: (1) they have boundaries, and (2) their answers are not functions with a “+ C” tacked on to the end—their answers are numbers. These are, indeed, two giant differences, but you’ll be surprised by how much they actually have in common with our previous integrals, which we will now refer to by their proper name, indefinite integrals.

The Fundamental Theorem, Part One

NOTE. Remember, you can’t spell Fundamental Theorem without “fun”!

If f(x) is a continuous function on [a,b] with antiderivative g(x), then

Translation: In order to evaluate the definite integral , find the antiderivative of f(x). Once you’ve done that, plug the upper bound, b, into the antiderivative. You should get a number. From that number, subtract the result of plugging the lower bound into the antiderivative.

NOTE. The a and b in definite integrals are called the limits of integration. However, they have little to do with the limits of Chapter 3, so don’t worry.

What is the purpose of definite integrals? They give the exact area beneath a curve. Let’s return to a problem from the Trapezoidal Rule section. You used 2, 3, and 4 subintervals to approximate the area beneath y = x² + 1 on [0,4], Let’s find out what the exact area is.

Example 5: Find the exact area beneath y = x² + 1 on [0,4],

Solution: The specified area is the result of the following integral:

So, you need to find the antiderivative of x² + 1. When you do, drop the integration sign and dx, as you did before.

The problem is not yet over, and you signify that the boundaries of integration still must be evaluated with the vertical line (or right bracket, if you prefer) and the boundaries next to the antiderivative. To finish the problem, then, you plug the upper limit of integration into the expression (both x’s!) and then subtract the lower limit plugged in:

units squared

Example 6: Find the area beneath sin x on [0,π].

Solution: This area is found by evaluating the definite integral

Integrate sin x to get

Now, substitute in π and 0 and subtract the two results:

—cos π — (—cos 0)

—(—1) + 1 = 2 units squared

Example 7: Evaluate and explain the answer geometrically. Solution: To begin, apply the Fundamental Theorem.

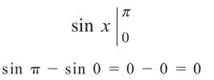
How can a curve have no area beneath it? Consider the graph of y = cos x on [0,π]:

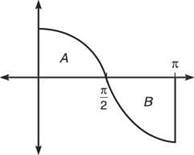
The area is made up of two separate areas, marked A and B on the diagram. Let’s find those two separate areas:

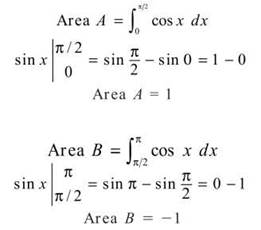
NOTE. You may argue that no area is technically negative. That’s true. However, any area that falls beneath the x-axis is considered negative. To avoid this logical dilemma, the area bounded by definite integrals is often referred to as signed area—area that is positive or negative based on its position with relation to the x-axis.

These answers should not be too surprising. They are the same, although one is located under the x-axis, so its signed area is the opposite of the other. When you add these two areas together, you get 0. So, the geometric explanation is that the areas are opposites, and the resulting sum is 0.

Example 7 is a great segue to a few definite integral properties that are essential to know:

Translation: You can split up an integral into two parts and add them up separately. Instead of integrating from a to c, you can integrate from a to b and add the area from b to c. We did this in Example 7. In that case, a = 0, c = π, and b = π/2.

Translation: If you start and end at the same x value, you are technically not covering any area. Therefore, if the upper and lower limits of integration are equal, the resulting area and definite integral have a value of 0.

Translation: In a typical definite integral, the upper bound, b, is greater than the lower bound, a. If you switch them, the answer you get will be the opposite of your original answer. For example, let’s redo Example 6 with the limits of integration switched:

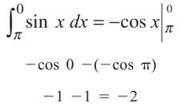
In essence, switching the boundaries of integration has the effect of commuting (switching the order of) the subtraction problem dictated by the Fundamental Theorem, making it g(a) — g(b) instead of g(b) — g(a). This causes the sign change.

• The definite integral represents accumulated change.

Translation: In the same way that derivatives expressed a rate of change, the integral goes in the other direction and reports accumulated change. For example, consider the graph below of a car’s velocity:

At any time t, the graph tells how fast the car was going. (This is the graph of the rate of change of position, velocity.) However, definite integrals give accumulated change.

Therefore, actually gives the distance the car traveled between time a and b. If the graph represented the rate of sale of socks over time, then the definite integral represents the number of socks sold over that time. More appropriate for me, if the graph represents the rate of hair loss over time, then the definite integral represents the actual amount of hair lost over the interval of time. Get the picture? More on this in Chapter 9.

Now that you know quite a bit about definite integrals, it’s time to spring Part Two of the Fundamental Theorem on you. In essence, this theorem shows that differentiation and integration are opposites of one another.

The Fundamental Theorem, Part Two

If c is a constant and t and x are variables,

NOTE. If the upper bound is something other than a single variable, according to the Chain Rule, you must multiply by its derivative.

Translation: This theorem is very specific in its focus and purpose. It applies only if (1) you are finding the derivative of a definite integral, (2) you are differentiating with respect to the same variable that is in the upper limit of integration, and (3) the lower limit of integration is a constant. If all these conditions are met, the derivative of the integral is simply the function inside the integral (the derivative and integral cancel each other out) with the upper bound plugged in.

Although this part of the Fundamental Theorem may sound awfully complicated (and some books make it sound nearly impossible), it is really quite easy. The following example will lead you through the process.

NOTE. I will refer to Part Two of the Fundamental Theorem as “Part Two” to avoid repeating myself. However, refer to it as merely the Fundamental Theorem if justifying an answer on the AP test.

Example 8: Evaluate the following derivatives:

You are taking the derivative of an integral with respect to the variable in the upper limit of integration. In addition, the lower limit is a constant. Because all these things are true, you may apply Part Two. In order to do so, simply plug the upper bound, x, into the function to get cos x. That’s all there is to it.

If you forgot this handy trick, you can still integrate as in the past:

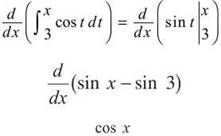
Because sin 3 is a constant, its derivative is 0. This is why the lower boundary must be a constant for the theorem to work and also why it doesn’t matter what that lower boundary is.

The upper bound is h² rather than just h, but the variable you are deriving with respect to still matches, so you can still apply Part Two. Simply plug the upper bound into the function to get

NOTE. There is no “+ C” on your answer because you are finding a derivative, even though it’s the derivative of an integral.

However, this is not the final answer. Because the upper bound is not merely h, you have to multiply by its derivative according to the Chain Rule. In this case, just multiply your previous answer by

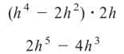
If you like, you can verify this by using Part One of the Fundamental Theorem.

Bad news: The lower bound of this integral is not a constant. Therefore, we cannot apply Part Two. So, we default back to Part One of the Fundamental Theorem. It helps to rewrite √t as t^1/2 before you begin.

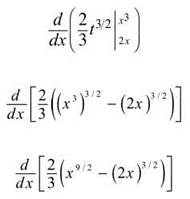
Don’t forget to take the derivative once you’re finished integrating.

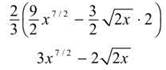
EXERCISE 4

Directions: Solve each of the following problems. Decide which is the best of the choices given and indicate your responses in the book.

YOU MAY USE A GRAPHING CALCULATOR FOR PROBLEMS 3 AND 4 ONLY.

1. Evaluate the following definite integrals:

2. If f(x) is defined by the graph below and consists of a semicircle and numerous line segments, evaluate the following:

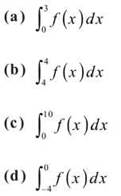
3. Find the vertical line x = c that splits the area bounded by y = √x, y = 0, and x = 8 exactly in half.

4. Evaluate the following derivatives:

ANSWERS AND EXPLANATIONS

1. (a) Begin by rewriting the expression and distributing the x^1/3.

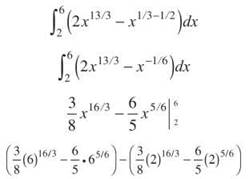
Without a calculator, there’s really no need to continue simplifying. What was the purpose of a problem that doesn’t simplify? Get used to getting weird answers that don’t work out evenly. Have confidence in answers that look and feel weird.

(b) Distribute the sec x to get

You can integrate each of those terms.

(c) Even though we never discussed absolute value definite integrals, the answer is as simple as looking at the graph:

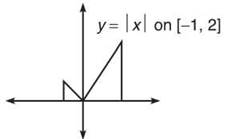
The area beneath the graph is composed of two right triangles, and all you need to find the area of a triangle is Therefore, the area of both triangles is

Not bad, eh? Look at the problems at the end of the chapter to practice nonlinear absolute value definite integrals.

2. Your work on 1(c) should make this easier. In order to calculate the definite integrals, use geometric formulas for triangles and semicircles.

(a) —3: This is a triangle with base 3 and height 2. It is also below the v-axis, so its signed area is negative.

(b) 0 : One of the properties of definite integrals stated that an integral with equivalent upper and lower limits of integration has zero value, as no area is accumulated.

(c) 2π — 1: The area from 0 to 4 is —4. The area from 4 to 8 is a semicircle of radius 2, which has area The area from 8 to 10 is a right triangle of area 3. The sum is 2π — 1.

(d) 5/2: In order to do this problem, you first need to find the v-intercept of the line segment from (-4,3) to (-1,-1). To do this, find the equation of the line by finding the slope

and substituting a point.

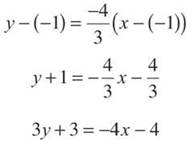
Then, set the y equal to 0 in order to find the x-intercept:

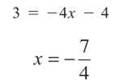
Therefore, the area from —4 to 0 consists of two right triangles: one with positive area (height = 3 and base = ) and one with negative area (height = 1 and base = 7/4). Therefore, the total area will be

3. The region bounded by all those graphs is simply

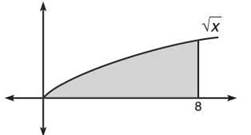
and this area is given by

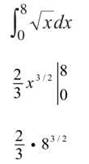
We are looking for a c between 0 and 8 that has exactly half of the area, or an area of 1/3 ∙ 8^3/2. In other words,

Integrate the left side to get

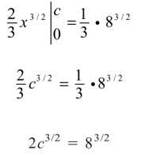
Square both sides to solve

4. (a) You cannot apply Part Two right away since the lower bound isn’t a constant. However, one of the properties of definite integrals says that you can switch the order of the bounds if you take the opposite of the integral:

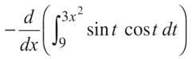
TIP. Ever wonder when to calculate area and when to calculate signed area? When computing definite integrals, it’s always signed area.

Apply Part Two and be finished. Don’t forget to multiply by the derivative of the upper bound.

—6x(sin 3x² cos 3x²)

(b) No Part Two here, as the lower bound again isn’t a constant, and this time you can’t do much about it. Just use the Fundamental Theorem Part One:

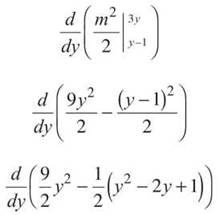
Again, don’t forget to take the derivative to finish!