Master AP Calculus AB & BC

Part II. AP CALCULUS AB & BC REVIEW

CHAPTER 10. Differential Equations

OVERVIEW

• Hands-On Activity 10.1: Separation of variables

• Hands-On Activity 10.2: Slope fields

• Euler’s method (BC topic only)

• Exponential growth and decay

• Logistic growth (BC topic only)

• Technology: A differential equations calculator program

• Summing it up

A differential equation is simply an equation that contains a derivative. Your typical goal in a differential equation problem is to find the equation that has the given derivative; in other words, you are trying to find an antiderivative. How is this different from the integrals you have been finding until now? Well, it’s not very different at all. You will be using all of your integration techniques to find particular solutions (no + C) using the method of separation of variables. However, the vast majority of differential equations in the real world cannot be solved using this method. We will then further examine those solutions using slope fields and Euler’s Method. Finally, we will look at some applications of differential equations in exponential and logarithm ic growth. After we’re done, we’ll go get an ice cream cone, and I’ll buy you that pony you’ve always wanted.

HANDS-ON ACTIVITY 10.1: SEPARATION OF VARIABLES

Even though the study of differential equations is complex, you are only required to know and understand the easiest of all methods for solving them— separation of variables. The name says it all, and you’ve already performed all the tasks that are involved in the process. Therefore, this section represents a new topic with nothing substantially new to learn (I love those kinds of topics).

1. What about the equation makes it a differential equation?

________________________________________________________

________________________________________________________

2. Your goal will be to find an equation (in the form y = f(x)) whose derivative is —x/y. Why can’t you simply integrate right away? What makes this equation different from the equation which you can integrate right away?

________________________________________________________

________________________________________________________

3. This topic is very similar to a differentiation topic for the reason you cited in number 2. What is the name of that topic, and why is it similar?

________________________________________________________

4. Before you can integrate you must separate the variables (put all the y’s on one side and the x’s on the other). How can you accomplish this in our equation?

________________________________________________________

________________________________________________________

5. Go ahead and separate the variables using the method you named in number 4. Now, you should be able to integrate both sides of the equation separately. Integrate, remembering to include a C for any constant. What geometric shape is the solution to the differential equation?

________________________________________________________

________________________________________________________

________________________________________________________

6. The answer we have is very general (because of the C). What if you knew that the solution curve passed through the point (0,3)? Given this information, what would your solution be?

________________________________________________________

________________________________________________________

7. Let’s try a new differential equation Separate the variables, and integrate both sides separately.

________________________________________________________

________________________________________________________

________________________________________________________

8. Your y expression ends up contained in a natural log. In such cases, it is preferred to solve the equation for y, not ln y. Solve for y.

________________________________________________________

________________________________________________________

9. If you knew that the particular solution you were looking for satisfied the condition y(3) = 5, what is C, and what is the solution to the differential equation?

________________________________________________________

________________________________________________________

________________________________________________________

SOLUTIONS TO HANDS-ON ACTIVITY 10.1

TIP. Even though you could have grouped the - with the y, it’s better to leave it (and any constants a problem might have) on the side with the x’s; this makes solving for y easier, and most differential equation solutions are in that form.

1. It contains a derivative.

2. contains both x’s and v’s; until now, all of our integrals have contained just x’s.

3. This is similar to implicit differentiation; those expressions contained both x’s and v’s and thus required a different method.

4. Separation can be accomplished in this problem by cross-multiplying. That may not work for all problems, but separation is usually achieved through very simple methods (see number 7).

5. Cross-multiplying gives you

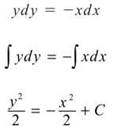
Multiply everything by 2 and move the x term to get

x² + y² = C

Therefore, the solution to the differential equation is a circle. (Often, solutions are written solved for y, but in this case, the answer is more clearly a circle when you leave it in standard form for a circle. Either way, however, the answer is right.)

TIP. This is not the same C as in the previous step—it just indicates an arbitrary number. Some textbooks use different constants each time because of this, but that’s silly—just remember that C might never be the same number.

6. Plug in the x = 0 and y = 3, since these values (if on the graph) must make the equation true.

0² + 3² = C

C = 9

Now that we know the specific value for C, we can plug it into the solution:

x² + y² = 9

So, this specific solution is a circle of radius 3.

7. Begin by factoring the x² out of the numerator.

Divide both sides by (1 + y), and multiply both sides by dx to separate the variables.

Notice how the constant stays with the x terms so that you can solve for y more easily. Now, integrate both sides.

NOTE. Rewriting as is the same thing as rewriting

8. To solve for y, raise e to the power of both sides to get

You can rewrite the right side of the equation as (using properties of exponents), and e^C is just another constant, which you can then write as C. This gives you

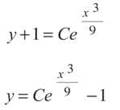
NOTE. When you are given a point value and can find C in a differential equation, the resulting solution is called a particular solution, since it is only one of many possible solutions if no point values were indicated.

9. y(3) = 5 means that plugging a value of 3 into the equation (for x) gives an output of 5; it’s similar to saying f(3) = 5. If that confuses you, remember that y(3) = 5 means the point (3,5) is on the graph. Either way, plug in x = 3 and y = 5 in order to find C.

Therefore, the solution to this differential equation is

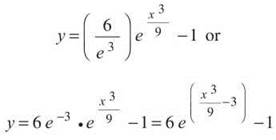
EXERCISE 1

Directions: Solve each of the following problems. Decide which is the best of the choices given and indicate your responses in the book.

DO NOT USE A CALCULATOR TO SOLVE THE FOLLOWING.

1. Find the particular solution to that satisfies the condition that y(0) = 1.

2. What function has derivative f'(x) = e^x esc y and passes through the origin?

3. In some cases, the rate of change of a quantity is proportional to the quantity itself. This is written as

where y is the quantity and k is the proportionality constant. What is the general solution to this very important differential equation?

4. A particle moves along the x-axis with acceleration at time t given by a(t) = 3t. Find the function describing the particle’s position if it travels at a rate of 2 ft/sec when t = 0 and is 3 feet to the right of the origin when t = 1.

5. If a ball is thrown upward and reaches its maximum height when t = 2 seconds, answer the following questions:

(a) Give the function representing the ball’s velocity.

(b) If the ball is 85 feet off the ground when t = 1.5 seconds, what is the position function for the ball?

ANSWERS AND EXPLANATIONS

1. Divide both sides by √y, and multiply by dx to separate the variables.

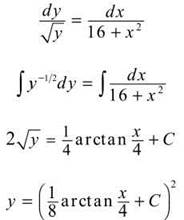
Now, plug in the given values for x and y, and you find (easily) that C = 1. Therefore, the particular solution is

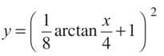
NOTE. Your solution to problem 3 is the foundation for the section on exponential growth and decay, where all the quantities in question have the property problem 3 describes.

2. Begin by rewriting the equation like this:

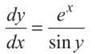
Now, cross-multiplying will separate the variables.

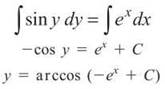
Now, plug in the point (0,0).

cos (0) = cos (arccos (—1 + C))

—1 + C = 1

C = 2

The final answer is y = arccos (—e^x + 2).

3. Divide both sides by y, and multiply them by dt to get the necessary separation. Remember that k is a constant.

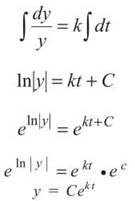
NOTE. When you multiply by a negative, you don’t have to write “-C”, since C could already be negative! It’s a mystery number for now.

4. (a) The integral of acceleration is velocity, and we know that v(0) = 2. So, find the antiderivative of a(t), and plug in the given information.

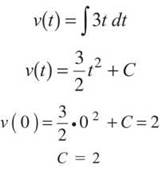
Therefore, the equation for velocity is In order to find position, integrate again, and use the fact that s(1) = 3.

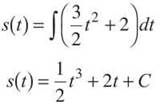
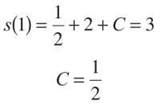
Therefore, the position equation is

5. (a) Remember that the equation for projectile position is s(t) = —16t² + v₀t + h₀, where v₀ is the initial velocity and h₀ is the initial height. You are looking for the velocity equation, so take the derivative:

v(t) = —32t + v₀

The ball reaches its maximum height when t = 2. Therefore, the derivative must equal 0 when t = 2. This allows you to find v₀.

v(2) = —32(2) + C = 0

C = 64

Therefore, the velocity equation is v(t) = —32t + 64.

(b) To find position, integrate velocity, and use the fact that s(1.5) = 85.

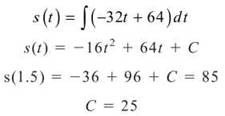
Therefore, the position equation for the ball is

s(t) = —16t² + 64t + 25