5 Steps to a 5: AP Calculus AB 2017 (2016)
STEP 4
Review the Knowledge You Need to Score High
CHAPTER 14
Big Idea 3: Integrals and the Fundamental Theorems of Calculus
More Applications of Definite Integrals
IN THIS CHAPTER
Summary: In this chapter, you will learn to solve problems using a definite integral as accumulated change. These problems include distance traveled problems, temperature problems, and growth problems. You will also learn to work with slope fields and to solve differential equations.
Key Ideas
Average Value of a Function
Mean Value Theorem for Integrals
Distance Traveled Problems
Definite Integral as Accumulated Change
Differential Equations
Slope Fields
14.1 Average Value of a Function
Main Concepts: Mean Value Theorem for Integrals, Average Value of a Function on [a , b ]
Mean Value Theorem for Integrals
If f is continuous on [a , b ], then there exists a number c in [a , b ] such that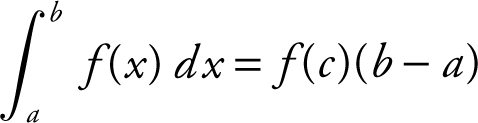 
. (See Figure 14.1-1 .)
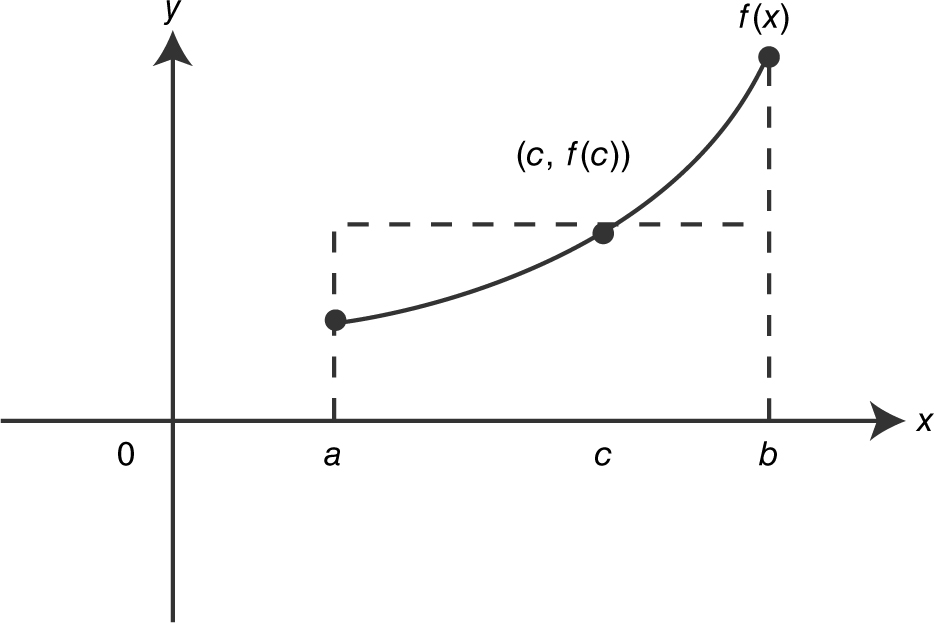
Figure 14.1-1
Example 1
Given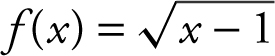 
, verify the hypotheses of the Mean Value Theorem for Integrals for f on [1, 10] and find the value of c as indicated in the theorem.
The function f is continuous for x ≥ 1, thus:
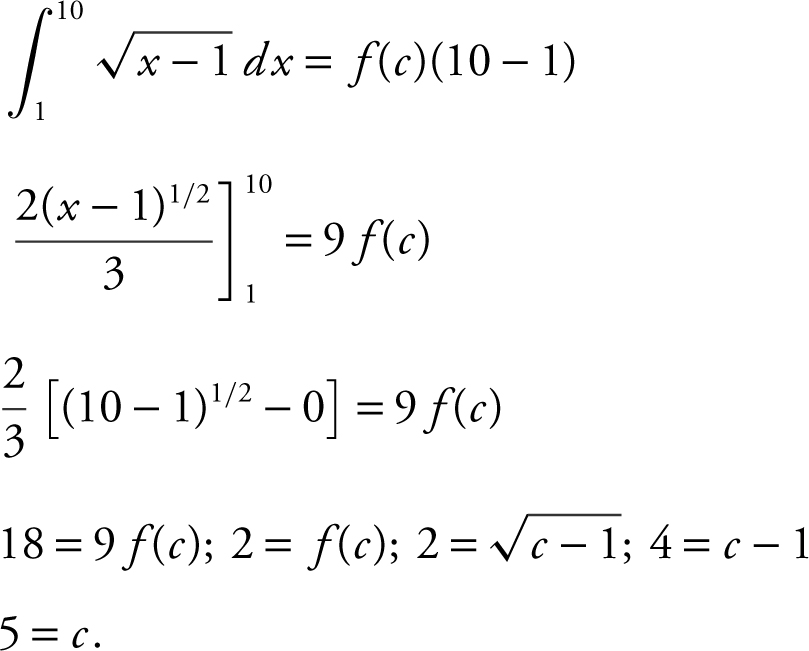
Example 2
Given f (x ) = x 2 , verify the hypotheses of the Mean Value Theorem for Integrals for f on [0, 6] and find the value of c as indicated in the theorem.
Since f is a polynomial, it is continuous and differentiable everywhere,
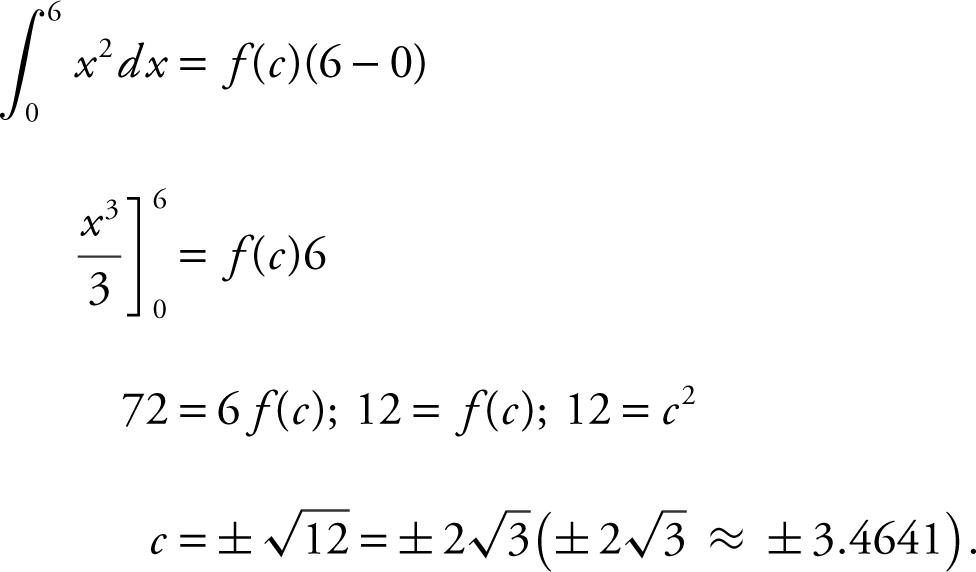
Since only 
is in the interval [0, 6], c = .
• Remember: If f′ is decreasing, then f″ < 0 and the graph of f is concave downward.
Average Value of a Function on [a, b ]
Average Value of a Function on an Interval
If f is a continuous function on [a , b ], then the Average Value of f on [a , b ]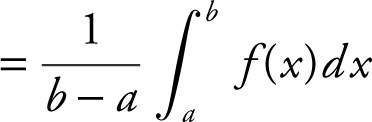 
.
Example 1
Find the average value of y = sin x between x = 0 and x = π .
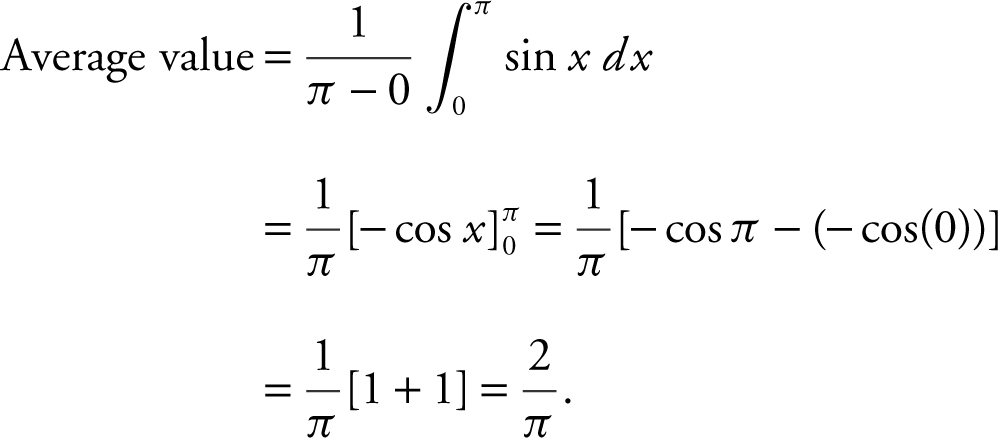
Example 2
The graph of a function f is shown in Figure 14.1-2 . Find the average value of f on [0, 4].
Figure 14.1-2
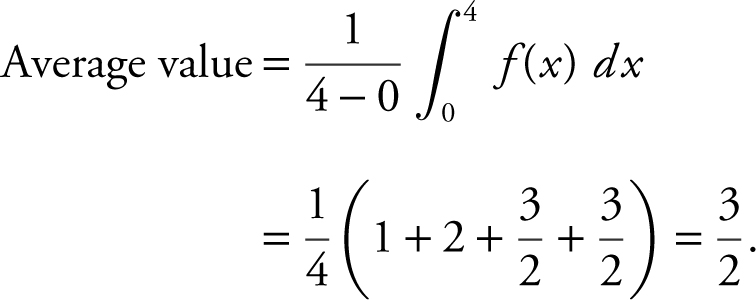
Example 3
The velocity of a particle moving on a line is v (t ) = 3t 2 – 18t + 24. Find the average velocity from t = 1 to t = 3.
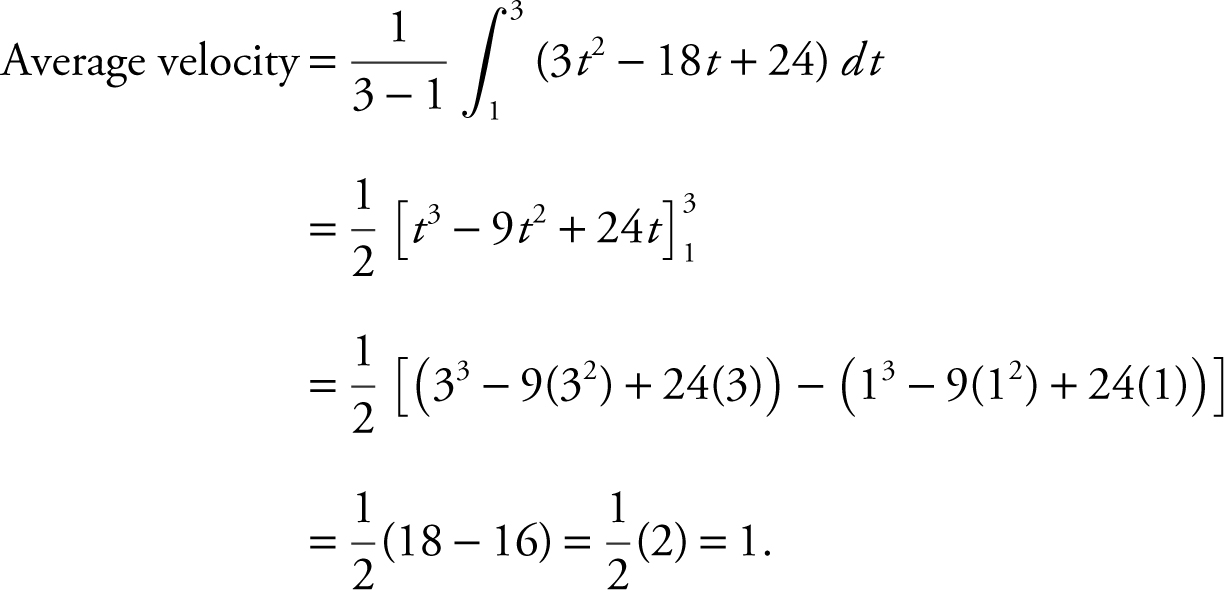
Note: The average velocity for t = 1 to t = 3 is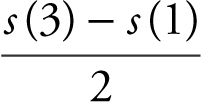 
, which is equivalent to the computations above.
14.2 Distance Traveled Problems
Summary of Formulas
Position Function: 
.
Velocity: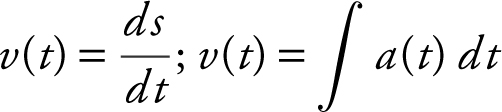 
.
Acceleration: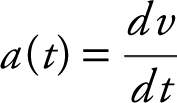 
.
Speed: |v (t )|.
Displacement from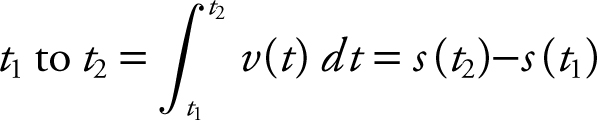 
.
Total Distance Traveled from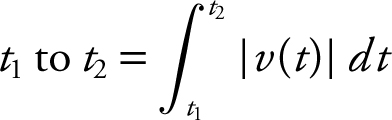 
.
Example 1
See Figure 14.2-1 .
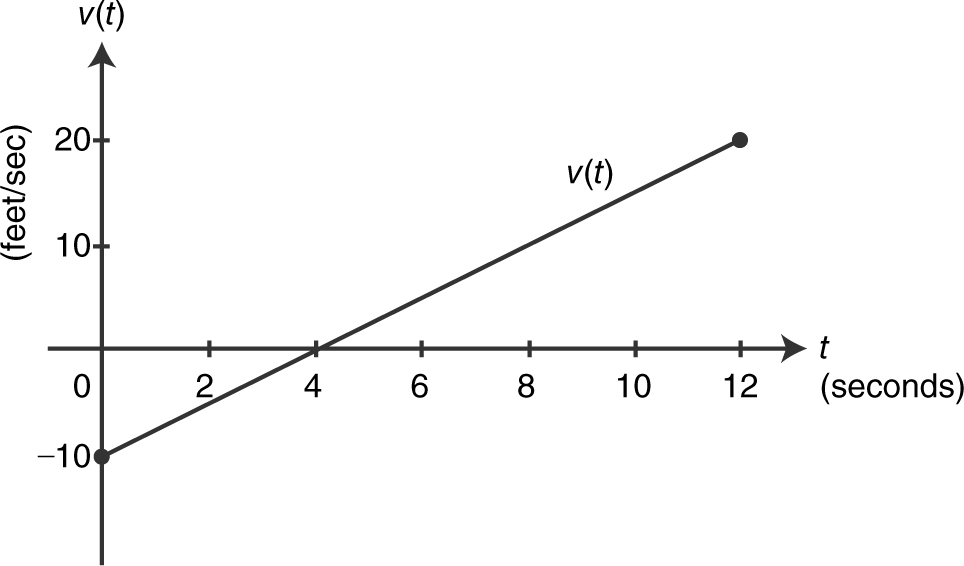
Figure 14.2-1
The graph of the velocity function of a moving particle is shown in Figure 14.2-1 . What is the total distance traveled by the particle during 0 ≤ t ≤ 12?
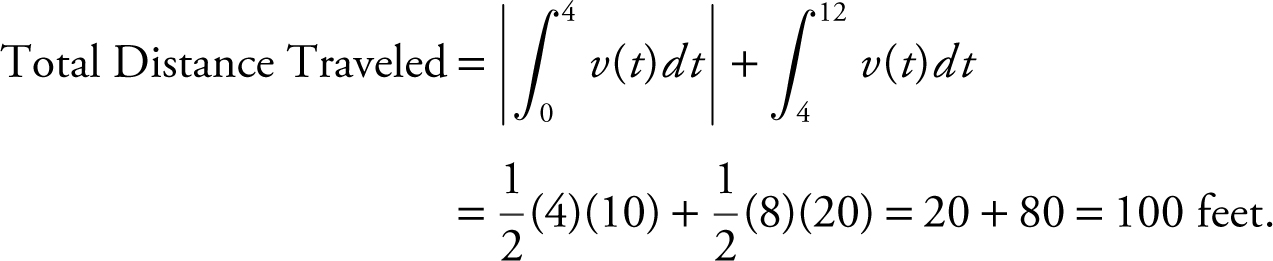
Example 2
The velocity function of a moving particle on a coordinate line is v (t ) = t 2 + 3t – 10 for 0 ≤ t ≤ 6. Find (a) the displacement by the particle during 0 ≤ t ≤ 6, and (b) the total distance traveled during 0 ≤ t ≤ 6.
The total distance traveled by the particle is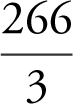 
or approximately 88.667.
Example 3
The velocity function of a moving particle on a coordinate line is v (t ) = t 3 – 6t 2 + 11t – 6.
Using a calculator, find (a) the displacement by the particle during 1 ≤ t ≤ 4, and (b) the total distance traveled by the particle during 1 ≤ t ≤ 4.
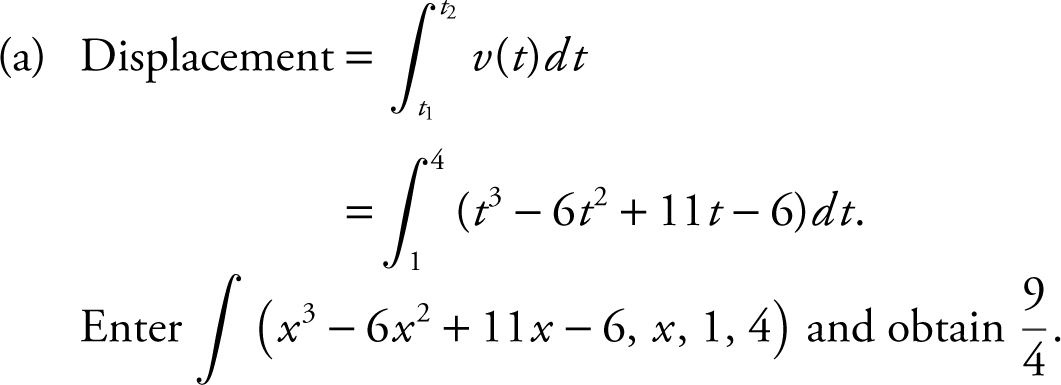
(b) Total Distance Traveled =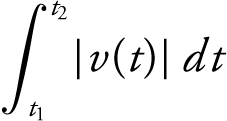 
.
Enter y 1 = x ∧ 3 – 6x ∧ 2 + 11x – 6 and use the [Zero ] function to obtain x -intercepts at x = 1, 2, 3.
Example 4
The acceleration function of a moving particle on a coordinate line is a (t ) = –4 and v 0 = 12 for 0 ≤ t ≤ 8. Find the total distance traveled by the particle during 0 ≤ t ≤ 8.
Total distance traveled by the particle is 68.
Example 5
The velocity function of a moving particle on a coordinate line is v (t ) = 3 cos(2t ) for 0 ≤ t ≤ 2π . Using a calculator:
(a) Determine when the particle is moving to the right.
(b) Determine when the particle stops.
(c) The total distance traveled by the particle during 0 ≤ t ≤ 2π .
Solution:
(a) The particle is moving to the right when v (t ) > 0.
Enter y 1 = 3 cos(2x ). Obtain y 1 = 0 when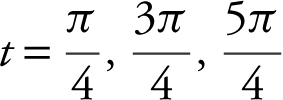 
, and 
.
The particle is moving to the right when:
.
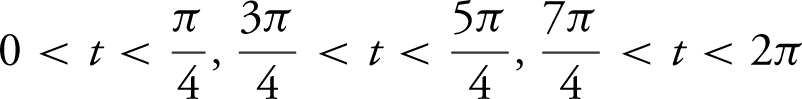(b) The particle stops when v (t ) = 0.
Thus the particle stops at 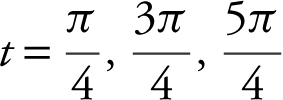, and .
(c) Total distance traveled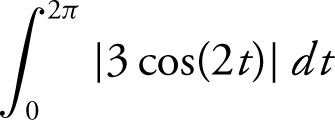 
.
Enter 
(abs(3 cos(2x )), x , 0, 2π ) and obtain 12.
The total distance traveled by the particle is 12.
14.3 Definite Integral as Accumulated Change
Main Concepts: Business Problems, Temperature Problem, Leakage Problem, Growth Problem
Business Problems
P ′(x ), R ′(x ), and C ′(x ) are the instantaneous rates of change of profit, revenue, and cost, respectively.
Example 1
The marginal profit of manufacturing and selling a certain drug is P ′(x ) = 100 – 0.005x .
How much profit should the company expect if it sells 10,000 units of this drug?
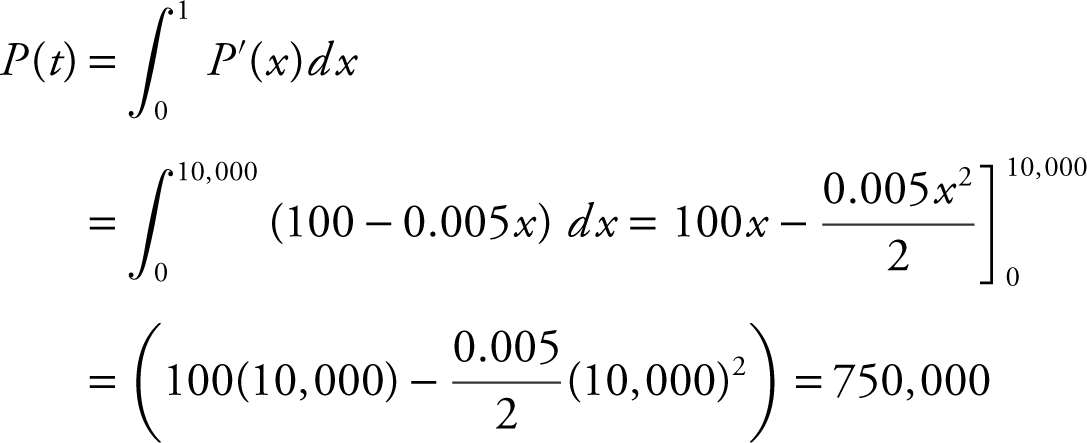
• If f ″(a ) = 0, f may or may not have a point of inflection at x = a , e.g., as in the function f (x ) = x 4 , f ″(0) = 0 but at x = 0, f has an absolute minimum.
Example 2
If the marginal cost of producing x units of a commodity is C ′(x ) = 5 + 0.4x ,
find (a) the marginal cost when x = 50;
(b) the cost of producing the first 100 units.
Solution:
(a) Marginal cost at x = 50:
C ′(50) = 5 + 0.4(50) = 5 + 20 = 25.
(b) Cost of producing 100 units:
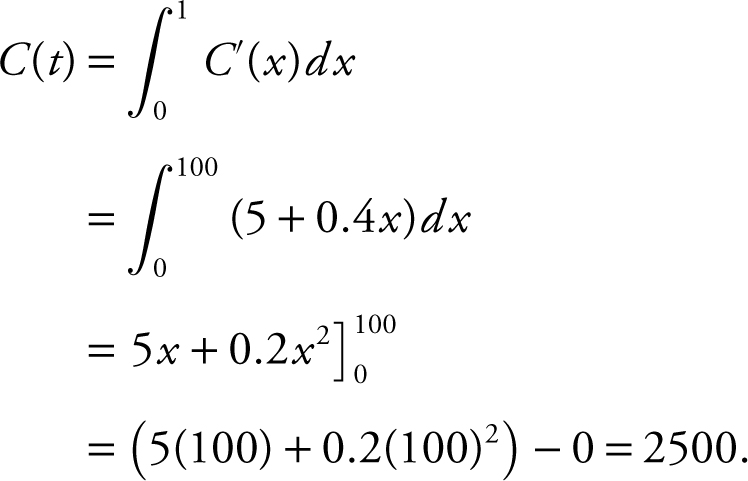
Temperature Problem
Example
On a certain day, the changes in the temperature in a greenhouse beginning at 12 noon are represented by 
degrees Fahrenheit, where t is the number of hours elapsed after 12 noon. If at 12 noon, the temperature is 95°F, find the temperature in the greenhouse at 5 p.m.
Let F (t ) represent the temperature of the greenhouse.
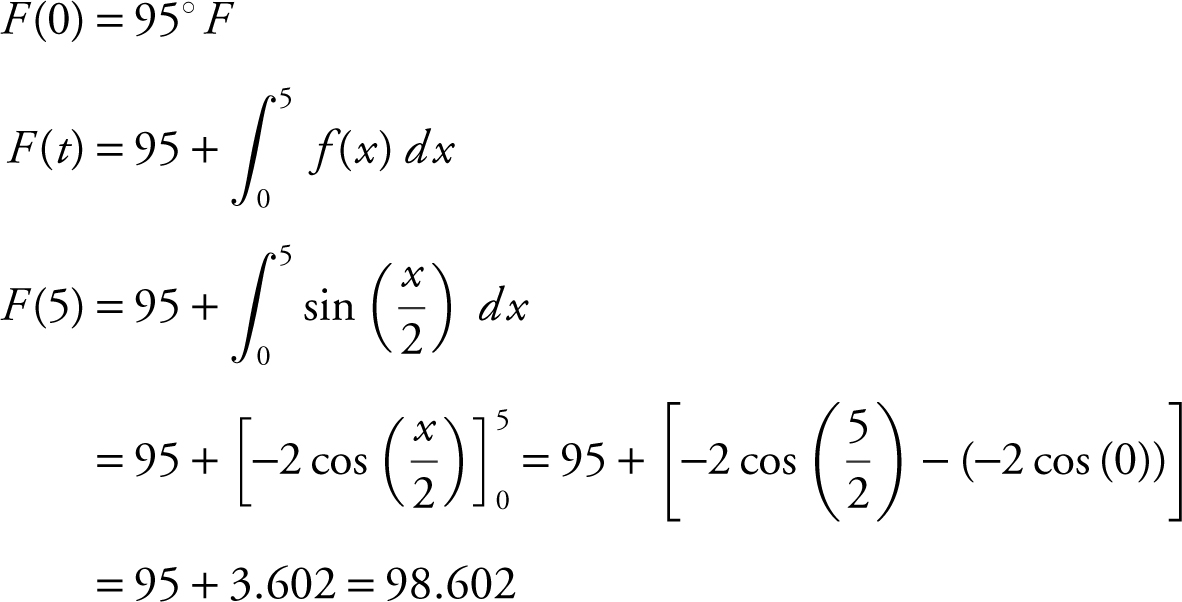
The temperature in the greenhouse at 5 p.m. is 98.602°F.
Leakage Problems
Example
Water is leaking from a faucet at the rate of l (t ) = 10e -0.5t gallons per hour, where t is measured in hours. How many gallons of water will have leaked from the faucet after a 24-hour period?
Let L (x ) represent the number of gallons that have leaked after x hours.
Using your calculator, enter ∫ (10e ^(−0.5x ), x , 0, 24) and obtain 19.9999. Thus, the number of gallons of water that have leaked after x hours is approximately 20 gallons.
• You are permitted to use the following 4 built-in capabilities of your calculator to obtain an answer: plotting the graph of a function, finding the zeros of a function, finding the numerical derivative of a function, and evaluating a definite integral. All other capabilities of your calculator can only be used to check your answer. For example, you may not use the built-in [Inflection ] function of your calculator to find points of inflection. You must use calculus using derivatives and showing change of concavity.
Growth Problem
Example
On a farm, the animal population is increasing at a rate which can be approximately represented by g (t ) = 20 + 50 ln(2 + t ), where t is measured in years. How much will the animal population increase to the nearest tens between the third and fifth years?
Let G (x ) be the increase in animal population after x years.
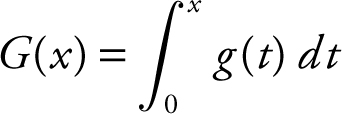
Thus, the population increase between the third and fifth years
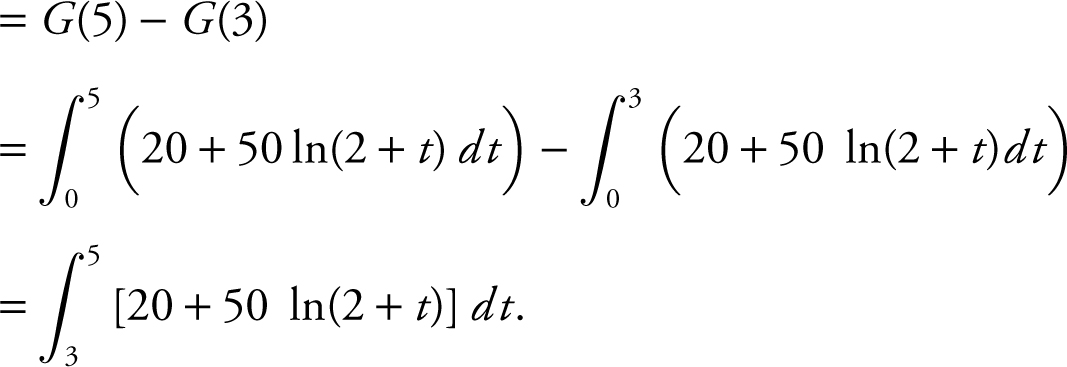
Enter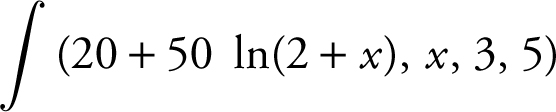 
and obtain 218.709.
Thus the animal population will increase by approximately 220 between the third and fifth years.
14.4 Differential Equations
Main Concepts: Exponential Growth/Decay Problems, Separable Differential Equations
Exponential Growth/Decay Problems
1. If 
, then the rate of change of y is proportional to y .
2. If y is a differentiable function of t with 
, then y (t ) = y 0 e kt ; where y 0 is initial value of y and k is constant. If k > 0, then k is a growth constant and if k < 0, then k is the decay constant.
Example 1—Population Growth
If the amount of bacteria in a culture at any time increases at a rate proportional to the amount of bacteria present and there are 500 bacteria after one day and 800 bacteria after the third day:
(a) approximately how many bacteria are there initially, and
(b) approximately how many bacteria are there after 4 days?
Solution:
(a) Since the rate of increase is proportional to the amount of bacteria present, then:
where y is the amount of bacteria at any time.
Therefore, this is an exponential growth/decay model: y (t ) = y 0 e kt .
Step 1: y (1) = 500 and y (3) = 800
500 = y 0 e k and 800 = y 0 e 3k
Step 2: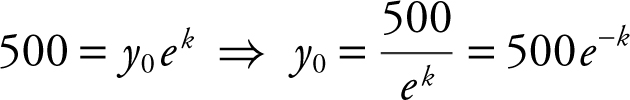 
Substitute y 0 = 500e -k into 800 = y 0 e 3k .
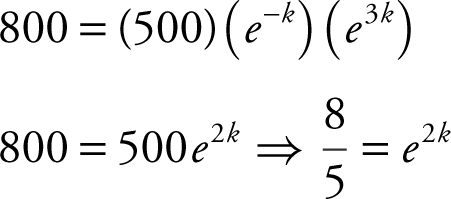
Take the ln of both sides :
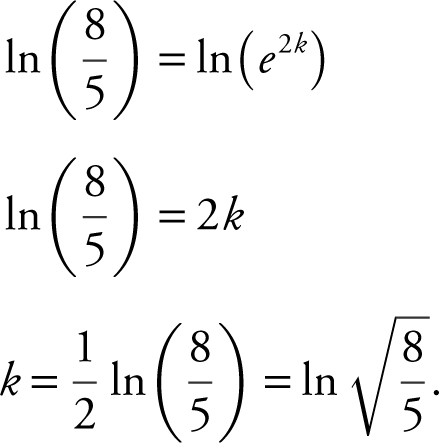
Step 3: Substitute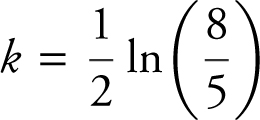 
into one of the equations.
Thus, there are 395 bacteria present initially.
(b) 
Thus there are approximately 1012 bacteria present after 4 days.
• Get a good night’s sleep the night before. Have a light breakfast before the exam.
Example 2—Radioactive Decay
Carbon-14 has a half-life of 5750 years. If initially there are 60 grams of carbon-14, how many grams are left after 3000 years?
Step 1: y (t ) = y 0 e kt = 60e kt
Since half-life is 5750 years,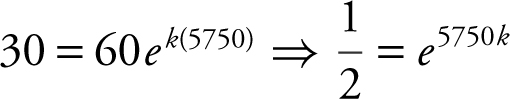 
.
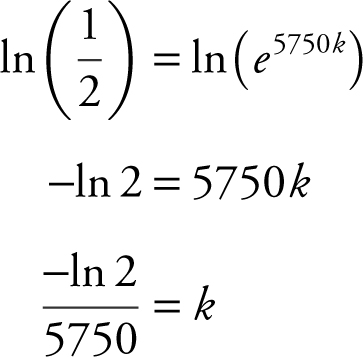
Step 2: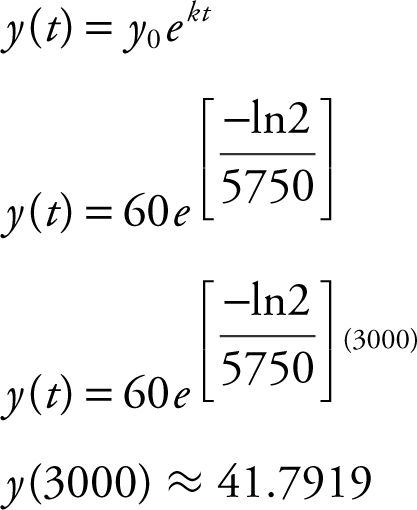 
Thus, there will be approximately 41.792 grams of carbon-14 after 3000 years.
Separable Differential Equations
General Procedure
Steps:
1. Separate the variables: g (y )dy = f (x )dx .
2. Integrate both sides: ∫g (y )dy = ∫ f (x )dx .
3. Solve for y to get a general solution.
4. Substitute given conditions to get a particular solution.
5. Verify your result by differentiating.
Example 1
Given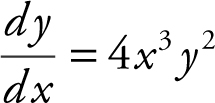 
and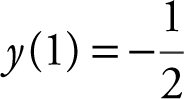 
, solve the differential equation.
Step 1: Separate the variables: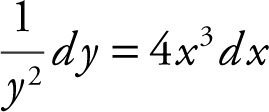 
.
Step 2: Integrate both sides: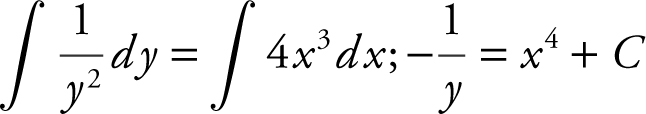 
.
Step 3: General solution: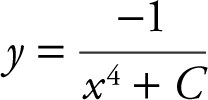 
.
Step 4: Particular solution: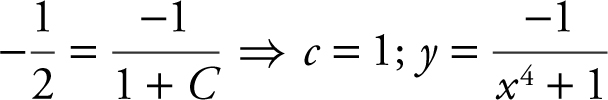 
.
Step 5: Verify result by differentiating.
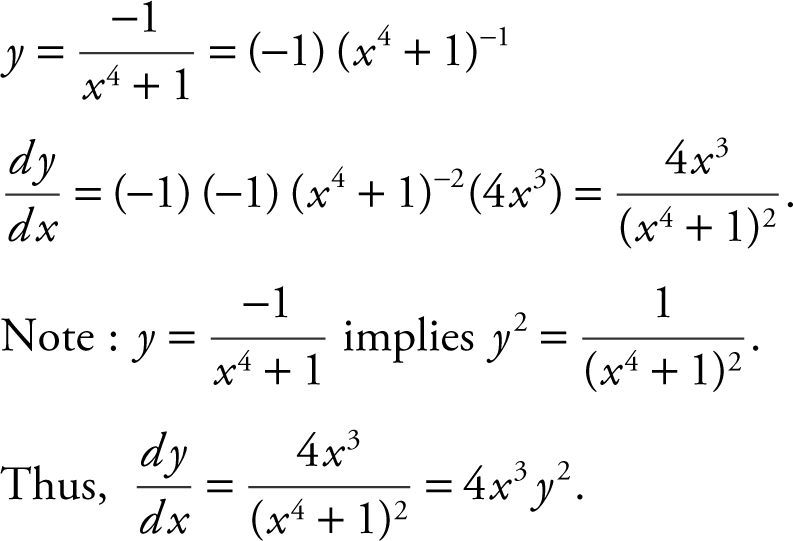
Example 2
Find a solution of the differentiation equation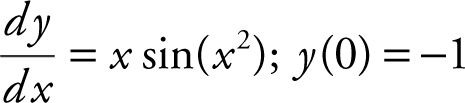 
.
Step 1: Separate variables: dy = x sin(x 2 )dx .
Step 2: Integrate both sides: 
.
Let u = x 2 ; du = 2x dx or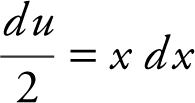 
.
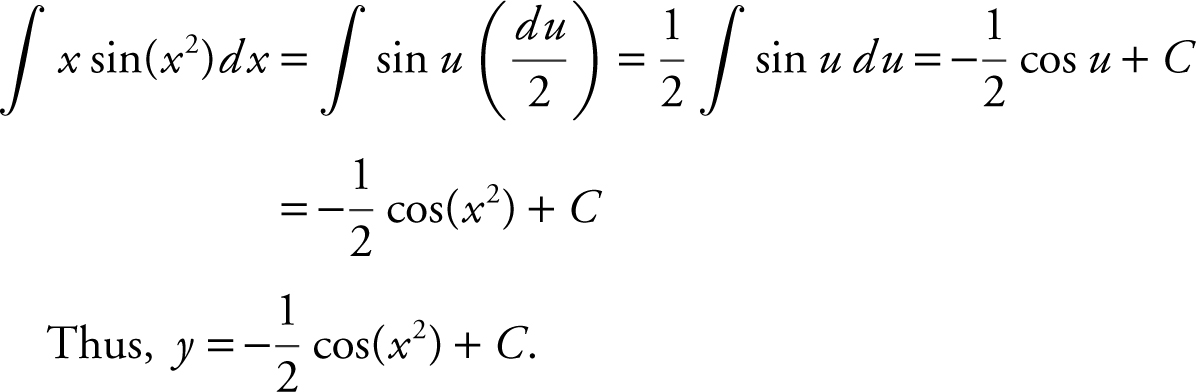
Step 3: Substitute given condition:
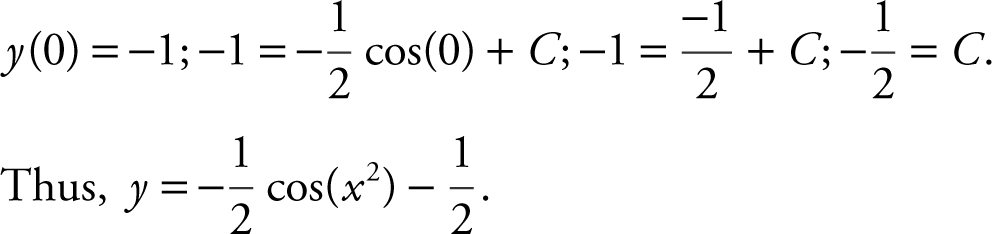
Step 4: Verify result by differentiating:
Example 3
If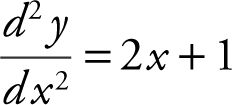 
and at x = 0, y ′ = –1, and y = 3, find a solution of the differential equation.
Step 1: Rewrite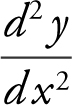 
as 
.
Step 2: Separate variables: dy ′ = (2x + 1)dx .
Step 3: Integrate both sides: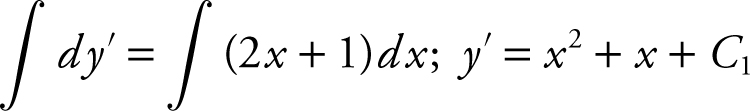 
.
Step 4: Substitute given condition: At x = 0, y ′ = –1; –1 = 0 + 0 + C 1 ⇒ C 1 = –1. Thus, y ′ = x 2 + x – 1.
Step 5: Rewrite: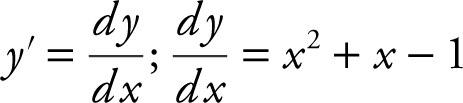 
.
Step 6: Separate variables: dy = (x 2 + x – 1)dx .
Step 7: Integrate both sides: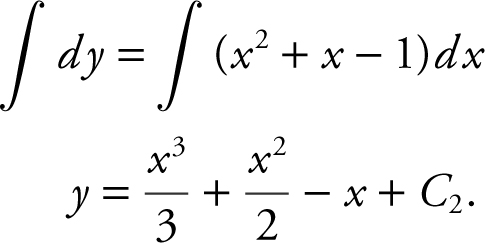 
Step 8: Substitute given condition: At x = 0, y = 3; 3 = 0 + 0 – 0 + C 2 ⇒ C 2 = 3.
Therefore,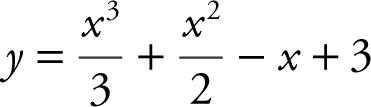 
.
Step 9: Verify result by differentiating:
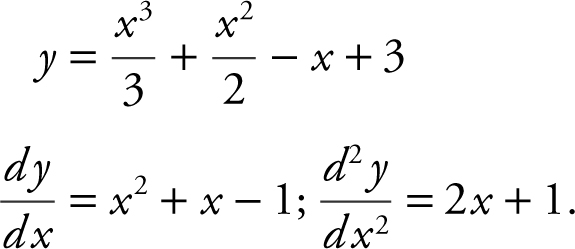
Example 4
Find the general solution of the differential equation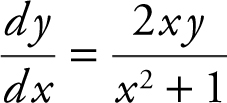 
.
Step 1: Separate variables:
.
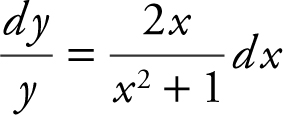Step 2: Integrate both sides: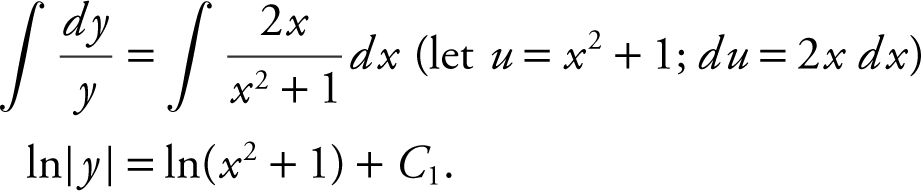 
Step 3: General solution: solve for y .
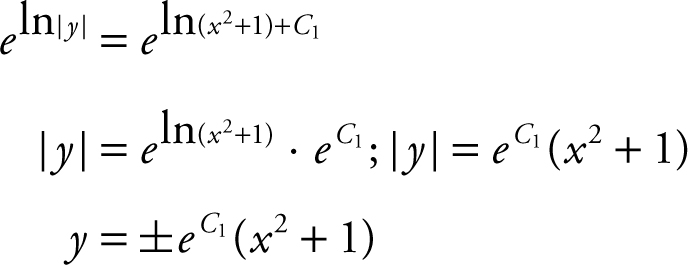
The general solution is y = C (x 2 + 1).
Step 4: Verify result by differentiating:
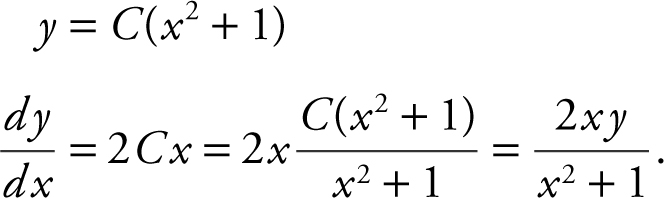
Example 5
Write an equation for the curve that passes through the point (3, 4) and has a slope at any point (x , y ) as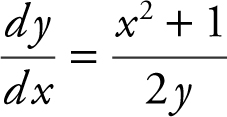 
.
Step 1: Separate variables: 2y dy = (x 2 + 1)dx .
Step 2: Integrate both sides: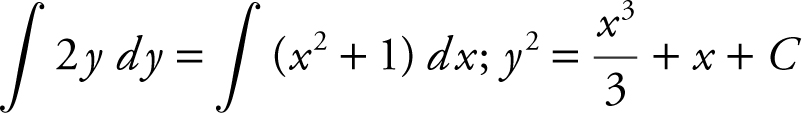 
.
Step 3: Substitute given condition: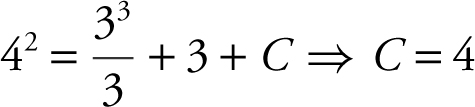 
.
Thus,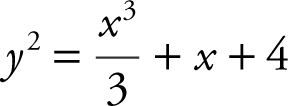 
.
Step 4: Verify the result by differentiating:
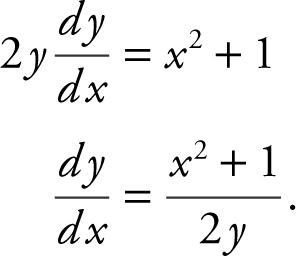
14.5 Slope Fields
Main Concepts: Slope Fields, Solution of Different Equations
A slope field (or a direction field ) for first-order differential equations is a graphic representation of the slopes of a family of curves. It consists of a set of short line segments drawn on a pair of axes. These line segments are the tangents to a family of solution curves for the differential equation at various points. The tangents show the direction which the solution curves will follow. Slope fields are useful in sketching solution curves without having to solve a differential equation algebraically.
Example 1
If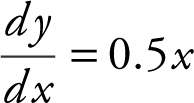 
, draw a slope field for the given differential equation.
Step 1: Set up a table of values for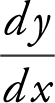 
for selected values of x .
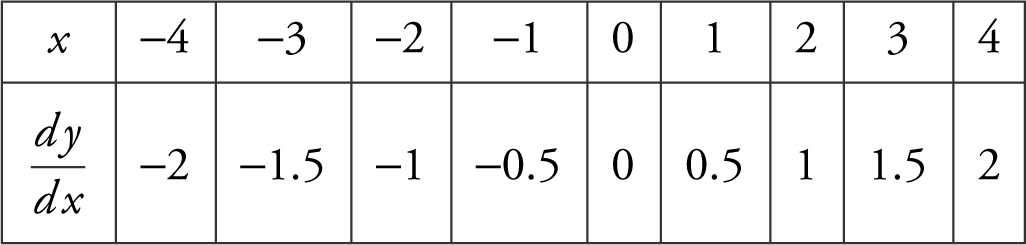
Note that since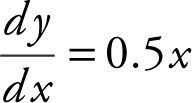 
, the numerical value of 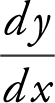is independent of the value of y . For example, at the points (1, –1), (1, 0), (1, 1), (1, 2), (1, 3), and at all the points whose x -coordinates are 1, the numerical value of 
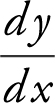is 0.5 regardless of their y -coordinates. Similarly, for all the points, whose x -coordinates are 2 (e.g., (2, – 1), (2, 0), (2, 3), etc.), 
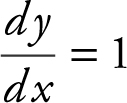. Also, remember that 
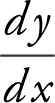represents the slopes of the tangent lines to the curve at various points. You are now ready to draw these tangents.
Step 2: Draw short line segments with the given slopes at the various points. The slope field for the differential equation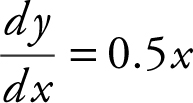 
is shown in Figure 14.5-1 .
Figure 14.5-1
Example 2
Figure 14.5-2 shows a slope field for one of the differential equations given below. Identify the equation.
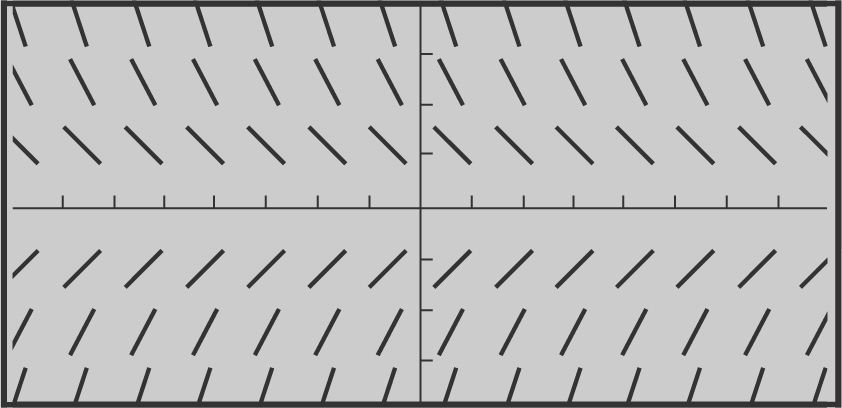
Figure 14.5-2
(a) 
(b)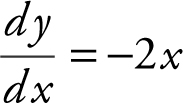 
(c)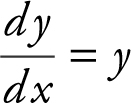 
(d) 
(e)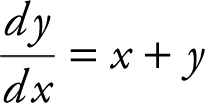 
Solution:
If you look across horizontally at any row of tangents, you’ll notice that the tangents have the same slope. (Points on the same row have the same y -coordinate but different x -coordinates.) Therefore, the numerical value of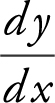 
(which represents the slope of the tangent) depends solely on the y -coordinate of a point and it is independent of the x -coordinate. Thus, only choice (c) and choice (d) satisfy this condition. Also notice that the tangents have a negative slope when y > 0 and have a positive slope when y < 0.
Therefore, the correct choice is (d),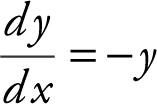 
.
Example 3
A slope field for a differential equation is shown in Figure 14.5-3 . Draw a possible graph for the particular solution y = f (x ) to the differential equation function, if (a) the initial condition is f (0) = –2 and (b) the initial condition is f(0) = 0.
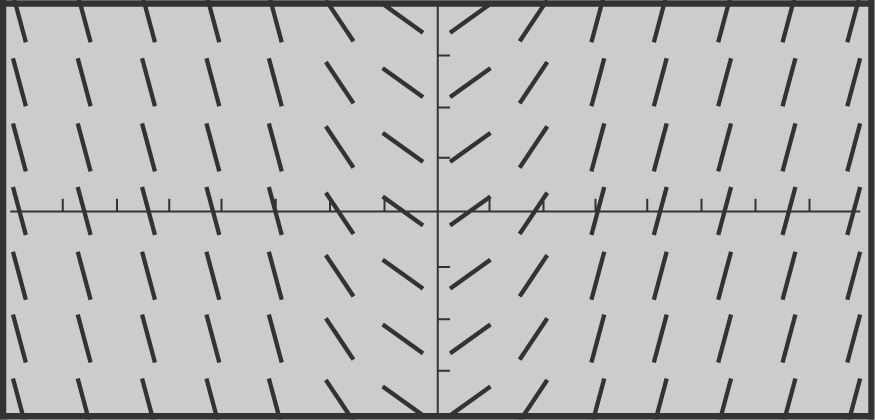
Figure 14.5-3
Solution:
Begin by locating the point (0, –2) as given in the initial condition. Follow the flow of the field and sketch the graph of the function. Repeat the same procedure with the point (0, 0). See the curves as shown in Figure 14.5-4 .
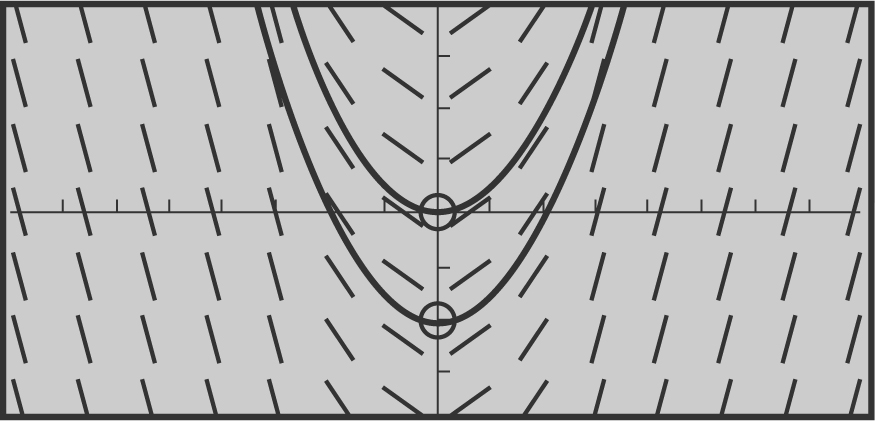
Figure 14.5-4
Example 4
Given the differential equation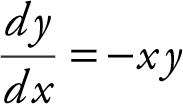 
.
(a) Draw a slope field for the differential equation at the 15 points indicated on the provided set of axes in Figure 14.5-5 .
Figure 14.5-5
(b) Sketch a possible graph for the particular solution y = f (x ) to the differential equation with the initial condition f (0) = 3.
(c) Find, algebraically, the particular solution y = f (x ) to the differential equation with the initial condition f (0) = 3.
Solution:
(a) Set up a table of values for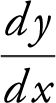 
at the 15 given points.
Then sketch the tangents at the various points as shown in Figure 14.5-6 .
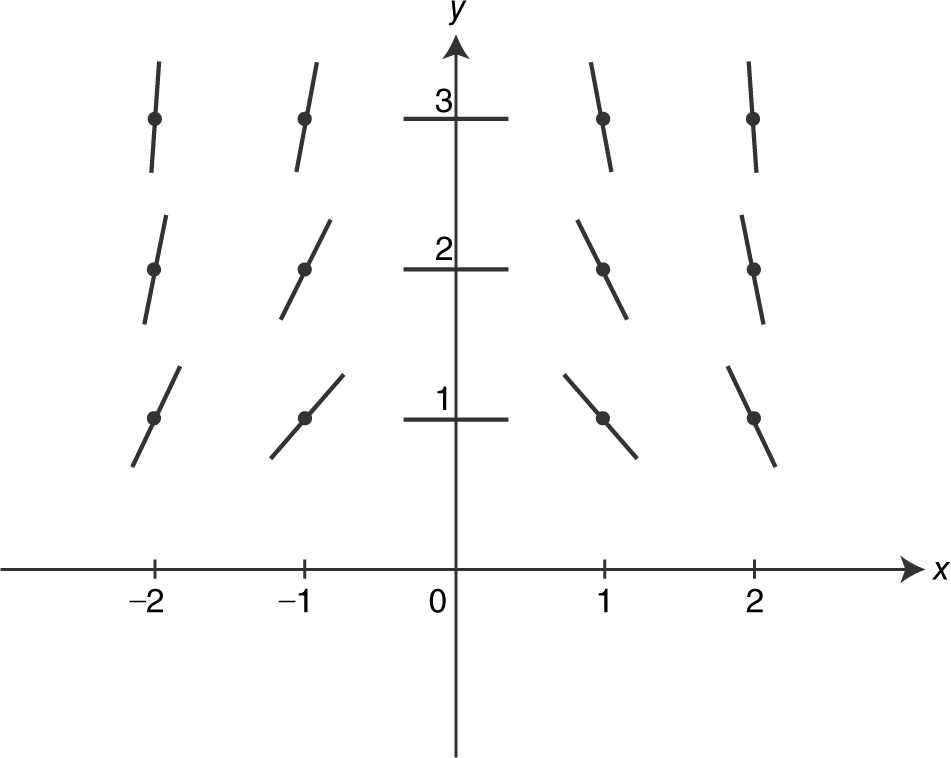
Figure 14.5-6
(b) Locate the point (0, 3) as indicated in the initial condition. Follow the flow of the field and sketch the curve as shown Figure 14.5-7 .
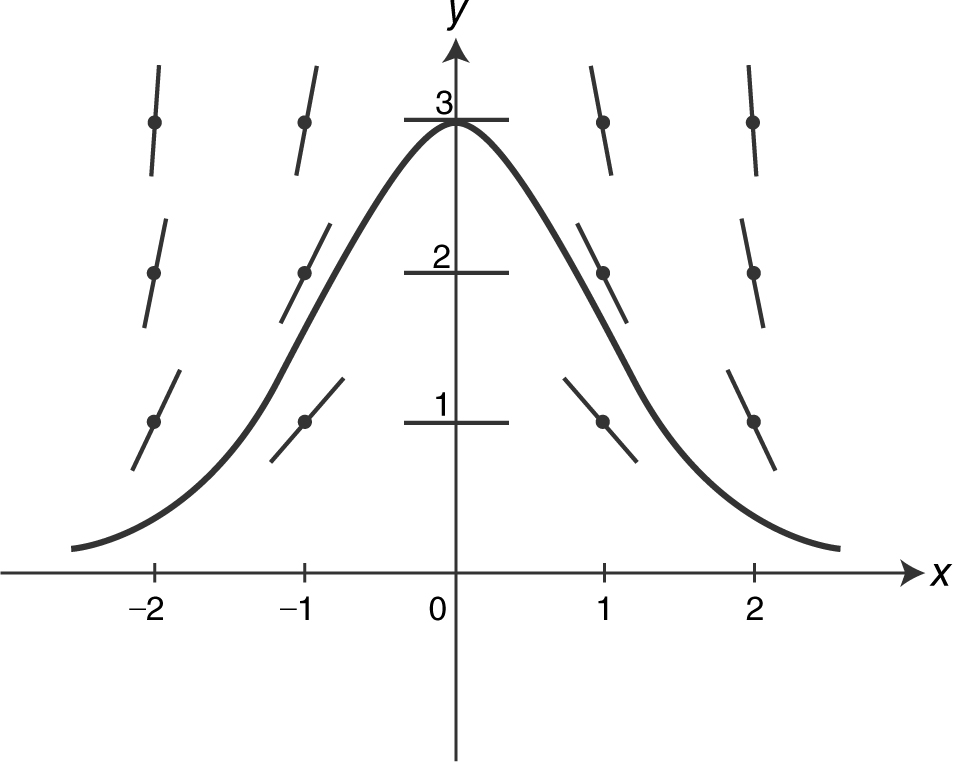
Figure 14.5-7
(c) Step 1: Rewrite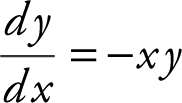 
as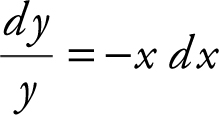 
.
Step 2: Integrate both sides 
and obtain ln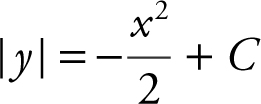 
.
Step 3: Apply the exponential function to both sides and obtain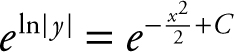 
.
Step 4: Simplify the equation and get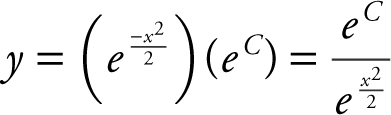 
.
Let k = e C and you have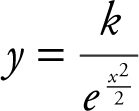 
.
Step 5: Substitute initial condition (0, 3) and obtain k = 3. Thus, you have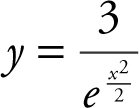 
.
14.6 Rapid Review
1. Find the average value of y = sin x on [0, π ].
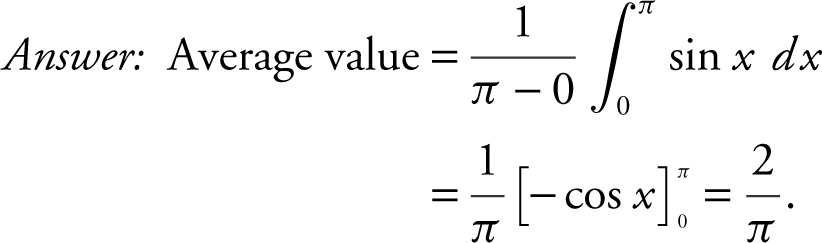
2. Find the total distance traveled by a particle during 0 ≤ t ≤ 3 whose velocity function is shown in Figure 14.6-1 .
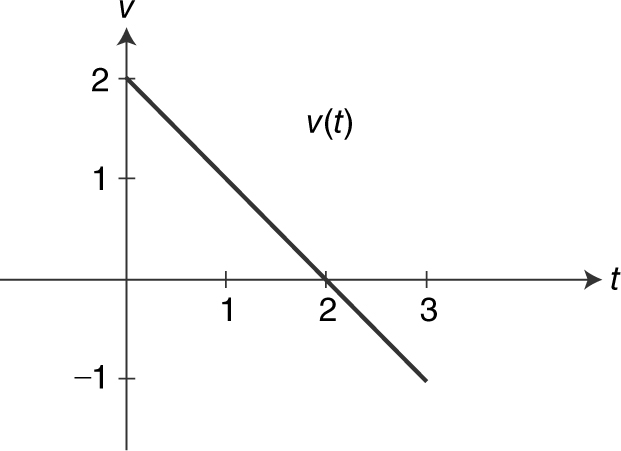
Figure 14.6-1
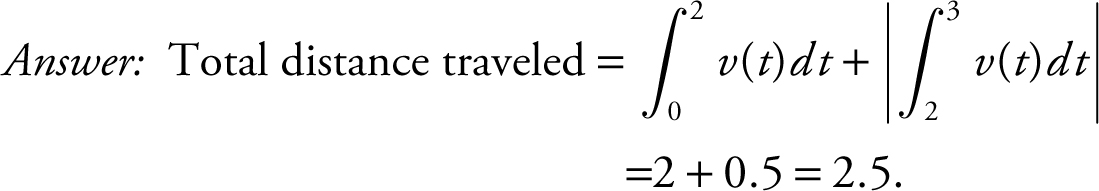
3. Oil is leaking from a tank at the rate of f (t ) = 5e -0.1t gallons/hour, where t is measured in hours. Write an integral to find the total number of gallons of oil that will have leaked from the tank after 10 hours. Do not evaluate the integral.
.
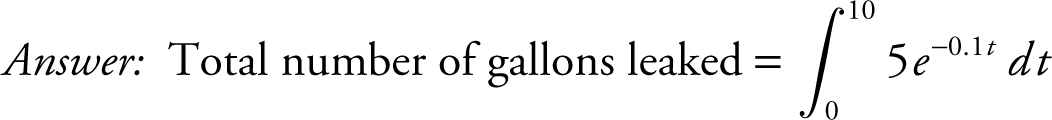4. How much money should Mary invest at 7.5% interest a year compounded continuously, so that she will have $100,000 after 20 years.
Answer: y (t ) = y 0 e kt , k = 0.075, and t = 20. y (20) = 100,000 = y 0 e (0.075)(20) . Thus, using a calculator, you obtain y 0 ≈ 22313, or $22,313.
5. Given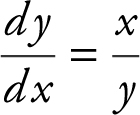 
and y (1) = 0, solve the differential equation.
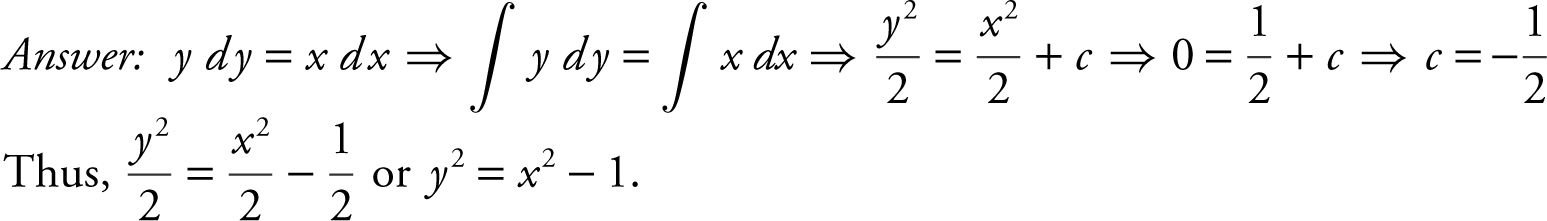
6. Identify the differential equation for the slope field shown.
Answer: The slope field suggests a hyperbola of the form y 2 – x 2 = k , so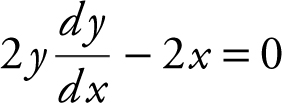 
and 
.
14.7 Practice Problems
Part A—The use of a calculator is not allowed.
1 . Find the value of c as stated in the Mean Value Theorem for Integrals for f (x ) = x 3 on [2, 4].
2 . The graph of f is shown in Figure 14.7-1 . Find the average value of f on [0, 8].
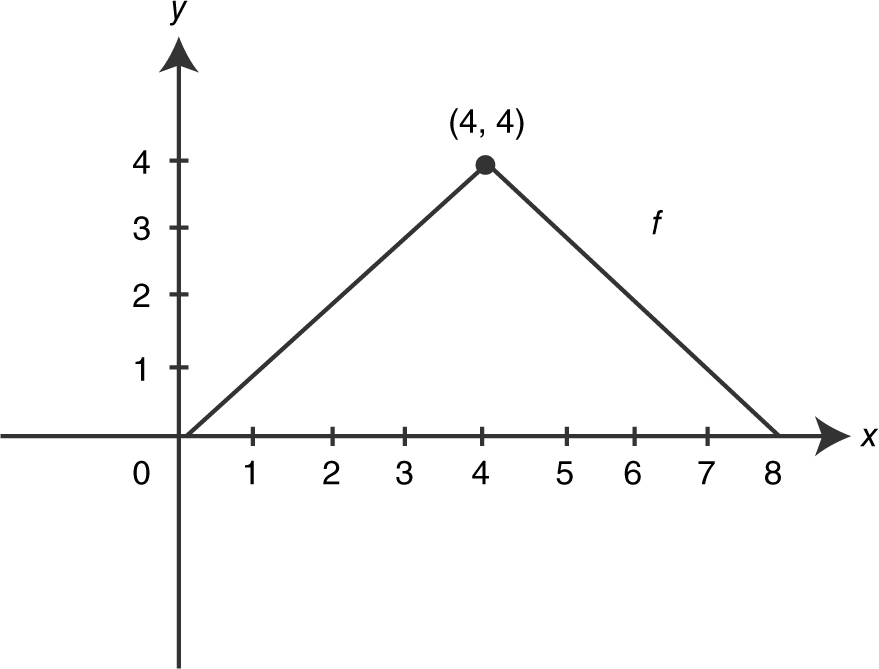
Figure 14.7-1
3 . The position function of a particle moving on a coordinate line is given as s (t ) = t 2 – 6t – 7, 0 ≤ t ≤ 10. Find the displacement and total distance traveled by the particle from 1 ≤ t ≤ 4.
4 . The velocity function of a moving particle on a coordinate line is v (t ) = 2t + 1 for 0 ≤ t ≤ 8. At t = 1, its position is –4. Find the position of the particle at t = 5.
5 . The rate of depreciation for a new piece of equipment at a factory is given as p (t ) = 50t – 600 for 0 ≤ t ≤ 10, where t is measured in years. Find the total loss of value of the equipment over the first 5 years.
6 . If the acceleration of a moving particle on a coordinate line is a (t ) = –2 for 0 ≤ t ≤ 4, and the initial velocity v 0 = 10, find the total distance traveled by the particle during 0 ≤ t ≤ 4.
7 . The graph of the velocity function of a moving particle is shown in Figure 14.7-2 . What is the total distance traveled by the particle during 0 ≤ t ≤ 12?
Figure 14.7-2
8 . If oil is leaking from a tanker at the rate of f (t ) = 10e 0.2t gallons per hour where t is measured in hours, how many gallons of oil will have leaked from the tanker after the first 3 hours?
9 . The change of temperature of a cup of coffee measured in degrees Fahrenheit in a certain room is represented by the function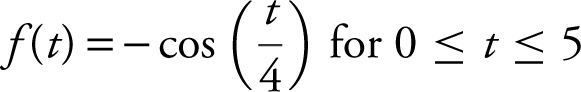 
, where t is measured in minutes. If the temperature of the coffee is initially 92°F, find its temperature after the first 5 minutes.
10 . If the half-life of a radioactive element is 4500 years, and initially there are 100 grams of this element, approximately how many grams are left after 5000 years?
11 . Find a solution of the differential equation:
.
12 . If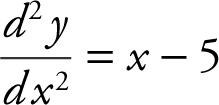 
and at x = 0, y ′ = –2 and y = 1, find a solution of the differential equation.
Part B—Calculators are allowed.
13 . Find the average value of y = tan x from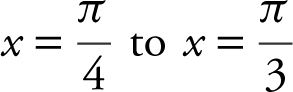 
.
14 . The acceleration function of a moving particle on a straight line is given by a (t ) = 3e 2t , where t is measured in seconds, and the initial velocity is 
. Find the displacement and total distance traveled by the particle in the first 3 seconds.
15 . The sales of an item in a company follow an exponential growth/decay model, where t is measured in months. If the sales drop from 5000 units in the first month to 4000 units in the third month, how many units should the company expect to sell during the seventh month?
16 . Find an equation of the curve that has a slope of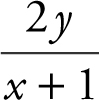 
at the point (x , y ) and passes through the point (0, 4).
17 . The population in a city was approximately 750,000 in 1980, and grew at a rate of 3% per year. If the population growth followed an exponential growth model, find the city’s population in the year 2002.
18 . Find a solution of the differential equation 4e y = y ′ – 3xe y and y (0) = 0.
19 . How much money should a person invest at 6.25% interest compounded continuously so that the person will have $50,000 after 10 years?
20 . The velocity function of a moving particle is given as v (t ) = 2 – 6e -t , t ≥ 0 and t is measured in seconds. Find the total distance traveled by the particle during the first 10 seconds.
14.8 Cumulative Review Problems
(Calculator) indicates that calculators are permitted.
21 . If 3e y = x 2 y , find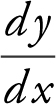 
.
22 . Evaluate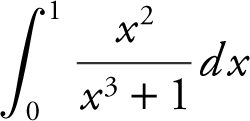 
.
23 . The graph of a continuous function f which consists of three line segments on [–2, 4] is shown in Figure 14.8-1 . If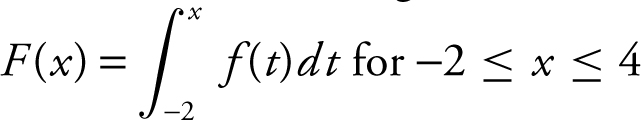 
,
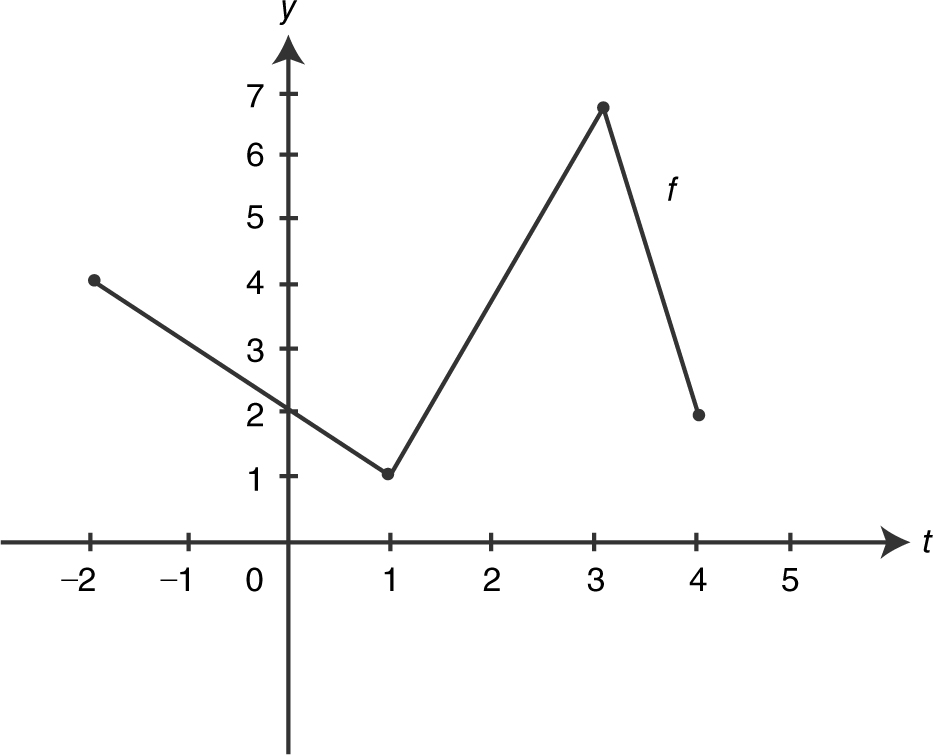
Figure 14.8-1
(a) Find F (–2) and F (0).
(b) Find F ′(0) and F ′(2).
(c) Find the value of x such that F has a maximum on [–2, 4].
(d) On which interval is the graph of F concave upward?
24 . (Calculator) The slope of a function y = f (x ) at any point (x , y ) is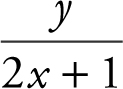 
and f (0) = 2.
(a) Write an equation of the line tangent to the graph of f at x = 0.
(b) Use the tangent in part (a) to find the approximate value of f (0.1).
(c) Find a solution y = f (x ) for the differential equation.
(d) Using the result in part (c), find f (0.1).
25 . (Calculator) Let R be the region in the first quadrant bounded by f (x ) = e x – 1 and g (x ) = 3 sin x .
(a) Find the area of region R .
(b) Find the volume of the solid obtained by revolving R about the x -axis.
(c) Find the volume of the solid having R as its base and semicircular cross sections perpendicular to the x -axis.
14.9 Solutions to Practice Problems
Part A—The use of a calculator is not allowed.
1 . 
2 .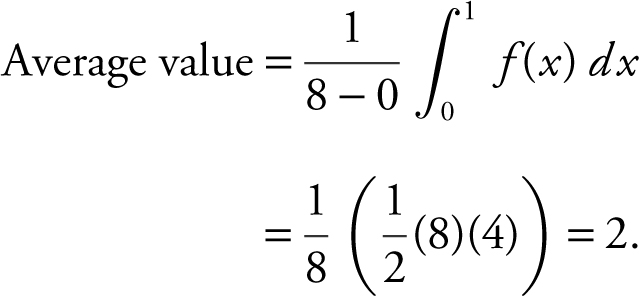 
3 . 
4 . 
5 .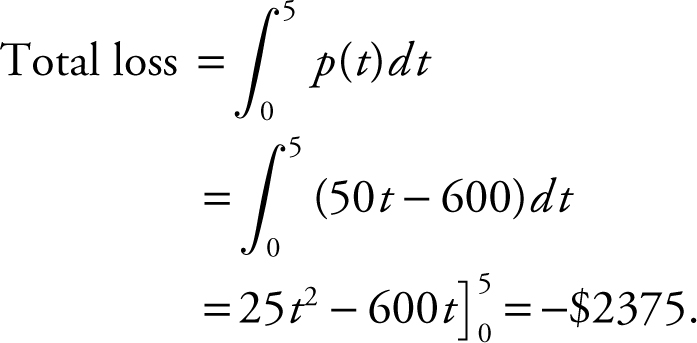 
6 .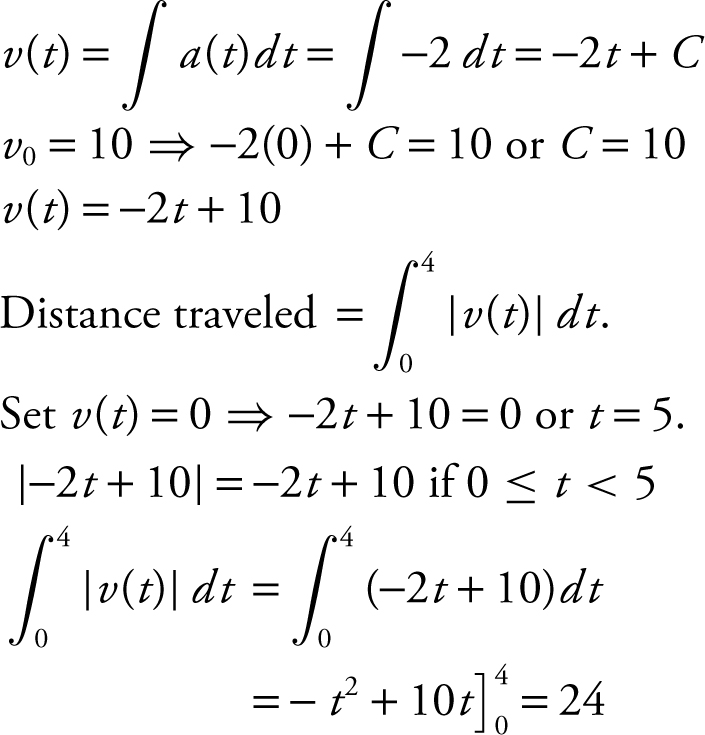 
7 . Total Distance Traveled
8 .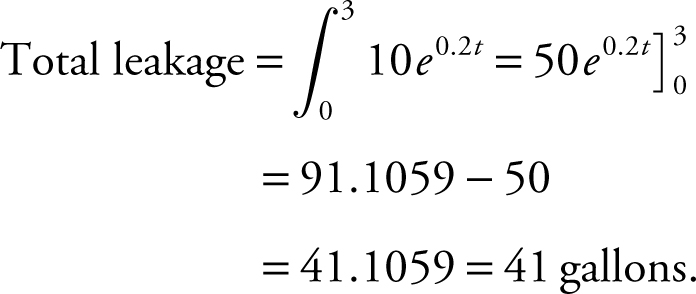 
9 . Total change in temperature
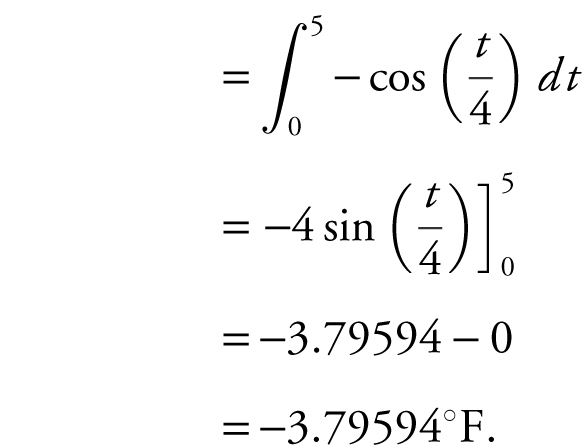
Thus the temperature of coffee after 5 minutes is (92 – 3.79594) ≈ 88.204°F.
10 . y (t ) = y 0 e kt
Half-life = 4500 years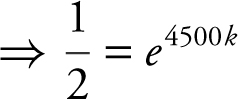 
.
Take ln of both sides:
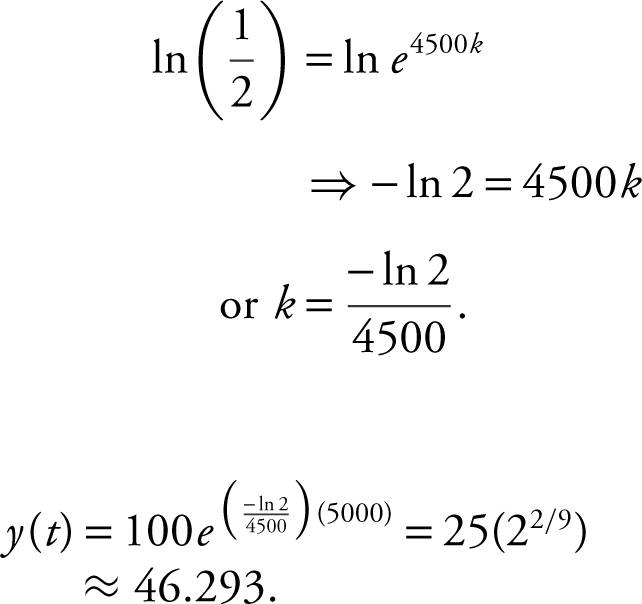
There are approximately 46.29 grams left.
11 . Step 1: Separate variables:
dy = x cos(x 2 ) dx .
Step 2: Integrate both sides:
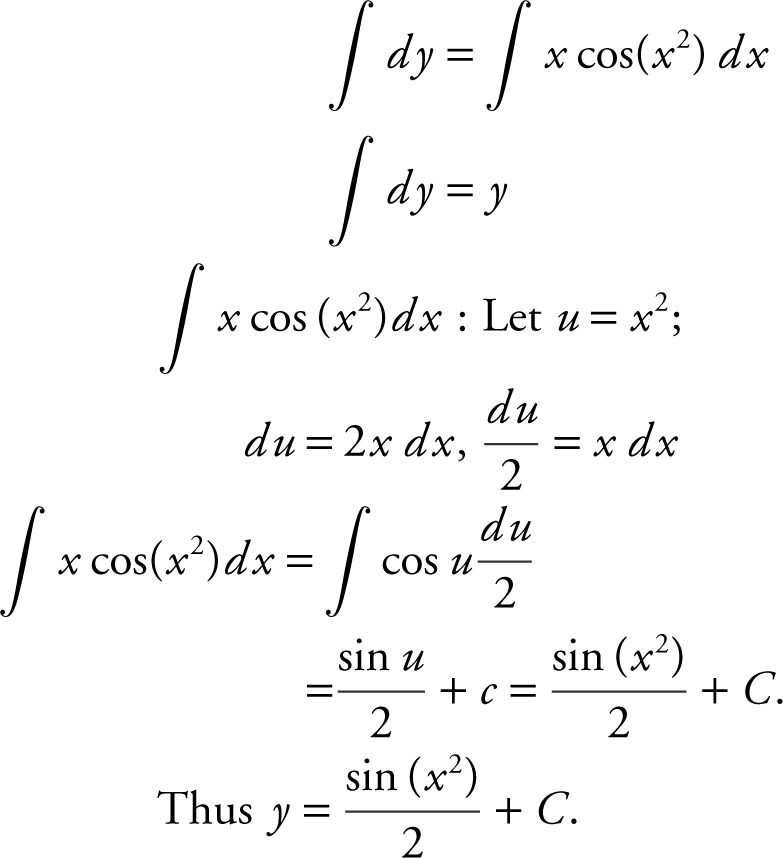
Step 3: Substitute given values.
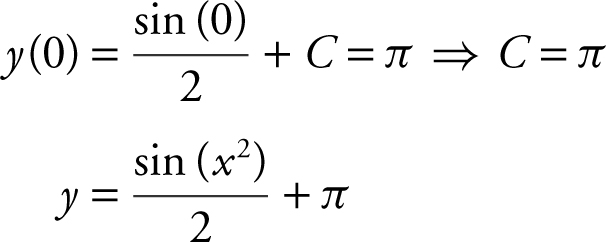
Step 4: Verify result by differentiating:
.
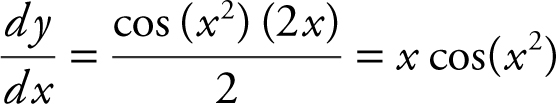12 . Step 1: Rewrite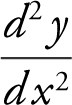 
as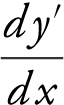 
.
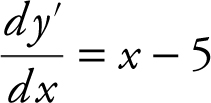Step 2: Separate variables:
dy ′ = (x – 5)dx .
Step 3: Integrate both sides:
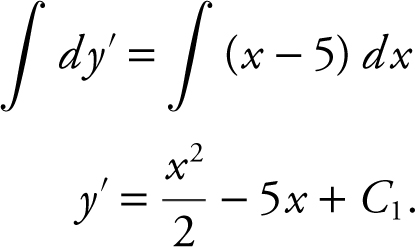
Step 4: Substitute given values:
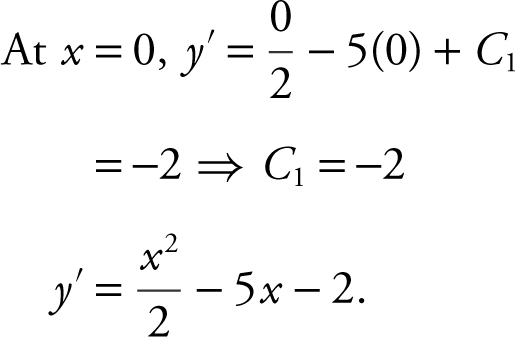
Step 5: Rewrite: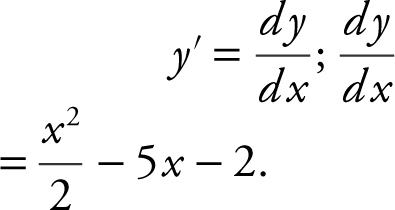 
Step 6: Separate variables:
.
Step 7: Integrate both sides:
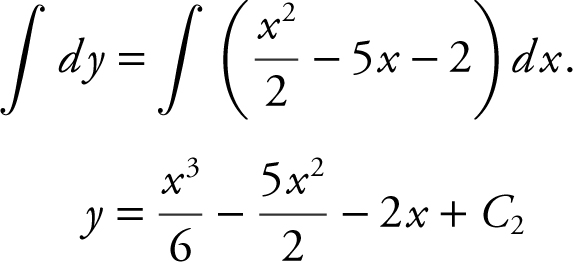
Step 8: Substitute given values:
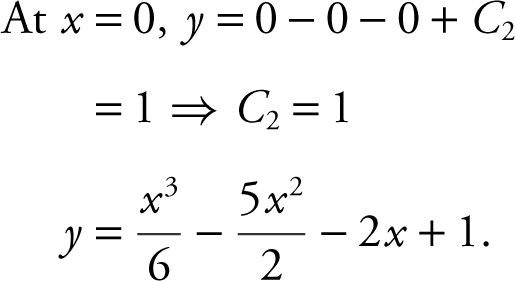
Step 9: Verify result by differentiating:
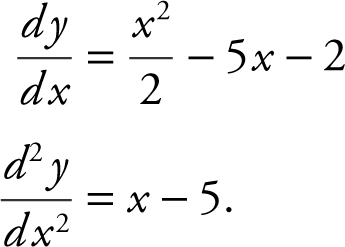
Part B—Calculators are allowed.
13 . Average value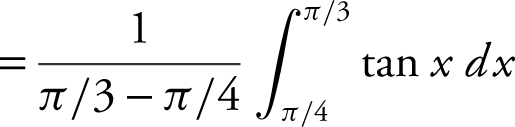 
.
Enter = (1/(π /3 – π /4)) (tan x, x, π /4, π /3)
and obtain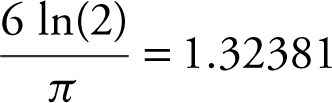 
.
14 .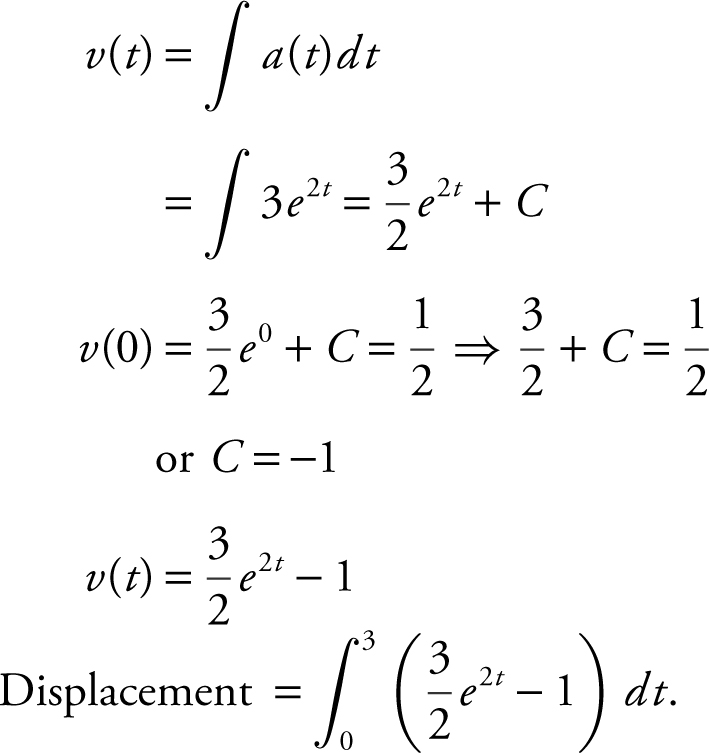 
Enter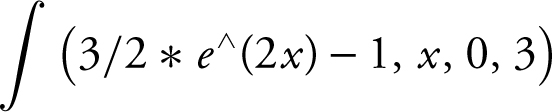 
and obtain 298.822.
Distance traveled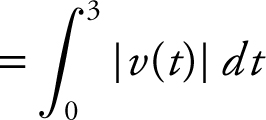 
.
Since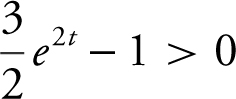 
for t ≥ 0,
.
15 . Step 1: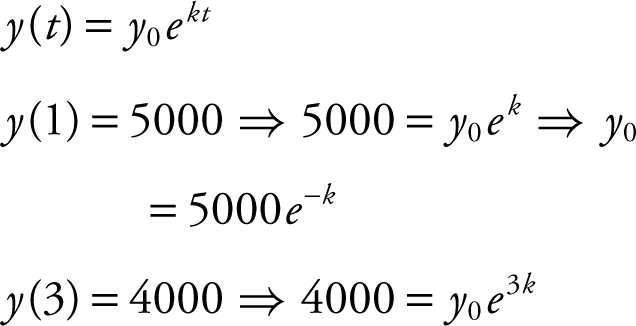 
Substituting:
Step 2: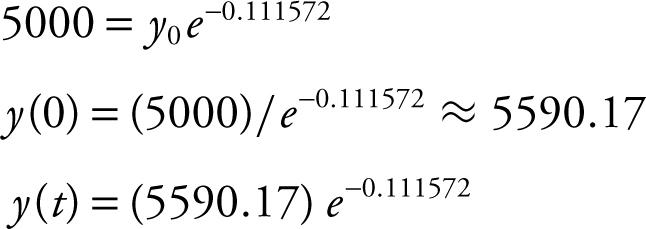 
Step 3: y (7) = (5590.17)e -0.111572(7)
≈ 2560
Thus, sales for the 7th month are approximately 2560 units.
16 . Step 1: Separate variables:
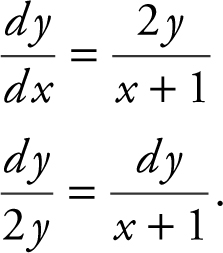
Step 2: Integrate both sides:
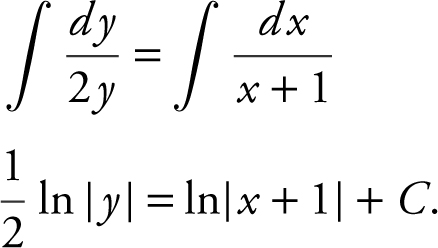
Step 3: Substitute given value (0, 4):
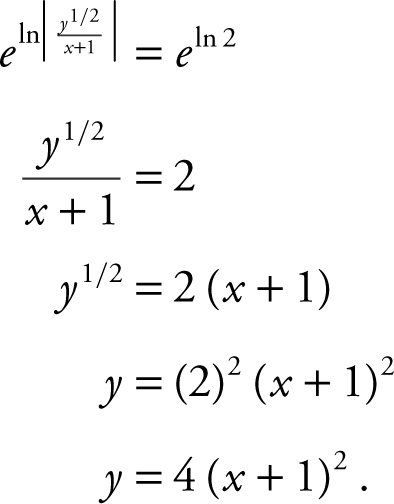
Step 4: Verify result by differentiating:
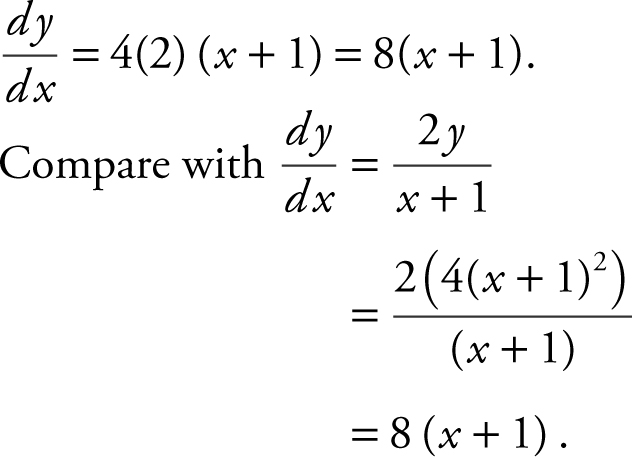
17 . 
18 . Step 1: Separate variables:
Step 2: Integrate both sides:
Step 3: Substitute given value: y (0) = 0 ⇒ e 0 = 0 – 0 + c ⇒ c = 1.
Step 4: Take ln of both sides:
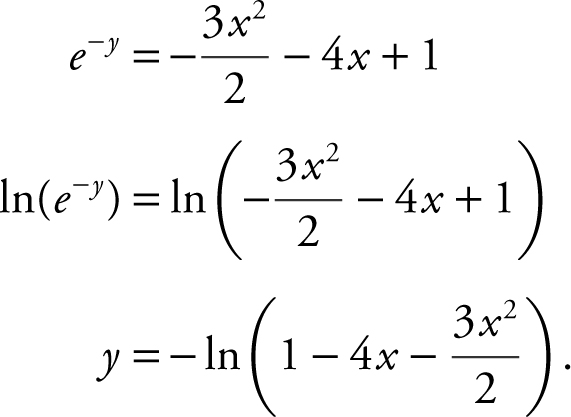
Step 5: Verify result by differentiating: Enter d (– ln(1 – 4x – 3 (x –^2 )/2), x ) and obtain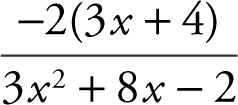 
, which is equivalent to e y (4 + 3x ).
19 .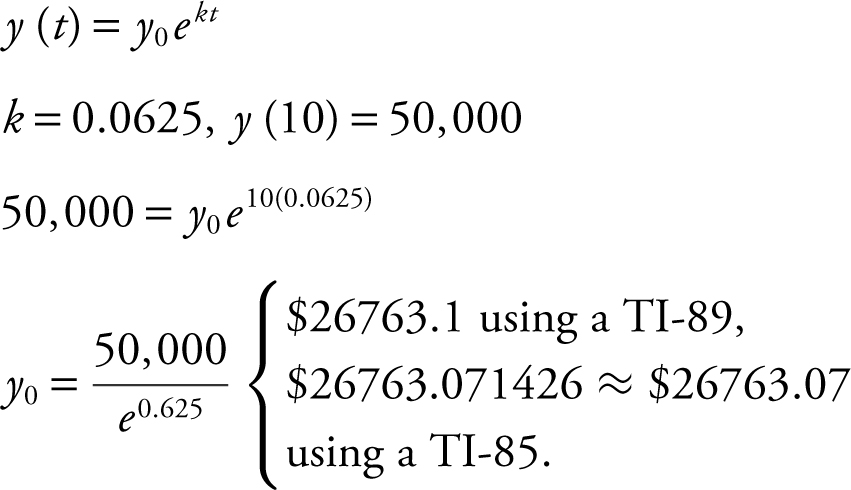 
20 . Set v (t ) = 2 – 6e -t = 0. Using the [Zero ] function on your calculator, compute t = 1.09861.
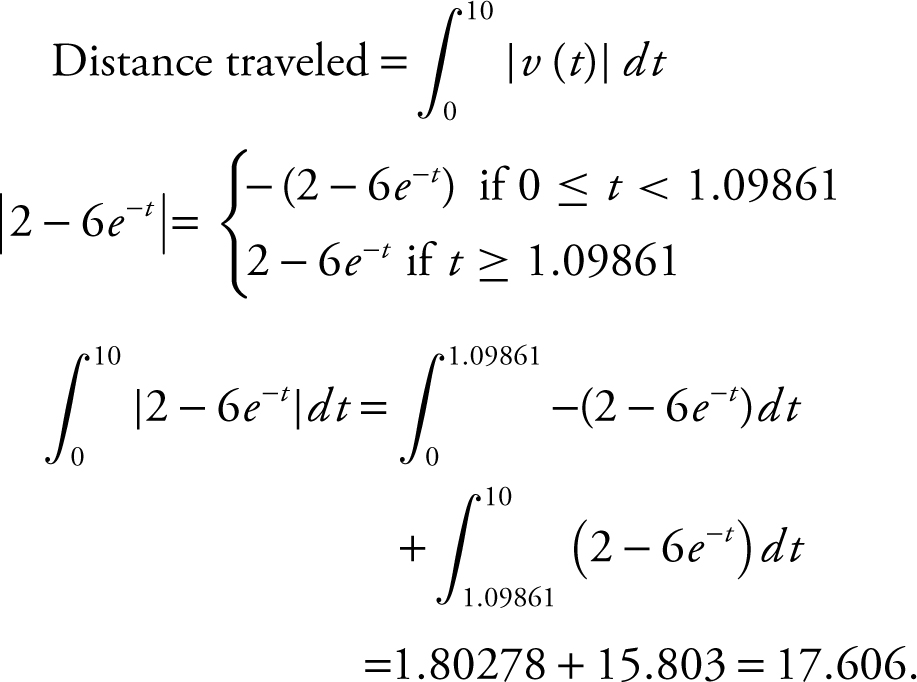
Alternatively, use the [nInt ] function on the calculator.
Enter nInt(abs(2 – 6e ^ (–x )), x , 0, 10) and obtain the same result.
14.10 Solutions to Cumulative Review Problems
21 . 3e y = x 2 y
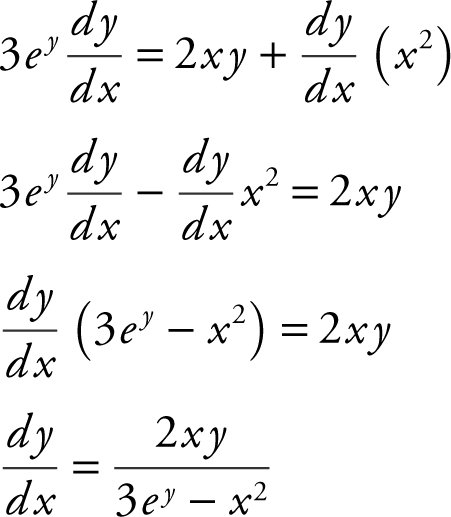
22 .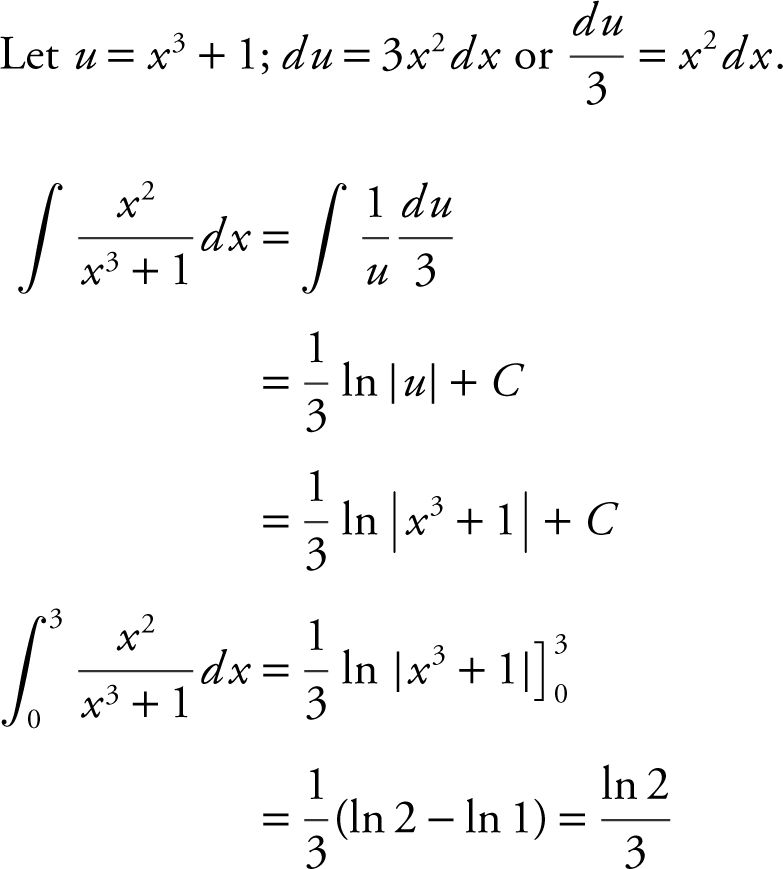 
.
23 . (a)
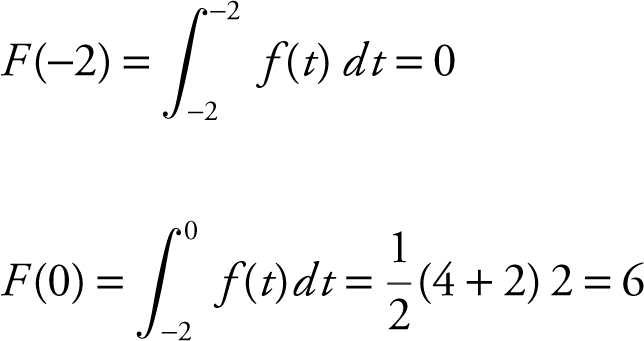
(b) F ′ (x ) = f (x ); F ′ (0) = 2 and F ′ (2) = 4.
(c) Since f > 0 on [–2, 4], F has a maximum value at x = 4.
(d) The function f is increasing on (1, 3) which implies that f ′ > 0 on (1, 3).
Thus, F is concave upward on (1, 3). (Note: f ′ is equivalent to the 2nd derivative of F .)
24 . (a)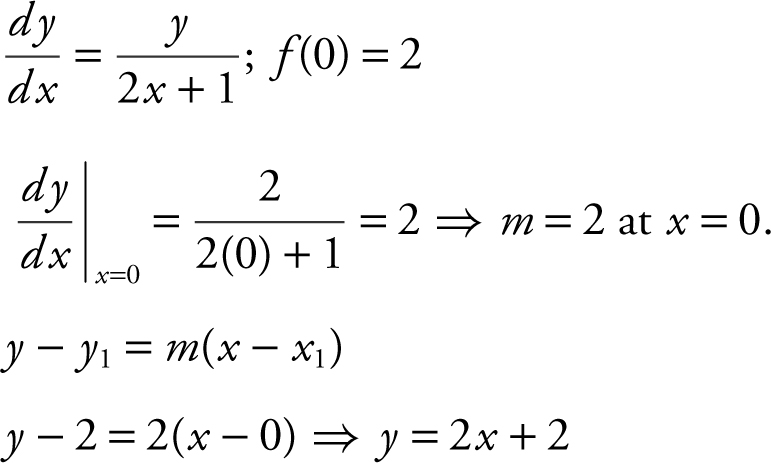 
The equation of the tangent to f at x = 0 is y = 2x + 2.
(b) f (0.1) = 2 (0.1) + 2 = 2.2
(c) Solve the differential equation:
.
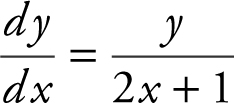Step 1: Separate variables:
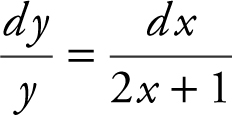
Step 2: Integrate both sides:
.
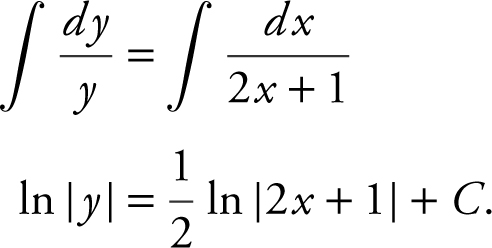Step 3: Substitute given values (0, 2):
.
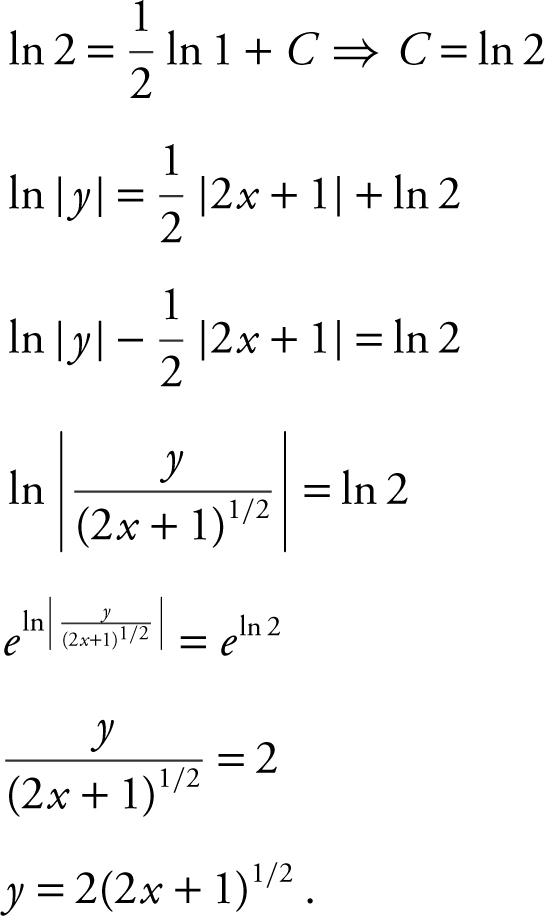Step 4: Verify result by differentiating
.
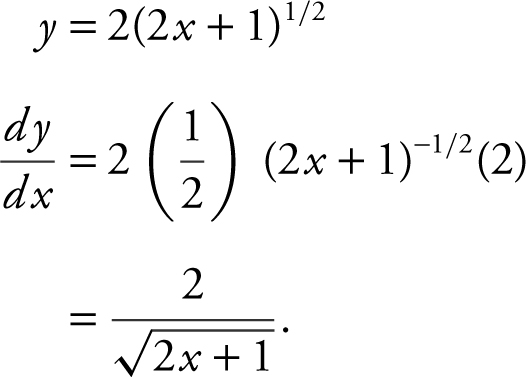Compare this with:
.
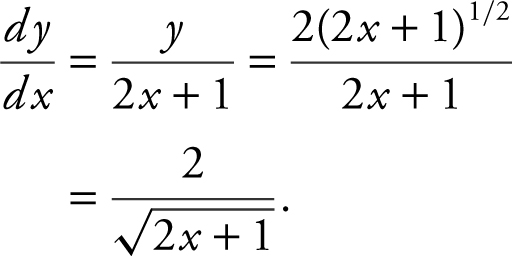Thus, the function is y = f (x ) = 2(2x + 1)1/2 .
(d)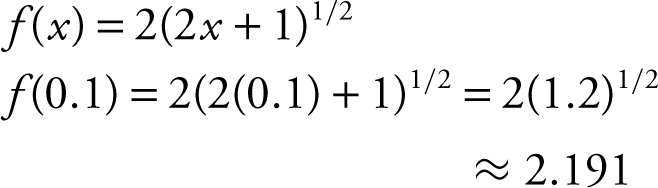 
25 . See Figure 14.10-1 .
Figure 14.10-1
(a) Intersection points: Using the [Intersection ] function on the calculator, you have x = 0 and x = 1.37131.
.
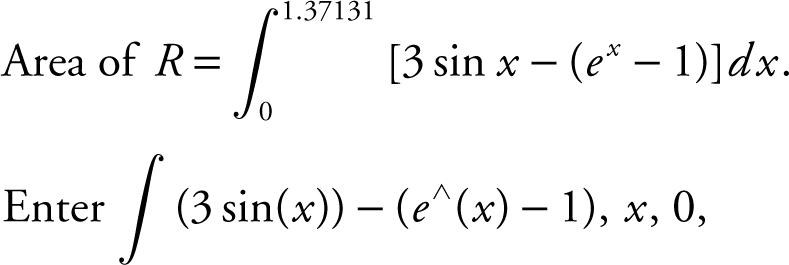1.37131 and obtain 0.836303.
The area of region R is approximately 0.836.
(b) Using the Washer Method, volume of
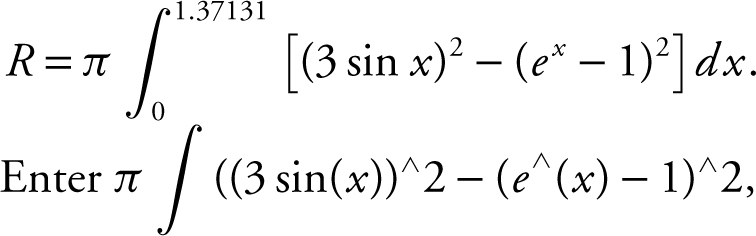
x , 0, 1.37131) and obtain 2.54273π or 7.98824.
The volume of the solid is 7.988.
(c)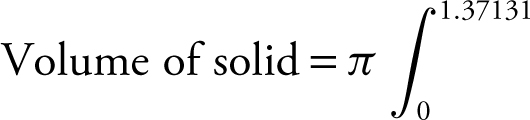 
(Area of Cross Section)dx .
Area of Cross Section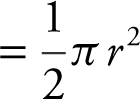 
.
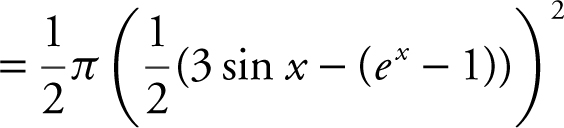Enter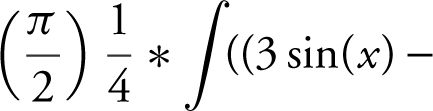 
(e ^(x ) – 1))^2, x , 0, 1.37131) and obtain 0.077184 π or 0.24248. The volume of the solid is approximately 0.077184 π or 0.242.