Basic Math & Pre-Algebra For Dummies, 2nd Edition (2014)

Part III. Parts of the Whole: Fractions, Decimals, and Percents

Chapter 10. Parting Ways: Fractions and the Big Four Operations

IN THIS CHAPTER

Looking at multiplication and division of fractions

Adding and subtracting fractions in a bunch of different ways

Applying the four operations to mixed numbers

In this chapter, the focus is on applying the Big Four operations to fractions. I start by showing you how to multiply and divide fractions, which isn’t much more difficult than multiplying whole numbers. Surprisingly, adding and subtracting fractions is a bit trickier. I show you a variety of methods, each with its own strengths and weaknesses, and I recommend how to choose which method will work best, depending on the problem you have to solve.

Later in the chapter, I move on to mixed numbers. Again, multiplication and division won’t likely pose too much of a problem because the process in each case is almost the same as multiplying and dividing fractions. I save adding and subtracting mixed numbers for the very end. By then, you’ll be much more comfortable with fractions and ready to tackle the challenge.

Multiplying and Dividing Fractions

One of the odd little ironies of life is that multiplying and dividing fractions is easier than adding or subtracting them — just two easy steps and you’re done! For this reason, I show you how to multiply and divide fractions before I show you how to add or subtract them. In fact, you may find multiplying fractions easier than multiplying whole numbers because the numbers you’re working with are usually small. More good news is that dividing fractions is nearly as easy as multiplying them. So I’m not even wishing you good luck — you don’t need it!

Multiplying numerators and denominators straight across

Everything in life should be as simple as multiplying fractions. All you need for multiplying fractions is a pen or pencil, something to write on (preferably not your hand), and a basic knowledge of the multiplication table. (See Chapter 3 for a multiplication refresher.)

Here’s how to multiply two fractions:

1. Multiply the numerators (the numbers on top) to get the numerator of the answer.

2. Multiply the denominators (the numbers on the bottom) to get the denominator of the answer.

For example, here’s how to multiply :

Sometimes when you multiply fractions, you have an opportunity to reduce to lowest terms. (For more on when and how to reduce a fraction, see Chapter 9.) As a rule, math people are crazy about reduced fractions, and teachers sometimes take points off a right answer if you could’ve reduced it but didn’t. Here’s a multiplication problem that ends up with an answer that’s not in its lowest terms:

Because the numerator and the denominator are both even numbers, this fraction can be reduced. Start by dividing both numbers by 2:

Again, the numerator and the denominator are both even, so do it again:

This fraction is now fully reduced.

When multiplying fractions, you can often make your job easier by canceling out equal factors in the numerator and denominator. Canceling out equal factors makes the numbers that you’re multiplying smaller and easier to work with, and it also saves you the trouble of reducing at the end. Here’s how it works:

· When the numerator of one fraction and the denominator of the other are the same, change both of these numbers to 1s. (See the nearby sidebar for why this works.)

· When the numerator of one fraction and the denominator of the other are divisible by the same number, factor this number out of both. In other words, divide the numerator and denominator by that common factor. (For more on how to find factors, see Chapter 8.)

For example, suppose you want to multiply the following two numbers:

You can make this problem easier by canceling out the number 13, as follows:

You can make it even easier by noticing that 20 and 5 are both divisible by 5, so you can also factor out the number 5 before multiplying:

ONE IS THE EASIEST NUMBER

With fractions, the relationship between the numbers, not the actual numbers themselves, is most important. Understanding how to multiply and divide fractions can give you a deeper understanding of why you can increase or decrease the numbers within a fraction without changing the value of the whole fraction.

When you multiply or divide any number by 1, the answer is the same number. This rule also goes for fractions, so

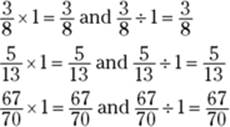
And as I discuss in Chapter 9, when a fraction has the same number in both the numerator and the denominator, its value is 1. In other words, the fractions are all equal to 1. Look what happens when you multiply the fraction :

The net effect is that you’ve increased the terms of the original fraction by 2. But all you’ve done is multiply the fraction by 1, so the value of the fraction hasn’t changed. The fraction is equal to .

Similarly, reducing the fraction by a factor of 3 is the same as dividing that fraction by (which is equal to 1):

So is equal to .

Doing a flip to divide fractions

Dividing fractions is just as easy as multiplying them. In fact, when you divide fractions, you really turn the problem into multiplication.

To divide one fraction by another, multiply the first fraction by the reciprocal of the second. (As I discuss in Chapter 9, the reciprocal of a fraction is simply that fraction turned upside down.)

For example, here’s how you turn fraction division into multiplication:

As you can see, I turn into its reciprocal — — and change the division sign to a multiplication sign. After that, just multiply the fractions as I describe in “Multiplying numerators and denominators straight across”:

As with multiplication, in some cases, you may have to reduce your result at the end. But you can also make your job easier by canceling out equal factors. (See the preceding section.)

All Together Now: Adding Fractions

When you add fractions, one important item to notice is whether their denominators (the numbers on the bottom) are the same. If they’re the same — woo-hoo! Adding fractions that have the same denominator is a walk in the park. But when fractions have different denominators, adding them becomes a tad more complex.

To make matters worse, many teachers make adding fractions even more difficult by requiring you to use a long and complicated method when, in many cases, a short and easy one will do.

In this section, I first show you how to add fractions with the same denominator. Then I show you a foolproof method for adding fractions when the denominators are different. It always works, and it’s usually the simplest way to go. After that, I show you a quick method that you can use only for certain problems. Finally, I show you the longer, more-complicated way to add fractions that usually gets taught.

Finding the sum of fractions with the same denominator

To add two fractions that have the same denominator (bottom number), add the numerators (top numbers) and leave the denominator unchanged.

For example, consider the following problem:

As you can see, to add these two fractions, you add the numerators (1 + 2) and keep the denominator (5).

Why does this work? Chapter 9 tells you that you can think about fractions as pieces of cake. The denominator in this case tells you that the entire cake has been cut into five pieces. So when you add , you’re really adding one piece plus two pieces. The answer, of course, is three pieces — that is, .

Even if you have to add more than two fractions, as long as the denominators are all the same, you just add the numerators and leave the denominator unchanged:

Sometimes when you add fractions with the same denominator, you have to reduce its to the answer lowest terms (to find out more about reducing, flip to Chapter 9). Take this problem, for example:

The numerator and the denominator are both even, so you know they can be reduced:

In other cases, the sum of two proper fractions is an improper fraction. You get a numerator that’s larger than the denominator when the two fractions add up to more than 1, as in this case:

If you have more work to do with this fraction, leave it as an improper fraction so that it’s easier to work with. But if this is your final answer, you may need to turn it into a mixed number (I cover mixed numbers in Chapter 9):

When two fractions have the same numerator, don’t add them by adding the denominators and leaving the numerator unchanged.

Adding fractions with different denominators

When the fractions that you want to add have different denominators, adding them isn’t quite as easy. At the same time, it doesn’t have to be as hard as most teachers make it.

Now, I’m shimmying out onto a brittle limb here, but this needs to be said: Fractions can be added in a very simple way. It always works. It makes adding fractions only a little more difficult than multiplying them. And as you move up the math food chain into algebra, it becomes the most useful method.

So why doesn’t anybody talk about it? I think it’s a clear case of tradition being stronger than common sense. The traditional way to add fractions is more difficult, more time-consuming, and more likely to cause an error. But generation after generation has been taught that it’s the right way to add fractions. It’s a vicious cycle.

But in this book, I’m breaking with tradition. I first show you the easy way to add fractions. Then I show you a quick trick that works in a few special cases. Finally, I show you the traditional way to add fractions.

Using the easy way

At some point in your life, I bet some teacher somewhere told you these golden words of wisdom: “You can’t add two fractions with different denominators.” Your teacher was wrong! Here’s the way to do it:

1. Cross-multiply the two fractions and add the results together to get the numerator of the answer.

Suppose you want to add the fractions . To get the numerator of the answer, cross-multiply. In other words, multiply the numerator of each fraction by the denominator of the other:

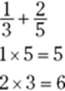
Add the results to get the numerator of the answer:

2. Multiply the two denominators to get the denominator of the answer.

To get the denominator, just multiply the denominators of the two fractions:

The denominator of the answer is 15.

3. Write your answer as a fraction.

As you discover in the earlier section “Finding the sum of fractions with the same denominator,” when you add fractions, you sometimes need to reduce the answer you get. Here’s an example:

Because the numerator and the denominator are both even numbers, you know that the fraction can be reduced. So try dividing both numbers by 2:

This fraction can’t be reduced further, so is the final answer.

As you also discover in “Finding the sum of fractions with the same denominator,” sometimes when you add two proper fractions, your answer is an improper fraction:

If you have more work to do with this fraction, leave it as an improper fraction so that it’s easier to work with. But if this is your final answer, you may need to turn it into a mixed number (see Chapter 9 for details).

In some cases, you have to add more than one fraction. The method is similar, with one small tweak. For example, suppose you want to add :

1. Start by multiplying the numerator of the first fraction by the denominators of all the other fractions.

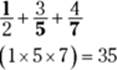
2. Do the same with the second fraction, and add this value to the first.

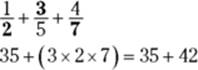
3. Do the same with the remaining fraction(s).

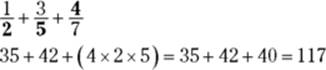
When you’re done, you have the numerator of the answer.

4. To get the denominator, just multiply all the denominators together:

As usual, you may need to reduce or change an improper fraction to a mixed number. In this example, you just need to change to a mixed number (see Chapter 9 for details):

Trying a quick trick

I show you a way to add fractions with different denominators in the preceding section. It always works, and it’s easy. So why do I want to show you another way? It feels like déjà vu.

In some cases, you can save yourself a lot of effort with a little bit of smart thinking. You can’t always use this method, but you can use it when one denominator is a multiple of the other. (For more on multiples, see Chapter 8.) Look at the following problem:

First, I solve it the way I show you in the preceding section:

Those numbers are pretty big, and I’m still not done because the numerator is larger than the denominator. The answer is an improper fraction. Worse yet, the numerator and denominator are both even numbers, so the answer still needs to be reduced.

With certain fraction addition problems, I can give you a smarter way to work. The trick is to turn a problem with different denominators into a much easier problem with the same denominator.

Before you add two fractions with different denominators, check the denominators to see whether one is a multiple of the other (for more on multiples, flip to Chapter 8). If it is, you can use the quick trick:

1. Increase the terms of the fraction with the smaller denominator so that it has the larger denominator.

Look at the earlier problem in this new way:

As you can see, 12 divides into 24 without a remainder. In this case, you want to raise the terms of so that the denominator is 24:

I show you how to do this kind of problem in Chapter 9. To fill in the question mark, the trick is to divide 24 by 12 to find out how the denominators are related; then multiply the result by 11:

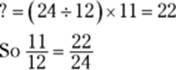
2. Rewrite the problem, substituting this increased version of the fraction, and add as I show you earlier in “Finding the sum of fractions with the same denominator.”

Now you can rewrite the problem this way:

As you can see, the numbers in this case are much smaller and easier to work with. The answer here is an improper fraction; changing it to a mixed number is easy:

Relying on the traditional way

In the two preceding sections, I show you two ways to add fractions with different denominators. They both work great, depending on the circumstances. So why do I want to show you yet a third way? It feels like déjà vu all over again.

The truth is that I don’t want to show you this way. But they’re forcing me to. And you know who they are, don’t you? The man — the system — the powers that be. The ones who want to keep you down in the mud, groveling at their feet. Okay, so I’m exaggerating a little. But let me impress on you that you don’t have to add fractions this way unless you really want to (or unless your teacher insists on it).

Here’s the traditional way to add fractions with two different denominators:

1. Find the least common multiple (LCM) of the two denominators (for more on finding the LCM of two numbers, see Chapter 8).

Suppose you want to add the fractions . First find the LCM of the two denominators, 4 and 10. Here’s how to find the LCM using the multiplication table method:

· Multiples of 10: 10, 20, 30, 40

· Multiples of 4: 4, 8, 12, 16, 20

So the LCM of 4 and 10 is 20.

2. Increase the terms of each fraction so that the denominator of each equals the LCM (for more on how to do this, see Chapter 9).

Increase each fraction to higher terms so that the denominator of each is 20.

3. Substitute these two new fractions for the original ones and add as I show you earlier in “Finding the sum of fractions with the same denominator.”

At this point, you have two fractions that have the same denominator:

When the answer is an improper fraction, you still need to change it to a mixed number:

As another example, suppose you want to add the numbers .

1. Find the LCM of 6, 10, and 15.

This time, I use the prime factorization method (see Chapter 8 for details on how to do this). Start by decomposing the three denominators to their prime factors:

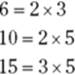
These denominators have a total of three different prime factors — 2, 3, and 5. Each prime factor appears only once in any decomposition, so the LCM of 6, 10, and 15 is

2. You need to increase the terms of all three fractions so that their denominators are 30:

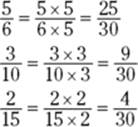
3. Simply add the three new fractions:

Again, you need to change this improper fraction to a mixed number:

Because both numbers are divisible by 2, you can reduce the fraction:

Picking your trick: Choosing the best method

As I say earlier in this chapter, I think the traditional way to add fractions is more difficult than either the easy way or the quick trick. Your teacher may require you to use the traditional way, and after you get the hang of it, you’ll get good at it. But given the choice, here’s my recommendation:

· Use the easy way when the numerators and denominators are small (say, 15 or under).

· Use the quick trick with larger numerators and denominators when one denominator is a multiple of the other.

· Use the traditional way only when you can’t use either of the other methods (or when you know the LCM just by looking at the denominators).

Taking It Away: Subtracting Fractions

Subtracting fractions isn’t really much different than adding them. As with addition, when the denominators are the same, subtraction is easy. And when the denominators are different, the methods I show you for adding fractions can be tweaked for subtracting them.

So to figure out how to subtract fractions, you can read the section “All Together Now: Adding Fractions” and substitute a minus sign (–) for every plus sign (+). But it’d be just a little chintzy if I expected you to do that. So in this section, I show you four ways to subtract fractions that mirror what I discuss earlier in this chapter about adding them.

Subtracting fractions with the same denominator

As with addition, subtracting fractions with the same denominator is always easy. When the denominators are the same, you can just think of the fractions as pieces of cake.

To subtract one fraction from another when they both have the same denominator (bottom number), subtract the numerator (top number) of the second from the numerator of the first and keep the denominator the same. For example:

Sometimes, as when you add fractions, you have to reduce:

Because the numerator and denominator are both even, you can reduce this fraction by a factor of 2:

Unlike addition, when you subtract one proper fraction from another, you never get an improper fraction.

Subtracting fractions with different denominators

Just as with addition, you have a choice of methods when subtracting fractions. These three methods are similar to the methods I show you for adding fractions: the easy way, the quick trick, and the traditional way.

The easy way always works, and I recommend this method for most of your fraction subtracting needs. The quick trick is a great timesaver, so use it when you can. And as for the traditional way — well, even if I don’t like it, your teacher and other math purists probably do.

Knowing the easy way

This way of subtracting fractions works in all cases, and it’s easy. (In the next section, I show you a quick way to subtract fractions when one denominator is a multiple of the other.) Here’s the easy way to subtract fractions that have different denominators:

1. Cross-multiply the two fractions and subtract the second number from the first to get the numerator of the answer.

For example, suppose you want to subtract . To get the numerator, cross-multiply the two fractions and then subtract the second number from the first number (see Chapter 9 for info on cross-multiplication):

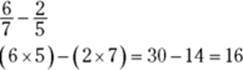
After you cross-multiply, be sure to subtract in the correct order. (The first number is the numerator of the first fraction times the denominator of the second.)

2. Multiply the two denominators to get the denominator of the answer.

3. Putting the numerator over the denominator gives you your answer.

Here’s another example to work with:

This time, I put all the steps together:

With the problem set up like this, you just have to simplify the result:

In this case, you can reduce the fraction:

Cutting it short with a quick trick

The easy way I show you in the preceding section works best when the numerators and denominators are small. When they’re larger, you may be able to take a shortcut.

Before you subtract fractions with different denominators, check the denominators to see whether one is a multiple of the other (for more on multiples, see Chapter 8). If it is, you can use the quick trick:

1. Increase the terms of the fraction with the smaller denominator so that it has the larger denominator.

For example, suppose you want to find . If you cross-multiply these fractions, your results are going to be much bigger than you want to work with. But fortunately, 80 is a multiple of 20, so you can use the quick way.

First, increase the terms of so that the denominator is 80 (for more on increasing the terms of fractions, see Chapter 9):

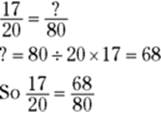
2. Rewrite the problem, substituting this increased version of the fraction, and subtract as I show you earlier in “Subtracting fractions with the same denominator.”

Here’s the problem as a subtraction of fractions with the same denominator, which is much easier to solve:

In this case, you don’t have to reduce to lowest terms, although you may have to in other problems. (See Chapter 9 for more on reducing fractions.)

Keeping your teacher happy with the traditional way

As I describe earlier in this chapter in “All Together Now: Adding Fractions,” you want to use the traditional way only as a last resort. I recommend that you use it only when the numerator and denominator are too large to use the easy way and when you can’t use the quick trick.

To use the traditional way to subtract fractions with two different denominators, follow these steps:

1. Find the least common multiple (LCM) of the two denominators (for more on finding the LCM of two numbers, see Chapter 8).

For example, suppose you want to subtract . Here’s how to find the LCM of 8 and 14:

So the LCM of 8 and 14 is 56.

2. Increase each fraction to higher terms so that the denominator of each equals the LCM (for more on how to do this, see Chapter 9).

The denominators of both now are 56:

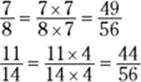
3. Substitute these two new fractions for the original ones and subtract as I show you earlier in “Subtracting fractions with the same denominator.”

This time, you don’t need to reduce because 5 is a prime number and 56 isn’t divisible by 5. In some cases, however, you have to reduce the answer to lowest terms.

Working Properly with Mixed Numbers

All the methods I describe earlier in this chapter work for both proper and improper fractions. Unfortunately, mixed numbers are ornery little critters, and you need to figure out how to deal with them on their own terms. (For more on mixed numbers, flip to Chapter 9.)

Multiplying and dividing mixed numbers

I can’t give you a direct method for multiplying and dividing mixed numbers. The only way is to convert the mixed numbers to improper fractions and multiply or divide as usual. Here’s how to multiply or divide mixed numbers:

1. Convert all mixed numbers to improper fractions (see Chapter 9 for details).

For example, suppose you want to multiply . First convert and to improper fractions:

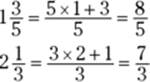
2. Multiply these improper fractions (as I show you earlier in this chapter, in “Multiplying and Dividing Fractions”).

3. If the answer is an improper fraction, convert it back to a mixed number (see Chapter 9).

In this case, the answer is already in lowest terms, so you don’t have to reduce it.

As a second example, suppose you want to divide .

1. Convert and to improper fractions:

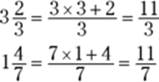
2. Divide these improper fractions.

Divide fractions by multiplying the first fraction by the reciprocal of the second (see the earlier “Multiplying and Dividing Fractions” section):

In this case, before you multiply, you can cancel out factors of 11 in the numerator and denominator:

3. Convert the answer to a mixed number.

Adding and subtracting mixed numbers

One way to add and subtract mixed numbers is to convert them to improper fractions, much as I describe earlier in this chapter in “Multiplying and dividing mixed numbers,” and then to add or subtract them using a method from the “All Together Now: Adding Fractions” or “Take It Away: Subtracting Fractions” sections. Doing so is a perfectly valid way of getting the right answer without learning a new method.

Unfortunately, teachers just love to make people add and subtract mixed numbers in their own special way. The good news is that a lot of folks find this way easier than all the converting stuff.

Working in pairs: Adding two mixed numbers

Adding mixed numbers looks a lot like adding whole numbers: You stack them one on top of the other, draw a line, and add. For this reason, some students feel more comfortable adding mixed numbers than adding fractions. Here’s how to add two mixed numbers:

1. Add the fractional parts using any method you like; if necessary, change this sum to a mixed number and reduce it.

2. If the answer you found in Step 1 is an improper fraction, change it to a mixed number, write down the fractional part, and carry the whole number part to the whole number column.

3. Add the whole number parts (including any number carried).

You may also need to reduce your answer to lowest terms (see Chapter 9). In the examples that follow, I show you everything you need to know.

SUMMING UP MIXED NUMBERS WHEN THE DENOMINATORS ARE THE SAME

As with any problem involving fractions, adding is always easier when the denominators are the same. For example, suppose you want to add . Doing mixed number problems is often easier if you place one number above the other:

As you can see, this arrangement is similar to how you add whole numbers, but it includes an extra column for fractions. Here’s how you add these two mixed numbers step by step:

1. Add the fractions.

2. Switch improper fractions to mixed numbers; write down your answer.

Because is a proper fraction, you don’t have to change it.

3. Add the whole number parts.

Here’s how your problem looks in column form:

This problem is about as simple as they get. In this case, all three steps are pretty easy. But sometimes, Step 2 requires more attention. For example, suppose you want to add . Here’s how you do it:

1. Add the fractions.

2. Switch improper fractions to mixed numbers, write down the fractional part, and carry over the whole number.

Because the sum is an improper fraction, convert it to the mixed number (flip to Chapter 9 for more on converting improper fractions to mixed numbers). Write down and carry the 1 over to the whole number column.

3. Add the whole number parts, including any whole numbers you carried over when you switched to a mixed number.

Here’s how the solved problem looks in column form. (Be sure to line up the whole numbers in one column and the fractions in another.)

As with any other problems involving fractions, sometimes you need to reduce at the end of Step 1.

The same basic idea works no matter how many mixed numbers you want to add. For example, suppose you want to add :

1. Add the fractions.

2. Switch improper fractions to mixed numbers, write down the fractional part, and carry over the whole number.

Because the result is an improper fraction, convert it to the mixed number and then reduce it to (for more on converting and reducing fractions, see Chapter 9). I recommend doing these calculations on a piece of scrap paper.

Write down and carry the 2 to the whole number column.

3. Add the whole numbers.

Here’s how the problem looks after you solve it:

SUMMING UP MIXED NUMBERS WHEN THE DENOMINATORS ARE DIFFERENT

The most difficult type of mixed number addition is when the denominators of the fractions are different. This difference doesn’t change Steps 2 or 3, but it does make Step 1 tougher.

For example, suppose you want to add .

1. Add the fractions.

Add . You can use any method from earlier in this chapter. Here, I use the easy way:

2. Switch improper fractions to mixed numbers, write down the fractional part, and carry over the whole number.

This fraction is improper, so change it to the mixed number .

Fortunately, the fractional part of this mixed number isn’t reducible.

Write down the and carry over the 1 to the whole number column.

3. Add the whole numbers.

Here’s how the completed problem looks:

Subtracting mixed numbers

The basic way to subtract mixed numbers is close to the way you add them. Again, the subtraction looks more like what you’re used to with whole numbers. Here’s how to subtract two mixed numbers:

1. Find the difference of the fractional parts using any method you like.

2. Find the difference of the two whole number parts.

Along the way, though, you may encounter a couple more twists and turns. I keep you on track so that, by the end of this section, you can do any mixed-number subtraction problem.

TAKING AWAY MIXED NUMBERS WHEN THE DENOMINATORS ARE THE SAME

As with addition, subtraction is much easier when the denominators are the same. For example, suppose you want to subtract . Here’s what the problem looks like in column form:

In this problem, I subtract . Then I subtract 7 – 3 = 4. Not too terrible, agreed?

One complication arises when you try to subtract a larger fractional part from a smaller one. Suppose you want to find . This time, if you try to subtract the fractions, you get

Obviously, you don’t want to end up with a negative number in your answer. You can handle this problem by borrowing from the column to the left. This idea is similar to the borrowing that you use in regular subtraction, with one key difference.

When borrowing in mixed-number subtraction,

1. Borrow 1 from the whole-number portion and add it to the fractional portion, turning the fraction into a mixed number.

To find , borrow 1 from the 11 and add it to , making it the mixed number :

2. Change this new mixed number into an improper fraction.

Here’s what you get when you change into an improper fraction:

The result is . This answer is a weird cross between a mixed number and an improper fraction, but it’s what you need to handle the job.

3. Use the result in your subtraction.

In this case, you have to reduce the fractional part of the answer:

SUBTRACTING MIXED NUMBERS WHEN THE DENOMINATORS ARE DIFFERENT

Subtracting mixed numbers when the denominators are different is just about the hairiest thing you’re ever going to have to do in pre-algebra. Fortunately, though, if you work through this chapter, you acquire all the skills you need.

Suppose you want to subtract . Because the denominators are different, subtracting the fractions becomes more difficult. But you have another question to think about: In this problem, do you need to borrow? If is greater than , you don’t have to borrow. But if is less than , you do. (For more on borrowing in mixed-number subtraction, see the preceding section.) In Chapter 9, I show you how to test two fractions to see which is greater by cross-multiplying:

Because 28 is less than 33, is less than , so you do have to borrow. I get the borrowing out of the way first:

Now the problem looks like this:

The first step, subtracting the fractions, is the most time-consuming, so as I show you earlier in “Subtracting fractions with different denominators,” you can take care of that on the side:

The good news is that this fraction can’t be reduced (72 and 77 have no common factors: and ). So the hard part of the problem is done, and the rest follows easily:

This problem is about as difficult as a mixed-number subtraction problem gets. Take a look at it step by step. Better yet, copy the problem and then close the book and try to work through the steps on your own. If you get stuck, that’s okay: Better now than on an exam!