Calculus For Dummies, 2nd Edition (2014)

Part II. Warming Up with Calculus Prerequisites

Chapter 6. The Trig Tango

IN THIS CHAPTER

Socking it to ’em with SohCahToa

Everybody’s got an angle: 30°, 45°, 60°

Circumnavigating the unit circle

Graphing trig functions

Investigating inverse trig functions

Many calculus problems involve trigonometry, and the calculus itself is enough of a challenge without having to relearn trig at the same time. So, if your trig is rusty — I’m shocked — review these trig basics, or else!

Studying Trig at Camp SohCahToa

The study of trig begins with the right triangle. The three main trig functions (sine, cosine, and tangent) and their reciprocals (cosecant, secant, and cotangent) all tell you something about the lengths of the sides of a right triangle that contains a given acute angle — like angle x in Figure 6-1. The longest side of this right triangle (or any right triangle), the diagonal side, is called the hypotenuse. The side that’s 3 units long in this right triangle is referred to as the opposite side because it’s on the opposite side of the triangle from angle x, and the side of length 4 is called the adjacent side because it’s adjacent to, or touching, angle x.

FIGURE 6-1: Sitting around the campfire, studying a right triangle.

SohCahToa is a meaningless mnemonic device that helps you remember the definitions of the sine, cosine, and tangent functions. SohCahToa uses the initial letters of sine, cosine, and tangent, and the initial letters of hypotenuse, opposite, and adjacent to help you remember the following definitions. (To remember how to spell SohCahToa, note its pronunciation and the fact that it contains three groups of three letters each.) For any angle ,

For the triangle in Figure 6-1,

The other three trig functions are reciprocals of these: Cosecant (csc) is the reciprocal of sine, secant (sec) is the reciprocal of cosine, and cotangent (cot) is the reciprocal of tangent.

So for the triangle in Figure 6-1,

Two Special Right Triangles

Because so many garden variety calculus problems involve 30°, 45°, and 60° angles, it’s a good idea to memorize the two right triangles in Figure 6-2.

FIGURE 6-2: The 45°-45°-90° triangle and the 30°-60°-90° triangle.

The 45°-45°-90° triangle

Every 45°-45°-90° triangle is the shape of a square cut in half along its diagonal. The 45°-45°-90° triangle in Figure 6-2 is half of a 1-by-1 square. The Pythagorean theorem gives you the length of its hypotenuse, , or about 1.41.

The Pythagorean theorem: For any right triangle, , where a and b are the lengths of the triangle’s legs (the sides touching the right angle) and c is the length of its hypotenuse.

When you apply the SohCahToa trig functions and their reciprocals to the 45° angle in the 45°-45°-90° triangle, you get the following trig values:

The 30°-60°-90° triangle

Every 30°-60°-90° triangle is half of an equilateral triangle cut straight down the middle along its altitude.

The 30°-60°-90° in Figure 6-2 is half of a 2-by-2-by-2 equilateral triangle. It has legs of lengths 1 and (about 1.73), and a 2-unit long hypotenuse.

Don’t make the common error of switching the 2 with the in a 30°-60°-90° triangle. Remember that 2 is more than ( equals 2, so be must be less than 2) and that the hypotenuse is always the longest side of a right triangle.

When you sketch a 30°-60°-90° triangle, exaggerate the fact that it’s wider than it is tall (or taller than wide if you tip it up). This makes it obvious that the shortest side (length of 1) is opposite the smallest angle (30°).

Here are the trig values for the 30° angle in the 30°-60°-90° triangle:

The 30°-60°-90° triangle kills two birds with one stone because it also gives you the trig values for a 60° angle. Look at Figure 6-2 again. For the 60° angle, the side of the triangle is now the opposite side for purposes of SohCahToa because it’s on the opposite side of the triangle from the 60° angle. The 1-unit side becomes the adjacent side for the 60° angle, and the 2-unit side is still, of course, the hypotenuse. Now use SohCahToa again to find the trig values for the 60° angle:

The mnemonic device SohCahToa, along with the two oh-so-easy-to-remember right triangles in Figure 6-2, gives you the answers to 18 trig problems!

Circling the Enemy with the Unit Circle

SohCahToa only works with right triangles, and so it can only handle acute angles — angles less than 90°. (The angles in a triangle must add up to 180°; because a right triangle has a 90° angle, the other two angles must each be less than 90°.) With the unit circle, however, you can find trig values for any size angle. The unit circle has a radius of one unit and is set in an x-y coordinate system with its center at the origin. See Figure 6-3.

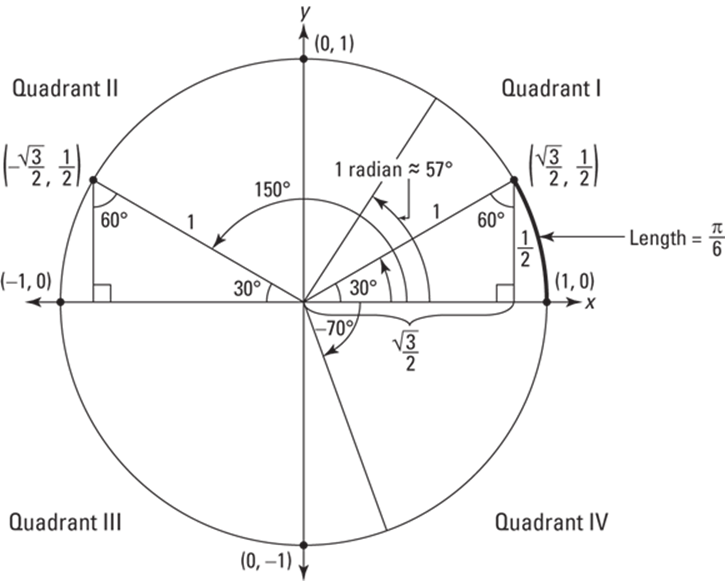
FIGURE 6-3: The so-called unit circle.

Figure 6-3 has quite a lot of information, but don’t panic; it will all make perfect sense in a minute.

Angles in the unit circle

Measuring angles: To measure an angle in the unit circle, start at the positive x-axis and go counterclockwise to the terminal side of the angle.

For example, the 150° angle in Figure 6-3 begins at the positive x-axis and ends at the segment that hits the unit circle at . If you go clockwise instead, you get an angle with a negative measure (like the angle in the figure).

Measuring angles with radians

You know all about degrees. You know what 45° and 90° angles look like; you know that about face means a turn of 180° and that turning all the way around till you’re back to where you started is a 360° turn.

But degrees aren’t the only way to measure angles. You can also use radians. Degrees and radians are just two different ways to measure angles, like inches and centimeters are two ways to measure length.

Definition of radian: The radian measure of an angle is the length of the arc along the circumference of the unit circle cut off by the angle.

Look at the 30° angle in quadrant I of Figure 6-3. Do you see the bolded section of the circle’s circumference that is cut off by that angle? Because a whole circle is 360°, that 30° angle is one-twelfth of the circle. So the length of the bold arc is one-twelfth of the circle’s circumference. Circumference is given by the formula . This circle has a radius of 1, so its circumference equals . Because the bold arc is one-twelfth of that, its length is , which is the radian measure of the 30° angle.

equals radians. The unit circle’s circumference of makes it easy to remember that 360° equals radians. Half the circumference has a length of , so 180° equals radians.

If you focus on the fact that 180° equals radians, other angles are easy:

· 90° is half of 180°, so 90° equals half of , or radians.

· 60° is a third of 180°, so 60° equals a third of , or radians.

· 45° is a fourth of 180°, so 45° equals a fourth of , or radians.

· 30° is a sixth of 180°, so 30° equals a sixth of , or radians.

Formulas for converting from degrees to radians and vice versa:

· To convert from degrees to radians, multiply the angle’s measure by .

· To convert from radians to degrees, multiply the angle’s measure by .

By the way, the word radian comes from radius. Look at Figure 6-3 again. An angle measuring 1 radian (about 57°) cuts off an arc along the circumference of this circle of the same length as the circle’s radius. This is true not only of unit circles, but of circles of any size. In other words, take the radius of any circle, lay it along the circle’s circumference, and that arc creates an angle of 1 radian.

Radians are preferred over degrees. In this or any other calculus book, some problems use degrees and others use radians, but radians are the preferred unit. If a problem doesn’t specify the unit, do the problem in radians.

Honey, I shrunk the hypotenuse

Look at the unit circle in Figure 6-3 again. See the 30°-60°-90° triangle in quadrant I? It’s the same shape but half the size of the one in Figure 6-2. Each of its sides is half as long. Because its hypotenuse now has a length of 1, and because when H is 1, equals O, the sine of the 30° angle, which equals , ends up equaling the length of the opposite side. The opposite side is , so that’s the sine of 30°. Note that the length of the opposite side is the same as the y-coordinate of the point . If you figure the cosine of 30° in this triangle, it ends up equaling the length of the adjacent side, which is the same as the x-coordinate of . Notice that these values for sin 30° and cos 30° are the same as the ones given by the 30°-60°-90° triangle in Figure 6-2. This shows you, by the way, that shrinking a right triangle down (or blowing it up) has no effect on the trigonometric values for the angles in the triangle.

Now look at the 30°-60°-90° triangle in quadrant II in Figure 6-3. Because it’s the same size as the 30°-60°-90° triangle in quadrant I, which hits the circle at , the triangle in quadrant II hits the circle at a point that’s straight across from and symmetric to . The coordinates of the point in quadrant II are . But remember that angles on the unit circle are all measured from the positive x-axis, so the hypotenuse of this triangle indicates a 150° angle; and that’s the angle, not 30°, associated with the point . The cosine of 150° is given by the x-coordinate of this point, , and the sine of 150° equals the y-coordinate, .

Coordinates on the unit circle tell you an angle’s cosine and sine. The terminal side of an angle in the unit circle hits the circle at a point whose x-coordinate is the angle’s cosine and whose y-coordinate is the angle’s sine. Here’s a mnemonic: x and y are in alphabetical order as are cosine and sine.

Putting it all together

Look at Figure 6-4. Now that you know all about the 45°-45°-90° triangle, you can easily work out — or take my word for it — that a 45°-45°-90° triangle in quadrant I hits the unit circle at . And if you take the 30°-60°-90° triangle in quadrant I that hits the unit circle at and flip it on its side, you get another 30°-60°-90° triangle with a 60° angle that hits the circle at . As you can see, this point has the same coordinates as those for the 30° angle but reversed.

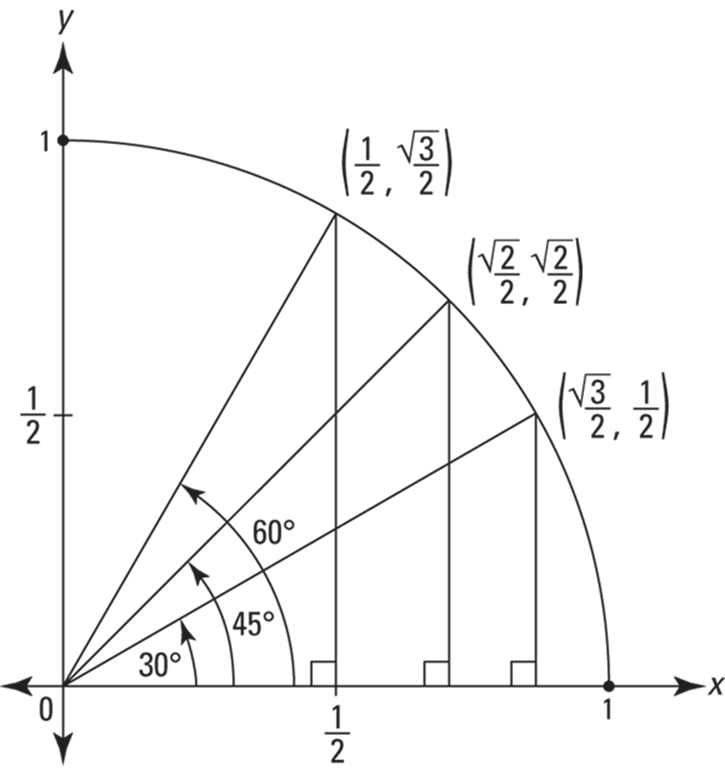
FIGURE 6-4: Quadrant I of the unit circle with three angles and their coordinates.

How to draw a right triangle in the unit circle: Whenever you draw a right triangle in the unit circle, put the acute angle you care about at the origin — that’s — and then put the right angle on the x-axis — never on the y-axis.

is greater than . To keep from mixing up the numbers and when dealing with a 30° or a 60° angle, note that because is more than 1, must be greater than . Thus, because a 30° angle hits the circle further out to the right than up, the x-coordinate must be greater than the y-coordinate. So, the point must be , not the other way around. It’s vice versa for a 60° angle.

Now for the whole enchilada. Because of the symmetry in the four quadrants, the three points in quadrant I in Figure 6-4 have counterparts in the other three quadrants, giving you 12 known points. Add to these the four points on the axes, , , , and , and you have 16 total points, each with an associated angle, as shown in Figure 6-5.

FIGURE 6-5: The unit circle with 16 angles and their coordinates.

These 16 pairs of coordinates automatically give you the cosine and sine of the 16 angles. And because , you can obtain the tangent of these 16 angles by dividing an angle’s y-coordinate by its x-coordinate. (Note that when the cosine of an angle equals zero, the tangent will be undefined because you can’t divide by zero.) Finally, you can find the cosecant, secant, and cotangent of the 16 angles because these trig functions are just the reciprocals of sine, cosine, and tangent. (Same caution: whenever sine, cosine, or tangent equals zero, the reciprocal function will be undefined.) You’ve now got, at your fingertips — okay, maybe that’s a bit of a stretch — the answers to 96 trig questions.

Learn the unit circle. Knowing the trig values from the unit circle is quite useful in calculus. So quiz yourself. Start by memorizing the 45°-45°-90° and the 30°-60°-90° triangles. Then picture how these triangles fit into the four quadrants of the unit circle. Use the symmetry of the quadrants as an aid. With some practice, you can get pretty quick at figuring out the values for the six trig functions of all 16 angles. (Try to do this without looking at something like Figure 6-5.) And quiz yourself with radians as well as with degrees. That would bring your total to 192 trig facts! Quick — what’s the secant of 210°, and what’s the cosine of ? Here are the answers (no peeking): .

All Students Take Calculus. Here’s a final tip to help you with the unit circle and the values of all the trig functions. Take any old unit circle (like the one in Figure 6-5) and write the initial letters of All Students Take Calculus in the four quadrants: Put an A in quadrant I, an S in quadrant II, a T in quadrant III, and a C in quadrant IV. These letters now tell you whether the various trig functions have positive or negative values in the different quadrants. The A in quadrant I tells you that All six trig functions have positive values in quadrant I. The S in quadrant II tells you that Sine (and its reciprocal, cosecant) are positive in quadrant II and that all other trig functions are negative there. The T in quadrant III tells you that Tangent (and its reciprocal, cotangent) are positive in quadrant III and that the other functions are negative there. Finally, the C in quadrant IV tells you that Cosine (and its reciprocal, secant) are positive there and that the other functions are negative. That’s a wrap.

Graphing Sine, Cosine, and Tangent

Figure 6-6 shows the graphs of sine, cosine, and tangent, which you can, of course, produce on a graphing calculator.

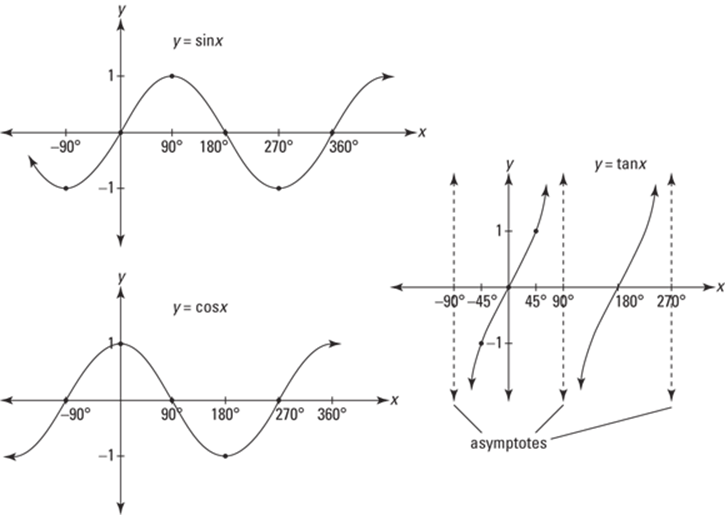
FIGURE 6-6: The graphs of the sine, cosine, and tangent functions.

Definitions of periodic and period: Sine, cosine, and tangent — and their reciprocals, cosecant, secant, and cotangent — are periodic functions, which means that their graphs contain a basic shape that repeats over and over indefinitely to the left and the right. The period of such a function is the length of one of its cycles.

If you know the unit circle, you can easily reproduce these three graphs by hand. First, note that the sine and cosine graphs are the same shape — cosine is the same as sine, just slid 90° to the left. Also, notice that their simple wave shape goes as high as 1 and as low as and goes on forever to the left and right, with the same shape repeating every 360°. That’s the period of both functions, 360°. (It’s no coincidence, by the way, that 360° is also once around the unit circle.) The unit circle tells you that , , , , and that . If you start with these five points, you can sketch one cycle. The cycle then repeats to the left and right. You can use the unit circle in the same manner to sketch the cosine function.

Notice in Figure 6-6 that the period of the tangent function is 180°. If you remember that and the basic pattern of repeating backward S-shapes, sketching it isn’t difficult. Because , you can use the unit circle to determine that , , and . That gives you the points , , and . Since and are both undefined (because at these points gives you a zero in the denominator), you draw vertical asymptotes at and 90°.

Definition of vertical asymptote: A vertical asymptote is an imaginary line that a curve gets closer and closer to (but never touches) as the curve goes up toward infinity or down toward negative infinity. (In Chapters 7and 8, you see more vertical asymptotes and also some horizontal asymptotes.)

The two asymptotes at and 90° and the three points at , , and show you where to sketch one backward S. The S-shapes then repeat every 180° to the left and the right.

Inverse Trig Functions

An inverse trig function, like any inverse function, reverses what the original function does. For example, , so the inverse sine function — written as — reverses the input and output. Thus, . It works the same for the other trig functions.

The negative 1 superscript in the sine inverse function is not a negative 1 power, despite the fact that it looks just like it. Raising something to the negative 1 power gives you its reciprocal, so you might think that is the reciprocal of but the reciprocal of sine is cosecant, not sine inverse. Pretty weird that the same symbol is used to mean two different things. Go figure.

The only trick with inverse trig functions is memorizing their ranges — that’s the interval of their outputs. Consider sine inverse, for example. Because both and , you wouldn’t know whether equals 30° or 150° unless you know how the interval of sine inverse outputs is defined. And remember, in order for something to be a function, there can’t be any mystery about the output for a given input. If you reflect the sine function over the line to create its inverse, you get a vertical wave that isn’t a function because it doesn’t pass the vertical line test. (See the definition of the vertical line test in Chapter 5.) To make sine inverse a function, you have to take a small piece of the vertical wave that does pass the vertical line test. The same thing goes for the other inverse trig functions. Here are their ranges:

· The range of is , or .

· The range of is , or .

· The range of is , or .

· The range of is , or .

Note the pattern: The range of is the same as , and the range of is the same as .

Believe it or not, calculus authors don’t agree on the ranges for the secant inverse and cosecant inverse functions. You’d think they could agree on this like they do with just about everything else in mathematics. Humph. Use the ranges given in your particular textbook. If you don’t have a textbook, use the range for its cousin , and use the range for . (By the way, I don’t refer to as the reciprocal of because it’s not its reciprocal — even though csc x is the reciprocal of sin x. Ditto for and .)

Identifying with Trig Identities

Remember trig identities like and ? Tell the truth now — most people remember trig identities about as well as they remember nineteenth century vice-presidents. They come in handy in calculus though, so a list of other useful ones is in the online Cheat Sheet. Go to www.dummies.com and search for “Calculus For Dummies Cheat Sheet” in the Search box.