The Calculus Primer (2011)
Part III. Differentiation of Algebraic Functions
Chapter 9. DERIVATIVE OF THE POWER FUNCTION
3—5. The Derivative of a Variable with a Constant Exponent. Consider the power function y = vn. Applying the General Rule, and expanding by the Binomial Theorem, we obtain:
Step 1.y + Δy = (v + Δv)n.
Step 2.Δy = (v + Δv)n − vn.
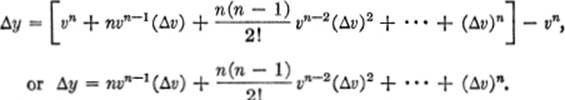
Step 3.
Now, as Δx → 0, Δv → 0; hence
Step 4.
RULE. The derivative of a variable with a constant exponent is equal to the product of that exponent, the variable raised to a power one less than the original exponent, and the derivative of the variable.
NOTE 1.It is assumed, in the above proof, that the exponent n is a positive integer. If this were not so, we could not have applied the binomial theorem, for when n is negative or fractional, the number of terms in the binomial expansion is infinite; we could not pass to the limit, therefore, since Principle (III) of §1—15 applies only to a finite number of terms. However, we shall find later that the relation
holds for any rational value of n, whether positive, negative, or fractional.
NOTE 2.As a special case of formula [5] above, we let v = x, and obtain:
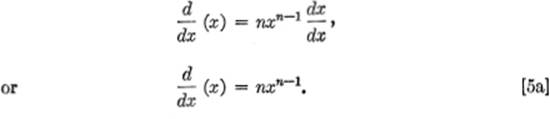
NOTE 3.Formulas [5] and [5a], in view of what was said in NOTE 1, may be applied to a power function when the exponent is negative or fractional, as shown in Examples 3 and 4 below; thus
EXAMPLE 1.Differentiate y = x3 + 5x2 + 4.
Solution.
= 3x2 + 10x.
EXAMPLE 2.Differentiate y = 5x4 + 6x3 − 3x2 + 9x − 21.
Solution. = 20x3 + 18x2 − 6x + 9.
EXAMPLE 3.Differentiate y = 
Solution.y = x−3 + 3x−2 − 5x−1.
= −3(x−4) + (3)(−2)(x−3) − 5(−1)(x−2),
or
EXAMPLE 4.Differentiate 
Solution. y = 2x½ + (3x)½ − 4(x−⅓).
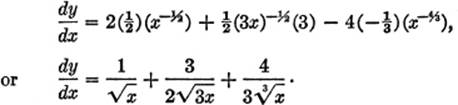
EXAMPLE 5.Find :y = (5x + 3)2.
Solution.v = 5x + 3, and y = v2.
Hence = nvn−1 = 2v(5) = 10(5x + 3).
This result may be verified by expanding the given function before differentiating; thus
y = (5x + 3)2 = 25x2 + 30x + 9;
= 50x + 30 = 10(5x + 3).
EXAMPLE 6.Differentiate with respect to x: y = 4 (x2 + 6x)3.
Solution.
Here v = x2 + 6x, and y = 4v3.
Hence = nvn−1 
= (4)(3)v2
= (4)(3)(x2 + 6x)2(2x + 6)
= 12(x2 + 6x)2(2x + 6).
EXAMPLE 7.Differentiate y = 
Solution.y = 
(x2 − 4)½;v = x2 − 4
EXERCISE 3—1
Differentiate:
1.y = 2x3 − 5x + 8
2.y = x5 − 3x4 + 2x3 + x
3.y = 
4.y = 5
+ 6
5.y = v1t + 
gt2
6.y = x−3 − 3x−2 + 4x−1
7.y = 
8.s = 
9.y = (x2 − 5x)2
10.s = k(a + 2t)3
11.y = xm − mx3
12.y = 
13.y = (x3 − 2x)4
14.y = 
15.y = 
16.y = 