The Calculus Primer (2011)

Part VI. Further Applications of the Derivative

Chapter 24. RELATED TIME RATES

6—12.The Derivative as the Ratio of Two Rates. Consider the path generated by a moving point whose motion follows the law y = f(x). The coordinates of point P in any position may be regarded as functions of the time, as indicated in §6—8. Differentiating with respect to t:

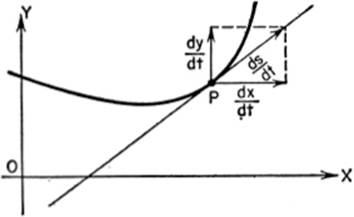
Equation [1] may be stated in words as follows:

At any instant, the time rate of change of a function (y) equals the derivative of the function multiplied by the time rate of change of the independent variable.

This equation [1] may also be written as:

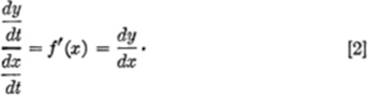
In words, equation [2] states:

The derivative is a measure of the time rate of change of y to the time rate of change of x.

The reader should note carefully that here we are regarding the derivative as the ratio of two separate rates.

Since represents the time rate of change of length of arc, we have, from §6—8:

6—13.Related Time Rates. The principle represented by equations [1] and [2] finds ready application to many problems involving related time rates. Thus, suppose that two or more quantities, each of which varies with the time, are also functionally related to one another, i.e., connected by an equation; then the relation between their time rates of change may be determined by writing the functional relation between the variables, and differentiating with respect to the time. We illustrate the procedure to be followed.

EXAMPLE 1.A ladder 18 ft. long leans against the side of a building with one end resting on the horizontal ground. The foot of the ladder is drawn away from the building at the rate of 3 ft. per sec. (a) Find the rate at which the top of the ladder is descending when the foot is 4 ft. from the building. (b) Find how far the foot of the ladder will be from the building when the top is descending at the rate of 4 ft./sec.

Solution.

x² + y² = 18².(1)

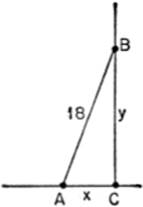
(a)To find the rate at which the top is descending when x = 4 ft.:

When x = 4,

substituting in (2), when = 3 ft./sec and y = 2:

(2)(4)(3) + 2(2) = 0,

= .68 ft./sec.

(b)To find the distance (x) of A from C when B is descending at the rate of 4 ft./sec, substitute in equation (2):

(2)(x)(3) + 2(y)(4) = 0,

or3x + 4y = 0.(3)

Solving (1) and (3) simultaneously, x = = 14.4 ft.

EXAMPLE 2.If a point moves on the parabola 8x = y² so that when y = 6, the ordinate is increasing at the rate of 2 ft./sec: (a) How fast is the abscissa moving at that instant? (b) At what point is the ordinate increasing 4 times as fast as the abscissa?

Solution.

(a)8x = y².(1)

Substituting in (2):

(b) = 4;

hence, from (2):8 = 2y(4),
y = 1,

and, from (1), ify = 1, x = .

Therefore, at (, 1) the ordinate is increasing 4 times as fast as the abscissa.

EXAMPLE 3.A boy standing on a dock 8 ft. above the level of a lake is hauling in a canoe by means of a rope. If the rope is being pulled in at the rate of 2 ft./sec., how fast is the canoe moving when 17 ft. of tow rope are still paid out?

Solution.

x² + 8² = 17²;x = = 15 ft.

y² – x² = 8²;2y – 2x = 0.

Hence when y = 17, x = 15: = 2;

therefore2(17)(2) – 2(15) = 0,

or

EXAMPLE 4.A man 5 ft. tall walks towards a street lamp at the rate of 4 ft./sec. The lamp is 18 ft. high. (a) How fast is his shadow growing shorter? (b) How fast is the end of his shadow moving?

Solution.

Let BC represent the street lamp, DE the man, and let x = AE be the length of his shadow.

Hence, by similar triangles:

(a)By hypothesis, = − 4 ft./sec., since y decreases as t increases.
Differentiating (1):

(b)To find how fast point A is moving, we must determine the rate of change of the distance CA = y + x, which is given by + . From(a):

the rate at which the tip of the shadow (point A) is moving.

EXERCISE 6—6

1. A man is walking at the rate of 3 miles an hour toward the base of a tower 50 feet high. At what rate is he approaching the top of the tower when he is 120 ft. from the base of the tower?

2. The altitude of a cone and the diameter of its base are constantly equal. (a) If the volume of the cone is increasing at the rate of 8 cu. ft./min., how fast is the radius changing when the radius equals foot? (b) How fast is the lateral surface changing when the radius is increasing at l ft./min. and h = 6 ft.?

3. In the parabola y² = 16x, x increases uniformly at the rate of 4 in. per second. (a) At what rate is y increasing when x = 9 inches? (b) At what point on the parabola do x and y increase equally fast?

4. Two trains leave the same terminal at the same time, one traveling 60 mi./hr. southward, the other 80 mi./hr. westward. How fast are they separating after 3 hours?

5. In an expanding sphere, at any instant when the radius equals r, the rate of increase in volume is how many times as great as the rate of increase in area?

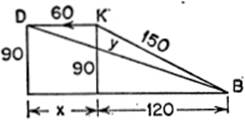
6. A kite is 90 ft. high, and 150 ft. of string are paid out. The kite drifts horizontally directly away from the boy flying the kite at the rate of 20 ft. per minute. How fast is the string being paid out at the instant the kite has drifted 60 ft. from K to D?

7. A plane flies horizontally at the rate of 240 mi. per hour and passes directly over a beacon light below at an elevation of 2 miles. How fast is its distance from the beacon increasing half a minute later?

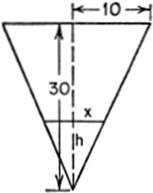
8. A solution is being poured into a conical vessel at the rate of 40 cc. per minute. The vessel is 20 cm. across in diameter, and 30 cm. deep. Find the rate at which the surface of the liquid is rising at the instant the level is 6 cm. above the apex of the cone.

9. In the accompanying figure, where y = sin ø and ø = s, determine the value of ø, in the first quadrant, when the arc s is increasing twice as fast as the sin ø.

10. In each of the following, find the rate at which the length of arc is increasing, at the ordinate (or abscissa) given, when one of the variables is changing at the given rate:

(a)y² = 4x; x = 4; = 8.

(b)xy = 24; y = 3; = 6.

(c)y² = 2x³; x = 2; = 8.

EXERCISE 6—7

Review

1. If the side of an equilateral triangle is increasing uniformly at the rate of 6 cm. per second, at what rate is its altitude increasing?

2. If the side of an equilateral triangle is increasing uniformly at the rate of 10 inches per second, at what rate is the area increasing when the side is 4 feet?

3. In the parabola y² = 24x, find: (a) the point at which the ordinate and abscissa are increasing equally rapidly; (b) the point at which the ordinate is increasing half as fast as the abscissa.

4. Determine the points of inflection of

5. Find the maximum rectangle that can be inscribed in the ellipse whose semiaxes are a and b.

6. A cylindrical tin can of given volume is to be made with the least amount of metal. What must be its relative dimensions?

7. The slant height of a right circular cone is S. What must be its altitude if the cone is to have a maximum volume?

8. Find the right circular cylinder of greatest convex surface that can be cut from a sphere of radius R.

9. Find the altitude of the right circular cone of greatest volume that can be cut from a sphere of radius R.

10. A spherical rubber balloon is being deflated at the rate of 20 cu. in. per second. How fast is the surface of the balloon decreasing when the radius is 4 inches?