The Calculus Primer (2011)
Part XI. Expansion of Functions
Chapter 40. TESTS FOR CONVERGENCE AND DIVERGENCE
11—5. Fundamental Conditions for Convergence. It can be shown that a series
u1 + u2 + u3 + … + un + …
cannot converge unless
This is only a necessary, but not a sufficient condition for convergence. Thus, some series for which 
un = 0 are divergent. Hence this principle is useful only in establishing the fact that a given series is not convergent. We may say, therefore, without exception, that in a series u1 + u2 + u3 + … + un + … , if 
un is a number different from zero, then the series is divergent [2]
EXAMPLES. Each of the following series is divergent, since in each case the un ≠ 0:
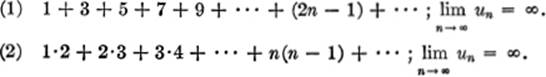
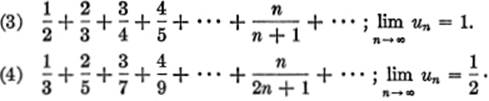
11—6. Testing for Convergence and Divergence. The reader should realize that the problem of determining whether a given series converges or not is a very different problem from finding the exact value to which it converges. In fact, the problem of summation, or finding the value of Sn, is, in general, complex and difficult, and must be left to more advanced treatises. There are, however, a number of tests available for finding out whether a series is convergent or divergent. Some of the most common of these tests will now be discussed.
11—7. Comparison Tests for Convergence and Divergence. This test is based on the following principle, which is here stated without proof.
RULE I. A series of positive terms converges if each term is less than, or at most, equal to, the corresponding term of another series of positive terms that is known to be convergent.
RULE II. A series of positive terms diverges if each term is equal to or greater than the corresponding term of another series of positive terms that is known to be divergent.
The known series, with which the series to be tested is compared, is called the comparison series. For purposes of such comparisons, we often use (1) the geometric series; (2) the so-called p-series; (3) the telescopic series; and (4) the arithmetic series.
(1)The Geometric Series:
a + ar + ar2 + ar3 + … + arn + … .
From algebra, it will be recalled that this series is convergent when r < 1, and divergent when r 
l.
(2)The p-Series:
(a)When p > 1, the p-series converges, as is shown in standard texts in algebra.
(b)When p = 1, the p-series becomes the harmonic series, which has already been shown to be divergent (§11—3, Example 3).
(c)When p < 1, the p-series is also divergent, since the terms, after the first, are greater than the corresponding terms of the harmonic series.
(3)The Telescopic Series:
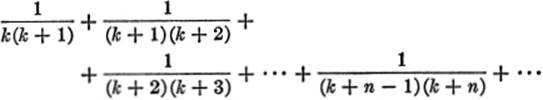
where k is a constant > 0. It can be shown that in this series,
hence, by §11—2, this series is convergent when k > 0.
(4)The Arithmetic Series: The series
a+ (a + d) + (a + 2d) + ... +[a+ (n – l)d] + ...
obviously diverges for all values of a and d, since, by §11—5, [2], 
11—8. Summary of Series Used for Comparisons.
I.Convergent Series:
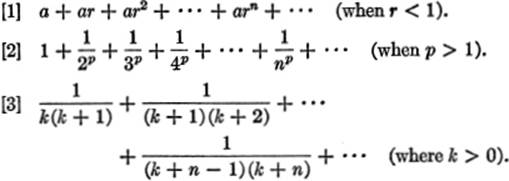
II.Divergent Series:
[4] a + ar + ar2 + … + arn + … (when r 1).
[6] a + (a + d) + (a + 2d) + … + [a + (n − 1)d] + …;
EXAMPLE 1. Show that the series
Solution. We write the given series as
Comparing (1) with the harmonic series, §11—8, [5], omitting the first term,
we see that each term of (1) is greater than the corresponding term of (2). But (2) is known to be divergent; hence (1) is also divergent.
NOTE. The convergence or divergence of a series is not affected by dropping off (or adding on) a finite number of terms; this merely affects the value of Sn, not its existence.
EXAMPLE 2. Show that the series
Solution. Compare the given series with the p-series (§11—8, [2]) when p = 2:
Each term of (1) is equal to or less than the corresponding term of (2); hence (1) is convergent.
EXAMPLE 3. Test for convergence or divergence:
Solution. Compare (1) with the harmonic series (§11—8, [5]):
Each term of (1) is equal to or greater than the corresponding term of (2), since 
, etc.; hence (2) is divergent.
EXAMPLE 4. Test for convergence:
Solution. Compare (1) with the geometric series
Series (1) may be written
Since each term of (3) is equal to or less than the corresponding term of (2), then, by §11—8, [1], series (1) is convergent.
EXERCISE 11—2
Test the following series for convergence or divergence by using the comparison test:
11—9. Ratio Tests for Convergence and Divergence. In addition to the comparison tests discussed in §11—8, a number of so-called ratio tests can be used to determine convergence and divergence. The commonest of these is the ratio of the (n + l)st term to the nth term, or the test ratio un+1/un. It can be shown that, if the absolute value of the limit of this ratio as n — ∞ exists, and is designated by R, then:
(A)If R < 1, the series converges;
(B)If R > 1, the series diverges;
(C)If R = 1, the test fails;
where 
Using the absolute value of the ratio enables us to employ the test ratio for series which have negative as well as positive terms.
EXAMPLE 1. Using the ratio test, prove the convergence of
Solution. Here the general term un equals 
; hence
Therefore:
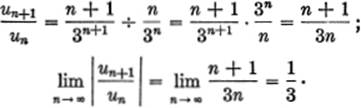
Hence the series is convergent, since R < 1.
EXAMPLE 2. Test for convergence or divergence:
Solution. Here
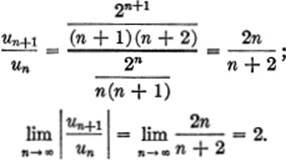
Hence R > 1, and the series is divergent.
EXAMPLE 3. Test for convergence or divergence:
Thus the test fails in this case. Some other method must be used. Comparing the given series with the harmonic series
shows that the original series is divergent.
EXERCISE 11—3
By using the ratio test, determine the convergence or divergence of the following series:
11—10. Alternating Series. Two basic principles, given here without proof, are useful in discussing alternating series, that is, series whose terms are alternating positive and negative.
I. An alternating series converges if the absolute value of each term is less than that of the preceding term, and if the nth term approaches zero as n becomes infinite.
EXAMPLE. 
is convergent.
II. A series of real terms converges if the series formed from the absolute values of the terms converges; that is, a series u1 + u2 + u3 + … converges if the series |u1| + |u2| + |u3| + … converges.
EXAMPLE. The series
is convergent
because the series
converges.