Pre-Calculus For Dummies, 2nd Edition (2012)

Part II. The Essentials of Trigonometry

Chapter 10. Taking Charge of Oblique Triangles with the Laws of Sines and Cosines

In This Chapter

Mastering the Law of Sines

Wielding the Law of Cosines

In order to solve a triangle, you need to find the measures of all three angles and the lengths of all three sides. Three of these pieces of information are given to you as part of the math problem, so you need to find only the other three. Up until now throughout this book, we work in depth with right triangles. In Chapter 6, we help you find the lengths of missing sides by using the Pythagorean theorem, find missing angles by using right-triangle trigonometry, and evaluate trig functions for specific angles. But what happens if you need to solve a triangle that isn’t right?

You can connect any three points in a plane to form a triangle. Unfortunately, in the real world, these triangles won’t always be right triangles. Finding missing angles and sides of oblique triangles can be more confusing because they don’t have a right angle. And without a right angle, the triangle has no hypotenuse, which means the Pythagorean theorem is useless. But don’t worry; this chapter shows you the beaten path. The Law of Sines and the Law of Cosines are two methods that you can use to solve for missing parts of oblique triangles. The proofs of both laws are long and complicated, and you don’t need to concern yourself with them. Instead, use these laws as formulas in which you can plug in information given to you and use algebra to solve for the missing pieces. Whether you use sines or cosines to solve the triangle, the types of information (sides or angles) given to you and their location on the triangle are factors that help you decide which method is the best to use.

You may be wondering why we don’t discuss the Law of Tangents in this chapter. The reason is simple: You can solve every oblique triangle with either the Law of Sines or the Law of Cosines, which are far less complicated than the Law of Tangents. Textbooks rarely refer to it, and teachers often steer clear.

The techniques we present here have tons of real-world applications, too. You can deal with everything from sailing a boat to putting out a forest fire by using triangles. For example, if two forest-fire stations get a call for a fire, they can use the Law of Cosines to figure out which station is closest to the fire.

Before attempting to solve a triangle, always draw a picture that has the sides and angles clearly labeled. This approach helps you visualize which pieces of information you still need. You can use the Law of Sines whenever there’s enough information provided in the problem to give you a pair: an angle measure and the side length across from it. So if you have the time, try to use the Law of Sines first. If the Law of Sines isn’t an option, you’ll know because you won’t have both a given angle and its partner side. When that happens, the Law of Cosines is there to save the day. The Law of Cosines is specifically needed when you have just the three sides provided with no angles or when you have two sides provided with the angle between them.

Whether you’re using the Law of Sines or the Law of Cosines to solve for missing parts of a triangle, try not to do any of the calculations using your calculator until the very end. Using the calculator too early gives you more rounding error in your final answers. So for example, instead of evaluating the sines of all three angles and using the decimal approximations from the beginning, solve the equations and plug the final (extremely complicated) numeric expression into your calculator all at one time.

Solving a Triangle with the Law of Sines

You use the Law of Sines to find the missing parts of a triangle when you’re given any three pieces of information involving at least one angle and at least one side directly opposite from it. This information comes in three forms:

ASA (angle-side-angle): You’re given two angles and the side in between them.

AAS (angle-angle-side): You’re given two angles and a consecutive side.

SSA (side-side-angle): You’re given two sides and a consecutive angle.

Following is the formula for the Law of Sines:

In order to solve for an unknown variable in the Law of Sines, you set two of the fractions equal to each other and use cross multiplication. When you’re setting up for the cross multiplication, you can set any two parts equal to each other, but be careful not to have one equation with two unknown variables.

The bummer about problems that use the Law of Sines is that they take some time and careful work. Even though you may be tempted to try to solve everything at once, take it one small step at a time. And don’t overlook the obvious in order to blindly stick with the formula. If you’re given two angles and one side, for instance, finding the third angle is easy because all angles in a triangle must add up to 180°. Fill in the formula with what you know and get crackin’!

In the following sections, we show you how to solve a triangle in different situations using the Law of Sines.

When you solve for an angle by using the Law of Sines, you have to assume that a second set of solutions (or none at all) may exist. We cover that conundrum in this section as well. (In case you’re really curious, that consideration applies only when you’re working with a problem where you know two side measures and one angle measure of a triangle.)

When you know two angle measures

In this section, we take a look at the first two cases where you can use the Law of Sines to solve a triangle: angle-side-angle (ASA) and angle-angle-side (AAS). Whenever you’re given two angles, find the third one immediately and work from there. In both of these cases, you can find exactly one solution for the triangle in question.

A side sandwich: ASA

An ASA triangle means that you’re given two angles and the side between them in a problem. For example, a problem could state that ∠A = 32°, ∠B = 47°, and c = 21, as in Figure 10-1. You also could be given ∠A, ∠C, and b,or ∠B, ∠C, and a. Figure 10-1 has all the given and unknown parts labeled for you.

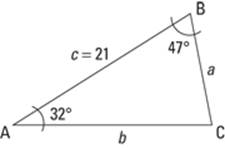
To find the missing information with the Law of Sines, follow these steps:

1. Determine the measure of the third angle.

As a rule, ∠A + ∠B + ∠C = 180°. So by plugging in what you know about the angles in this problem, you can solve for the missing angle:

32° + 47° + ∠C = 180°

180° – 79° = ∠C = 101°

2. Set up the Law of Sines formula, filling in what you know.

3. Set two of the parts equal to each other and cross multiply.

We use the first and third fractions, which look like this:

Cross multiplying, you have a(sin 101°) = 21(sin 32°).

4. Find the decimal approximation of the missing side using your calculator.

Because sin 101° is just a number, you can divide both sides of the equation by it to isolate the variable:

5. Repeat Steps 3 and 4 to solve for the other missing side.

Setting the second and third fractions equal to each other, you have this equation:

This equation becomes b(sin 101°) = 21(sin 47°) when you cross multiply. Isolate the variable and solve for it:

b ≈ 15.65

6. State all the parts of the triangle as your final answer.

Some answers may be approximate, so make sure you maintain the proper signs:

• ∠A = 32° a ≈ 11.34

• ∠B = 47° b ≈ 15.65

• ∠C = 101° c = 21

Leaning toward the angle side: AAS

In many trig problems, you’re given two angles and a side that isn’t between them. This type of problem is called an AAS problem. For example, you may be given ∠B = 68°, ∠C = 29°, and b = 15.2, as shown by Figure 10-2. Notice that if you start at side b and move counterclockwise around the triangle, you come to ∠C and then ∠B. This check is a good way to verify whether a triangle is an example of AAS.

After you find the third angle, an AAS problem just becomes a special case of ASA. Here are the steps to solve:

1. Determine the measure of the third angle.

You can say that 68° + 29° + ∠A = 180°. Then ∠A = 83°.

2. Set up the Law of Sines formula, filling in what you know.

3. Set two of the parts equal to each other and then cross multiply.

We choose to use a and b:

Cross multiplying, you have a(sin 68°) = 15.2(sin 83°).

4. Solve for the missing side.

You divide by sin 68°, so

a ≈ 16.27

5. Repeat Steps 3 and 4 to solve for the other missing side.

Setting b and c equal to each other, you have this equation:

Cross multiply:

15.2(sin 29°) = c(sin 68°)

Divide by sin 68° to isolate the variable and solve:

c ≈ 7.95

6. State all the parts of the triangle as your final answer.

Your final answer sets up as follows:

• ∠A = 83° a ≈ 16.27

• ∠B = 68° b = 15.2

• ∠C = 29° c ≈ 7.95

When you know two consecutive side lengths

In some trig problems, you may be given two sides of a triangle and an angle that isn’t between them, which is the classic case of SSA. In this scenario, you may have one solution, two solutions, or no solutions.

Wondering why the number of solutions varies? Recall from geometry that you can’t prove that two triangles are congruent using SSA, because these conditions can build you two triangles that aren’t the same. Figure 10-3 shows two triangles that fit SSA but aren’t congruent.

If you begin with an angle and then continue around to draw the other two sides, you’ll find that sometimes you can’t make a triangle with those measurements. And sometimes, you can make two different triangles. Unfortunately, the latter means actually solving two different triangles.

Most SSA cases have only one solution, because if you use what you’re given to sketch the triangle, most of the time you’ll have only one way to draw it. When you’re faced with an SSA problem, you may be tempted to figure out how many solutions you need to find before you start the solving process. Not so fast! In order to determine the number of possible solutions in an SSA problem, you should start solving first. You’ll either arrive at one solution or find that no solutions exist (because you get an error message in your calculator). If you find one solution, you can look for the second set of solutions. If you get a negative angle in the second set, you’ll know that the triangle only has one set of solutions.

In our opinion, the best approach is to always assume that you’ll find two solutions, because remembering all the rules that determine the number of solutions probably will take up far too much time and energy (which is why we don’t even go into them here; they’re too complicated and too variable heavy). If you treat every SSA problem as if it has two solutions until you gather enough information to prove otherwise, you’ll be twice as likely to find all the appropriate solutions.

Preparing for the worst: Two solutions

Gaining some experience with solving a triangle that has more than one solution is helpful. The first set of solutions that you find in such a situation is always an acute triangle. The second set of solutions is an obtuse triangle. Remember to always look for two solutions for any problem.

For example, say you’re given a = 16, c = 20, and ∠A = 48°. Figure 10-4a shows you what the picture may look like. However, couldn’t the triangle also look like Figure 10-4b? Both situations follow the constraints of the given information of the triangle. If you start by drawing your picture with the given angle, the side next to the angle has a length of 20, and the side across from the angle is 16 units long. The triangle could be formed two different ways. Angle C could be an acute angle or an obtuse angle; the given information isn’t restrictive enough to tell you which one it is. Therefore, you have to find both sets of solutions.

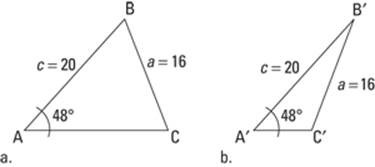
Solving this triangle by using steps similar to those described for both the ASA and AAS cases gives you the two possible solutions shown in Figure 10-4. Because you have two missing angles, you need to find one of them first, which is why the steps here are different than the other two cases:

1. Fill in the Law of Sines formula with what you know.

The formula here sets up like this:

2. Set two fractions equal to each other so that you have only one unknown.

If you decide to solve for ∠C, you set the first and third fractions equal to each other, so you have this equation:

3. Cross multiply and isolate the sine function.

This step gives you 20(sin 48°) = 16(sin C). To isolate the sine function, you divide by 16:

4. Take the inverse sine of both sides.

The right-hand side goes right into your handy calculator to give you ∠C ≈ 68.27°.

5. Determine the third angle.

You know that 48° + 68.27° + ∠B = 180°, so ∠B ≈ 63.73°.

6. Plug the final angle back into the Law of Sines formula to find the third side.

This step gives you 16(sin 63.73°) = b(sin48°).

Finally, you can solve:

Of course, this solution to the triangle isn’t the only one. Refer to Step 4, where you solved for ∠C, and then look at Figure 10-5.

Triangle ABC is the solution that you solve for in the previous steps. Triangle AB'C' is the second set of solutions you must look for. A certain trig identity isn’t used in solving or simplifying trig expressions because it isn’t helpful for those, but it is useful for solving triangles. This identity says that sin(180° – θ) = sin θ.

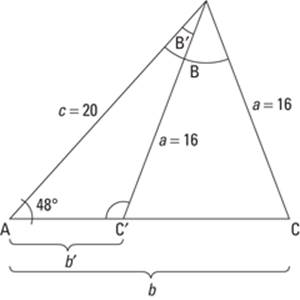
In the case of the previous example, sin(68.27°) = sin(180° – 68.27°) = sin(111.73°). Notice that although sin 68.27° ≈ 0.9319, sin 111.73° ≈ 0.9319 as well. However, if you plug sin^–1(0.9319) into your calculator to solve for θ,68.27° is the only solution you get. Subtracting this value from 180° gives you the other ambiguous solution for ∠C, which is usually denoted as ∠C' so you don’t confuse it with the first solution.

The following steps build on these actions so you can find all the solutions for this SSA problem:

1. Use the trig identity sin(180° – θ) = sin θ to find the second angle of the second triangle.

Because ∠C ≈ 68.27°, subtract this value from 180° to find that ∠C' ≈ 111.73°.

2. Find the measure of the third angle.

If ∠A = 48° and ∠C' ≈ 111.73°, then ∠B' ≈ 20.27° because the three angles must add to 180°.

3. Plug these angle values into the Law of Sines formula.

4. Set two parts equal to each other in the formula.

You need to find b'. Set the first fraction equal to the second:

5. Cross multiply to solve for the variable.

You set b'(sin 48°) = 16(sin 20.27°). Isolate b' to get this solution:

6. List all the answers to the two triangles (see the previous numbered list).

Originally, you were given that a = 16, c = 20, and ∠A = 48°. The answers that you found are as follows:

• First triangle: ∠B ≈ 63.73°, ∠C ≈ 68.27°, b = 19.31

• Second triangle: ∠B' ≈ 20.27°, ∠C' ≈ 111.73°, b' ≈ 7.46

Arriving at the ideal: One solution

If you don’t get an error message in your calculator when attempting to solve a triangle, you know you can find at least one solution. But how do you know if you’ll find only one? The answer is, you don’t. Keep solving as if a second one exists, and in the end, you will see whether there’s only one.

For example, say you’re asked to solve a triangle if a = 19, b = 14, and ∠A = 35°. Figure 10-6 shows what this triangle looks like.

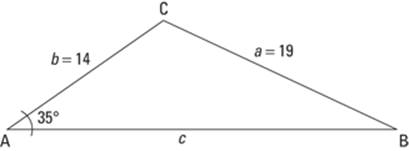
Because you know only one of the angles of the triangle, you use the two given sides and the given angle to find one of the missing angles first. That process leads you to the third angle and then the third side. Follow these steps to solve this triangle:

1. Fill in the Law of Sines formula with what you know.

2. Set two parts of the formula equal to each other.

Because you’re given a, b, and A, solve for ∠B first. If you try to solve for side c or ∠C first, you’ll have two unknown variables in your equation, which would create a dead end.

Following this advice, you have this equation:

3. Cross multiply the equation.

You set 19(sin B) = 14(sin 35°).

4. Isolate the sine function.

5. Take the inverse sine of both sides of the equation.

This step sets up as

which simplifies to

6. Determine the measure of the third angle.

You know that 35° + 25° + ∠C = 180°, so ∠C ≈ 120°.

7. Set the two parts equal to each other so that you have only one unknown.

8. Cross multiply and then isolate the variable to solve.

You start with 19(sin 120°) = c(sin 35°), so you rearrange to get this equation:

c ≈ 28.69

9. Write out all six pieces of information devised from the formula.

Your answer sets up as follows:

• ∠A = 35° a = 19

• ∠B = 25° b = 14

• ∠C = 120° c ≈ 28.69

10. Look for a second set of solutions.

The first thing you did in this example was to find ∠B. You see from Step 5 that B is approximately 25°. If the triangle has two solutions, the measure of B' is 180° – 25°, or 155°. Then, to find the measure of angle ∠C', you start with ∠A + ∠B' + ∠C' = 180°. This equation simplifies to 35° + 155° + ∠C' = 180°, or ∠C' = –10°.

Angles can’t have negative measures, so this answer tells you that the triangle has only one solution. Don’t you feel better knowing that you exhausted the possibilities?

Kind of a pain: No solutions

If a problem gives you an angle and two consecutive sides of a triangle, you may find that the second side won’t be long enough to reach the third side of the triangle. In this situation, no solution exists for the problem. However, you may not be able to tell this just by looking at the picture — you really need to solve the problem to know for sure. So begin to solve the triangle just as you do in the previous sections.

For example, say b = 19, ∠A = 35°, and a = 10. Figure 10-7 shows what the picture should look like.

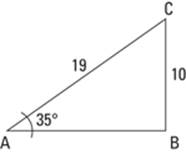
If you start solving this triangle by using the methods from previous setups, something very interesting happens: Your calculator gives you an error message when you try to find the unknown angle. This error is because the sine of an angle must be between –1 and 1. If you try to take the inverse sine of a number outside this interval, the value for the angle is undefined (meaning it doesn’t exist). The following steps illustrate this occurrence:

1. Fill in the Law of Sines formula with what you know.

2. Set two fractions equal and cross multiply.

You start with this equation:

and end up with 19(sin 35°) = 10(sin B).

3. Isolate the sine function.

This step lets you solve for sin B:

sin B ≈ 1.09

4. Take the inverse sine of both sides to find the missing angle.

Notice that you get an error message when you try to plug this into your calculator, which happens because sin B ≈ 1.09 but the sine of an angle can’t be larger than 1 or less than –1. Therefore, the measurements given can’t form a triangle, meaning that the problem has no solution.

Conquering a Triangle with the Law of Cosines

You use the Law of Cosines formulas to solve a triangle if you’re given one of the following situations:

Two sides and the included angle (SAS)

All three sides of the triangle (SSS)

In order to solve for the angles of a triangle by using the Law of Cosines, you first need to find the lengths of all three sides. You have three formulas at your disposal to find missing sides, and three formulas to find missing angles. If a problem gives you all three sides to begin with, you’re all set because you can manipulate the side formulas to come up with the angle formulas (we explain how in the following section). If a problem gives you two sides and the angle between them, you first find the missing side and then find the missing angles.

To find a missing side of a triangle, use the following formulas, which comprise the Law of Cosines:

a² = b² + c² – 2bc cos A

b² = a² + c² – 2ac cos B

c² = a² + b² – 2ab cos C

The side formulas are very similar to one another, with only the letters changed around. So if you can remember just two of them, you can change the order to quickly find the other. The following sections put the Law of Cosines formulas into action to solve SSS and SAS triangles.

When you use the Law of Cosines to solve a triangle, you find only one set of solutions (one triangle), so don’t waste any time looking for a second set. With this formula, you’re solving SSS and SAS triangles from triangle congruence postulates in geometry. You can use these congruence postulates because they lead to only one triangle every time. (For more on geometry rules, check out Geometry For Dummies, by Mark Ryan [Wiley].)

SSS: Finding angles using only sides

Some textbooks provide three formulas students can use to solve for an angle by using the Law of Cosines. However, you don’t have to memorize the three angle formulas to solve SSS problems. If you remember the formulas to find missing sides using the Law of Cosines (see the introduction to this section), you can use algebra to solve for an angle. Here’s how, for example, to solve for angle A:

1. Start with the formula.

a² = b² + c² – 2bc cos A

2. Subtract b² from both sides.

a² – b² = c² – 2bc cos A

3. Subtract c² from both sides.

a² – b² – c² = –2bc cos A

4. Divide both sides by –2bc.

5. Distribute the negative and rearrange terms.

6. Take the inverse cosine of both sides.

The same process applies to finding angles B and C, so you end up with these formulas for finding angles:

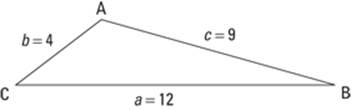
Suppose you have three pieces of wood of different lengths. One board is 12 feet long, another is 9 feet long, and the last is 4 feet long. If you want to build a sandbox using these pieces of wood, at what angles must you lay down all the pieces so that each side meets? If each piece of wood is one side of the triangular sandbox, you must use the Law of Cosines to solve for the three missing angles.

Let a = 12, b = 4, and c = 9. You can find any of the angles first. Follow these steps to solve:

1. Decide which angle you want to solve for first and then plug the sides into the formula.

We solve for ∠A:

2. Solve for the other two angles.

∠B:

∠C:

3. Check your answers by adding the angles you found.

You find that 130.75° + 14.63° + 34.62° = 180°.

Picturing your solutions, the angle across from the 12-foot board (∠A) needs to be 130.75°; the angle across from the 4-foot board (∠B) needs to be 14.63°; and the angle across from the 9-foot board (∠C) needs to be 34.62°. See Figure 10-8.

SAS: Tagging the angle in the middle (and the two sides)

If a problem gives you the lengths of two sides of a triangle and the measure of the angle in between them, you use the Law of Cosines to find the other side (which you need to do first). When you have the third side, you can easily use all the side measures to calculate the remaining angle measures.

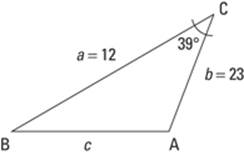
For example, if a = 12, b = 23, and ∠C = 39°, you solve for side c first and then solve for ∠A and ∠B. Follow these simple steps:

1. Sketch a picture of the triangle and clearly label all given sides and angles.

By drawing a picture, you can make sure that the Law of Cosines is the method you should use to solve the triangle. Figure 10-9 has all the parts labeled.

2. Decide which side formula you need to use first.

Because sides a and b are given, you use the following formula to find side c:

c² = a² + b² – 2ab cos C

3. Plug the given information into the proper formula.

This step gives you this equation:

c² = (12)² + (23)² – 2(12)(23)cos(39°)

If you have a graphing calculator, you can plug in this formula exactly as it’s written and then skip directly to Step 6. If you don’t have a graphing calculator (meaning you’re using a scientific calculator), be very mindful of the order of operations.

Following the order of operations when using the Law of Cosines is extremely important. If you try to type the pieces into your calculator all in one step, without the correct use of parentheses, your results probably will be incorrect. Be sure you’re comfortable with your calculator. Some scientific calculators require that you type in the degrees before you hit the trig-function button. If you try to type in an inverse cosine without parentheses to separate the top and bottom of the fraction (see Step 7), your answer will be incorrect as well. The best method is to do all the squaring separately, combine like terms in the numerator and the denominator, divide the fraction, and then take the inverse cosine, as you’ll see from this point on.

4. Square each number and multiply by the cosine separately.

You end up with this equation:

c² = 144 + 529 – 428.985

5. Combine all the numbers.

c² = 244.015

6. Square root both sides.

c ≈ 15.6

7. Find the missing angles.

Starting with ∠A, you set up the formula (from the preceding section), plug in the info you know, and solve for A:

When using your graphing calculator, be sure to use parentheses to separate the numerator and denominator from each other. Put parentheses around the whole numerator and the whole denominator.

You can find the third angle quickly by subtracting the sum of the two known angles from 180°. However, if you’re not pressed for time, we recommend that you use the Law of Cosines to find the third angle because it allows you to check your answer.

Here’s how you find ∠B:

8. Check to make sure that all angles add to 180°.

39° + 28.9° + 112.2° = 180.1°

Because of rounding error, sometimes the angles don’t add to 180° exactly. For most instructors, an answer within a half a degree or so is considered acceptable.

Filling in the Triangle by Calculating Area

Geometry provides a nice formula to find the area of triangles: A = 1/2 · b · h. This formula comes in handy only when you know the base and the height of the triangle. But in an oblique triangle, where is the base? And what is the height? You can use two different methods to find the area of an oblique triangle, depending on the information you’re given.

Finding area with two sides and an included angle (for SAS scenarios)

Lucky Heron (see the next section) got a formula named after him, but the SAS guy to whom this section is dedicated remains nameless.

You use the following formula to find the area when you know two sides of a triangle and the angle between those sides (SAS):

Area = (1/2)a b sin C

In the formula, C is the angle between sides a and b.

For example, when building the sandbox — as in the earlier section “SSS: Finding angles using only sides” — you know that a = 12 and b = 4, and you find using the Law of Cosines that C = 34.62. Now you can find the area:

Area = (1/2)(12)(4) sin 34.62 ≈ 13.64

Using Heron’s Formula (for SSS scenarios)

You can find the area of a triangle when given only the lengths of all three sides (no angles, in other words) by using a formula called Heron’s Formula. It says that

where

The variable s is called the semiperimeter — or half the perimeter.

For example, you can find the area of the sandbox (see the example from the previous section) without having to solve for any angles. When you know all three sides, you can use Heron’s Formula. For a triangle with sides 4, 9, and 12, follow these steps:

1. Calculate the semiperimeter, s.

Follow this simple calculation:

2. Plug s, a, b, and c into Heron’s Formula.

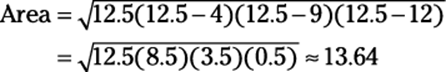
You find that the area of the sandbox is the same with both formulas we present here. In the future the triangles won’t be the same, but you’ll still know when to use the SAS formula and when to use Heron’s Formula.