SAT SUBJECT TEST MATH LEVEL 2
PART 2
REVIEW OF MAJOR TOPICS
CHAPTER 2
Geometry and Measurement
• Coordinate Geometry
• Three-Dimensional Geometry
2.1 Coordinate Geometry
TRANSFORMATIONS AND SYMMETRY
The three types of transformations in the coordinate plane are translations, stretches/shrinks, and reflections. Translation preserves the shape of a graph, only moving it around the coordinate plane. Translation is accomplished by addition. Changing the scale (stretching and shrinking) can change the shape of a graph. This is accomplished by multiplication. Finally, reflection preserves the shape and size of a graph but changes its orientation. Reflection is accomplished by negation.
Suppose y = f(x) defines any function.
• y = f(x) + k translates y = f(x) k units vertically (up if k > 0; down if k < 0)
• y = f(x – h) translates y = f(x) h units horizontally (right if h > 0; left if h < 0)
EXAMPLES
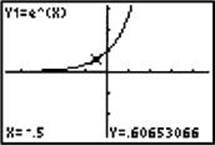
1. Suppose y = f(x) = ex . Describe the graph of y = ex + 3.
In this example, k = 3, so ex + 3 = f(x) + 3. As shown in the figures below, each point on the graph of y = ex + 3 is 3 units above the corresponding point on the graph of y = ex .
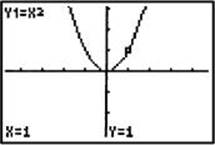
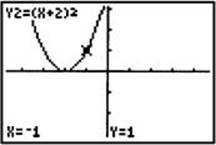
2. Suppose y = x2. Describe the graph of y = (x + 2)2.
In this example h = –2, so (x + 2)2 = f(x + 2). As shown in the figures below, each point on the graph of y = (x + 2)2 is 2 units to the left of the corresponding point on the graph of y = x2.
• y = af(x) stretches (shrinks) f(x) vertically by a factor of |a| if |a| > 1(|a| < 1).
• y = f(ax) shrinks (stretches) f(x) horizontally by a factor of 
if |a| > 1(|a| < 1).
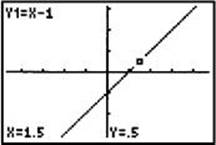
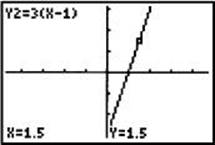
3. Suppose y = x – 1. Describe the graph of y = 3(x – 1).
In this example |a| = 3, so 3(x + 1) = 3f(x). As shown in the graphs below, for x = 1.5 the y-coordinate of each point on the graph of y = 3(x – 1) is 3 times the y-coordinate of the corresponding point on the graph of y = x – 1.
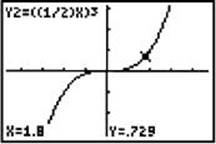
4. Suppose y = x3. Describe the graph of 
.
In this example, 
. As shown in the graphs below, for y = 0.729 the x-coordinate of each point on the graph of 
is 2 times the x- coordinate of the corresponding point on the graph of y = x3.
• y = –f(x) reflects y = f(x) about the x -axis. (The reflection is vertical.)
• y = f (–x) reflects y = f(x) about the y-axis. (The reflection is horizontal.)
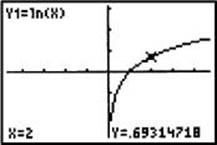
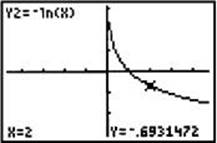
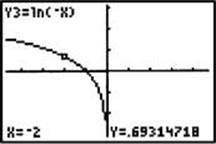
5. Suppose y = ln x . Describe the graphs of y = –ln x and y = ln(–x).
As shown in the graphs below, the graph of y = –ln x is the reflection of the graph of y = ln x about the x -axis, and the graph of y = ln (–x) is the reflection about the y-axis.
Translations, stretching/shrinking, and reflections can be combined to produce functions. Vertical transformations occur when adding, multiplying, or negating takes place after the function is applied (i.e., to y). The order in which multiple vertical transformations are executed does not matter. Horizontal transformations occur when adding, multiplying, or negating takes place before the function is applied (i.e., to x). These transformations must be taken in the following order: reflect; change the scale; then translate. Moreover, the scale factor |a| must be factored out of a translation.
6. Suppose y = f(x). Use words to describe the transformation y = f (–ax + b).
Observe that all of these transformations are horizontal. First we have to write –ax + b as 
. The x-coordinate of a point (x,y) on the graph of y = f(x) goes through the following sequence of transformations: reflected about the y-axis; horizontally shrunk by a factor of 
or stretched by a factor of |a|; and translated 
to the right.
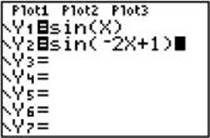
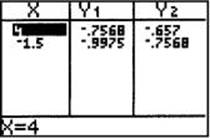
7. Suppose y = sin x . Describe the sequence of transformations to get the graph of y = sin(–2x + 1).
Observe that all transformations are horizontal. Write the function as 
. Consider the point (4,–0.7568 . . .) on the graph of y = sin x . First reflect this point about the y-axis to (–4,–0.7568...). Then shrink by a factor of 
to (–2,–0.7568...). Then translate units right, to (–1.5,–0.7568...). The screens below show Y1 = sin x and Y2 = sin(–2x + 1) and a table showing the points (4,–0.7568...) for Y1 and (–1.5,–0.7568...) for Y2.
Reflections about the x - and y-axes represent two types of symmetry in a graph. Symmetry through the origin is a third type of graphical symmetry. A graph defined by an equation in x and y is symmetrical with respect to the
• x -axis if replacing x by –x preserves the equation;
• y-axis if replacing y by –y preserves the equation; and
• origin if replacing x and y by –x and –y, respectively, preserves the equation.
These symmetries are defined for functions as follows:
• Symmetry about the y-axis: f(x) = f (–x) for all x .
• Symmetry about the x -axis: f(x) = –f(x) for all x .
• Symmetry about the origin: f(x) = –f (–x) for all x .
As defined previously, functions that are symmetric about the y-axis are even functions, and those that are symmetric about the origin are odd functions.
8. Discuss the symmetry of f(x) = cos x .
Since cos x = cos (–x), cosine is symmetric about the y-axis (an even function).
However, since cos x 
–cos x and cos (–x) –cos x, the cosine is not symmetric with respect to the x -axis or origin.
9. Discuss the symmetry of x2 + xy + y2 = 0.
If you substitute –x for x or –y for y, but not both, the equation becomes x2 – xy + y2 = 0, which does not preserve the equation. Therefore, the graph is not symmetrical with respect to either axis. However, if you substitute both –x for x and –y for y, the equation is preserved, so the equation is symmetric about the origin.
EXERCISES
1. Which of the following functions transforms y = f(x) by moving it 5 units to the right?
(A) y = f(x + 5)
(B) y = f(x – 5)
(C) y = f(x) + 5
(D) y = f(x) – 5
(E) y = 5f(x)
2. Which of the following functions stretches y = cos (x) vertically by a factor of 3?
(A) y = cos(x + 3)
(B) y = cos(3x)
(C) 
(D) y = 3 cos x
(E) 
3. The graph of y = f(x) is shown.
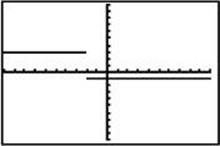
Which of the following is the graph of y = f (–x) – 2?
(A)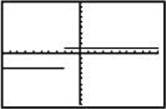 
(B)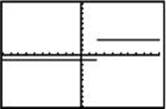 
(C)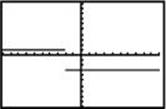 
(D)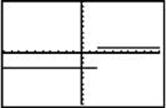 
(E) 
4. Which of the following is a transformation of y = f(x) that translates this function down 3, shrinks it horizontally by a factor of 2, and reflects it about the x -axis.
(A) y = –2f(x – 3)
(B) y = f (–2x) – 3
(C) 
(D) y = –f (2x) – 3
(E) 