Miscellaneous - SAT Math 1 & 2 Subject Tests

SAT Math 1 & 2 Subject Tests

Chapter 11 Miscellaneous

The techniques and rules covered in this chapter are relatively rare on the Math Subject Tests. They occur only on the Math Level 2 or the difficult third of the Math Level 1; if you”re not supposed to be tackling those questions, don”t waste your time on this chapter. If you will take the Math Level 2, or will take the Math Level 1 very aggressively, then it”s a good idea to learn the rules in this chapter—but remember, the material in the preceding chapters is still more important.

LOGARITHMS

Exponents can also be written in the form of logarithms. For example, log₂ 8 represents the exponent that turns 2 into 8. In this case, the “base” of the logarithm is 2. It”s easy to make a logarithmic expression look like a normal exponential expression. Here you can say log₂ 8 = x, where x is the unknown exponent that turns 2 into 8. Then you can rewrite the equation as 2^x = 8. Notice that, in this equation, 2 is the base of the exponent, just as it was the base of the logarithm. Logarithms can be rearranged into exponential form using the following definition:

Definition of a Logarithm

log_b n = x ⇔ b^x = n

A logarithm that has no written base is assumed to be a base-10 logarithm. Base-10 logarithms are called “common logarithms,” and are so frequently used that the base is often left off. Therefore, the expression “log 1,000” means log₁₀ 1,000. Most calculations involving logarithms are done in base-10 logs. When you punch a number into your calculator and hit the “log” button, the calculator assumes you”re using a base-10 log. There will be times when you”re dealing with other bases. A nifty formula allows you to use your calculator to evaluate logs with other bases.

Change of Base Formula

log_B A =

For example, log₇ 54 can be entered into your calculator as log(54)/log(7), which gives you 2.0499.

DRILL

Test your understanding of the definition of a logarithm with the following exercises. The answers to these drills can be found in Chapter 12.

1. log₂ 32 =________________

2. log₃ x = 4: x =________________

3. log 1000 =________________

4. logb 64 = 3: b =________________

5. x^log_x _y=________________

6. log₇ 1 =________________

7. log_x x =________________

8. logx x¹² =________________

9. log 37 =________________

10. log 5 =________________

A Nifty Trick!

So you know how any
number to the first power
is that same number? For
example, 4¹ = 4. Well, that
means that log₄4 = 1. Any
log that has the same
base as number is going to
equal 1. So any time you
see that, no work is necessary.
Pretty cool, huh?

For the Math Level 1 Subject Test, that”s about all you need to know about logarithms. As long as you can convert them from logarithmic form to exponential form, you should be able to handle any logarithm question you run into. For the Math Level 2 Subject Test, however, you will need to work with logarithms in more complicated ways.

Logarithmic Rules

There are three properties of logarithms that are often useful on the Math Level 2 Subject Test. These properties are very similar to the rules for working with exponents—which isn”t surprising, because logarithms and exponents are the same thing. The first two properties deal with the logarithms of products and quotients.

The Product Rule

log_b (xy) = log_b x + log_b y

The Quotient Rule

log_b () = log_b x − log_b y

These rules are just another way of saying that when you multiply terms, you add exponents, and when you divide terms, you subtract exponents. Be sure to remember that when you use them, the logarithms in these cases all have the same base.

The third property of logarithms deals with the logarithms of terms raised to powers.

The Power Rule

log_b (x ^r) = r log_b x

This means that whenever you take the logarithm of a term with an exponent, you can pull the exponent out and make it a coefficient.

log (7²) = 2 log 7 = 2(0.8451) = 1.6902

log₃ (x⁵) = 5 log₃ x

These logarithm rules are often used in reverse to simplify a string of logarithms into a single logarithm. Just as the product and quotient rules can be used to expand a single logarithm into several logarithms, the same rules can be used to consolidate several logarithms that are being added or subtracted into a single logarithm. In the same way, the power rule can be used backward to pull a coefficient into a logarithm, as an exponent. Take a look at how these rules can be used to simplify a string of logarithms with the same base.

log 8 + 2 log 5 − log 2 =

log 8 + log 5² − log 2 = (Power Rule)

log 8 + log 25 − log 2 =

log (8 × 25) − log 2 = (Product Rule)

log 200 − log 2 =

log () = (Quotient Rule)

log 100 = 2

DRILL

In the following exercises, use the Product, Quotient, and Power rules of logarithms to simplify each logarithmic expression into a single logarithm with a coefficient of 1. The answers to these drills can be found in Chapter 12.

1. log 5 + 2 log 6 − log 9 =

2. 2 log₅ 12 − log⁵ 8 − 2 log⁵ 3 =

3. 4 log 6 − 4 log 2 − 3 log 3 =

4. log₄ 320 − log₄ 20 =

5. 2 log 5 + log 3 =

Logarithms in Exponential Equations

Logarithms can be used to solve many equations that would be very difficult or even impossible to solve any other way. The trick to using logarithms in solving equations is to convert all of the exponential expressions in the equation to base-10 logarithms, or common logarithms. Common logarithms are the numbers programmed into your calculator”s logarithm function. Once you express exponential equations in term of common logarithms, you can run the equation through your calculator and get real numbers.

When using logarithms to solve equations, be sure to remember the meaning of the different numbers in a logarithm. Logarithms can be converted into exponential form using the definition of a logarithm provided at the beginning of this section.

Let”s take a look at the kinds of tough exponential equations that can be solved using logarithms:

39. If 5x = 2⁷⁰⁰, then what is the value of x ?

This deceptively simple equation is practically impossible to solve using conventional algebra. Two to the 700th power is mind-bogglingly huge; there”s no way to calculate that number. There”s also no way to get x out of that awkward exponent position. This is where logarithms come in. Take the logarithm of each side of the equation.

log 5^x = log 2⁷⁰⁰

Now use the Power Rule of logarithms to pull the exponents out.

x log 5 = 700 log 2

Then isolate x.

x = 700 ×

Now use your calculator to get decimal values for log 2 and log 5, and plug them into the equation.

x = 700 ×

x = 700 × .4307

x = 301.47

And voilà, a numerical value for x. This is the usual way in which logarithms will prove useful on the Math Subject Tests (especially the Math Level 2). Solving tough exponent equations will usually involve taking the common log of both sides of the equation, and using the Power Rule to bring exponents down. Another method can be used to find the values of logarithms with bases other than 10, even though logarithms with other bases aren”t programmed into your calculator. For example:

25. What is the value of x if log₃ 32 = x ?

You can”t do this one in your head. The logarithm is asking, “What exponent turns 3 into 32?” Obviously, it”s not an integer. You know that the answer will be between 3 and 4, because 3³ = 27 and 3⁴ = 81. That might be enough information to eliminate an answer choice or two, but it probably won”t be enough to pick one answer choice. Here”s how to get an exact answer:

x = log₃ 32

x = (Change of Base Formula)

x =

x = 3.1546

And there”s the exact value of x.

DRILL

In the following examples, use the techniques you”ve just seen to solve these exponential and logarithmic equations. The answers to these drills can be found in Chapter 12.

1. If 2₄ = 3^x, then x =

2. log₅ 18 =

3. If 10ⁿ = 137, then n =

4. log₁₂ 6 =

5. If 4^x = 5, then 4^x ^{+ 2} =

6. log₂ 50 =

7. If 3^x = 7, then 3^x ^{+ 1} =

8. If log₃ 12 = log₄ x, then x =

Natural Logarithms

On the Math Level 2 Subject Test, you may run into a special kind of logarithm called a natural logarithm. Natural logarithms are logs with a base of e, a constant that is approximately equal to 2.718.

The constant e is a little like π. It”s a decimal number that goes on forever without repeating itself, and, like π, it”s a basic feature of the universe. Just as π is the ratio of a circle”s circumference to its diameter, no matter what, e is a basic feature of growth and decay in economics, physics, and even in biology.

The role of e in the mathematics of growth and decay is a little complicated. Don”t worry about that, because you don”t need to know very much about e for the Math Level 2. Just memorize a few rules and you”re ready to go.

Natural logarithms are so useful in math and science that there”s a special notation for expressing them. The expression ln x (which is read as “ell-enn x”) means the log of x to the base e, or log_e x. That means that there are three different ways to express a natural logarithm.

Definitions of a Natural Logarithm

ln n = x ⇔ log_e n = x ⇔ e^x = n

You can use the definitions of a natural logarithm to solve equations that contain an e^x term. Since e equals 2.718281828…, there”s no easy way to raise it to a specific power. By rearranging the equation into a natural logarithm in “ln x” form, you can make your calculator do the hard work for you. Here”s a simple example:

19. If e^x = 6, then x =

(A) 0.45

(B) 0.56

(D) 1.79

(E) 2.56

Here”s How to Crack It

The equation in the question, e^x = 6, can be converted directly into a logarithmic equation using the definition of a logarithm. It would then be written as log_e 6 = x, or ln 6 = x. To find the value of x, just hit the “LN” key on your calculator and punch in 6. You”ll find that x = 1.791759. The correct answer is (D).

Calculator Tip

On some scientific calculators,
you”ll punch in 6 first,
and then hit the “ln x” key

For the Math Level 2 Subject Test, you may also have to know the shapes of some basic graphs associated with natural logs.

Here they are:

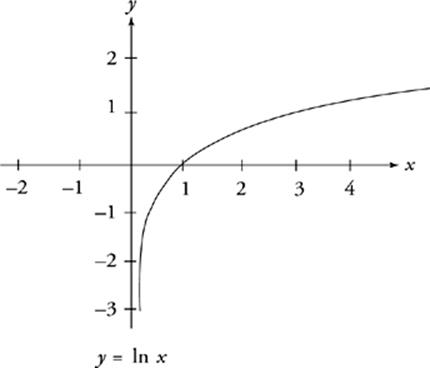
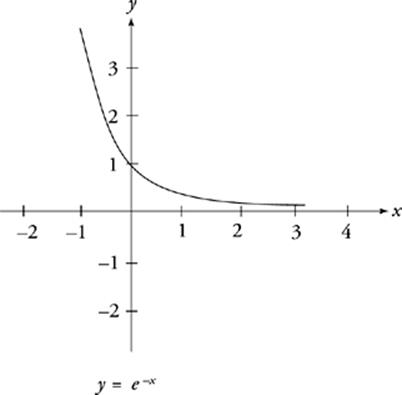
Finally, some questions may require you to estimate the value of e to answer a question. Just remember that e ≈ 2.718. If you forget the value of e, you can always get your calculator to give it to you. Just hit the “2nd” key followed by the “LN” key, and punch in 1. The result will be e to the first power, which is just plain e.

DRILL

The answers to these drills can be found in Chapter 12.

18. If e^z = 8, then ^z =

(A) 1.74

(B) 2.08

(D) 2.94

(E) 3.04

23. If set M = {π, e, 3}, then which of the following shows the elements in set M in descending order?

(A) {π, e, 3}

(B) {e, 3, π}

(D) {3, π, e}

(E) {3, e, π}

38. If 6 = 5, then what is the value of n ?

(A) −0.55

(B) −0.18

(D) 0.64

(E) 1.19

VISUAL PERCEPTION

Some questions on the Math Subject Tests ironically do not appear to test mathematical skills in any conventional sense of the phrase. One type of non-mathematical question on the test is the visual perception question, which asks you to visualize (that is, draw) a picture of a situation described in two or three dimensions.

The only technique for such questions is to draw your best representation of the situation described and use that as a guide in eliminating answers. You don”t have to be a great artist, but a simple diagram will go a long way.

24. Which of the following equations describes the set of points equidistant from the lines described by the equations y = 2x + 7 and y = 2x + 1 ?

(A) y = 4x + 8

(B) y = 4x + 6

(D) y = 2x + 6

(E) y = 2x + 4

This is asked in words because if there were a picture, it would be too easy. So make it easier by drawing a picture.

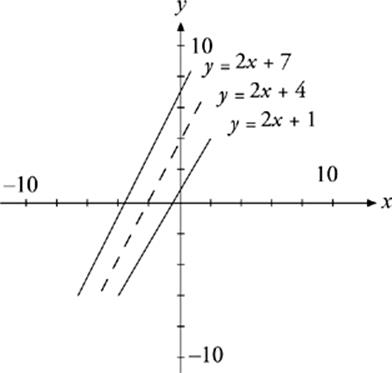
If you draw points halfway between the two lines, you get another line. It”s parallel to the other two (so its slope is 2, eliminate A and B), and it”s halfway between the two, so its y-intercept is halfway between 7 and 1. That”s a y-intercept of 4, so E is the answer.

DRILL

Try the following practice questions about visual perception. The answers to these drills can be found in Chapter 12.

27. Lines l, m, n, and o are all distinct lines which lie in the same plane. If line l is perpendicular to line m, line m is parallel to line o, and line o is perpendicular to line n, which of the following must be true?

I. Line l is parallel to line n.

II. Line n is perpendicular to line l.

III. Line n is parallel to line m.

(A) I only

(B) II only

(D) I and III only

(E) I, II, and III

42. Which of the following could be the number of circles created by the intersection of a sphere and a cube?

I. 5

II. 6

III. 7

(A) I only

(B) II only

(D) I and II only

(E) I and III only

ARITHMETIC AND GEOMETRIC SEQUENCES

The average Math Subject Test has one question dealing with arithmetic or geometric sequences. They”re very easy once you know how they work, so read the next few paragraphs and fear not.

Arithmetic Sequences

The big-forehead people at ETS define an arithmetic sequence as “one in which the difference between successive terms is constant.” Real human beings just say that an arithmetic sequence is what you get when you pick a starting value and add the same number again and again.

Here are some sample arithmetic sequences.

{a_n} = 1, 7, 13, 19, 25, 31,…

{b_n} = 3, 13, 23, 33, 43, 53,…

{c_n} = 12, 7, 2, −3, −8, −13,…

It”s not hard to figure out what difference separates any two terms in a sequence. To continue a sequence, you would just continue adding that difference. The larger letter in each case is the name of the sequence (these are sequences a, b, and c). The subscript, n, represents the number of the term in the sequence. The expression a₄, for example, represents the fourth term in the a sequence, which is 19. The expression b₇ means the seventh term in the b sequence, which would be 63.

The typical arithmetic sequence question asks you to figure out the difference between any two successive terms in the sequence, and then calculate the value of a term much farther along. There”s just one trick to that—to calculate the value of a₂₆, for example, start by figuring out the difference between any two consecutive terms. You”ll find that the terms in the a sequence increase at intervals of 6. Now here”s the trick: To get to the 26th term in the sequence, you”ll start with a₁, which is 1, and increase it by 6 twenty-five times. The term a₂₆ = 1 + (25 × 6), or 151. It”s like climbing stairs in a building; to get to the fifth floor, you climb 4 flights. To get to the 12th floor, you climb 11 flights, and so on. In the same way, it takes 11 steps to get to the 12th term in a sequence from the first term. To get to the nth term in a sequence, take (n − 1) steps from the first term.

Here”s another example—to figure out the value of c₁₇, start with 12 and add −5 sixteen times. The value of c₁₇ = 12 + (16 × −5), or −68. That”s all there is to calculating values in arithmetic sequences.

Here”s the algebraic definition of the nth term of an arithmetic sequence, if the starting value is a₁ and the difference between any two successive terms is d.

The nth Term of an Arithmetic Sequence

a_n = a₁ + (n − 1)d

Finding the Sum of an Arithmetic Sequence

You might be asked to figure out the sum of the first 37 terms of an arithmetic sequence, or the first 48 terms, and so on. To figure out the sum of a chunk of an arithmetic sequence, take the average of the first and last terms in that chunk, and multiply by the number of terms you”re adding up. For example,

{a_n} = 5, 11, 17, 23, 29, 35,…

What is the sum of the first 40 terms of a_n ?

The first term of a_n is 5. The fortieth term is 239. The sum of these terms will be the average of these two terms, 122, multiplied by the number of terms, 40. The product of 122 and 40 is 4,880. That”s the sum of the first 40 terms of the sequence. Here”s the algebraic definition of the sum of the first n terms of an arithmetic sequence, where the difference between any two successive terms is d.

Sum of the First n Terms of an Arithmetic Sequence

sum =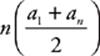

Summations

A summation (or series) is a list of numbers to be added together. First, plug the number below the sigma (Σ) into the formula and get a result. Then do this for every integer up to the number above the sigma. Finally, add up all of your results to get your final answer.

43. 2k + 1

(A) 20

(B) 36

(D) 72

(E) 80

Here”s How to Crack It

Here, plug k = 1 into the formula to get 2(1) + 1 = 3. Now, repeat for k = 2, k = 3, etc., up to and including k = 8. You end up with 3 + 5 + … + 17. You could also use the formula for the sum of the first n terms of an arithmetic sequence, and you”d get 8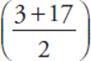, which is 80. The answer is (E).

Geometric Sequences

A geometric sequence is formed by taking a starting value and multiplying it by the same factor again and again. While any two successive terms in an arithmetic sequence are separated by a constant difference, any two successive terms in a geometric sequence are separated by a constant factor. Here are some sample geometric sequences.

{a_n} = 2, 6, 18, 54, 162, 486,…

{b_n} = 8, 4, 2, 1, 0.5, 0.25,…

{c_n} = 3, 15, 75, 375, 1,875,…

Just like arithmetic sequence questions, geometric sequence questions most often test your ability to calculate the value of a term farther along in the sequence. As with arithmetic sequences, the trick to geometric sequences is that it takes 19 steps to get to the 20th term, 36 steps to get to the 37th term, and so on.

To find the value of a₁₀, for example, start with the basic information about the sequence. Its starting value is 2, and each term increases by a factor of 3. To get to the tenth term, start with 2 and multiply it by 3 nine times—that is, multiply 2 by 3⁹. You get 39,366, which is the value of a₁₀. As you can see, geometric sequences tend to grow much faster than arithmetic sequences do.

Here”s the algebraic definition of the nth term in a geometric sequence, where the first term is a₁ and the factor separating any two successive terms is r.

The nth Term of a Geometric Sequence

a_n = a₁rⁿ ^{− 1}

The Sum of a Geometric Sequence

You may also be asked to find the sum of part of a geometric sequence. This is a bit tougher than calculating the sum of an arithmetic sequence. To add the first n terms of a geometric sequence, use this formula. Once again, the first term in the sequence is a₁, and the factor separating any two successive terms is r.

Sum of the First n Terms of a Geometric Sequence

sum =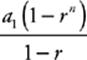

This is not a formula that is called upon very often, but it”s good to know it if you”re taking the Math Level 2.

The Sum of an Infinite Geometric Sequence

Every now and then, a question will ask you to figure out the sum of an infinite geometric sequence—that”s right, add up an infinite number of terms. There”s a trick to this as well. Whenever the factor between any two terms is greater than 1, the sequence keeps growing and growing. The sum of such a sequence is infinitely large—it never stops increasing, and its sum cannot be calculated.

The sum of an infinite geometric series can be calculated only when the constant factor is between −1 and 1.

When the constant factor of a geometric sequence is less than 1, the terms in the sequence continually decrease, and there exists some value that the sum of the sequence will never exceed. For example:

{a_n} = 1, 0.5, 0.25, 0.125, 0.0625,…

The sequence a_n above will never be greater than 2. The more of its terms you add together, the closer the sum gets to 2. If you add all of its terms, all the way out to infinity, you get exactly 2. Here”s the formula you use to figure that out. Once again, a₁ is the first term in the sequence, and rthe factor between each two terms. Remember that r must be between −1 and 1.

Sum of an Infinite Geometric Sequence

sum = for −1 > r >

In most cases, though, you can simply use approximation to eliminate ridiculously large or small answer choices. The five formulas in the boxes are all you”ll ever need to work with arithmetic and geometric sequences on the Math Subject Tests.

DRILL

Try the following practice questions about arithmetic and geometric sequences. The answers to these drills can be found in Chapter 12.

14. In an arithmetic sequence, the second term is 4 and the sixth term is 32. What is the fifth term in the sequence?

(A) 8

(B) 15

(D) 24

(E) 25

19. In the arithmetic sequence a_n, a₁ = 2 and a₇ = 16. What is the value of a₃₃ ?

(A) 72.00

(B) 74.33

(D) 75.14

(E) 76.67

26. If the second term of a geometric sequence is 4, and the fourth term of the sequence is 25, then what is the ninth term in the sequence?

(A) 804.43

(B) 976.56

(D) 2441.41

(E) 6103.52

34. 3 + 1 + + + + … =

(A) 4.17

(B) 4.33

(D) 5.00

(E) ∞

LIMITS

A limit is the value a function approaches as its independent variable approaches a given constant. That may be confusing to read, but the idea is really fairly simple. A limit can be written in different ways, as the following examples show:

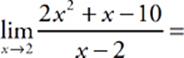
What is the limit of as x approaches 2?

If f(x) = , then what value does f(x) approach as x approaches 2?

These three questions are equivalent. The first of the three is in limit notation and is read exactly like the question, “What is the limit of as x approaches 2?”

Finding a limit is very simple. Just take the value that x approaches and plug it into the expression. The value you get is the limit. It”s so simple that you just know there”s got to be a hitch—and there is. The limits that appear on the Math Subject Tests share a common problem—tricky denominators. The question introduced above is no exception. Let”s take a look at it again.

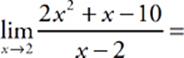
You can find the value of this limit just by plugging 2 into the expression as x. But there”s a hitch. When x = 2, the fraction”s denominator is undefined, and it seems that the limit does not exist. The same solution always applies to such questions. You need to factor the top and bottom of the fraction and see whether there”s anything that will make the denominator cancel out and stop being such a nuisance. Let”s see how this expression factors out.

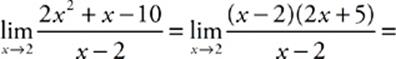
Now, you can cancel out that pesky (x − 2).

(2x + 5)

Now the expression is no longer undefined when you Plug In 2. It simply comes out to 2(2) + 5, or 9. The limit of 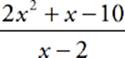as x approaches 2 is 9.

That”s all there is to limit questions. Just factor the top and bottom of the expression as much as possible, and try to get the problematic terms to cancel out so that the limit is no longer undefined. When it”s no longer undefined, just Plug In the constant value to find the limit.

One more dirty trick—you might run into a limit problem in which it”s impossible to cancel out the term that makes the expression undefined. Take a look at this example:

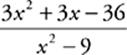
Because the constant that x approaches, −3, makes the limit undefined, you”ve got to factor the expression and try to cancel out the problematic part of the denominator.

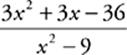
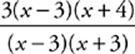
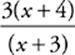
The expression can be factored, and you can even cancel out a term in the denominator. When the dust clears, however, you find that the denominator of the fraction still approaches zero, and that the limit remains undefined. When this happens, it”s said that the limit does not exist, and that would be the correct answer.

DRILL

Try the following practice questions involving limits. The answers to these drills can be found in Chapter 12.

30. What value does the expression 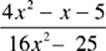approach as x approaches 1.25 ?

(A) 0

(B) 0.225

(D) 1.275

(E) 2.250

38.

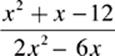
(A) 1.17

(B) 2.25

(D) 6.67

(E) The limit does not exist.

40.

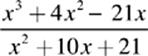
(A) −3.00

(B) 2.46

(D) 10.33

(E) The limit does not exist.

VECTORS

A vector is a visual representation of something that has both direction and magnitude. A vector can represent a force, a velocity, a distance traveled, or any of a variety of physical quantities. On the Math Subject Tests, vectors usually represent travel.

A vector arrow”s orientation indicates the direction of travel. Its length represents the distance traveled (this is the magnitude of the vector). Sometimes, test questions will deal with vectors without telling you what they represent.

Basically, there are only two things you have to do with vectors on the Math Subject Tests—compute their lengths, and add or subtract them. Computing their lengths is generally done on the coordinate plane, where it”s just a matter of using the Pythagorean theorem. Adding and subtracting vectors is also pretty simple. Here”s how it”s done.

Adding Vectors

Suppose you wanted to add these two vectors together:

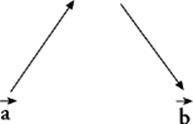
To add them, redraw the second vector so that its tail stands on the tip of the first vector. Then draw the resulting vector, closing the triangle (make sure that the resulting vector”s direction is in agreement with the vectors you added). This is what the addition of vectors a and b looks like.

Vector c is the sum of vectors a and b.

If ETS gives you a figure and asks you to add two vectors, they need to be connected tip-to-tail. If necessary, move one of the vectors, and then try using the Law of Cosines.

Subtracting Vectors

To subtract vectors, you”ll use the same technique you used to add them, with one extra step. First, reverse the sign of the vector that”s being subtracted. You do this by simply moving the arrowhead to the other end of the vector. Then add the two vectors as you usually would. Here”s an example of subtraction using the two vectors you just added. First, reverse the sign of the subtracted vector:

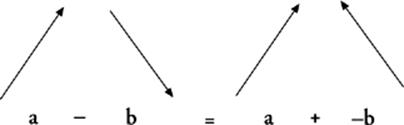
And then, add them up:

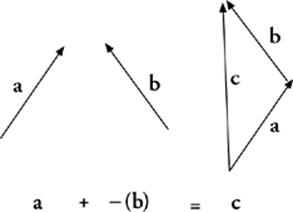
Tip to Tail

Remember, you”re always
going to connect vectors
tip to tail.

Vector c is the vector produced by subtracting vector b from vector a.

You can add or subtract two vectors by adding or subtracting their x and y components. For example, if vector u has components (1, 3) and vector v has components (−1, 5), then the resulting vector u + v would have components (1 + (−1), 3 + 5) = (0, 8).

DRILL

The answers to these drills can be found in Chapter 12.

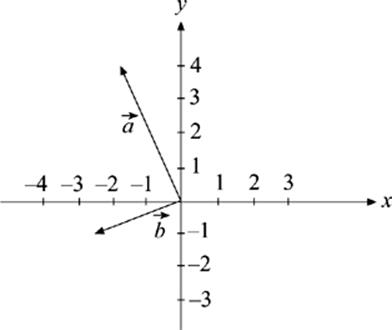
36. If = + , then what is the magnitude of ?

(A) 2

(B) 3

(D) 5

(E) 6

41. Vector a has components (8, 15), and vector b has components (3, 3). If c = a − b, what is the magnitude of vector c ?

(A) 10.5

(B) 13.0

(D) 16.5

(E) 21.1

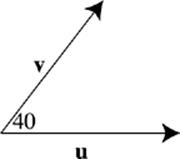
44. If, in the figure above, the magnitude of vector u is 9 and the magnitude of vector v is 7, what is the magnitude of vector (u + v) ?

(A) 5.79

(B) 7.00

(D) 12.26

(E) 15.05

LOGIC

Every now and then, as you proceed innocently through a Math Subject Test, you will come upon a question asked in simple English that seems to have nothing at all to do with math. This is a logic question. Here”s a typical example.

24. If every precious stone is harder than glass, which of the following statements must also be true?

(A) Glass can be a precious stone.

(B) Every stone harder than glass is a precious stone.

(D) Some stones softer than glass are precious stones.

(E) Every stone softer than glass is not a precious stone.

Here”s How to Crack It

This is madness. There”s no math here at all. However, there is a rule here for you to work with. The rule states that given one statement, there”s only one other statement that is logically necessary, the contrapositive. This is what the contrapositive states:

The Contrapositive

Given the statement A → B, you also know ∼B → ∼A.

In English, that means that the statement “If A, then B” also tells you that “If not B, then not A.” To find the contrapositive of any statement, switch the order of the first and second parts of the original statement, and negate their meaning. But you can”t be sure of anything else. For example, “If not A” doesn”t necessarily mean “then not B.” And “if B” doesn”t necessarily mean “then A.” This is how you”d find the contrapositive of the statement, “Every precious stone is harder than glass.” Start by making sure that you clearly see what the two parts of the original statement are.

stone is precious → stone harder than glass

Then switch the order of the statement”s parts, and negate their meanings:

stone not harder than glass → stone is not precious

This is the contrapositive. Once you”ve found it, just check the answer choices for a statement with an equivalent meaning. In this case, answer choice (E) is equivalent to the contrapositive. Joe Bloggs answers will typically say things like “Every stone that is harder than glass is precious” or “Every stone that is not precious is softer than glass.”

Almost all logic questions test your understanding of the contrapositive. There are just a couple of other points that might come up in logic questions.

• If you see the statement “Some A are B,” then you also know that “Some B are A.” For example, “Some teachers are pretty cool people” also means that “Some pretty cool people are teachers.”

• To disprove the claim, “X might be true,” or “X is possible,” you must show that X is never, ever true, in any case, anywhere.

• To disprove the claim, “X is true,” you only need to show that there”s one exception, somewhere, sometime.

In other words, a statement that something may be true is very hard to disprove; you”ve got to demonstrate conclusively that there”s no way it could be true. On the other hand, a statement that something is definitely true is easy to disprove; all you have to do is find one exception. If you remember the three bullet points above and the contrapositive, you”ll be prepared for any logic question on the Math Subject Tests.

DRILL

Exercise your powers of logic on these practice questions. The answers to these drills can be found in Chapter 12.

28. At Legion High School in a certain year, no sophomore received failing grades. Which of the following statements must be true?

(A) There were failures in classes other than the sophomore class.

(B) Sophomores had better study skills than other students that year.

(D) Any student who received failing grades was not a sophomore.

(E) There were more passing grades in the sophomore class than in other classes.

33. “If one commits arson, a building burns.” Which of the following is a contradiction to this statement?

(A) Many people would refuse to commit arson.

(B) A building did not burn, and yet arson was committed.

(D) A building burned, although no arson was committed.

(E) Arson is a serious crime.

35. In a necklace of diamonds and rubies, some stones are not genuine. If every stone that is not genuine is a ruby, which of the following statements must be true?

(A) There are more diamonds than rubies in the necklace.

(B) The necklace contains no genuine rubies.

(D) Diamonds are of greater value than rubies.

(E) The necklace contains no genuine diamonds.

IMAGINARY NUMBERS

Almost all math on the Math Subject Tests is confined to real numbers. Only a few questions deal with the square roots of negative numbers—imaginary numbers. For the sake of simplicity, imaginary numbers are expressed in terms of i. The quantity i is equal to the square root of −1. It”s used to simplify the square roots of negative numbers. On the Math Level 1 Subject Test, ETS will remind you of this in the question by saying “If i = ” or “If i² = 1.” On the Math Level 2 Subject Test, they won”t bother. For example, here”s how i can be used to simplify square roots of negative numbers.

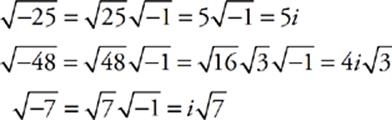
There are three basic kinds of questions on the Math Subject Tests that require you to work with imaginary numbers.

Computing Powers of i

You may run into a question that asks you to find the value of i³⁴, or something equally outrageous. This may seem difficult or impossible at first, but, as usual, there”s a trick to it. The powers of i repeat in a cycle of 4 values, over and over.

i¹ = i	i⁵ = i
i² = −1	i⁶ = −1
i³ = −i	i⁷ = −i
i⁴ = 1	i⁸ = 1

And so on. These are the only four values that can be produced by raising i to an integer power. To find the value of i³⁴, either write out the cycle of four values up to the 34th power, which would take less than a minute, or, more simply, divide 34 by 4. You find that 34 contains eight cycles of 4, with a remainder of 2. The eight cycles of 4 just bring you back to where you started. It”s the remainder that”s important. The remainder of 2 means that the value of i³⁴ is equal to the value of i², or −1. In order to raise i to any power, just divide the exponent by 4 and use the remainder as your exponent.

Doing Algebra with i

Algebra that includes complex numbers is no different from ordinary algebra. You just need to remember that i raised to an exponent changes in value, which can have some odd effects in algebra.

Here”s an example.

(x − 3i)(2x + 6i) =

2x² − 6ix + 6ix + 18i ² =

2x² − 18i ² =

2x² − 18(−1) =

2x² + 18

An ETS Trick

As you can see, i sometimes
has a way of
dropping out of algebraic
expressions. ETS likes
this trick, so keep an eye out
for it.

The Complex Plane

A complex number is a specific kind of imaginary number—specifically, the sum of a real number and an imaginary number, such as 5 + 3i. A complex number is one that takes the form a + bi, where a and b are real numbers and i is the imaginary unit, the square root of −1. On the Math Level 2 Subject Test, the principal importance of complex numbers is that they can be represented on the complex plane. This is what the complex plane looks like.

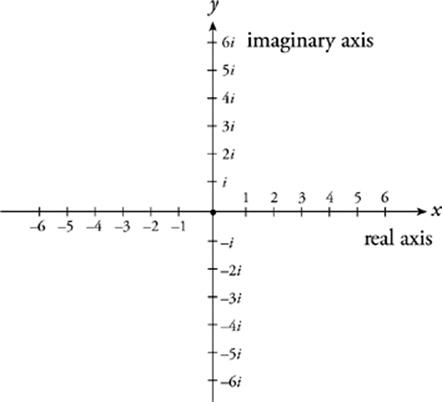
Notice that the complex plane looks just like the ordinary coordinate plane, but the axes have different meanings. On the complex plane, the x-axis is referred to as the real axis. The y-axis is referred to as the imaginary axis. Each unit on the x-axis equals 1—a real unit. Each unit on the imaginary axis equals i—the imaginary unit. Any complex number in the form a + bi, such as 5 + 3i, can be plotted on the complex plane almost like a coordinate pair. Just plot a, the real component of the complex number, on the x-axis; and bi, the imaginary component, on the y-axis.

Here are several complex numbers plotted on the complex plane.

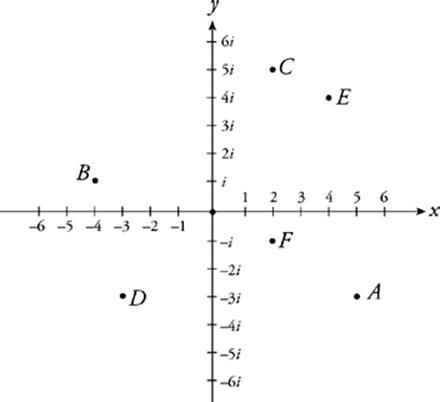
A = 5− 3i C = 2 + 5i E = 4 + 4i

B = −4 + i D = −3 − 3i F = 2 − i

Once you”ve plotted a complex number on the complex plane, you can use all of the usual coordinate-geometry techniques on it, including the Pythagorean theorem and even right-triangle trigonometry. The most common complex-plane question asks you to find the distance between a complex number and the origin, using the Pythagorean theorem. This distance is most often referred to as the magnitude or absolute value of a complex number. If you”re asked to compute |4 + 3i|, just plot the number on the complex plane and use the Pythagorean theorem to find its distance from the origin. This distance is the absolute value of the complex number.

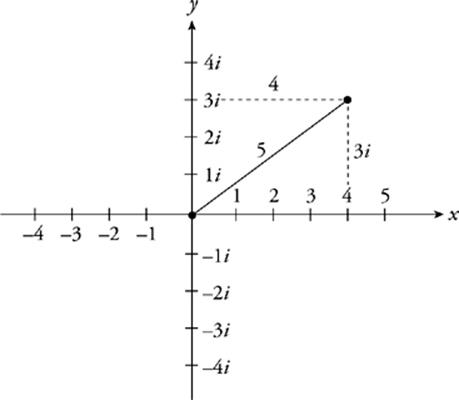
The Pythagorean theorem will quickly show you that |4 + 3i| = 5.

DRILL

Test your understanding of imaginary numbers with the following practice questions. The answers to these drills can be found in Chapter 12.

25. If i² = −1, then what is the value of i⁵¹ ?

(A) 0

(B) −1

(D) i

(E) 1

36. If i² = −1, then which of the following expressions is NOT equal to zero?

(A) i⁰ − i¹²

(B) i + i³

(D) i¹¹ − i⁹

(E) i⁸ − i¹²

40.

(A) 2.2

(B) 4.0

(D) 5.0

(E) 8.4

43. |5 − 12i| =

(A) 7i

(B) 7

(D) 13

(E) 13i

POLYNOMIAL DIVISION

Most of the factoring questions on the Math Subject Tests are very traditional, using only the tools reviewed in Chapter 4. You will rarely need anything more advanced than the reverse FOIL technique for quadratics. On the Math Level 2 Subject Test, however, you may run into a question that requires you to factor a polynomial of a higher degree than a quadratic. You could use polynomial division, a messy algebraic process. But since there are variables in the answer choices of these questions, it”s much easier to Plug In. See the following for typical questions of this type.

21. If x³ + x² − 7x + 20 = (x + 4) • f(x), where f(x) is a polynomial in x, then f(x) =

(A) x + 20

(B) x² + 5

(D) x² − 3x + 5

(E) x² − 7x + 20

Here”s How to Crack It

To figure out f(x), you must divide x³ + x² − 7x + 20 by x + 4. That”s polynomial division. Polynomial division is actually just like ordinary division. You set it up like this:

Now, just Plug In a number for x. Let”s pick x = 2. Now, we”re just dividing 18 by 6, which gives us 3, with no remainder. So our target answer is 3. Plug In 2 for x in the answers to see which one equals 3. It”s (D).

30. What is the remainder when x⁴ − 5x² + 12x + 18 is divided by (x + 1) ?

(A) x² − 1

(B) x − 6

(D) 3

(E) 2

Here”s How to Crack It

Once again, just Plug In x = 2. Now the question is asking for the remainder when 38 is divided by 3. The remainder is 2, our target answer. So the answer is (E). That”s all there is to polynomial division. As we mentioned in Chapter 4, don”t Plug In 0 or 1. When you Plug In on polynomial division questions that ask for a remainder, you”ll find that bigger numbers, such as 10, are better. If you Plug In and something weird happens, Plug In a different number.

DRILL

Try your talents on these practice questions. The answers to these drills can be found in Chapter 12.

21. If x⁴ − 5x³ − 2x² + 24x = g(x) • (x + 2), then which of the following is g(x) ?

(A) x + 12

(B) x² + 3x − 18

(D) x³ + 10x² + 6x

(E) x⁴ − 3x³ + 2x² − 6

27. What is the remainder when x³ + 2x² − 27x + 40 is divided by (x − 3) ?

(A) 4

(B) 16

(D) x² − 5

(E) x² + 5x − 12

WHAT IS THE MATRIX?

If you”ve never seen matrices before, don”t worry; there”s a good chance that you won”t even see one on your Math Level 2. But don”t say we didn”t warn you.

The determinant of the 2 × 2 matrix 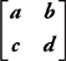is ad − bc.

The determinant of a matrix is sometimes indicated by plain vertical bars around the elements, like a big absolute value symbol. The folks at ETS may simply write 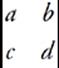if they want you to find the determinant of the matrix above.

A Clue

A good way to remember
the determinant of a
2 × 2 matrix is that you
multiply the diagonals and
subtract.

The determinant of the 3 × 3 matrix

is aei + bfg + cdh − bdi − afh − ceg.

If you take the first two columns of the matrix and recopy them to the right of the original matrix, the parts of the formula form diagonal lines of three elements, with the positive parts going from the upper left to the bottom right, and the negative parts going from the upper right down to the bottom left, like this:

A What by What?

When you”re looking at
a particular matrix, for
example, a 3 × 2 matrix,
remember that this describes
the matrix as row
by column. So there are 3
rows and 2 columns.

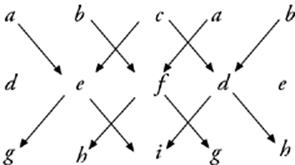
Right now you may be feeling a bit like Alice, tumbling down the rabbit hole. Just remember a few other facts about matrices:

• You can only multiply matrices if the first matrix has the same number of columns as the second matrix has rows. And when you multiply an m × n matrix by an n × p matrix, you get an m × p matrix.

• When you are shown simultaneous equations, the coefficient matrix refers to the matrix formed by the coefficients of the variables (which are on the left side of the equals signs).

DRILL

If you feel ready for the matrix, try these examples. The answers to these drills can be found in Chapter 12.

30. If matrix X has dimension 3 × 2, matrix Y has dimension 2 × 5, and XY = Z, then matrix Z must have dimension

(A) 2 × 2

(B) 2 × 5

(D) 3 × 5

(E) 6 × 10

40. If A = 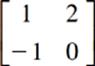, then what is the determinant of A ?

(A) −2

(B) −1

(D) 1

(E) 2

45. If = X, then |X| =

(A) −2

(B) 0

(D) 2

(E) 3

2x + 3y − z = 12

x − 3y + 2z = −5

x + z = 3

Use Technology!

A graphing calculator can
find the determinant of a
3 × 3 matrix a lot faster
than you can. Check your
owner s manual for advice.

46. What is the determinant of the coefficient matrix of the system of equations shown above?

(A) −6

(B) 0

(D) 3

(E) 10

Summary

· A logarithm is just another way to write exponents, so make sure you”re up to speed on your exponent rules.

· The concepts in this chapter pop up rarely on the SAT Math Subject Tests but are still worth knowing if you want a top score.

· An arithmetic sequence is created by adding the same number to the previous member in the sequence.

· The nth term of an arithmetic sequence can be found with the formula a_n = a₁ + (n − 1)d.

· The sum of the first n terms of an arithmetic sequence can be found with the formula .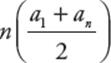

· The only thing you need to know for logic questions is the contrapositive. If the initial statement is “If A, then B,” then the contrapositive states “If not B, then not A.”

· There are three types of questions that ETS will ask about imaginary numbers:

· ETS will ask questions that use the definition of i, which is .

· The powers of i create a pattern: i, −1, −i, 1. It then repeats. So find the closest multiple of 4 to the power in your question and just count forward or back until you get to your number.

· You may have to use FOIL on a question with imaginary numbers. Treat it like a regular quadratic, and then simplify i as your last step.

· Here are some Level 2–only concepts:

· A summation is a list of numbers to be added together. You”ll recognize it because of the sigma (Σ). Put the number below the sigma into the equation given. Find the result of that and every following integer up to the number above the sigma. Then add your results.

· A geometric sequence is created by taking an initial value and multiplying it by the same number again and again.

· The nth term of a geometric sequence can be found with this formula: a_n = a₁rⁿ^-1.

· The sum of the first n terms of a geometric sequence can be found with this formula: .

· The sum of an infinite geometric sequence is . This can be found only if −1 < r < 1. If r is bigger than 1, there is no sum, because the sequence never converges.

· A limit is the value a function approaches as its independent variable approaches a given constant.

· A vector contains direction and magnitude and is represented by a line with an arrow. When adding or subtracting vectors, make sure you”re connecting tip to tail. When subtracting vectors, add the opposite vector instead.

· A complex number is made up of a real number and an imaginary number. The complex plane is a coordinate plane in which the y-axis is imaginary numbers and the x-axis is real numbers.

· The best and fastest way to conquer a polynomial division question is to Plug In.

· Matrix questions appear in the form of determinant questions. They pop up rarely, but make sure you know your determinant formulas or how to work with matrices on your calculator.