Homework Helpers: Physics

4 Rotational and Circular Motion

Do you realize that you probably conducted physics experiments in the park before you were old enough to go to school? Your experiences with seesaws, merry-go-rounds, and swings offered early opportunities to learn the principles of physics involved in rotational and circular motion. Years later, even if you no longer visit parks, you are still surrounded by examples of objects that exhibit these principles. From swinging doors to spinning CDs, we are surrounded by examples of rotational and circular motion. In this chapter we will explore the terms and formulas related to these types of motion.

Rotational motion is the motion of an object around an axis. This differs from translational motion, which we have been studying up until now, in that a rotating object may be in motion around an axis without showing an overall displacement with reference to a nearby object. A hockey puck sliding across a patch of ice shows translational motion. If the puck is also spinning, it has rotational motion as well.

A CD spinning in a stationary CD player shows rotational motion, but not translational motion, at least with reference to other objects in the room.

Many children conduct informal experiments involving rotational and circular motion at the park while riding on the merry-go-round. Whether riding on the type of merry-go-round found at many parks, which you have to push, or the carousel with horses found at amusement parks, you might recall that you seemed to travel faster when you sat near the outer rim of the circle. Just a few days before writing this lesson, I saw my son and daughter riding a merry-go-round at the park. My daughter, who is younger, sat near the center and had no trouble staying on. My son sat near the outer edge, where he found it difficult to stay on. He needed to hold on tight to avoid being hurled from the ride because he was actually moving faster than his sister, even though she was on the same ride!

Looking back at your own experience, does it seem odd to you that you can have two people on the same merry-go-round, which is rotating at a certain speed, and yet the two people can be traveling at different speeds? To explain this, let”s start off by making sure that certain definitions are clear.

When we talk about the motion of the merry-go-round itself, we are talking about rotational motion. The merry-go-round experiences rotational motion, as it rotates about its axis of rotation, or pivot point. In this way, it is similar to the seesaw, only with a different orientation. The horizontal orientation of a seesaw only allows for a limited range of rotation, because one end or the other will eventually hit the ground. The vertical orientation allows the merry-go-round to rotate completely around or, as some might say, 360°.

You are probably accustomed to describing circles or angles in terms of degrees, but in this chapter we will talk about another common unit called radians (rad). Just as a pizza can be cut into six, eight, or even 37 slices, a circle can be divided into any number of pieces. We usually break up a circle into 360 pieces, and call them degrees. We could also break the same circle into 6.28 pieces and call them radians.

360° = 6.28 rad
1 rad = 57.3° (approximately)

It may seem odd to break a circle up into an uneven number of pieces. Why are there 6.28 radians per circle? Did you notice that 6.28 is twice 3.14, the standard value of pi to three digits? Is there a connection between pi and radians beyond the fact that they are both concerned with circles?

A radian is defined as the measure of an angle whose arc length (s) is equal to its radius (r). When a circular object, such as a merry-go-round, has rotated through an angle where the arc length of its path is equal to its radius, we say that it has rotated through an angle (θ) equal to one radian, or that it has an angular displacement of 1 rad.

We use radians to measure the angular displacement of an object that experiences rotational motion.

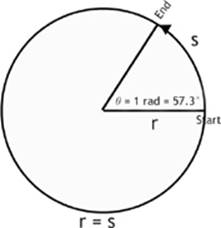
Figure 4.1

The change of position of a rotating body as measured by the angle through which it rotates.

or

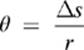
Example 1

Find the angular displacement in radians of a bug sitting 3.5 cm from the center of a rotating CD as it traces out an arc length of 8.3 cm.

Given: r = 3.5 cm Δs = 8.3 cm

Find: θ

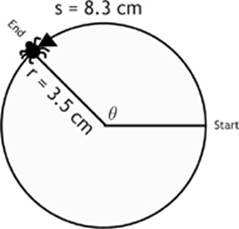
Figure 4.2

Now, suppose a merry-go-round rotates completely around in what we might call a 360° circle. The arc length (s) it traces out would be equal to the diameter of the entire circle. The formula for the diameter of a circle is 2πr. Let”s see what happens when we put that value in for the arc length (s) in our angular displacement formula.

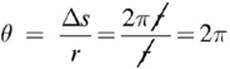
So, an angular displacement of 360° represents 2π rad, or 6.28 rad, and that is why there are 6.28 rad in every circle.

If you were asked to describe the speed of the merry-go-round, you would probably describe it in terms of its angular speed. The angular velocity of an object is the rate at which it rotates around its axis in a particular direction. This quantity, which is usually measured in radians per second (rad/s), can be determined by the following formula.

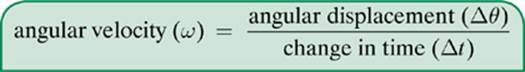
or

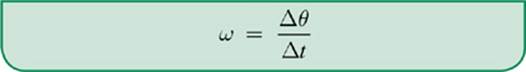
Most sources will consider an object rotating clockwise to have a negative angular velocity. When the direction of the rotation is not considered, then the quantity may be referred to as angular speed.

Example 2

As a merry-go-round rotates in a clockwise direction around its axis, it undergoes an angular displacement of –9.80 radians in 2.0 seconds. What is the angular velocity of the merry-go-round?

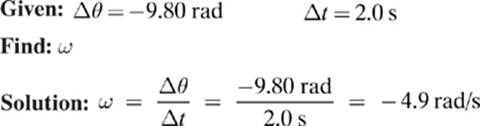
The negative sign in our answer indicates that the angular velocity is in the clockwise direction. To help you visualize how fast this is, you could always convert radians to degrees 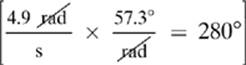and see that the merry-go-round is completing a little bit less than 80 percent of a rotation every second.

Two other terms that we should discuss at this point are period and frequency. Period (T) is the amount of time it takes to complete one cycle of motion. The term period, as it relates to rotational and/or circular motions, is the amount of time it takes for an object to complete a full rotation or revolution, usually measured in seconds. If it takes a merry-go-round 3.4 seconds to go around one time, it has a period of 3.4 s. Frequency is the inverse of period. Frequency (f) is the number of cycles of motion that take place each second. The term frequency, as it relates to rotational and/or circular motions, is the number of rotations or revolutions completed in one second. Frequency is sometimes described with the units cycles/second, rotations/second, or revolutions/seconds, but it is most commonly shown with the generic unit called hertz (Hz).

Example 3

A wagon wheel has an angular velocity of 7.50 rad/s. Calculate the period and frequency of the wheel.

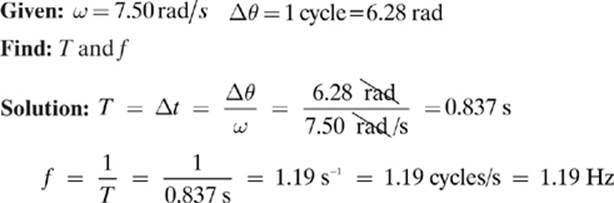
Look at the answers to Example 3 and see if you understand them. The wheel has an angular velocity of 7.50 rad/s. A complete rotation represents 6.28 rad, so this wheel is rotating around completely (period) every 0.837 s. It completes almost 1.2 rotations (frequency) every second. Notice that you could have solved for frequency first by dividing the angular velocity by the number of radians in one cycle or rotation.

Then, we would find the period by taking the inverse of the frequency.

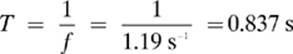
Now try the following review problems.

Lesson 4–1 Review

1. _______________is the rate at which a body rotates in a particular direction.

2. Convert 5.81 rad to degrees.

3. Find the angular velocity of a bicycle tire that undergoes an angular displacement of 55.7 rad in 11.5 s.