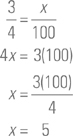Advanced Arithmetic - How to Crack the Math Section - Cracking the SAT test

Cracking the SAT

Part III

How to Crack the Math Section

Chapter 13

Advanced Arithmetic

Now that we have reviewed some mathematical fundamentals and some algebra, it is time to jump into our review of the more advanced arithmetic concepts you will find on the SAT. Many math questions test concepts you learned in junior high school, such as averages and proportions. Some difficult questions build on these basic concepts and require you to combine multiple techniques. In this chapter we will brush off the cobwebs and review the advanced arithmetic you’ll need to know for the SAT.

Many students get extremely nervous when they are asked to work with ratios. But there’s no need to be nervous. A ratio is a comparison between the quantities of ingredients you have in a mixture, be it a class full of people or a bowl of cake batter. Ratios can be written to look like fractions—don’t get them confused.

The ratio of x to y can be expressed in the following three ways:

1. 

2. the ratio of x to y

3. x:y

Ratios are a lot like fractions. In fact, anything you can do to a fraction (convert it to a decimal or percentage, reduce it, and so on), you can do to a ratio. The difference is that a fraction gives you a part (the top number) over a whole (the bottom number), while a ratio typically gives you two parts (boys to girls, CDs to cassettes, sugar to flour), and it is your job to come up with the whole. For example, if there is one cup of sugar for every two cups of flour in a recipe, that’s three cups of stuff. The ratio of sugar to flour is 1:2. Add the parts to get the whole.

Ratios vs. Fractions

Keep in mind that a ratio
compares part of
something to another part.
A fraction compares
part of something to the
whole thing.

Ratio:

Fraction:

If a class contains 3 students and the ratio of boys to girls in that class is 2:1, how many boys and how many girls are there in the class? Of course: There are 2 boys and 1 girl.

Now, suppose a class contains 24 students and the ratio of boys to girls is still 2:1. How many boys and how many girls are there in the class? This is a little harder, but the answer is easy to find if you think about it. There are 16 boys and 8 girls.

How did we get the answer? We added up the number of “parts” in the ratio (2 parts boys plus 1 part girls, or 3 parts all together) and divided it into the total number of students. In other words, we divided 24 by 3. This told us that the class contained 3 equal parts of 8 students each. From the given ratio (2:1), we knew that two of these parts consisted of boys and one of them consisted of girls.

An easy way to keep track of all this is to use a tool we call the Ratio Box. Every time you have a ratio problem, set up a Ratio Box with the information provided in the problem and use it to find ETS’s answer.

Here’s how it works:

Let’s go back to our class containing 24 students, in which the ratio of boys to girls is 2:1. Quickly sketch a table that has columns and rows, like this:

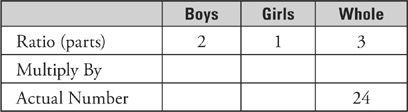
This is the information you have been given. The ratio is 2:1, so you have 2 parts boys and 1 part girls, for a total of 3 parts. You also know that the actual number of students in the whole class is 24. You start by writing these numbers in the proper spaces in your box.

Your goal is to fill in the two empty spaces in the bottom row. To do that, you will multiply each number in the parts row by the same number. To find that number, look in the last column. What number would you multiply by 3 to get 24? You should see easily that you would multiply by 8. Therefore, write an 8 in all three blanks in the Multiply By row. (The spaces in this row will always contain the same number, although of course it won’t always be an 8.) Here’s what your Ratio Box should look like now:

The next step is to fill in the empty spaces in the bottom row. You do that the same way you did in the last column, by multiplying. First, multiply the numbers in the boys column (2 × 8 = 16). Then multiply the numbers in the girls column (1 × 8 = 8).

Here’s what your box should look like now:

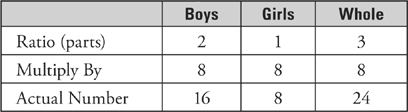
Now you have enough information to answer any question that ETS might ask you. Here are some examples:

· What is the ratio of boys to girls? You can see easily from the ratio (parts) row of the box that the ratio is 2:1.

· What is the ratio of girls to boys? You can see easily from the ratio (parts) row of the box that the ratio is 1:2.

· What is the total number of boys in the class? You can see easily from the bottom row of the box that it is 16.

· What is the total number of girls in the class? You can see easily from the bottom row of the box that it is 8.

· What fractional part of the class is boys? There are 16 boys in a class of 24, so the fraction representing the boys is , which can be reduced to .

As you can see, the Ratio Box is an easy way to find, organize, and keep track of information on ratio problems. And it works the same no matter what information you are given. Just remember that all the boxes in the Multiply By row will always contain the same number.

Here’s another example:

10. In a jar of red and green jelly beans, the ratio of green jelly beans to red jelly beans is 5:3. If the jar contains a total of 160 jelly beans, how many of them are red?

(A) 30

(B) 53

(C) 60

(D) 100

(E) 160

Mark It!

Always draw the
Ratio Box when working
with ratio questions!

Here’s How to Crack It

First, sketch out a Ratio Box.

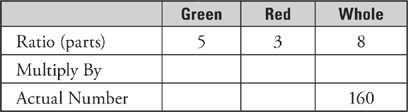
Now find the multiplier. What do you multiply by 8 to get 160? You multiply 8 by 20. Now write 20 in each box on the Multiply By row.

What You Need

Always keep an eye on
what you are being asked.
You don’t want to do more
work than necessary.
Question 10 never asks
about green jelly beans, so
leave that box empty.

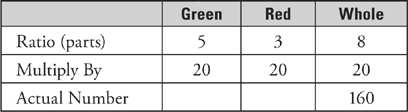
The problem asks you to find how many red jelly beans there are. Go to the red column and multiply 3 by 20. The answer is 60. ETS’s answer is C. Notice that you would have set up the box in exactly the same way if the question had asked you to determine how many jelly beans were green. (How many are green? The answer is 5 × 20, which is 100.)

Don’t forget that you can use more than one technique to solve a problem. There’s no reason why you can’t combine the ratio box with some form of plugging in. In fact, if one technique makes the problem easy, two techniques might make it downright simple!

Here’s a problem for which combining techniques is just the ticket:

17. In Miss Hoover’s class, the ratio of boys to girls is x to y. If the total number of children in the class is five times the number of boys in the class, which of the following is an expression for the number of girls in the class, in terms of x and y ?

(A)

(B)

(C)

(D) 5x

(E) 5xy

Here’s How to Crack It

Since the problem uses the word ratio, you definitely want to use the ratio box. However, the ratio box works best with numbers rather than variables. What to do? Plug in, of course! Make x = 3 and y = 2. So the total of the ratio is 5. Since the question says that the total number of students is 5 times the number of boys, we know that the total number of students is 5(3) or 15. Now, it’s time to draw the ratio box and fill in what you know:

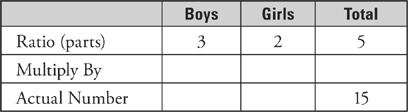
Now, it’s time to use the box to find the target. Since the question wants to know about the number of girls in the class, don’t bother with the number of boys. Here’s what your filled-in box should look like:

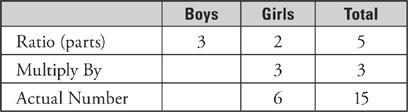
In this example, there are 6 girls in the class. Don’t forget to circle your target. Now, all you need to do is find which answer equals 6 when x = 3 and y = 2. Only answer choice C works.

Some SAT math problems will contain two proportional, or equal, ratios from which one piece of information is missing.

Here’s an example:

5. If 2 packages contain a total of 12 doughnuts, how many doughnuts are there in 5 packages?

(A) 12

(B) 24

(C) 30

(D) 36

(E) 60

Here’s How to Crack It

This problem simply describes two equal ratios, one of which is missing a single piece of information. Here’s the given information represented as two equal ratios:

Because ratios can be written so they look like fractions, we can treat them exactly like fractions. To find the answer, all you have to do is figure out what you could plug in for x that would make . Now cross-multiply:

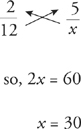
The answer is C.

Fraction Magic

Which way can I simplify?
Here’s the easy trick to
remember: Always simplify
in the direction of the
symbol; that is, a multiplication
sign (×) shows you
can simplify diagonally
and an equal sign (=) says
simplify straight across.

Problems dealing with direct variation (a fancy term for proportion) are exactly what you’ve just seen: If one quantity grows or decreases by a certain amount (a factor), the other quantity grows or decreases by the same amount. Indirect variations (also known as indirect proportions) are just the opposite of that. As one quantity grows or decreases, the other quantity decreases or grows by the same factor.

What’s in a Name?

When you see variation,
think proportion.

The main formula you want to remember for indirect proportions is

x1y1 = x2y2

Try one:

15. The amount of time it takes to consume a buffalo carcass is inversely proportional to the number of vultures. If it takes 12 vultures 3 days to consume a buffalo, how many fewer hours will it take if there are 4 more vultures?

(A)

(B)

(C) 18

(D) 24

(E) 54

Translate!

Direct means divide.
Since indirect is the
opposite, indirect means
multiply.

Here’s How to Crack It

For inverse proportions, follow the formula. First, convert the days: 3 days is equal to 72 hours. Now set up the equation: (12 vultures)(72 hours) = (16 vultures)(x). We solve to get x = 54, which is 18 fewer hours. The answer is C.

Since ratios and proportions are related concepts, you might be wondering how you can tell when you should set the problem up as a proportion and when you should use a ratio box. Here are some guidelines to help you decide.

· If the question gives you a ratio and an actual number, use a ratio box.

· If the question compares items that have different units (like feet and seconds), set up a proportion.

· If you don’t need the total column in the ratio box, then you can also do the question by setting up a proportion.

There should be nothing frightening about a percentage. It’s just a convenient way of expressing a fraction whose bottom is 100.

Percent means “per 100” or “out of 100.” If there are 100 questions on your math test and you answer 50 of them, you will have answered 50 out of 100, or , or 50 percent. To think of it another way:

These show up all the time, so go ahead and memorize them.

0.01 = = 1 percent

0.1 = = 10 percent

0.2 = = 20 percent

0.25 = = 25 percent

0.5 = = 50 percent

0.75 = = 75 percent

To convert a percentage to a fraction, simply put the percentage over 100 and reduce:

Another Way

You can also convert
fractions to percentages
by cross-multiplying:

Because a percentage is just another way to express a fraction, you shouldn’t be surprised to see how easy it is to convert a fraction to a percentage. To do so, simply use your calculator to divide the top of the fraction by the bottom of the fraction, and then multiply the result by 100. Here’s an example:

Problem: Express as a percentage.

Solution: = 0.75 × 100 = 75 percent.

Converting fractions to percentages is easy with your calculator.

To convert a percentage to a decimal, simply move the decimal point two places to the left. For example, 25 percent can be expressed as the decimal 0.25; 50 percent is the same as 0.50 or 0.5; 100 percent is the same as 1.00 or 1.

To convert a decimal to a percentage, just do the opposite of what you did in the preceding section. All you have to do is move the decimal point two places to the right. Thus, 0.5 = 50 percent; 0.375 = 37.5 percent; 2 = 200 percent.

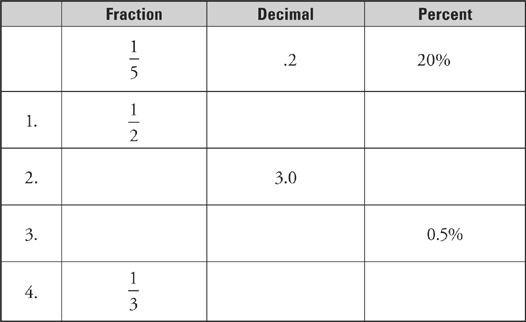
The following drill will give you practice working with fractions, decimals, and percentages.

Fill in the missing information in the following table. Answers can be found on this page.

Word

Symbol

is

=

greater than

+

of

× (multiply)

percent

/100

what

n (variable)

On a math test like the SAT, we can convert (or translate) words into arithmetic symbols. Here are some of the most common:

Problem:
What number is 10 percent greater than 20?

Joe Bloggs makes careless errors on questions like this because he doesn’t think about what the words he’s reading translate to in math. You won’t if you take them slowly and remember what those words look like when you use mathematical symbols. Let’s use the chart above to write this question in math. What number means “variable” so we can write that as n (or x or whatever number works for you!). Is means “equals,” so now we have n = 10%. Greater than translates to +. So now we’ve got our equation:

n = 10% + 20

10 percent of 20 is 2, so

n = 2 + 20
n = 22 

You will see the words of, is, product, sum, and what pop up a lot in the Math sections of the SAT. Don’t let these words fool you because they all translate into simple math functions. Memorize all of these terms and their math equivalents. It will save you time on the test and make your life with the SAT much nicer.

On harder SAT questions, you may be asked to determine the effect of a series of percentage increases or decreases. The key point to remember on such problems is that each successive increase or decrease is performed on the result of the previous one.

Here’s an example:

15. A business paid $300 to rent a piece of office equipment for one year. The rent was then increased by 10% each year thereafter. How much will the company pay for the first three years it rents the equipment?

(A) $920

(B) $960

(C) $990

(D) $993

(E) $999

Bite-Size Pieces

Always handle
percentage problems in
bite-size pieces:
one piece at a time.

Here’s How to Crack It

This problem is a great place to use bite-sized pieces. You know that the business paid $300 to rent the piece of office equipment for the first year. Then, you were told that the rent increases by 10 percent for each year thereafter. That’s a sure sign that you’re going to need the rent for the second year, so go ahead and calculate it. For the second year, the rent is

Now, the problem tells you that the business rents the equipment for three years. So, you need to do the calculation one more time. At this point, you might want to set up a chart to help keep track of the information.

Year 1: $300

Year 2: $330

Year 3:

To find the answer, all you need to do is add up the costs for each of the three years:

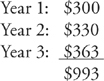
The correct answer is D, $993.

Sometimes you may find successive percentage problems in which you aren’t given actual numbers to work with. In such cases, you need to plug in some numbers.

Careful

Number 17 is a difficult
question. Beware of percentage
change problems
in the hard questions.
The answers to these
problems almost always
defy common sense.
Unless you are careful,
you may fall for a
Joe Bloggs attractor.

Here’s an example:

17. A number is increased by 25 percent and then decreased by 20 percent. The result is what percent of the original number?

(A) 80

(B) 100

(C) 105

(D) 120

(E) 125

Here’s How to Crack It

You aren’t given a particular number to work with in this problem—just “a number.” Rather than trying to deal with the problem in the abstract, you should immediately plug in a number to work with. What number would be easiest to work with in a percentage problem? Why, 100, of course.

1. 25 percent of 100 is 25, so 100 increased by 25 percent is 125.

2. Now you have to decrease 125 by 20 percent; 20 percent of 125 is 25, so 125 decreased by 20 percent is 100.

3. 100 (our result) is 100 percent of 100 (the number you plugged in), so ETS’s answer, once again, is B.

Remember: Never try to solve a percentage problem by writing an equation if you can plug in numbers instead. Plugging in on percentage problems is faster, easier, and more accurate. Why work through long, arduous equations if you don’t have to?

Sometimes ETS will give you a percent problem that uses variables rather than numbers. They’d like you to get confused because you’re not used to seeing percent questions like these. However, if you remember to plug in and to translate the words in the problem, these problems are no big deal.

Here’s an example:

18. Which of the following is equivalent to of 37% of y ?

(A)

(B)

(C)

(D)

(E)

Here’s How to Crack It

If there were numbers in the problem, you could pull out your trusty calculator and get to the answer pretty easily. So, fill in some numbers for the variables! If you make x = 2 and y = 100, you’ll have some pretty nice numbers to work with. With the numbers inserted, the problem now reads as follows:

18. Which of the following is equivalent to of 37% of 100 ?

Now, it’s time to translate. Don’t forget that of means multiply and percent means over 100. So, the words in the problem translate to . So, (or 18.5, if you prefer decimals) is the target. It’s time to go find that in the answer choices. Remember to translate them, as well. Here are the results:

(A)

(B)

(C)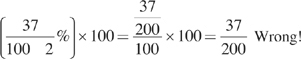

(D)

(E)

So, the answer is E.

There’s one more thing that you should know about percents. Some problems will ask for a percent increase or decrease. For these problems, use the following formula.

Most of the time that you use the formula, it will be pretty clear which number you should use for the original. However, if you’re not sure, remember that you should use the smaller number for the original if you are finding a percent increase. You should use the larger number for the original if you are finding a percent decrease.

Here’s an example of how to use the formula:

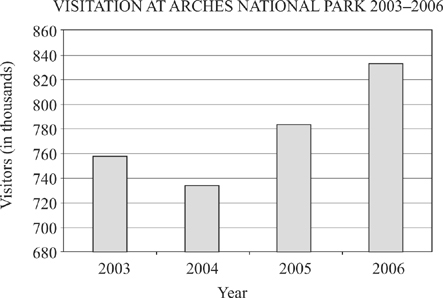
8. The chart shown above shows the number of visits, in thousands, at Arches National Park for the years 2003 to 2006. Which of the following is the closest approximation of the percent increase in the number of visits from 2004 to 2006?

(A) 1.5%

(B) 5%

(C) 15%

(D) 20%

(E) 115%

Here’s How to Crack It

First, you need to get the data from the chart. In 2004, the chart shows that there were approximately 730,000 visitors to Arches. In 2006, the chart shows that there were about 830,000 visitors to the park. Now, it’s time to use the percent change formula. The difference is about 100,000 and the original is the 730,000 visitors in 2004:

The correct answer is C.

The average (also called the arithmetic mean) of a set of n numbers is simply the sum of all the numbers divided by n. In other words, if you want to find the average of three numbers, add them up and divide by 3. For example, the average of 3, 7, and 8 is , which equals , or 6.

That was an easy example, but ETS does not always write average questions with clear solutions. That is, ETS doesn’t always give you the information for averages in a way that is easy to work with. For that reason, we have a visual aid, like the Ratio Box for ratios, that helps you organize the information on average questions and find ETS’s answer.

We call it the Average Pie. Here’s what it looks like:

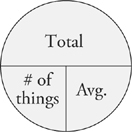
The total is the sum of all the numbers you’re averaging, and the number of things is the number of elements you’re averaging. Here’s what the Average Pie looks like using the simple average example we just gave you.

Here’s how the Average Pie works mathematically. The line in the middle means divide. If you know the total and the number of things, just divide to get the average (18 ÷ 3 = 6). If you know the total and the average, just divide to get the number of things (18 ÷ 6 = 3). If you know the average and the number of things, simply multiply to get the total (6 × 3 = 18). The key to most average questions is finding the total.

Here’s another simple example:

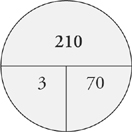
Problem: If the average of three test scores is 70, what is the total of all three test scores?

Solution: Just put the number of things (3 tests) and the average (70) in the pie. Then multiply to find the total, which is 210.

Total

When calculating
averages and means,
always find the total.
It’s the one piece of
information that
ETS loves to withhold.

To solve most difficult average problems, all you have to do is fill out one or more Average Pies. Most of the time you will use them to find the total of the number being averaged. Here’s an example:

Mark It!

Make sure you’re drawing
a new average pie each
time you see the word
average in a question.

10. Maria has taken four chemistry tests and has an average (arithmetic mean) score of 80. If she scores a 90 on her fifth chemistry test, what is her average for these five tests?

(A) 80

(B) 81

(C) 82

(D) 84

(E) 85

Here’s How to Crack It

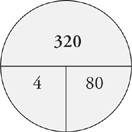
Start by drawing an average pie and filling in what you know. You can put 4 in for the number of things and 80 for the average. You can calculate that Maria has gotten 320 total points on her first four tests. Your pie should look like this:

Now, draw another average pie and fill in what you know. This time, there are five tests. The question wants to know the average, so you also need to fill the total. The total for all five tests is the total from the first four tests plus the score from the fifth test: 320 + 90 = 410. Put that on the pie and divide to find the average:

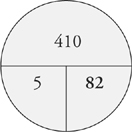
The answer is C, 82.

Now let’s try a difficult test question:

20. If the average (arithmetic mean) of eight numbers is 20, and the average of five of these numbers is 14, what is the average of the other three numbers?

(A) 14

(B) 17

(C) 20

(D) 30

(E) 34

Here’s How to Crack It

Start by drawing an Average Pie for all eight numbers; then multiply to find the total.

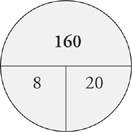
The total of the eight numbers is 160. Now draw another Average Pie for five of the numbers.

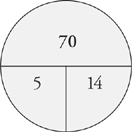
The total of those five numbers is 70. (Remember: Those are five out of the original eight numbers.) To find the average of the other three numbers, you need the total of those three numbers. You have the total of all eight numbers, 160, and the total of five of those numbers, 70, so you can find the total of the other three by subtracting 70 from 160. That means the total of the three remaining numbers is 90. It’s time to create one more Average Pie to find the average of those three numbers.

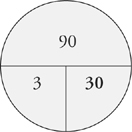
The average is 30, so the answer is D.

Don’t forget that you can also Plug In when using the average pie.

16. The average (arithmetic mean) of a list of 5 numbers is n. When an additional number is added to the list, the average of all 6 numbers is n + 3. Which of the following is the value, in terms of n, of the number added to the list?

(A) n

(B) 5n

(C) n + 6

(D) n + 18

(E) 6n + 18


Need More
Help?

For video
instruction, go to
www.princetonreview.com/cracking.

Here’s How to Crack It

Plug in for the value of n. If n = 20, then you can use the average pie to find the total of the five numbers on the list.

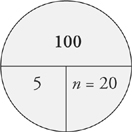
As shown on the average pie above, the total of the 5 numbers is 100. Now, it’s time for another average pie. For this pie, you know that there are 6 numbers and that their average is 20 + 3 = 23.

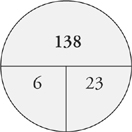
Using the average pie as shown above, the total of the six numbers is 138. Since the difference in the two totals was caused by the addition of the sixth number, the sixth number must be 138 – 100 = 38. That’s the target, so be sure to circle it. Only answer D is 38 when n = 20.

On the SAT, you’ll also need to know two other topics related to averages: median and mode.

The median of a list of numbers is the number that is exactly in the middle of the list when the list is arranged from smallest to largest, as on a number line. For example, in the group 3, 6, 6, 6, 6, 7, 8, 9, 10, 10, 11, the median is 7. Five numbers come before 7 in the list, and 5 come after. Remember it this way: Median sounds like middle.

Missing the Middle?

To find the median
of a set containing an
even number of items,
take the average of the
two middle numbers.

The mode of a group of numbers is the number in the list that appears most often. In the list 3, 4, 4, 5, 7, 7, 8, 8, 8, 9, 10, the mode is 8, because it appears three times while no other number in the group appears more than twice. Remember it this way: Mode sounds like most.

Probability is a mathematical expression of the likelihood of an event. The basis of probability is simple. The likelihood of any event is discussed in terms of all of the possible outcomes. To express the probability of a given event, x, you would count the number of possible outcomes, count the number of outcomes that give you what you want, and arrange them in a fraction, like this:

Every probability is a fraction. The largest a probability can be is 1; a probability of 1 indicates total certainty. The smallest a probability can be is 0, meaning that it’s something that cannot happen. Furthermore, you can find the probability that something WILL NOT happen by subtracting the probability that it WILL happen from 1. For example, if the weatherman tells you that there is a 0.3 probability of rain today, then there must be a 0.7 probability that it won’t rain, because 1 – 0.3 = 0.7. Figuring out the probability of any single event is usually simple. When you flip a coin, there are only two possible outcomes, heads and tails; the probability of getting heads is therefore 1 out of 2, or . When you roll a die, there are six possible outcomes, 1 through 6; the odds of getting a 6 is therefore . The odds of getting an even result when rolling a die are because there are 3 even results in 6 possible outcomes.

Here’s an example of a probability question:

12. A bag contains 7 blue marbles and 14 marbles that are not blue. If one marble is drawn at random from the bag, what is the probability that the marble is blue?

(A)

(B)

(C)

(D)

(E)

Here’s How to Crack It

Here, there are 21 marbles in the bag, 7 of which are blue. The probability that a marble chosen at random would be blue is therefore , or . The correct answer is B.

Some probability questions might include variables. Not to worry. Plugging in will save the day!

Here’s an example:

17. A jar contains only red marbles and white marbles. If the probability of selecting a red marble is , which of the following expressions gives the probability of selecting a white marble in terms of r and y ?

(A)

(B)

(C)

(D)

(E)

Here’s How to Crack It

Plug in! You could make the probability of choosing a red marble be . Then which means that r = 2 and y = 3.

Now, to get the numerical answer, you need to remember that the probabilities of all the things that can happen always add up to 1. Since there are only red marbles and white marbles in the jar, choosing a red marble or choosing a white marble are the only things that can happen. So, the probability of selecting a white marble can be found by subtracting the probability of getting a red marble from 1: Probability of white = 1 − . That’s your target, so circle it.

Now, go find the answer that gives you when r = 2 and y = 3. Answer B is . Since no other answer evaluates to , B is the answer.

A permutation is an arrangement of objects of a definite order. The simplest sort of permutations question might ask you how many different arrangements are possible for six different chairs in a row, or how many different four-letter arrangements of the letters in the word FUEL are possible. Both of these simple questions can be answered with the same technique.

Just draw a row of boxes corresponding to the positions you have to fill. In the case of the chairs, there are six positions, one for each chair. You would make a sketch like this:

Then, in each box, write the number of objects available to be put into that box. Keep in mind that objects put into previous boxes are no longer available. For the chair-arranging example, there would be six chairs available for the first box; only five left for the second box; four for the third, and so on until only one chair remained to be put into the last position. Finally, just multiply the numbers in the boxes together, and the product will be the number of possible arrangements, or permutations.

There are 720 possible permutations of a group of six chairs. This number can also be written as 6!. That’s not a display of enthusiasm—the exclamation point means factorial. The number is read “six factorial,” and it means 6 × 5 × 4 × 3 × 2 × 1, which equals 720. A factorial is simply the product of a series of integers counting down to 1 from the specified number. For example, the number 70! means 70 × 69 × 68 … 3 × 2 × 1.

Don’t Forget Your
Calculator!

Look at your calculator for
the factorial sign.
It can simplify your life.
On a scientific
calculator, it is often a
shift key and the symbol is
x!. On a graphing
calculator, it can usually
be found in [MATH]
in [Probability]. Knowing
where the key is and how
to use it can speed things
along. Don’t forget about
your parentheses!

The number of possible arrangements of any group with n members is simply n!. In this way, the number of possible arrangements of the letters in FUEL is 4!, because there are four letters in the group. That means 4 × 3 × 2 × 1 arrangements, or 24. If you sketched four boxes for the four letter positions and filled in the appropriate numbers, that’s exactly what you’d get.

Permutations get a little trickier when you work with smaller arrangements. For example, what if you were asked how many two-letter arrangements could be made from the letters in FUEL? It’s just a modification of the original counting procedure. Sketch two boxes for the two positions. Then fill in the number of letters available for each position. As before, there are four letters available for the first space, and three for the second; the only difference is that you’re done after two spaces:

As you did before, multiply the numbers in the boxes together to get the total number of arrangements. You should find there are 12 possible two-letter arrangements from the letters in FUEL.

That’s all there is to permutations. The box-counting procedure is the safest way to approach them. Just sketch the number of positions available, and fill in the number of objects available for each position, from first to last—then multiply those numbers together.

Try one:

17. Hal wrote 7 essays in his English class. He wants to put all 7 essays in his portfolio and is deciding in what order to place the essays. In how many different orders can Hal arrange his essays?

(A) 49

(B) 420

(C) 5,040

(D) 5,670

(E) 10,549

Here’s How to Crack It

There are seven essays that could be first. Once an essay is selected for first place, there are six left that could be second, then five that could be third, four that could be fourth, three that could be fifth, two that could be sixth, and one that will be last. Multiply all of those choices together: 7 × 6 × 5 × 4 × 3 × 2 × 1 = 5,040. The correct answer is C.

There’s one final thing to know about counting problems. Sometimes, some of the positions might have some restrictions on what you can put there. Be sure that you remember those restrictions when you write down how many things could go in each of those positions.

Here’s an example:

19. In the three-digit integer 735, the digits are all different and the hundreds digit and the units digit are both prime. How many three-digit integers have digits that are all different and have both prime hundreds and units digits?

(A) 24

(B) 96

(C) 120

(D) 160

(E) 250

Here’s How to Crack It

Since there are three digits in the number, start by drawing out three boxes. The hundreds digit needs to be prime, so it could be any of the digits 2, 3, 5, or 7. (Don’t forget that 1 is not prime!) So, there are 4 choices for the hundreds digit. Write 4 in your first box. The problem will be easier to think about if you fill in the choices for the more restricted of the remaining two boxes next. So, jump over to the last box. The units digit also needs to be prime but it also needs to be different from the hundreds digit. That means there are only 3 choices left for the units digit. Finally, it’s time to fill in the box for the tens digit. It doesn’t need to be prime but it does need to be different from the hundreds and the units digits. So, rather than there being 10 choices (the digits 0 through 9), there are really only 8 choices left. To finish the problem, all you need to do is multiply the choices together: 4 × 8 × 3 = 96. The correct answer is B.

Work these problems using the advanced algebra techniques and algebra tips we’ve covered in this chapter. Answers can be found on this page.

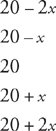
5. What is the average (arithmetic mean) of the list of numbers above?

(A) 20

(B) 100

(C)

(D) 4 + x

(E)

8. Henry needs to choose 2 statues to arrange on his lawn. If he has 9 statues, how many total arrangements of statues are possible?

(A) 18

(B) 36

(C) 72

(D) 81

(E) 108

9. The amount of time that Amy walks is directly proportional to the distance that she walks. If she walks a distance of 2.5 miles in 50 minutes, how many miles will she walk in 2 hours?

(A) 3

(B) 4.5

(C) 5

(D) 6

(E) 6.5

10. A total of 140,000 votes were cast for two candidates, Skinner and Whitehouse. If Skinner won by a ratio of 4 to 3, how many votes were cast for Whitehouse?

(A) 30,000

(B) 40,000

(C) 60,000

(D) 80,000

(E) 105,000

14. Which of the following is equivalent to 1/4 of 18% of 616?

(A) 18% of 2,464

(B) 4.5% of 154

(C) 72% of 616

(D) 18% of 154

(E) 18.25% of 616

16. Of all the houses in a certain neighborhood, 80% have garages. Of those houses with garages, 60% have two-car garages. If there are 56 houses with garages that are not two-car garages, how many houses are there in the neighborhood?

(A) 26

(B) 93

(C) 117

(D) 156

(E) 175

18. On Tuesday, a watchmaker made 4 more watches than he had made during the previous day. If he made 16% more watches on Tuesday than on Monday, how many watches did he make on Tuesday?

(A) 16

(B) 20

(C) 21

(D) 25

(E) 29

· A ratio can be expressed as a fraction, but ratios are not fractions. A ratio compares parts to parts; a fraction compares a part to the whole.

· Use a Ratio Box to solve ratio questions.

· Direct proportion is . Indirect proportion is x1y1 = x2 y2.

· A percentage is just a convenient way of expressing a fraction whose bottom is 100.

· To convert a percentage to a fraction, put the percentage over 100 and reduce.

· To convert a fraction to a percentage, use your calculator to divide the top of the fraction by the bottom of the fraction. Then multiply the result by 100.

· To convert a percentage to a decimal, move the decimal point two places to the left. To convert a decimal to a percentage, move the decimal point two places to the right.

· In problems that require you to find a series of percentage increases or decreases, remember that each successive increase or decrease is performed on the result of the previous one.

· If you need to find the percent increase or decrease use % change = × 100

· To find the average (arithmetic mean) of several values, add up the values and divide the total by the number of values.

· Use the Average Pie to solve problems involving averages. The key to most average problems is finding the total.

· The median of a group of numbers is the number that is exactly in the middle of the group when the group is arranged from smallest to largest, as on a number line. If there are an even number of numbers, the median is the average of the two middle numbers.

· The mode of a group of numbers is the number in the group that appears most often.

· Probability is expressed as a fraction:

· To find permutations, or possible orders of objects, use factorials. A factorial is the whole series of integers counting down from the given number, all multiplied together.