SAT Test Prep

CHAPTER 9
SPECIAL MATH PROBLEMS

Lesson 4: Rate Problems

What Are Rates?

The word rate comes from the same Latin root as the word ratio. All rates are ratios. The most common type of rate is speed, which is a ratio with respect to time, as in miles per hour or words per minute, but some rates don”t involve time at all, as in miles per gallon. Rate units always haveper in their names: miles per gallon, meters per second, etc. Per, remember, means divided by, and is like the colon (:) or fraction bar in a ratio.

The Rate Pyramid

The name of any rate is equivalent to its formula. For instance, speed is miles per hour

or

Since this formula is similar to the “average” formula, you can make a rate pyramid.

This can be a great tool for solving rate problems. If a problem gives you two of the quantities, just put them in their places in the pyramid, and do the operation between them to find the missing quantity.

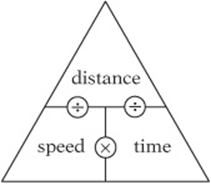
Example:

How long will it take a car to travel 20 miles at 60 miles per hour?

Simply fill the quantities into the pyramid: 20 miles goes in the distance spot, and 60 miles an hour goes in the speed spot. Now what? Just do the division the way the diagram says: 20 miles ÷ 60 miles per hour = 1/3 hour.

Watch Your Units

Whenever you work with formulas, you can check your work by paying attention to units. For instance, the problem above asks how long, so the calculation has to produce a time unit. Check the units in the calculation:

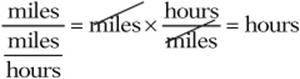
Two-Part Rate Problems

Rate problems are tougher when they involve two parts. When a problem involves, say, two people working together at different rates and times, or a two-part trip, you have to analyze the problem more carefully.

Example:

Toni bicycles to work at a rate of 20 miles per hour, then takes the bus home along the same route at a rate of 40 miles per hour. What is her average speed for the entire trip?

At first glance, it might seem that you can just average the two rates: miles per hour, since she is traveling the same distance at each of the two speeds. But this won”t work, because she isn”t spending the same time at each speed, and that is what”s important. But if that”s true, you might notice that she spends twice as much time going 20 miles per hour as 40 miles per hour (since it”s half as fast), so instead of taking the average of 20 and 40, you can take the average of two 20s and a 40:

miles per hour. Simple! But if that doesn”t make sense to you, think of it this way: Imagine, for simplicity”s sake, that her trip to work is 40 miles. (It doesn”t matter what number you pick, and 40 is an easy number to work with here.) Now the average speed is simply the total distance divided by the total time (as the pyramid says). The total distance, there and back, is 80 miles. The total time is in two parts. Getting to work takes her 40 miles ÷ 20 miles per hour = 2 hours. Getting home takes her 40 miles 40 miles ÷ per hour = 1 hour. So the totaltime of the trip is 3 hours. The average speed, then, must be 80 miles ÷ 3 hours = 26.67 miles per hour!

Concept Review 4: Rate Problems

For each of the following rates, write the formula of the rate and the corresponding “rate pyramid.”

1. Speed is miles per hour.

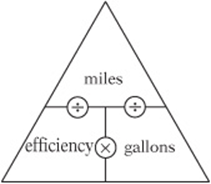
2. Efficiency is miles per gallon of fuel.

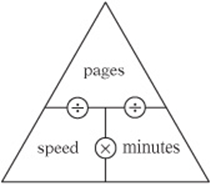
3. Typing speed is pages per minute.

Find the missing quantity, including the units, in each of these rate situations.

4. A train travels for 375 miles at 75 mph.

5. A car that gets 28 miles per gallon uses 4.5 gallons of fuel.

6. Harold can type 600 words in 5 minutes.

7. A landscaper who cuts 1.6 acres of grass per hour cuts an 8-acre lot.

8. A train leaves New York at 1:00 pm, going 50 mph, bound for Philadelphia, which is 90 miles away. If it makes no stops, at what time should it be expected to arrive?

9. Anne can paint a room in 2 hours, and Barbara can paint a room in 3 hours. When they work together, their work rate is the sum of their rates working separately. How long should it take them to paint a room if they work together?

SAT Practice 4: Rate Problems

1. Janice and Edward are editors at a newspaper. Janice can edit 700 words per minute and Edward can edit 500 words per minute. If each page of text contains 800 words, how many pages can they edit, working together, in 20 minutes?

2. Two cars leave the same point simultaneously, going in the same direction along a straight, flat road, one at 35 mph and one at 50 mph. After how many minutes will the cars be 5 miles apart?

3. What is the average speed, in miles per hour, of a sprinter who runs ¼ mile in 45 seconds?

(A) 11.25 mph

(B) 13.5 mph

(C) 20 mph

(D) 22 mph

(E) 25 mph

4. A car travels d miles in t hours and arrives at its destination 3 hours late. At what average speed, in miles per hour, should the car have gone in order to have arrived on time?

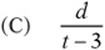
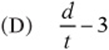
5. If , how many hours does it take a train traveling at miles per hour to travel ?

(C) x

6. In three separate 1-mile races, Ellen finishes with times of x minutes, y minutes, and z minutes. What was her average speed, in miles per hour, for all three races?

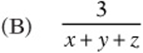
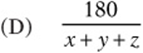
7. A hare runs at a constant rate of a mph, a tortoise runs at a constant rate of b mph, and . If they race each other for d miles, how many more hours, in terms of a, b, and d, will it take the tortoise to finish than the hare?

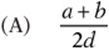
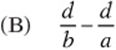
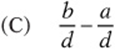
8. Sylvia drives 315 miles and arrives at her destination in 9 hours. If she had driven at an average rate that was 10 mph faster than her actual rate, how many hours sooner would she have arrived?

(A) 1.75

(B) 2.00

(C) 2.25

(D) 2.50

(E) 2.75

Answer Key 4: Rate Problems

Concept Review 4

1. Speed = #miles ÷ hours

2. Efficiency =#miles ÷#gallons

3. Typing speed =#pages ÷ #minutes

4. 375 miles ÷ 75 mph = 5 hours for the trip.

5. 28 miles per gallon × 4.5 gallons = 126 miles the car can go before it runs out of fuel.

6. 600 words ÷ 5 minutes = 120 words per minute is Harold”s typing speed.

7. 8 acres ÷ 1.6 acres per hour = 5 hours for the job.

8. 90 miles ÷ 50 mph = 1.8 hours, or 1 hour 48 minutes for the entire trip. At 1 hour and 48 minutes after 1:00 pm, it is 2:48 pm.

9. Anne can paint one room in 2 hours, so her rate is ½ room per hour. Barbara can paint one room in 3 hours, so her rate is room per hour. When they work together, their rate is room per hour. So to paint one room would take one room ÷ room per hour = hours, or 1.2 hours, or 1 hour 12 minutes.

SAT Practice 4

1. 30 Working together, they edit words per minute. Since each page is 800 words, that”s 1,200 words per minute ÷ 800 words per page = 1.5 pages per minute. In 20 minutes, then, they can edit pages.

2. 20 Since the two cars are traveling in the same direction, their relative speed (that is, the speed at which they are moving away from each other) is mph. In other words, they will be 15 miles farther apart each hour. Therefore, the time it takes them to get 5 miles apart is 5 miles ÷ 15 miles per hour = 1/3 hour, which is equivalent to 20 minutes.

3. C Since there are seconds in an hour, hour. Speed = distance ÷ time = ¼ mile ÷ 45/3,600 hour = 3,600/180 = 20 miles per hour.

4. C To arrive on time, the car must take hours for the whole trip. To travel d miles in hours, the car must go miles per hour.

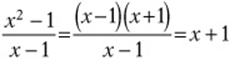5. E According to the rate pyramid, time = distance ÷ speed = miles ÷ miles per hour = hours. Or you can pick a simple value for x, like 2, and solve numerically.

6. D Speed = miles ÷ hours. Her total time for the three races is minutes, which we must convert to hours by multiplying by the conversion factor (1 hour/60 minutes), which gives us hours. Since her total distance is 3 miles, her overall speed is 3 miles ÷ hours = 180/ miles per hour.

7. B If the hare”s rate is a mph, then he covers d miles in d/a hours. Similarly, the tortoise covers d miles in d/b hours. The difference in their finishing times, then, is .

8. B Sylvia”s speed is 315 miles ÷ 9 hours = 35 mph. If she were to go 10 mph faster, then her speed would be 45 mph, so her time would be 315 miles ÷ 45 mph = 7 hours, which is 2 hours sooner.