CHEMISTRY THE CENTRAL SCIENCE
11 LIQUIDS AND INTERMOLECULAR FORCES
11.5 VAPOR PRESSURE
Molecules can escape from the surface of a liquid into the gas phase by evaporation. Suppose we place a quantity of ethanol (CH3CH2OH) in an evacuated, closed container, as in ![]() FIGURE 11.23. The ethanol quickly begins to evaporate. As a result, the pressure exerted by the vapor in the space above the liquid increases. After a short time the pressure of the vapor attains a constant value, which we call the vapor pressure.
FIGURE 11.23. The ethanol quickly begins to evaporate. As a result, the pressure exerted by the vapor in the space above the liquid increases. After a short time the pressure of the vapor attains a constant value, which we call the vapor pressure.
At any instant, some of the ethanol molecules at the liquid surface possess sufficient kinetic energy to overcome the attractive forces of their neighbors and, therefore, escape into the gas phase. At any particular temperature, the movement of molecules from liquid phase to gas phase goes on continuously. As the number of gas-phase molecules increases, however, the probability increases that a molecule in the gas phase will strike the liquid surface and be recaptured by the liquid, as shown in the flask on the right in Figure 11.23. Eventually, the rate at which molecules return to the liquid equals the rate at which they escape. The number of molecules in the gas phase then reaches a steady value, and the pressure exerted by the vapor becomes constant.

![]() FIGURE 11.23 Equilibrium vapor pressure over a liquid.
FIGURE 11.23 Equilibrium vapor pressure over a liquid.
The condition in which two opposing processes occur simultaneously at equal rates is called dynamic equilibrium (or simply equilibrium). A liquid and its vapor are in dynamic equilibrium when evaporation and condensation occur at equal rates. It may appear that nothing is occurring at equilibrium because there is no net change in the system. In fact, though, a great deal is happening as molecules continuously pass from liquid state to gas state and from gas state to liquid state. The vapor pressure of a liquid is the pressure exerted by its vapor when the liquid and vapor are in dynamic equilibrium.
Volatility, Vapor Pressure, and Temperature
When vaporization occurs in an open container, as when water evaporates from a bowl, the vapor spreads away from the liquid. Little, if any, is recaptured at the surface of the liquid. Equilibrium never occurs, and the vapor continues to form until the liquid evaporates to dryness. Substances with high vapor pressure (such as gasoline) evaporate more quickly than substances with low vapor pressure (such as motor oil). Liquids that evaporate readily are said to be volatile.
Hot water evaporates more quickly than cold water because vapor pressure increases with increasing temperature. To see why this statement is true, we begin with the fact that the molecules of a liquid move at various speeds. ![]() FIGURE 11.24 shows the distribution of kinetic energies of the molecules at the surface of a liquid at two temperatures. (The curves are like those shown for gases in Section 10.7.) As the temperature is increased, the molecules move more energetically and more of them can break free from their neighbors and enter the gas phase, increasing the vapor pressure.
FIGURE 11.24 shows the distribution of kinetic energies of the molecules at the surface of a liquid at two temperatures. (The curves are like those shown for gases in Section 10.7.) As the temperature is increased, the molecules move more energetically and more of them can break free from their neighbors and enter the gas phase, increasing the vapor pressure.
![]() GO FIGURE
GO FIGURE
As the temperature increases, does the rate of molecules escaping into the gas phase increase or decrease?
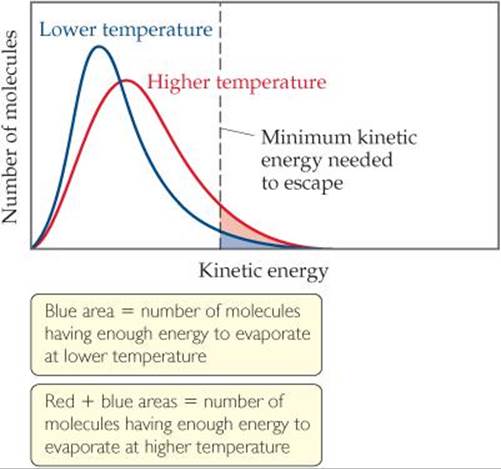
![]() FIGURE 11.24 The effect of temperature on the distribution of kinetic energies in a liquid.
FIGURE 11.24 The effect of temperature on the distribution of kinetic energies in a liquid.
![]() FIGURE 11.25 depicts the variation in vapor pressure with temperature for four common substances that differ greatly in volatility. Note that the vapor pressure in all cases increases nonlinearly with increasing temperature. The weaker the intermolecular forces in the liquid, the more easily molecules can escape and, therefore, the higher the vapor pressure at a given temperature.
FIGURE 11.25 depicts the variation in vapor pressure with temperature for four common substances that differ greatly in volatility. Note that the vapor pressure in all cases increases nonlinearly with increasing temperature. The weaker the intermolecular forces in the liquid, the more easily molecules can escape and, therefore, the higher the vapor pressure at a given temperature.
![]() GIVE IT SOME THOUGHT
GIVE IT SOME THOUGHT
Which compound do you think is more volatile at 25 °C: CCl4 or CBr4?
![]() GO FIGURE
GO FIGURE
What is the vapor pressure of ethylene glycol at its normal boiling point?

![]() FIGURE 11.25 Vapor pressure for four liquids as a function of temperature.
FIGURE 11.25 Vapor pressure for four liquids as a function of temperature.
Vapor Pressure and Boiling Point
A liquid boils when its vapor pressure equals the external pressure acting on the liquid surface. At this point, bubbles of vapor form within the liquid. The temperature at which a given liquid boils increases with increasing external pressure. The boiling point of a liquid at 1 atm (760 torr) pressure is called its normal boiling point. From Figure 11.25 we see that the normal boiling point of water is 100 °C.
The time required to cook food in boiling water depends on the water temperature. In an open container, that temperature is 100 °C, but it is possible to boil at higher temperatures. Pressure cookers work by allowing steam to escape only when it exceeds a predetermined pressure; the pressure above the water can therefore increase above atmospheric pressure. The higher pressure causes the water to boil at a higher temperature, thereby allowing the food to get hotter and to cook more rapidly.
The effect of pressure on boiling point also explains why it takes longer to cook food at high elevations than it does at sea level. The atmospheric pressure is lower at higher altitudes, so water boils at a temperature lower than 100 °C, and foods generally take longer to cook.
 A CLOSER LOOK
A CLOSER LOOK
THE CLAUSIUS–CLAPEYRON EQUATION
You might have noticed that the plots in Figure 11.25 have a distinct shape: For each substance, the vapor pressure curves sharply upward with increasing temperature. The relationship between vapor pressure and temperature is given by the Clausius–Clapeyron equation:
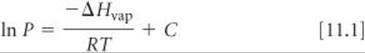
where P is the vapor pressure, T is the absolute temperature, R is the gas constant (8.314 J/mol-K), ΔHvap is the molar enthalpy of vaporization, and C is a constant. This equation predicts that a graph of ln P versus 1/T should give a straight line with a slope equal to ΔHvap/R. Thus, we can use such a plot to determine the enthalpy of vaporization of a substance by using the relationship
ΔHvap = —slope × R
As an example of how we use the Clausius-Clapeyron equation, the vapor-pressure data for ethanol shown in Figure 11.25 are graphed as ln P versus 1/T in ![]() FIGURE 11.26. The data lie on a straight line with a negative slope. We can use the slope to determine ΔHvap for ethanol, 38.56 kJ/mol. We can also extrapolate the line to obtain the vapor pressure of ethanol at temperatures above and below the temperature range for which we have data.
FIGURE 11.26. The data lie on a straight line with a negative slope. We can use the slope to determine ΔHvap for ethanol, 38.56 kJ/mol. We can also extrapolate the line to obtain the vapor pressure of ethanol at temperatures above and below the temperature range for which we have data.
RELATED EXERCISES: 11.83, 11.84, 11.85
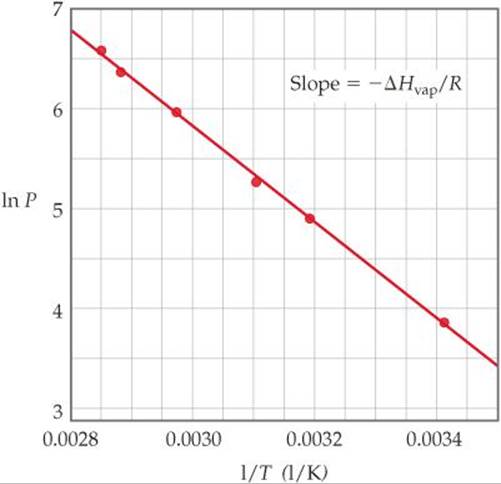
![]() FIGURE 11.26 The natural logarithm of vapor pressure versus 1/T for ethanol.
FIGURE 11.26 The natural logarithm of vapor pressure versus 1/T for ethanol.
SAMPLE EXERCISE 11.4 Relating Boiling Point to Vapor Pressure
Use Figure 11.25 to estimate the boiling point of diethyl ether under an external pressure of 0.80 atm.
SOLUTION
Analyze We are asked to read a graph of vapor pressure versus temperature to determine the boiling point of a substance at a particular pressure. The boiling point is the temperature at which the vapor pressure is equal to the external pressure.
Plan We need to convert 0.80 atm to torr because that is the pressure scale on the graph. We estimate the location of that pressure on the graph, move horizontally to the vapor pressure curve, and then drop vertically from the curve to estimate the temperature.
Solve The pressure equals (0.80 atm)(760 torr/atm) = 610 torr. From Figure 11.25 we see that the boiling point at this pressure is about 27 °C, which is close to room temperature.
Comment We can make a flask of diethyl ether boil at room temperature by using a vacuum pump to lower the pressure above the liquid to about 0.8 atm.
PRACTICE EXERCISE
At what external pressure will ethanol have a boiling point of 60 °C?
Answer: about 340 torr (0.45 atm)