MCAT General Chemistry Review
Chapter 5: Chemical Kinetics
Conclusion
We began with a consideration of chemical reactions and the mechanisms that illustrate the individual steps necessary to transform reactants into products. We demonstrated the way to derive a reaction’s rate law through the analysis of experimental data, and we looked at the factors that can affect the rates of chemical reactions.
After such an overview, you should begin to appreciate that many chemical principles in the human body rely on the principles of chemical kinetics. Why does the body maintain a certain temperature? Primarily to stabilize the enzymes that catalyze the metabolic reactions necessary for life. Why does the body maintain a pH buffer? Altering the concentration of protons affects not only the ability of an enzyme to maintain its secondary, tertiary, and quaternary structure, but can also directly affect the collisions between reactants. You will begin to appreciate these and many other questions from a clinical perspective throughout your medical career. In the next chapter, we will investigate chemical equilibria, which—although related to kinetics—are distinct (and commonly confused!) topics.
Concept Summary
Chemical Kinetics
· The change in Gibbs free energy (ΔG) determines whether or not a reaction is spontaneous.
· Chemical mechanisms propose a series of steps that make up the overall reaction.
o Intermediates are molecules that exist within the course of a reaction, but are neither reactants nor products overall.
o The slowest step, also known as the rate-determining step, limits the maximum rate at which the reaction can proceed.
· The collision theory states that a reaction rate is proportional to the number of effective collisions between the reacting molecules.
o For a collision to be effective, molecules must be in the proper orientation and have sufficient kinetic energy to exceed the activation energy.
o The Arrhenius equation is a mathematical way of representing collision theory.
· The transition state theory states that molecules form a transition state or activated complex during a reaction in which the old bonds are partially dissociated and the new bonds are partially formed.
o From the transition state, the reaction can proceed toward products or revert back to reactants.
o The transition state is the highest point on a free energy reaction diagram.
· Reaction rates can be affected by a number of factors.
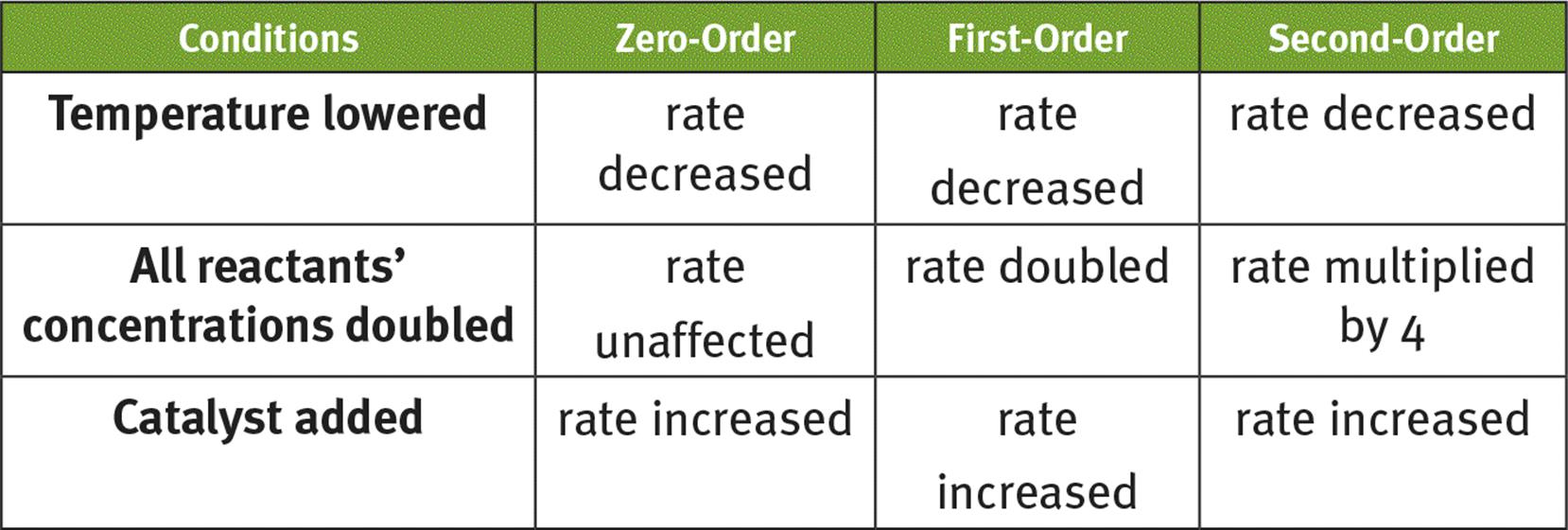
o Increasing the concentration of reactant will increase reaction rate (except for zero-order reactions) because there are more effective collisions per time.
o Increasing the temperature will increase reaction rate because the particles’ kinetic energy is increased.
o Changing the medium can increase or decrease reaction rate, depending on how the reactants interact with the medium.
o Adding a catalyst increases reaction rate because it lowers the activation energy. Homogeneous catalysts are the same phase as the reactants; heterogeneous catalysts are a different phase.
Reaction Rates
· Reaction rates are measured in terms of the rate of disappearance of a reactant or appearance of a product.
· Rate laws take the form of rate = k[A]x[B]y.
o The rate orders usually do not match the stoichiometric coefficients.
o Rate laws must be determined from experimental data.
· The rate order of a reaction is the sum of all individual rate orders in the rate law.
· Zero-order reactions have a constant rate that does not depend on the concentration of reactant.
o The rate of a zero-order reaction can only be affected by changing the temperature or adding a catalyst.
o A concentration vs. time curve of a zero-order reaction is a straight line; the slope of such a line is equal to –k.
· First-order reactions have a nonconstant rate that depends on the concentration of reactant.
o A concentration vs. time curve of a first-order reaction is nonlinear.
o The slope of a ln [A] vs. time plot is –k for a first-order reaction.
· Second-order reactions have a nonconstant rate that depends on the concentration of reactant.
o A concentration vs. time curve of a second-order reaction is nonlinear.
o The slope of a ![]() vs. time plot is k for a second-order reaction.
vs. time plot is k for a second-order reaction.
· Broken-order reactions are those with noninteger orders.
· Mixed-order reactions are those that have a rate order that changes over time.
Answers to Concept Checks
· 5.1
1. Two molecules of A2B come together in a combination reaction to form an intermediate, A4B2, which subsequently decomposes to produce the final products, two molecules of A2 and one molecule of B2.
2. The rate-determining step is the slowest step of a reaction. It determines the overall rate of the reaction because the reaction can only proceed as fast as the rate at which this step occurs.
3. The activation energy is the minimum energy needed for a chemical reaction to occur.
4. Both theories require a certain activation energy to be overcome in order for a reaction to occur (therefore not all reactions will occur). The transition state theory focuses on forming a high-energy activated complex that can then proceed forward or backward, forming the products or reverting to the reactants, respectively. The collision theory focuses on the energy and orientation of reactants, and considers each potential reaction to be “all-or-nothing” (either there is enough energy to form the products, or there is not).
· 5.2
1. 
2. The rate law is: rate = k[A]3[B]0[C]1 = k[A]3[C]. The rate order is 3 + 0 + 1 = 4.
Equations to Remember
(5.1) Collision theory: rate = Z × f
(5.2) Arrhenius equation: ![]()
(5.3) Definition of rate:
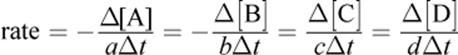
for the general reaction aA + bB → cC + dD
(5.4) Rate law: rate = k[A]x[B]y
(5.5) Radioactive decay: [A]t = [A]0e–kt
Shared Concepts
· Biochemistry Chapter 2
o Enzymes
· General Chemistry Chapter 3
o Bonding and Chemical Interactions
· General Chemistry Chapter 6
o Equilibrium
· General Chemistry Chapter 7
o Thermochemistry
· Organic Chemistry Chapter 4
o Analyzing Organic Reactions
· Physics and Math Chapter 3
o Thermodynamics