MCAT General Chemistry Review - Alexander Stone Macnow, MD 2019-2020
Thermochemistry
Heat
LEARNING GOALS
After Chapter 7.3, you will be able to:
· Differentiate between temperature and heat
· Compare specific heat and heat capacity
· Recall the specific heat of water
· Describe the processes for constant-volume and constant-pressure calorimetry: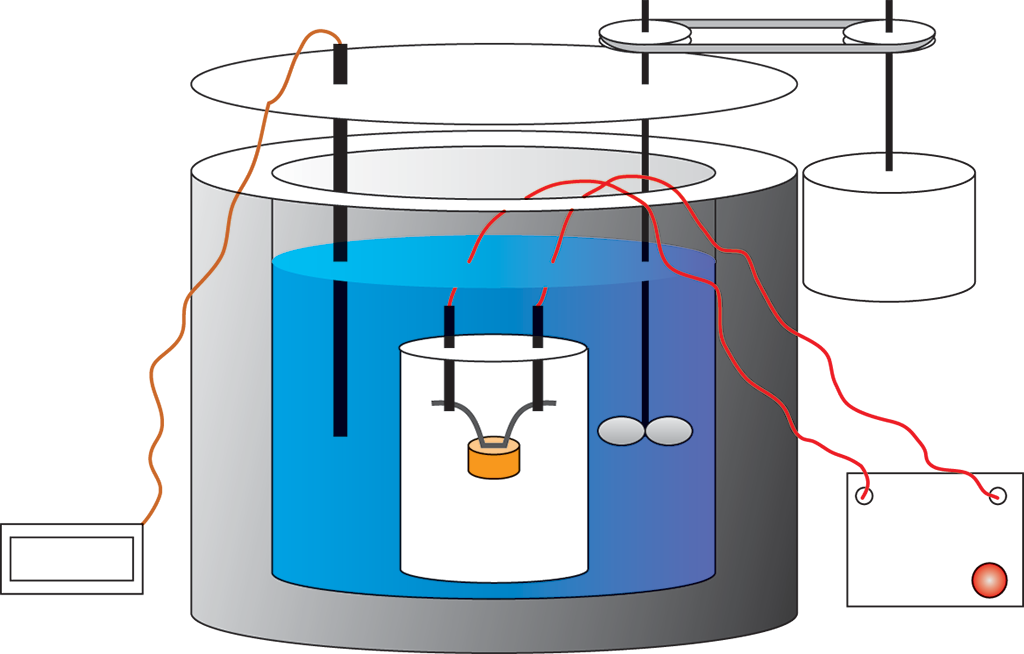
Before we can examine the first of the four state functions that are the focus of this chapter, we must address the topic of heat, which is a source of confusion for many students. Perhaps the greatest barrier to a proper understanding of heat is the semantic conflation of the terms heat and temperature. Many people use these terms interchangeably in everyday conversation, but this obscures the lexicon of thermodynamics. Temperature (T) is related to the average kinetic energy of the particles of a substance. Temperature is the way that we scale how hot or cold something is. We are familiar with a few temperature scales: Fahrenheit, Celsius, and Kelvin. The average kinetic energy of the particles in a substance is related to the thermal energy (enthalpy) of the substance, but because we must also include consideration of how much substance is present to calculate total thermal energy content, the most we can say about temperature is that when a substance’s thermal energy increases, its temperature also increases. Nevertheless, we cannot say that something that is hot necessarily has greater thermal energy (in absolute terms) than a substance that is cold. For example, we might determine that a large amount of lukewarm water has a greater total heat content than a very small amount of hot water.
Key Concept
Remember that heat and temperature are different. Heat is a specific form of energy that can enter or leave a system, while temperature is a measure of the average kinetic energy of the particles in a system.
The absolute temperature scale, Kelvin, was determined via the third law of thermodynamics, which elucidated that there is a finite limit to temperature below which nothing can exist. There can be no temperature below 0 K because, by definition, the system is said to be unable to lose any more heat energy. Quantum mechanics describes a state of molecular motions possible below absolute zero, but this is beyond the scope of the MCAT.
OVERVIEW
Heat (Q) is the transfer of energy from one substance to another as a result of their differences in temperature. In fact, the zeroth law of thermodynamics implies that objects are in thermal equilibrium only when their temperatures are equal. Heat is therefore a process function, not a state function: we can quantify how much thermal energy is transferred between two or more objects as a result of their difference in temperatures by measuring the heat transferred.
Remember that the first law of thermodynamics states that the change in the total internal energy (ΔU) of a system is equal to the amount of heat (Q) transferred to the system minus the amount of work (W) done by the system: ΔU = Q − W.
Because heat and work are measured independently, we can assess the transfer of energy in the form of heat through any process regardless of the amount of work done. Processes in which the system absorbs heat are called endothermic (ΔQ > 0), while those processes in which the system releases heat are called exothermic (ΔQ < 0). The unit of heat is the unit of energy: joule (J) or calorie (cal), for which 1 cal = 4.184 J. Enthalpy (ΔH) is equivalent to heat (Q) under constant pressure, which is an assumption the MCAT usually makes for thermodynamics problems.
Real World
One of the most important ways that the body works to prevent overheating is through the production of sweat—an exocrine secretion of water, electrolytes, and urea. However, it is not the production of sweat that is the cooling mechanism. It’s the evaporation of the sweat that helps cool the body. Evaporation (vaporization) from the liquid to gas phase is an endothermic process: energy must be absorbed from the body for the particles of the liquid to gain enough kinetic energy to escape into the gas phase. Hot, arid desert air has a lower partial pressure of water vapor than humid, tropical air, so sweat vaporizes more readily in the dry air than it does in the humid air. Accordingly, most people will feel more comfortable in dry heat than in humid heat.
When substances of different temperatures are brought into thermal contact with each other—that is, some physical arrangement that allows heat transfer—energy will move from the warmer substance to the cooler substance. When a substance undergoes an endothermic or exothermic reaction, heat energy will be exchanged between the system and the environment.
The process of measuring transferred heat is called calorimetry. Two basic types of calorimetry include constant-pressure calorimetry and constant-volume calorimetry. The coffee-cup calorimeter, introduced at the beginning of this chapter, is a low-tech example of a constant-pressure calorimeter, while a bomb calorimeter is an example of a constant-volume calorimeter.
Mnemonic
The equation for heat transfer, given a specific heat, is the same as the test you’re studying for! q = mcΔT looks a lot like “q equals MCAT.”
The heat (q) absorbed or released in a given process is calculated via the equation:
q = mcΔT
Equation 7.2
where m is the mass, c is the specific heat of the substance, and ΔT is the change in temperature (in Celsius or kelvins). Specific heat is defined as the amount of energy required to raise the temperature of one gram of a substance by one degree Celsius (or one kelvin). Specific heat values will generally be provided on Test Day, but one constant to remember is the specific heat of ![]()
Real World
When walking barefoot, a blacktop feels much hotter than a wooden walkway even when they are the same temperature. This is because they have different specific heats.
It requires less heat to raise the temperature of a glass of water the same amount as a swimming pool. While these two items have the same specific heat, c, they have different heat capacities—the product mc (mass times specific heat).
CONSTANT-PRESSURE AND CONSTANT-VOLUME CALORIMETRY
To picture the setup of a constant-pressure calorimeter, just think of the coffee-cup calorimeter: an insulated container covered with a lid and filled with a solution in which a reaction or some physical process, such as dissolution, is occurring. The incident pressure, which is atmospheric pressure, remains constant throughout the process, and the temperature can be measured as the reaction progresses. There should be sufficient thermal insulation (such as Styrofoam) to ensure that the heat being measured is an accurate representation of the reaction, without gain or loss of heat to the environment. Other commercial applications of these same principles include home insulation, padded clothing, and certain food containers such as thermoses.
Real World
Tests looking at plasma proteins or cancer diagnostics in medicine have utilized differential scanning calorimetry (DSC), which is a constant-pressure device, to identify blood components. Such results have shown that thermal properties of major plasma proteins are altered from early- to late-stage tumors.
The term bomb calorimeter may sound rather ominous, but a more accurate descriptive term is decomposition vessel. This better reflects what is actually taking place in constant-volume calorimetry. As shown in Figure 7.6, a sample of matter, typically a hydrocarbon, is placed in the steel decomposition vessel, which is then filled with almost pure oxygen gas. The decomposition vessel is then placed in an insulated container holding a known mass of water. The contents of the decomposition vessel are ignited by an electric ignition mechanism. The material combusts (burns) in the presence of the oxygen, and the heat that evolves is the heat of the combustion reaction. Because W = PΔV, no work is done in an isovolumetric process (ΔV = 0), so Wcalorimeter = 0. Furthermore, because of the insulation, the whole calorimeter can be considered isolated from the rest of the universe, so we can identify the system as the sample plus the oxygen and steel vessel, and the surroundings as the water.
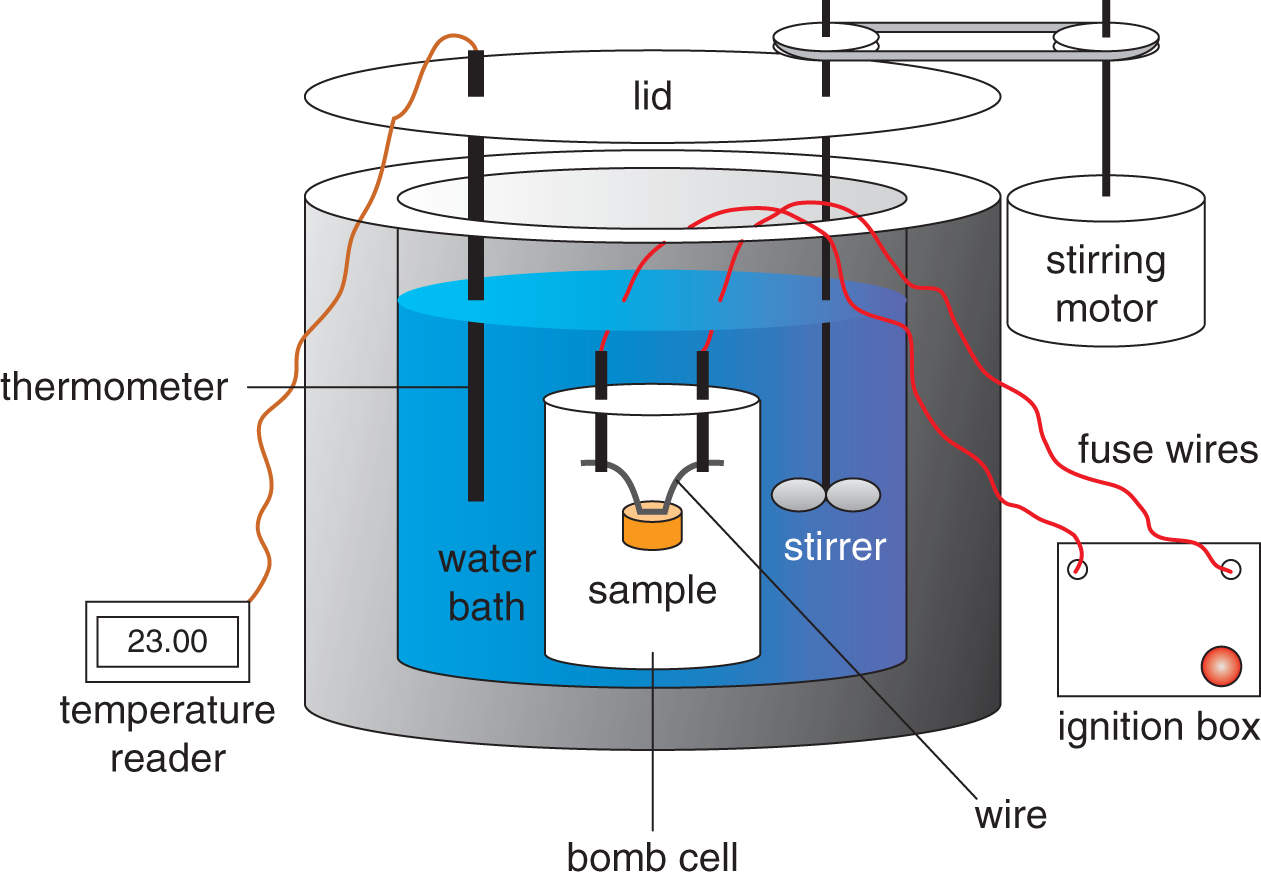 Figure 7.6. Diagram of a Bomb Calorimeter
Figure 7.6. Diagram of a Bomb Calorimeter
Real World
Bomb calorimeters have helped elucidate the thermodynamic properties of various chemical compounds, including food additives, to determine nutritional value (the caloric content of the additive).
Because no heat is exchanged between the calorimeter and the rest of the universe, Qcalorimeter is 0. So,
ΔUsystem + ΔUsurroundings = ΔUcalorimeter = Qcalorimeter — Wcalorimeter = 0
Therefore,
ΔUsystem = —ΔUsurroundings
and because no work is done,
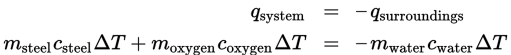
Note that by using the layer of insulation to isolate the entire calorimeter from the rest of the universe, we’ve created an adiabatic process. This means that no heat is exchanged between the calorimeter and the rest of the universe, but it is exchanged between the steel decomposition vessel and the surrounding water. As the previous derivation shows, heat exchange between the system and its surroundings makes it possible for us to calculate the heat of combustion.
MCAT Expertise
Knowing that heat can transfer energy from a system to the surroundings is a key concept tested on calorimetry questions. Whenever asked about equilibrium questions regarding the final temperature of a two-liquid (or liquid—solid) system, remember that the colder object gains thermal energy and the hotter object loses it. You should instinctively realize that a metal bar at 1000 K is hotter than a bath of water at 298 K even though water has a high specific heat. Thus, set up the equation as qcold = —qhot. This form of the equation avoids the pesky sign notation issues in the ΔT equation encountered in most general chemistry texts.
Example:
One cup containing 100 grams of water at 300 K is mixed into another cup containing 200 g of water at 450 K. What is the equilibrium temperature of the system? (Note: Assume that the pressure is sufficiently high to avoid boiling.)
Solution:
The two liquids undergo thermal exchange; thus, the heat given off by one liquid will be equal to the heat absorbed by the other.

Now plug in the values from the question. Because we are solving for final (equilibrium) temperature of a mixture, we can use any value of c so long as we are consistent for both liquids (in this case we have two quantities of water and will use ![]() ).
).
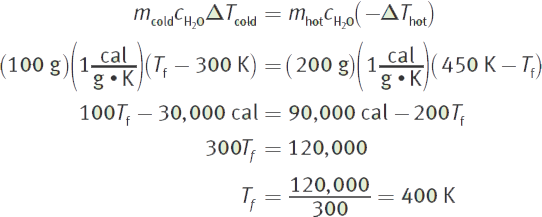
HEATING CURVES
When a compound is heated, the temperature rises until the melting or boiling point is reached. Then, the temperature remains constant as the compound is converted to the next phase (liquid or gas, respectively). Once the entire sample is converted, then the temperature begins to rise again. This is depicted in the heating curves in Figure 7.7.
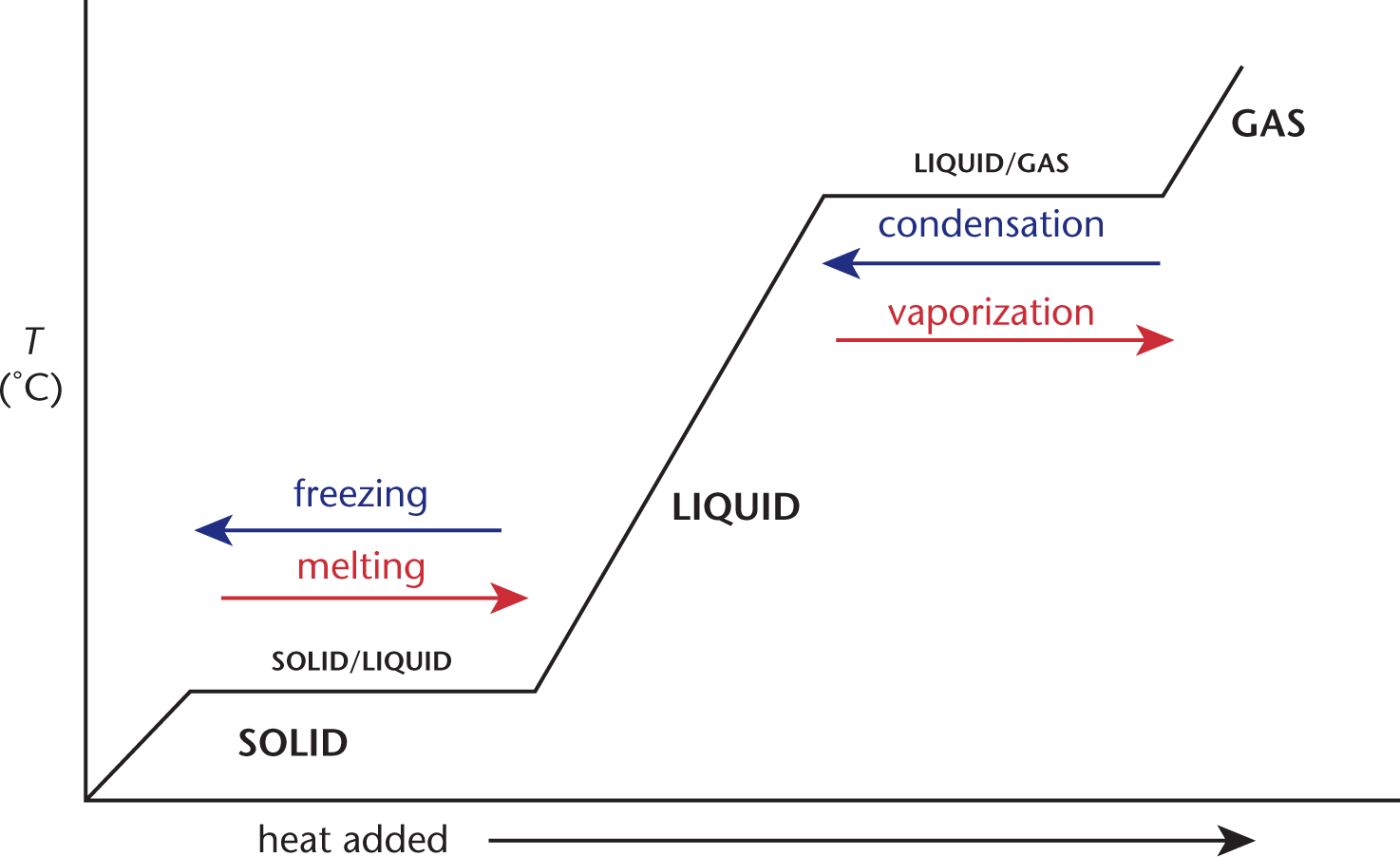 Figure 7.7. Heating Curve for a Single Compound
Figure 7.7. Heating Curve for a Single Compound
Heating curves show that phase change reactions do not undergo changes in temperature. For this reason, we cannot use q = mcΔT during this interval because ΔT = 0. We know intuitively that heat must continue to be added in order for the whole solid to melt, so where does this heat go? The solid absorbs energy, which allows particles to overcome the attractive forces that hold them in a rigid, three-dimensional arrangement. When melting an ice cube, all of the heat added during the process is used to overcome the intermolecular forces between water molecules in ice, forming liquid water. Once all of the ice has been turned into liquid water, the temperature of the liquid water can then increase again. The converse is also true: removing heat from a liquid at the solid—liquid phase transition temperature will cause the formation of a rigid lattice of water molecules.
During phase changes, we must use values based on enthalpy. When transitioning at the solid—liquid boundary, the enthalpy (or heat) of fusion (ΔHfus) must be used to determine the heat transferred during the phase change. When transitioning from solid to liquid, the change in enthalpy will be positive because heat must be added; when transitioning from a liquid to a solid, the change in enthalpy will be negative because heat must be removed. At the liquid—gas boundary, the enthalpy (or heat) of vaporization (ΔHvap) must be used, and its sign convention also follows a similar pattern. These are utilized in the equation
q = mL
Equation 7.3
where m is the mass and L is the latent heat, a general term for the enthalpy of an isothermal process, given in the units ![]()
Key Concept
We need a different formula to calculate q during phase changes when ΔT = 0. If we used q = mcΔT, we’d erroneously think q = 0.
The total amount of heat needed to cross multiple phase boundaries is simply a summation of the heats for changing the temperature of each of the respective phases and the heats associated with phase changes.
Example:
What amount of energy is required to change a 90 gram ice cube at —10°C to vapor at 110°C? (Note: ![]()
![]()
![]()
![]()
![]() )
)
Solution:
Some of the constants given are in terms of mass (g), and some are in terms of moles, so we should convert the mass (90 g) to moles:
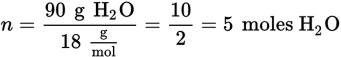
Because we are beginning in the ice phase, we must heat the ice cube to the solid—liquid phase transition, which occurs at 0°C. This first step involves a change in temperature, so we must use the heat formula that contains ΔT and all the pertinent variables for ice (solid water). Also, it is important to match all results in terms of J and kJ for the different steps of the calculation.
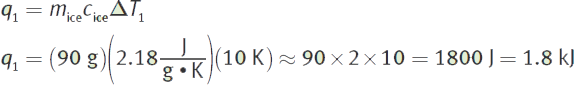
In step 2, we must convert the ice into liquid form. During this phase change, there will be no temperature change.
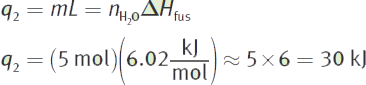
In step 3, we heat the water to its liquid—gas phase transition temperature at 100°C.
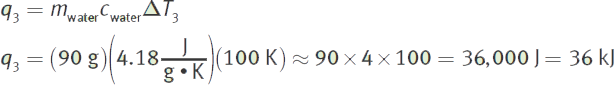
In step 4, we vaporize the water. Again, no temperature change will occur during this phase change.
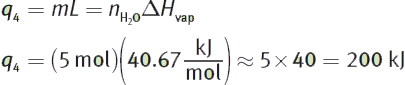
In step 5, we must finally heat the water to the target temperature of 110°C.
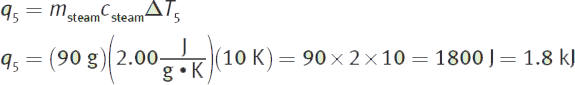
The total heat required for this whole phase change from beginning to end is:
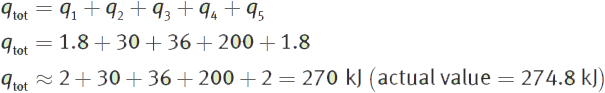
A question this involved is unlikely to be seen on the MCAT because so many steps must be calculated. However, understanding the significance and rationale of this calculation is definitely within the scope of the test.
MCAT Expertise
It is not in your best interest to memorize all the possible values for c and ΔH for Test Day. The MCAT will provide constants as needed, especially if the system is not water. That being said, practicing with heat calculations for water solutions and gaining familiarity with the heat capacities of water will help on Test Day.
MCAT Concept Check 7.3:
Before you move on, assess your understanding of the material with these questions.
1. Contrast temperature and heat.
o Temperature:
o Heat:
2. Contrast specific heat and heat capacity.
o Specific heat:
o Heat capacity:
3. Contrast constant-volume and constant-pressure calorimetry.
o Constant-volume:
o Constant-pressure:
4. What is the specific heat of liquid water (in calories)?