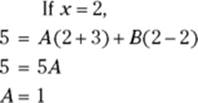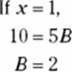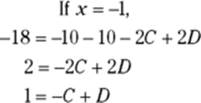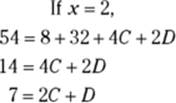Calculus For Dummies, 2nd Edition (2014)

Part V. Integration and Infinite Series

Chapter 16. Integration Techniques for Experts

IN THIS CHAPTER

Breaking down integrals into parts and finding trigonometric integrals

Returning to your roots with SohCahToa

Understanding the As, Bs, and Cs of partial fractions

LIATE: Lilliputians In Africa Tackle Elephants

I figure it wouldn’t hurt to give you a break from the kind of theoretical groundwork stuff that I lay on pretty thick in Chapter 15, so this chapter cuts to the chase and shows you just the nuts and bolts of several integration techniques. In Chapter 15, you saw three basic integration methods: the reverse rules, the guess-and-check method, and substitution. Now you graduate to four advanced techniques: integration by parts, trigonometric integrals, trigonometric substitution, and partial fractions. Ready?

Integration by Parts: Divide and Conquer

Integrating by parts is the integration version of the product rule for differentiation. Just take my word for it. The basic idea of integration by parts is to transform an integral you can’t do into a simple product minus an integral you can do. Here’s the formula:

Integration by parts formula:

And here’s a memory aid for it: In the first two chunks, and uv, the u and v are in alphabetical order. If you remember that, you can remember that the integral on the right is just like the one on the left, except the u and v are reversed.

Don’t try to understand the formula yet. You’ll see how it works in a minute. And don’t worry about understanding the first example below until you get to the end of it. The integration by parts process may seem pretty convoluted your first time through it, so you’ve got to be patient. After you work through a couple examples, you’ll see it’s really not that bad at all.

The integration by parts box: The integration by parts formula contains four things: u, v, du, and dv. To help keep everything straight, organize your problems with a box like the one in Figure 16-1.

FIGURE 16-1: The integration by parts box.

For our first example, let’s do . The integration by parts formula will convert this integral, which you can’t do directly, into a simple product minus an integral you’ll know how to do. First, you’ve got to split up the integrand into two chunks — one chunk becomes the u and the other the dv that you see on the left side of the formula. For this problem, the will become your u chunk. Then everything else is the dv chunk, namely . (  In the next section, I show you how to decide what goes into the u chunk; then, whatever is left over is automatically the dv chunk.) After rewriting the above integrand, you’ve got the following for the left side of the formula:

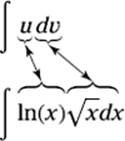
Now it’s time to do the box thing. For each new problem, you should draw an empty four-square box, then put your u (  in this problem) in the upper-left square and your dv ( in this problem) in the lower-right square. See Figure 16-2.

FIGURE 16-2: Filling in the box.

Next, you differentiate u to get your du, and you integrate dv to get your v. The arrows in Figure 16-2 remind you to differentiate on the left and to integrate on the right. Think of differentiation — the easier thing — as going down (like going downhill), and integration — the harder thing — as going up (like going uphill).

Now complete the box:

Figure 16-3 shows the completed box.

FIGURE 16-3: The completed box for

You can also use the four-square box to help you remember the right side of the integration-by-parts formula: start in the upper-left square and draw (or just picture) a number 7 going straight across to the right, then down diagonally to the left. See Figure 16-4.

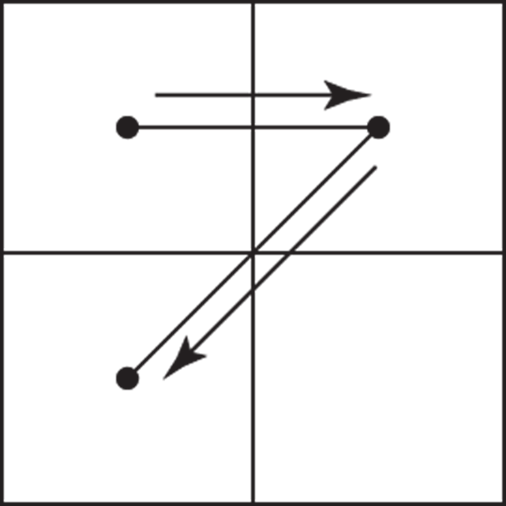
FIGURE 16-4: A box with a 7 in it. Who says calculus is rocket science?

Remembering how you “draw” the 7, look back to Figure 16-3. The right side of the integration-by-parts formula tells you to do the top part of the 7, namely minus the integral of the diagonal part of the 7, . By the way, all of this is much easier to do than to explain. Try it. You’ll see how this four-square-box scheme helps you learn the formula and organize these problems.

Ready to finish? Plug everything into the formula:

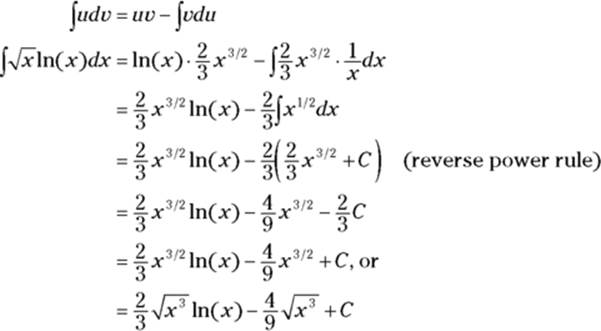
In the last step, you replace the with C because times any old number is still just any old number.

Picking your u

Here’s a great mnemonic device for how to choose your u chunk (again, once you’ve selected your u, everything else is automatically the dv chunk).

The LIATE mnemonic: Herbert E. Kasube came up with the acronym LIATE to help you choose your u (calculus nerds can check out Herb’s article in the American Mathematical Monthly 90, 1983 issue):

To pick your u chunk, go down this list in order; the first type of function on this list that appears in the integrand is the u.

Here are some helpful hints on how to remember the acronym LIATE. How about Let’s Integrate Another Tantalizing Example. Or maybe you prefer Lilliputians In Africa Tackle Elephants, or Lulu’s Indigo And Turquoise Earrings. The last one’s not so good because it could also be Lulu’s Turquoise And Indigo Earrings — whoops: Now you’ll never remember it.

Here’s an example. Integrate . (Note, integration by parts sometimes works for integrands like this one that contain a single function.)

1. Go down the LIATE list and pick the u.

You see that there are no logarithmic functions in , but there is an inverse trigonometric function, . So that’s your u. Everything else is your dv, namely, plain old dx.

2. Do the box thing.

See Figure 16-5 (and see Table 15-2 for the derivative of ).

3. Plug everything into the integration by parts formula or just draw the imaginary 7 in the box on the right in Figure 16-5.

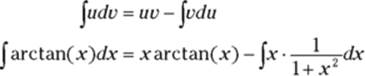
Now you can finish this problem by integrating with the substitution method, setting . Try it (see Chapter 15 for more on the substitution method). Note that the u in has nothing to do with the integration-by-parts u. Your final answer should be .

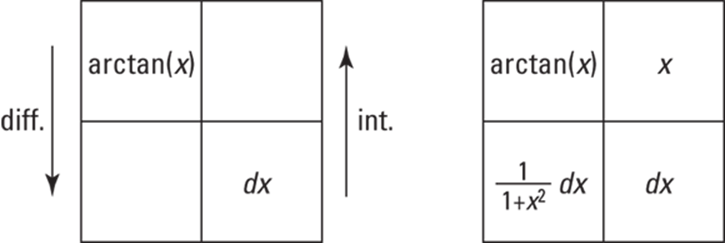
FIGURE 16-5: The box thing.

Here’s another one. Integrate :

1. Go down the LIATE list and pick the u.

Going down the LIATE list, the first type of function you find in is a very simple algebraic one, namely x, so that’s your u. Everything else is your dv.

2. Do the box thing.

See Figure 16-6.

3. Plug everything into the integration by parts formula or draw an imaginary 7 over the box on the right in Figure 16-6.

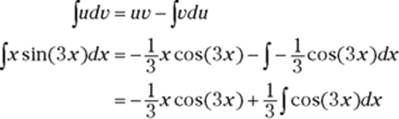
You can easily integrate with substitution or the guess-and-check method. Go for it. Your final answer: .

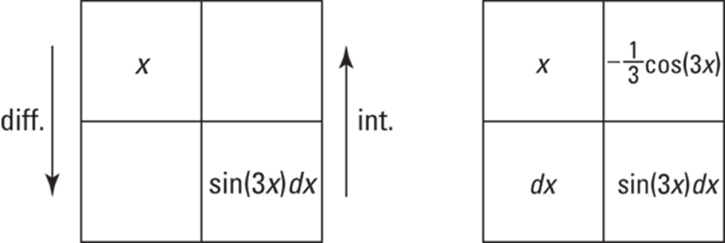
FIGURE 16-6: Yet more boxes.

Integration by parts: Second time, same as the first

Sometimes you have to use the integration by parts method more than once because the first run through the method takes you only partway to the answer. Here’s an example. Find :

1. Go down the LIATE list and pick the u.

contains an algebraic function, , and an exponential function, . (It’s an exponential function because there’s an x in the exponent.) The first on the LIATE list is , so that’s your u.

2. Do the box thing.

See Figure 16-7.

3. Use the integration by parts formula — or the “7” mnemonic.

You end up with another integral, , that can’t be done by any of the simple methods — reverse rules, guess and check, and substitution. But note that the power of x has been reduced from 2 to 1, so you’ve made some progress. When you use integration by parts again for , the x disappears entirely and you’re done. Here goes:

4. Integrate by parts again.

I’ll let you do most of this one on your own. Here’s the final step:

5. Take the result from Step 4 and substitute it for the in the answer from Step 3 to produce the whole enchilada.

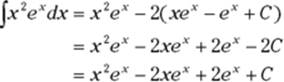
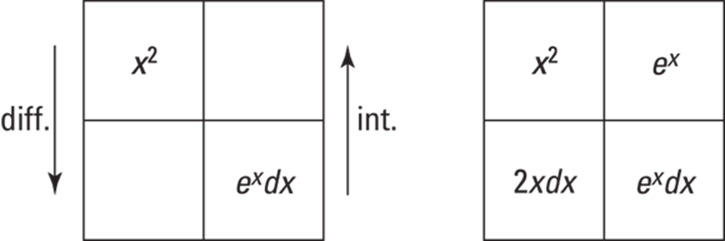
FIGURE 16-7: The boxes for

Tricky Trig Integrals

In this section, you integrate powers of the six trigonometric functions, like and , and products or quotients of different trig functions, like and . This is pretty tedious — time to order up a double espresso.

To use the following techniques, you must either have an integrand that contains just one of the six trig functions like or a certain pairing of trig functions, like . If the integrand has two trig functions, the two must be one of these three pairs: sine with cosine, secant with tangent, or cosecant with cotangent. If you have an integrand containing something other than one of these three pairs, you can easily convert the problem into one of these pairs by using trig identities like and . For instance,

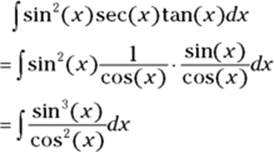
After doing any needed conversions, you want to get one of the following three cases:

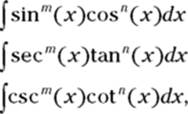
where either m or n (or both) is a positive integer.

The basic idea with most of the following trig integrals is to organize the integrand so that you can make a handy u-substitution and then integrate with the reverse power rule. You’ll see what I mean in a minute.

Integrals containing sines and cosines

This section covers integrals with — can you guess? — sines and cosines.

Case 1: The power of sine is odd and positive

If the power of sine is odd and positive, lop off one sine factor and put it to the right of the rest of the expression, convert the remaining sine factors to cosines with the Pythagorean identity, and then integrate with the substitution method where .

The Pythagorean identity: The Pythagorean identity tells you that, for any angle x, . And thus and .

Now integrate :

1. Lop off one sine factor and move it to the right.

2. Convert the remaining sines to cosines using the Pythagorean identity and simplify.

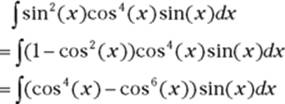
3. Integrate with the substitution method, where .

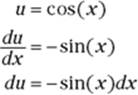
Shortcut for the u-substitution integration method. You can save a little time in all substitution problems by just solving for du — as I did here — and not bothering to solve for dx. You then tweak the expression inside integral so that it contains the thing du equals and compensate for that tweaking by adding something outside the integral. In the current problem, du equals . The integral contains a , so you multiply it by to turn it into and then compensate for that by multiplying the whole integral by . This is a wash because times equals 1. This may not sound like much of a shortcut, but it’s a good time saver once you get used to it.

So tweak your integral:

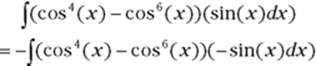
Now substitute and solve by the reverse power rule:

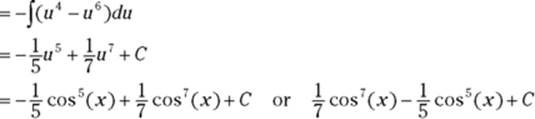
Case 2: The power of cosine is odd and positive

This problem works exactly like Case 1, except that the roles of sine and cosine are reversed. Find .

1. Lop off one cosine factor and move it to the right.

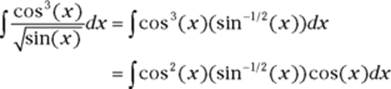
2. Convert the remaining cosines to sines with the Pythagorean identity and simplify.

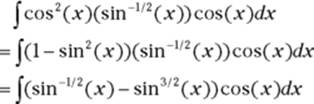
3. Integrate with substitution, where .

Now substitute:

And finish integrating as in Case 1.

Case 3: The powers of both sine and cosine are even and nonnegative

Here you convert the integrand into odd powers of cosines by using the following trig identities.

Two handy trig identities:

Then you finish the problem as in Case 2. Here’s an example:

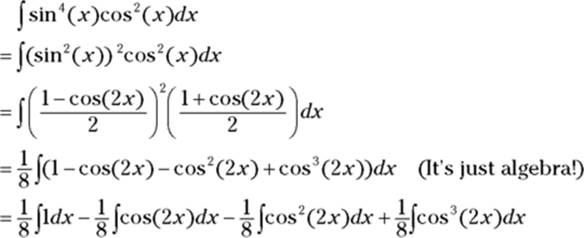
The first in this string of integrals is a no-brainer; the second is a simple reverse rule with a little tweak for the 2; you do the third integral by using the identity a second time; and the fourth integral is handled by following the steps in Case 2. Do it. Your final answer should be

A veritable cake walk.

Don’t forget your trig identities. If you get a sine-cosine problem that doesn’t fit any of the three cases discussed above, try using a trig identity like or to convert the integral into one you can handle.

For example, doesn’t fit any of the three sine-cosine cases, but you can use the Pythagorean identity to convert it to . This splits up into , and the rest is easy. Try it. See whether you can differentiate your result and arrive back at the original problem.

Integrals containing secants and tangents or cosecants and cotangents

The method for solving integrals containing the secant-tangent pairing or the cosecant-cotangent pairing is very similar to the method used for the sine-cosine problems. For examples, check out the trig integrals article at dummies.com.

Your Worst Nightmare: Trigonometric Substitution

With the trigonometric substitution method, you can do integrals containing radicals of the following forms: and (as well as powers of those roots), where a is a constant and u is an expression containing x. For instance, is of the form .

You’re going to love this technique … about as much as sticking a hot poker in your eye.

Desperate times call for desperate measures. Consider pulling the fire alarm on the day your teacher is presenting this topic. With any luck, your teacher will decide that he can’t afford to get behind schedule and he’ll just omit this topic from your final exam.

Before I show you how trigonometric substitution works, I’ve got some silly mnemonic tricks to help you keep the three cases of this method straight. (Remember, with mnemonic devices, silly (and vulgar) works.) First, the three cases involve three trig functions, tangent, sine, and secant. Their initial letters, t, s, and s, are the same letters as the initial letters of the name of this technique, trigonometric substitution. Pretty nice, eh?

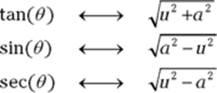
Table 16-1 shows how these three trig functions pair up with the radical forms listed in the opening paragraph.

TABLE 16-1 A Totally Radical Table

To keep these pairings straight, note that the plus sign in looks like a little t for tangent, and that the other two forms, and , contain a subtraction sign — s is for sine and secant. To memorize what sine and secant pair up with, note that begins with the letter a, and it’s a sin to call someone an ass. Okay, I admit this is pretty weak. If you can come up with a better mnemonic, use it!

Ready to do some problems? I’ve stalled long enough.

Case 1: Tangents

Find . First, note that this can be rewritten as , so it fits the form where and ; you can see that this pairs up with tangent in Table 16-1.

1. Draw a right triangle — basically a SohCahToa triangle — where equals , which is .

Because you know that (from SohCahToa — see Chapter 6), your triangle should have 3x as O, the side opposite the angle , and 2 as A, the adjacent side. Then, your radical, , or , will automatically be the correct length for the hypotenuse. It’s not a bad idea to confirm this with the Pythagorean theorem, . See Figure 16-8.

2. Solve for x, differentiate, and solve for dx.

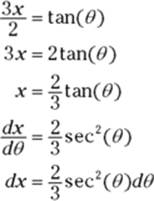
3. Find which trig function is represented by the radical over the a, and then solve for the radical.

Look at the triangle in Figure 16-8. The radical is the hypotenuse and a is 2, the adjacent side, so is , which equals secant. So , and thus .

4. Use the results from Steps 2 and 3 to make substitutions in the original problem and then integrate.

From Steps 2 and 3 you have and . Now you can finally do the integration.

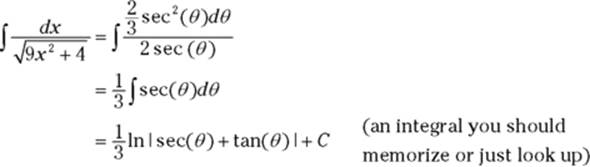
5. Substitute the x expressions from Steps 1 and 3 back in for and . You can also get the expressions from the triangle in Figure 16-8.

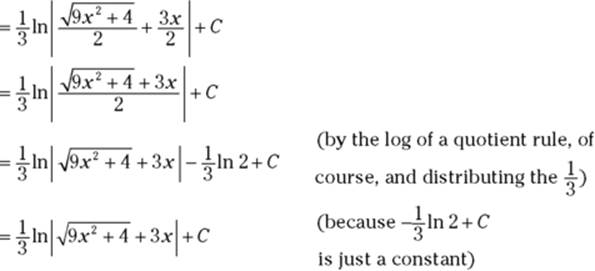
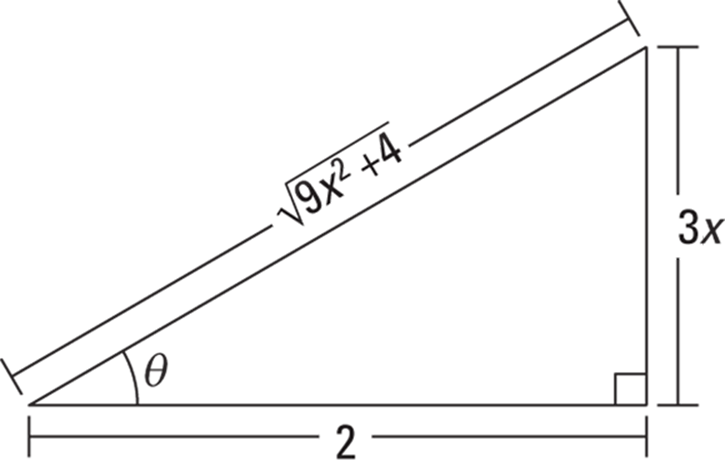
FIGURE 16-8: A SohCahToa triangle for the case. What sinister mind dreamt up this technique?

Now tell me, when was the last time you had so much fun? Before tackling Case 2, here are a couple tips.

Step 1 is . For all three cases in trigonometric substitution, Step 1 always involves drawing a triangle in which the trig function in question equals :

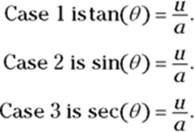
The fact that the u goes in the numerator of this fraction should be easy to remember because u is an expression in x and something like is somewhat simpler and more natural to see than . Just remember the x goes on top.

Step 3 is . For all three cases, Step 3 always involves putting the radical over the a. The three cases are given below, but you don’t need to memorize the trig functions in this list because you’ll know which one you’ve got by just looking at the triangle — assuming you know SohCahToa and the reciprocal trig functions (flip back to Chapter 6 if you don’t know them). I’ve left out what goes under the radicals because by the time you’re doing Step 3, you’ve already got the right radical expression.

In a nutshell, just remember for Step 1 and for Step 3.

Case 2: Sines

Integrate , rewriting it first as  so that it fits the form , where and .

1. Draw a right triangle where , which is .

Sine equals , so the opposite side is x and the hypotenuse is 4. The length of the adjacent side is then automatically equal to your radical, . (Confirm this with the Pythagorean theorem.) See Figure 16-9.

2. Solve for x, differentiate, and solve for dx.

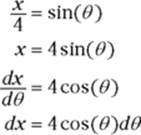
3. Find which trig function equals the radical over the a, and then solve for the radical.

Look at the triangle in Figure 16-9. The radical, , over the a, 4, is , which you know from SohCahToa equals cosine. That gives you

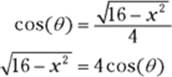
4. Use the results from Steps 2 and 3 to make substitutions in the original problem and then integrate.

Note that you have to make three substitutions here, not just two like in the first example. From Steps 2 and 3 you’ve got

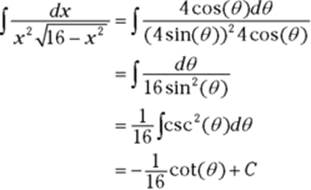
5. The triangle shows that . Substitute back for your final answer.

FIGURE 16-9: A SohCahToa triangle for the case.

It’s a walk in the park.

Case 3: Secants

In the interest of space — and sanity — I’m going to skip this case. But you won’t have any trouble with it because all the steps are basically the same as in Cases 1 and 2.

Try this one. Integrate . I’ll get you started. In Step 1, you draw a triangle, where , that’s . Now take it from there. Here’s the answer (no peeking if you haven’t done it yet): , or , or .

The As, Bs, and Cxs of Partial Fractions

Just when you thought it couldn’t get any worse than trigonometric substitution, I give you the partial fractions technique.

You use the partial fractions method to integrate rational functions like The basic idea involves “unadding” a fraction: Adding works like this: . So, you can “unadd” by splitting it up into plus . This is what you do with the partial fraction technique except that you do it with complicated rational functions instead of ordinary fractions.

Before using the partial fractions technique, you have to check that your integrand is a “proper” fraction — that’s one where the degree of the numerator is less than the degree of the denominator. If the integrand is “improper,” like , you first have to do long polynomial division to transform the improper fraction into a sum of a polynomial (which sometimes will be just a number) and a proper fraction. Here’s the division for this improper fraction. Basically, it works like regular long division:

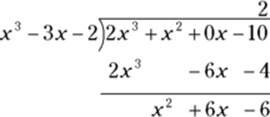
With regular division, if you divide, say, 23 (the dividend) by 4 (the divisor), you get a quotient of 5 and a remainder of 3, which tells you that equals , or . The four pieces in the above polynomial division (the dividend, the divisor, the quotient, and the remainder) work the same way. The quotient is 2 and the remainder is , thus equals . The original problem, therefore becomes . The first integral is just . You would then do the second integral with the partial fractions method. Here’s how it works. First a basic example and then a more advanced one.

Case 1: The denominator contains only linear factors

Integrate . This is a Case 1 problem because the factored denominator (see Step 1) contains only linear factors — in other words, first degree polynomials.

1. Factor the denominator.

2. Break up the fraction on the right into a sum of fractions, where each factor of the denominator in Step 1 becomes the denominator of a separate fraction. Then put capital-letter unknowns in the numerator of each fraction.

3. Multiply both sides of this equation by the left side’s denominator.

This is algebra I, so you can’t possibly want to see the steps, right?

4. Take the roots of the linear factors and plug them — one at a time — into x in the equation from Step 3, and solve for the capital-letter unknowns.

5. Plug these results into the A and B in the equation from Step 2.

6. Split up the original integral into the partial fractions from Step 5 and you’re home free.

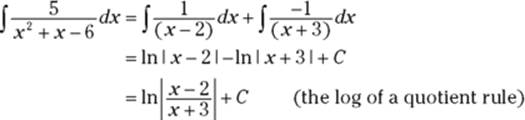
Case 2: The denominator contains irreducible quadratic factors

Sometimes you can’t factor a denominator all the way down to linear factors because some quadratics are irreducible — like prime numbers, they can’t be factored.

Check the discriminant. You can easily check whether a quadratic is reducible or not by checking its discriminant, . If the discriminant is negative, the quadratic is irreducible. If the discriminant is a perfect square like 0, 1, 4, 9, 16, 25, etc., the quadratic can be factored into factors like you’re used to seeing like . This is what happens in a Case 1 problem. The last possibility is that the discriminant equals a non-square positive number, as with the quadratic , for example, that has a discriminant of 96. In that case, the quadratic can be factored, but you get ugly factors involving square roots. You almost certainly will not get a problem like that.

Using the partial fractions technique with irreducible quadratics is a bit different. Here’s a problem: Integrate .

1. Factor the denominator.

It’s already done! Don’t say I never did anything for you. Note that is irreducible because its discriminant is negative.

2. Break up the fraction into a sum of “partial fractions.”

If you have an irreducible quadratic factor (like the ), the numerator for that partial fraction needs two capital-letter unknowns instead of just one. You write them in the form of .

3. Multiply both sides of this equation by the left-side denominator.

4. Take the roots of the linear factors and plug them — one at a time — into x in the equation from Step 3, and then solve.

5. Unlike in the Case 1 example, you can’t solve for all the unknowns by plugging in the roots of the linear factors, so you have more work to do.

6. Plug into the Step 3 equation the known values of A and B and any two values for x not used in Step 4 (low numbers make the arithmetic easier) to get a system of two equations in C and D.

7. Solve the system: and .

You should get and .

8. Split up the original integral and integrate.

Using the values obtained in Steps 4 and 6, , , , and , and the equation from Step 2, you can split up the original integral into three pieces:

And with simple algebra, you can split up the third integral on the right into two pieces, resulting in the final partial fraction decomposition:

The first two integrals are easy. For the third, you use substitution with and . The fourth is done with the arctangent rule, which you should memorize: .

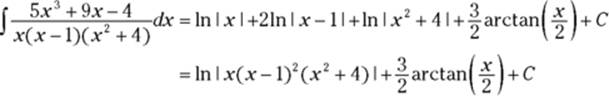
Bonus: Equating coefficients of like terms

Here’s another method for finding the capital-letter unknowns that you should have in your bag of tricks. Say you get the following for your Step 3 equation (this comes from a problem with two irreducible quadratic factors):

This equation has no linear factors, so you can’t plug in the roots to get the unknowns. Instead, expand the right side of the equation:

And collect like terms:

Then equate the coefficients of like terms from the left and right sides of the equation:

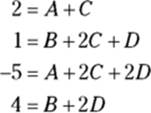
You then solve this system of simultaneous equations to get A, B, C, and D.

How about a shortcut? You can finish the Case 2 example a couple pages back by using a shortcut version of the equating of coefficients method. Once you have the values for A and B from Step 4, you could look back at the equation in Step 3, and equate the coefficients of the term on the left and right sides of the equation. Can you see, without actually doing the expansion, that on the right you’d get   ? So, , which means that , and because (from Step 4), C must equal 2. Then, using these values for A, B, and C, and any value of x (other than 0 or 1), you can get D. How about that for a simple shortcut?

Practice makes perfect. In a nutshell, you have three ways to find your capital-letter unknowns: 1) Plugging in the roots of the linear factors of the denominator if there are any, 2) Plugging in other values of x and solving the resulting system of equations, and 3) Equating the coefficients of like terms. With practice, you’ll get good at combining these methods to find your unknowns quickly.