GMAT Quantitative Review

5.0 Data Sufficiency

Data sufficiency questions appear in the Quantitative section of the GMAT® exam. Multiple-choice data sufficiency questions are intermingled with problem solving questions throughout the section. You will have 75 minutes to complete the Quantitative section of the GMAT exam, or about 2 minutes to answer each question. These questions require knowledge of the following topics:

· Arithmetic

· Elementary algebra

· Commonly known concepts of geometry

Data sufficiency questions are designed to measure your ability to analyze a quantitative problem, recognize which given information is relevant, and determine at what point there is sufficient information to solve a problem. In these questions, you are to classify each problem according to the five fixed answer choices, rather than find a solution to the problem.

Each data sufficiency question consists of a question, often accompanied by some initial information, and two statements, labeled (1) and (2), which contain additional information. You must decide whether the information in each statement is sufficient to answer the question or—if neither statement provides enough information—whether the information in the two statements together is sufficient. It is also possible that the statements in combination do not give enough information to answer the question.

Begin by reading the initial information and the question carefully. Next, consider the first statement. Does the information provided by the first statement enable you to answer the question? Go on to the second statement. Try to ignore the information given in the first statement when you consider whether the second statement provides information that, by itself, allows you to answer the question. Now you should be able to say, for each statement, whether it is sufficient to determine the answer.

Next, consider the two statements in tandem. Do they, together, enable you to answer the question?

Look again at your answer choices. Select the one that most accurately reflects whether the statements provide the information required to answer the question.

5.1 Test-Taking Strategies

1. Do not waste valuable time solving a problem.

You only need to determine whether sufficient information is given to solve it.

2. Consider each statement separately.

First, decide whether each statement alone gives sufficient information to solve the problem. Be sure to disregard the information given in statement (1) when you evaluate the information given in statement (2). If either, or both, of the statements give(s) sufficient information to solve the problem, select the answer corresponding to the description of which statement(s) give(s) sufficient information to solve the problem.

3. Judge the statements in tandem if neither statement is sufficient by itself.

It is possible that the two statements together do not provide sufficient information. Once you decide, select the answer corresponding to the description of whether the statements together give sufficient information to solve the problem.

4. Answer the question asked.

For example, if the question asks, “What is the value of y?” for an answer statement to be sufficient, you must be able to find one and only one value for y. Being able to determine minimum or maximum values for an answer (e.g., y = x + 2) is not sufficient, because such answers constitute a range of values rather than the specific value of y.

5. Be very careful not to make unwarranted assumptions based on the images represented.

Figures are not necessarily drawn to scale; they are generalized figures showing little more than intersecting line segments and the relationships of points, angles, and regions. So, for example, if a figure described as a rectangle looks like a square, do not conclude that it is, in fact, a square just by looking at the figure.

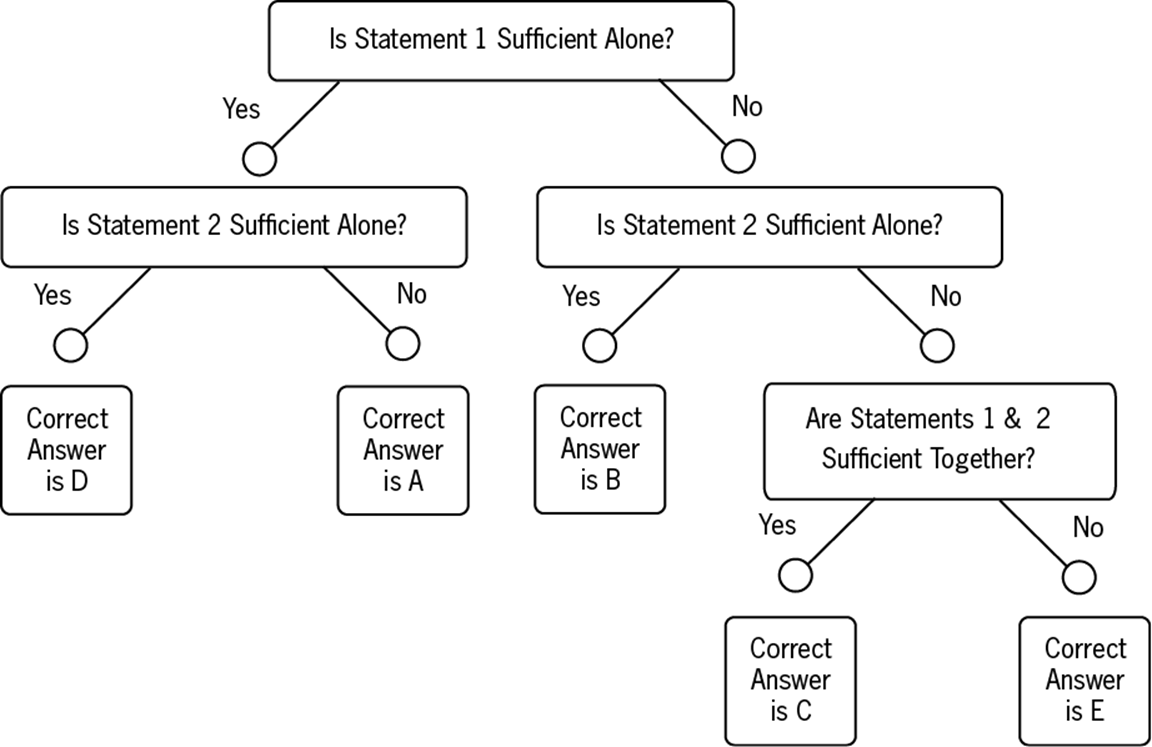
If statement 1 is sufficient, then the answer must be A or D.

If statement 2 is not sufficient, then the answer must be A.

If statement 2 is sufficient, then the answer must be D.

If statement 1 is not sufficient, then the answer must be B, C, or E.

If statement 2 is sufficient, then the answer must be B.

If statement 2 is not sufficient, then the answer must be C or E.

If both statements together are sufficient, then the answer must be C.

If both statements together are still not sufficient, then the answer must be E.