The history of mathematics: A brief course (2013)

Part I. What is Mathematics?

This first part of our history is concerned with the “front end” of mathematics (to use an image from computer algebra)—its relation to the physical world and human society. It contains some general considerations about mathematics, what it consists of, and how it may have arisen. This material is intended as an orientation for the main part of the book, where we discuss how mathematics has developed in various cultures around the world. Because of the large number of cultures that exist, a considerable paring down of the available material is necessary. We are forced to choose a few sample cultures to represent the whole, and we choose those that have the best-recorded mathematical history. The general topics studied in this part involve philosophical and social questions, which are themselves specialized subjects of study, to which a large amount of scholarly literature has been devoted. Our approach here is the naive commonsense approach of an author who is not a specialist in either philosophy or sociology. Since present-day governments have to formulate policies relating to mathematics and science, it is important that such questions not be left to specialists. The rest of us, as citizens of a republic, should read as much as time permits of what the specialists have to say and make up our own minds when it comes time to judge the effects of a policy.

Contents of Part I

1. Chapter 1 (Mathematics and Its History) considers the general nature of mathematics and gives an example of the way it can help to understand the physical world. We also outline a series of questions to be kept in mind as the rest of the book is studied, questions to help the reader flesh out the bare bones in the historical documents.

2. Chapter 2 (Proto-mathematics) studies the mathematical reasoning invented by people in the course of solving the immediate and relatively simple practical problems of administering a government or managing a construction site. In this area we are dependent on archaeologists and anthropologists for the historical information available.

Chapter 1. Mathematics and its History

. . .all histories, to the extent that they contain a system, a drama, or a moral, are so much literary fiction.

Those who cannot remember the past are condemned to repeat it. (Often misquoted as “Those who do not learn from history are doomed to repeat it.”)

George Santayana (1863–1952), Spanish–American philosopher
(born Jorge Agustin Nicolás Ruiz de Santayana y Borrás)

The history of mathematics is a hybrid subject, taking its material from mathematics and history, sometimes invoking other areas such as psychology, political history, sociology, and philosophy to give a detailed picture of the development of mathematics. Obviously, no one can be an expert in all of these areas, and some compromises have to be accepted. Especially in an introductory course, it is often necessary to oversimplify both the mathematics itself and the social and historical context in which it arose so that the most significant portions can be included. No history of the subject that covers more than a narrow band of time can aim for anything like completeness.

1.1 Two Ways to Look at the History of Mathematics

One of the most distinguished historians of mathematics, Ivor Grattan-Guinness (b. 1941), has made a distinction between history and heritage. History asks the question “What happened in the past?” Heritage asks “How did things come to be the way they are?” Obviously, the first of these two questions is more general than the second. Many things happened in the past that had no influence on the current shape of things, not only in mathematics but in all areas of human endeavor, including art, music, and politics. Such events are history, but not heritage. The study of history in this sense is a purely intellectual exercise, not aimed at any applications, nor to teach a moral, nor to make people better citizens. What it does aim at is getting an accurate picture of the past for the edification of those who have a taste for such knowledge. It is difficult to write such a history, as the first epigram from George Santayana given above shows.

Even on the most impersonal, objective level, we don't want the raw, unedited past, which is a raging tsunami of sneezes and hiccups; some judgment is needed to select the events in the past that are of interest. To that extent, Santayana's implication is correct: All history is literary fiction. The danger for the historian lies in trying to frame a particular picture of the past in order to make it tell the story that one personally would like to hear. In the history of mathematics, there is a special danger because the mathematics itself fits together in a very logical way, while the routes by which it has been discovered and developed have all the illogical disorder that is inherent in any process involving human thinking. For example, it is known that there is no finite algebraic formula involving only arithmetic operations and root extractions that will yield a root of every quintic equation when the coefficients of that equation are substituted for its variables. This result follows very neatly from what we now call Galois theory, after Evariste Galois (1811–1832), who first introduced its basic ideas. It is nowadays always proved using this technique. But the theorem was first stated and given a semblance of a proof by Paolo Ruffini (1765–1822) and Niels Henrik Abel (1802–1829), neither of whom knew Galois theory. They both proceeded by counting the number of different values that such a hypothetical formula would generate if all possible values were substituted in the formula for each nth root it contains. This example is typical of many cases in the history of mathematics, where the proof of a proposition resulted not from rigorously arranged steps following in logical order from one another, but from a number of independent ideas gradually coming into focus.

1.1.1 History, but not Heritage

During the fifteenth and sixteenth centuries, tables of sines were used to simplify multiplication and reduce it to addition and subtraction. This procedure was called prosthaphæresis, from the Greek words prosthairesis (πρoσθα´ιρεσις), meaning taking toward, and aphairesis (α^,ϕα ´ιρεσις), meaning taking away. This technique disappeared almost without a trace after the discovery of logarithms in the early seventeenth century, and it is nowadays unknown even to most professional mathematicians. Nevertheless, it was an important idea in its time and deserves to be remembered. We shall take the time to discuss it and practice it a bit. As we shall see, it is actually more efficient than logarithms for computing the formulas of spherical trigonometry.

1.1.2 Our Mathematical Heritage

The appeal of history is to a person of a particular “antiquarian” bent of mind. Heritage, which is parasitic upon history, has a somewhat more practical aim: to help us understand the world that we ourselves live in. This is the “useful” part of history that historians advertise to the public to gain support, and it is the point of view expressed in the second of Santayana's epigrams at the beginning of this lecture. (Notice that the two epigrams taken together imply that the human race needs a variety of history that is actually literary fiction.)

If you have taken a course called “modern algebra,” for example, you found yourself confronted with a collection of abstract objects—groups, rings, fields, vector spaces—that seemed to have nothing in common with high-school algebra except that they required the use of letters. How did these abstract subjects come to be referred to as algebra? By tracing the story of the unsolvability of the general equation of degree five, we can answer this question.

After algebraic formulas were found for solving equations of degree 3 and 4 in the sixteenth century, two centuries were spent in the quest for a mathematical “Holy Grail,” an algebraic formula to solve the general equation of degree 5. Some people thought they had succeeded; but in the late eighteenth and early nineteenth centuries, Ruffini, Abel (one of those who for a time thought he had succeeded in finding the formula), and William Rowan Hamilton (1805–1865) were able to show that no such formula could exist. The question then arose of determining which equations could be solved by algebraic operations (the operations of arithmetic, together with the extraction of roots) and which could not. The answer to this question, as shown by Galois, depends on the abstract nature of a certain set of permutations of the roots. This was the beginning of the study of groups, a word first used by Galois. The concept of an abstract group arose some decades later, along with the rest of these abstract creations, all of which found numerous applications in other areas of mathematics. The original problem that gave rise to much of this modern algebra was, in the end, only one part of the vast edifice of modern algebra.

1.2 The Origin of Mathematics

The farther we delve into the past, the more we find mathematics entangled with accounting, surveying, astronomy, and the general administration of empires. Mathematics arises wherever people think about the physical world or about the world of ideas embodied in laws and even theology. It grows like a plant, from a seed that germinates and later ramifies to produce roots, branches, leaves, flowers, and fruit. It is constantly growing.

1.2.1 Number

It seems nearly certain that the small positive integers, the kinds of numbers that are intuitively known to everyone, are the “seed” of mathematics. Essentially all mathematical concepts can be traced ultimately to the use of numbers to explain the world. Numbers seem to be a universal mode of human thought. They were probably used originally in a kind of informal accounting, when it was necessary to keep track of objects that could be regarded as interchangeable, such as the cattle in a herd. Through anthropology, archaeology, and written texts, we can trace a general picture of arithmetical progress in handling such discrete collections, from counting, through computation, and finally to abstract number theory. Many different cultures have shown a convergent development in this area, although in the final stage there is considerable variety in the choice of topics developed. Through this history, we shall gradually introduce the properties of numbers in the chapters that follow. At the moment, we take note of just one important property that they have, namely that discrete collections can be exactly equal: If I have $9845.63 in my checking account, and you have $9845.63 in your checking account, then we have exactly the same amount of money, for all financial purposes whatsoever. When you count—votes, pennies, or attendance at a football game—it is at least theoretically possible to get the outcome exactly right, with no error at all.

1.2.2 Space

While discrete collections are naturally handled through counting, nature presents us with the need to measure quantities that are continuous rather than discrete, quantities such as length, area, volume, weight, time, and speed. Number is invoked to solve such problems; but in each case, it is necessary to choose a unit and regard the continuous quantity as if it were a discrete collection of units. Doing so adds a layer of complication, since the unit is arbitrary and culturally dependent. When people from different groups meet and talk about such quantities, they need to reconcile their units.

The essence of continuous quantities is that they can be divided into pieces of arbitrarily small size. A continuous measurement therefore always has precision limited by the size of the unit chosen. Equality of two continuous objects of the same kind is always approximate, only up to the standard unit of measurement in which their sizes are expressed.¹

This distinction between the discrete and the continuous is fundamental in mathematics, and it brings with it many metaphysical and mathematical complications. In particular, the notion of infinite precision, which is required to define what we call “real numbers,” is difficult to visualize and define. In “real-world” applications, computers finesse the problem by replacing real numbers with floating-point numbers. The result is that most engineers and scientists never really have to encounter the difference between the two, and mathematicians who attempt to talk to them tend to forget that they are not really speaking the same language. Some computer algebra programs (Mathematica and Maple, for example) will handle irrational numbers like π and symbolically and will convert them to decimal approximations only when a numerical result is requested by the user.

The ideas needed to handle continuous quantities were first applied to lengths, areas, and volumes. They led to geometry, which arose in many different places as a way of comparing the sizes of objects having different shapes. Like the positive integers, these shapes seem to be a cultural universal, as all over the world we find people discussing triangles, squares, rectangles, circles, spheres, pyramids, and the like. Moreover, the shape of a standard unit area is universally square.

As happens with arithmetic, geometry passes through certain stages in a particular order in many different cultures. The first stage is simply measurement, finding physical ways of counting how many standard units of length, area, or volume there are in a piece of rope, a plot of land, or a ditch that is to be excavated. Soon, the processes of arithmetic are invoked to provide indirect ways of computing areas and volumes. At this stage, the universal shapes named above are isolated for study. Finally, relations among the parts of a geometric figure are studied, leading to abstract geometry, and some way is found to give geometric demonstrations of relations that are not obvious. Here again we find a cultural universal in the Pythagorean theorem, which was apparently discovered in several places independently. Since it invokes the notion of a square, it shows that human imagination is by nature Euclidean.² This third stage occurs in several places, among them Mesopotamia, China, India, and ancient Greece. In addition, the ancient Greeks mixed philosophy and abstract logic into their geometry and number theory, producing a number of long treatises that were unique in their time and became a model for later mathematical writing the world over.

1.2.3 Are Mathematical Ideas Innate?

The cross-cultural constancy of the arithmetic operations and the standard shapes of geometry is a very striking fact and lends some support to the views of the eighteenth-century philosopher Immanuel Kant (1724–1804), who thought mathematical knowledge was “synthetic but a priori.” By that he meant that statements such as 7 + 5 = 12 or that a triangle can be constructed having sides of three given lengths provided the sum of the smaller two exceeds the third (his examples) are not things we learn from observation, like that statement that pandas eat bamboo. Things learned from experience are a posteriori in Kant's language.

Mathematical facts are, as we would now say, “hard-wired” into the human brain, or, as Kant said, a priori (anterior to experience). At the same time, they are “synthetic,” that is, they are not mere tautologies like the statement that two first cousins have a common grandparent. Tautological statements were called analytic by Kant, meaning that the very definition of first cousinhood involves a common grandparent, but (Kant said) the notions of 7, 5, and addition do not by their nature involve the number 12. We shall return to this topic when we discuss logic in Chapter 45.

In the late nineteenth and early twentieth century, a school of philosophy of mathematics arose known as logicism. Its adherents defended the proposition that mathematics could be derived from logic. If they are correct, then Kant's belief that arithmetic and geometric propositions are synthetic must be wrong. It is true that logicists produce a formal proof of the simple fact that twice two make four. In that sense, they have made this proposition analytic rather than synthetic. Still, there is a more colloquial sense of the word proof that is violated in the process. A proof is usually thought of as deriving a proposition that is not obvious from others that are obvious. Unfortunately, the axioms of set theory are very far from being more obvious than the equality 2 + 2 = 4.

1.2.4 Symbolic Notation

We noted above that symbolism entered mathematics via algebra, as the most elegant way of giving a description of an unknown or unspecified number. Eventually, this symbolism conquered number theory and geometry as well, and there is now no branch of mathematics, pure or applied, that is not dominated by symbolic formulas. This tool for thinking is so important that we shall consider it a third ingredient of mathematics, after number and space. Algebra itself, however, got along without symbols for centuries. If algebra is defined as a subject where symbols are used to represent unspecified numbers in equations, then we shall find no algebra at all until a few centuries ago. But the essence of algebra is not in the symbolism, or even in the equation. It is in the process of naming an implicitly defined number, and that will be our definition. Thus, finding a number that yields 24 when squared and added to five times itself is an algebra problem. It need not be stated as the equation x² + 5x = 24.

1.2.5 Logical Relations

The fourth and last ingredient of mathematics is the logical organization of the subject. The strict formalism that we now associate with mathematical theories was first set out in connection with geometry and number theory in ancient Greece. The earliest major work embodying it is Euclid's Elements, which was the model for later work by Greek mathematicians such as Archimedes, Apollonius, Ptolemy, Pappus, and others and became the inspiration for many of the classic treatises of modern mathematics, such as Newton's famous Philosophiæ naturalis principia mathematica (Mathematical principles of natural philosophy).³

1.2.6 The Components of Mathematics

We shall consider mathematics as being made up of the four basic components just described. The first of these can be loosely described as arithmetic,⁴ the second as geometry, the third as algebra, and the fourth as mathematical reasoning.

Out of these four elements arise calculus, probability, statistics, set theory, topology, complex analysis, mathematical logic, and a host of other areas of modern mathematics that make it the magnificent monument to the human intellect that it is.

1.3 The Philosophy of Mathematics

If we were to study merely what happened in the past, even if we did it with an eye toward the present, the development of mathematics would seem very much like one wave after another breaking on the shore. Without some interesting conjectures as to what the creators were trying to do, it would be difficult to make any sense of this history. This problem is particularly acute in algebra, as you may recall from the “story problems” you were asked to solve in high school, which are without exception, colossally useless. Surely the complicated mathematical reasoning in this subject was not invented in order to find out when two trains will meet if they set out from different stations at different times. In order to flesh out the subject and paint it in brighter and more realistic colors, we need to ask ourselves broad philosophical questions while we are studying the past. Here is a short list of questions of interest.

Epistemological Questions (Theory of Knowledge)

1. What is the nature of mathematical objects such as numbers, triangles, probabilities, and functions? In what sense do they “exist”?

2. What can we know about infinite collections of things? Is a finite human mind capable of knowing infinitely many different things?

3. What is meant by continuity? Is it possible to formulate continuity in the discrete symbols of ordinary language?

Metaphysical Questions (Nature of Reality)

1. What is the relation of the objects of pure mathematics to those of applied mathematics? Many logical relations exist in pure mathematics; and when they are applied in real-world situations, they very often make predictions that can be verified by observation. Does that mean that pure-mathematics relations correspond to relations in the physical universe? If so, what is the nature of these physical relations? To paraphrase the Nobel Prize-winning physicist Eugene Wigner (1902–1995), what is the reason for “the unreasonable effectiveness of mathematics” in explaining the world? For a republication of Wigner's 1960 paper on this subject, see the following website:
http://www.dartmouth.edu/~matc/MathDrama/reading/Wigner.html

2. Why do probability and statistics work so well in practice that insurance companies and gambling casinos can rely on the seeming chaos of random events to stay predictable “in the large”? For that matter, why do we make the assumption that the future will resemble the past, so that we can make mathematical predictions about the future state of the physical universe?

Metamathematical Questions

1. The business of the pure mathematician is to prove theorems, that is, to make valid inferences from premises. What premises should be allowed, and what rules of inference can be trusted? There is a school of mathematicians—the intuitionists—that refuses to use certain basic logical and mathematical assumptions, chiefly the law of excluded middle (if not-A is false, then A is true) or the axiom of choice, which says intuitively that if you have a collection of containers and each one has something inside it, you can reach in and take one object out of each. The collections obtained in this way are the elements of a new set called the Cartesian product of the containers in the original collection.) Most mathematicians use these two principles freely and have no qualms about doing so.

2. How important is a formal, deductive presentation of a mathematical subject? Can a mathematical paper that appeals to intuition rather than formal proof be accepted as valid?

Sociological Questions

1. How important is mathematics to society? What genuine material or moral progress in the world can be traced to the activity of mathematicians?

2. What mathematics, if any, should be taught to every citizen of a modern democracy?

1.3.1 Mathematical Analysis of a Real-World Problem

We shall illustrate just one of the ways in which this course is intended to make you think about the mathematical link between the physical world around us and our thinking processes. We choose music as an example. On April 17, 1712, the philosopher–mathematician Leibniz (1646–1716) wrote to Christian Goldbach (1690–1764)

Musica est exercitium arithmeticæ occultum nescientis se numerare animi. (Music is a mysterious practicing of the numerical art by a mind that does not realize it is counting.)

(See Epistolæ ad diversos, edited by Christian Kortholt, Vol. 1, Leipzig, 1734, p. 241, Letter CLIV.)

What Leibniz probably meant in this aphorism is that the rhythm patterns that are part of all music can be analyzed and found to be regular repetitions of simple periodic patterns, superimposed in some very complex ways. He may also have suspected that the pitch and quality of the notes coming from string and wind instruments can be analyzed in the same way. The rhythm is a case of a discrete phenomenon, while the pitch involves continuous periodic waves. It is possible to represent the pitch and the overtones of musical instruments as superimposed simple sine waves of various frequencies, amplitudes, and phases. From those sine waves, it is not only theoretically but also practically possible (as we now know) to synthesize music. Theoretically, one can play an entire orchestral symphony with nothing but tuning forks struck with the proper strength and at the proper times. We shall illustrate the underlying principle with a simple discrete example, leaving the more complicated continuous case for later description.

If you can read music, try tapping out the rhythm pattern depicted in Fig. 1.1. If you cannot, ask someone who can read music to do this for you.

Figure 1.1 A rhythm pattern compounded of two simple periodic beats.

You will find that you can duplicate this rhythm pattern if you count by twelves, bringing both hands down on the count of 1, then alternating right and left hands on 4, 5, 7, 9, and 10. In this way, the left hand can be tapping out ONE-two-three-FOUR-five-six-SEVEN-eight-nine-TEN-eleven-twelve, while the right hand is tapping ONE-two-three-four-FIVE-six-seven-eight-NINE-ten-eleven-twelve. In other words, the left hand is tapping four beats to the bar while the right hand taps three beats to the bar. Each hand is tapping a simple periodic pulse every three beats or every four beats. The combined effect is the pattern ONE-two-three-FOUR-FIVE-six-SEVEN-eight-NINE-TEN-eleven-twelve, which sounds very syncopated. Imagine this example elaborated to describe a whole orchestra, and extended to the pitch of the tones each instrument is producing, and you get an idea of the complexity of music when it is analyzed mathematically. Musical patterns, however, are felt by musicians; they are not produced mechanically, at least not by good musicians. The quickest way to master this rhythm pattern is simply to hear it. Nearly everyone can reproduce it, much more rapidly than anyone can count aloud, after hearing it for a few seconds. That is the point of Leibniz’ comment that the mind does not realize it is counting. Mathematical analysis of tones and rhythms has the same relation to the pleasure of hearing music that chemical analysis of a cup of coffee has to the pleasure of drinking it.

It is not physically possible to play a symphony with tuning forks, and we don't actually do this. But we do an equivalent thing in our digital music. That is the point of the cartoon shown here, which appeared in The New Yorkeron October 4, 2010 (p. 71).

From the mathematician's point of view, digital radio amounts to breaking the sound into a finite set of simple frequencies, each having a particular amplitude and phase. (The amplitude associated with frequency n in the cartoon is .) When a digital radio receives that set of amplitudes and frequencies, it sends them in the form of electrical signals to the speakers, which then reproduce them as an audible signal. Since the human ear “truncates” the signal by being unable to pick up frequencies below 20 cycles per second or higher than 20,000 cycles per second, the result is what mathematicians call a band-limited signal. It is an important mathematical result—the Whittaker–Shannon interpolation theorem, named after Edmund Taylor Whittaker (1873–1956) and Claude Elwood Shannon (1916–2001)—that such a signal can be reproduced perfectly from a finite number of sample points.

The Fourier series of a symphony. Copyright © Jack Ziegler/The New Yorker Collection.

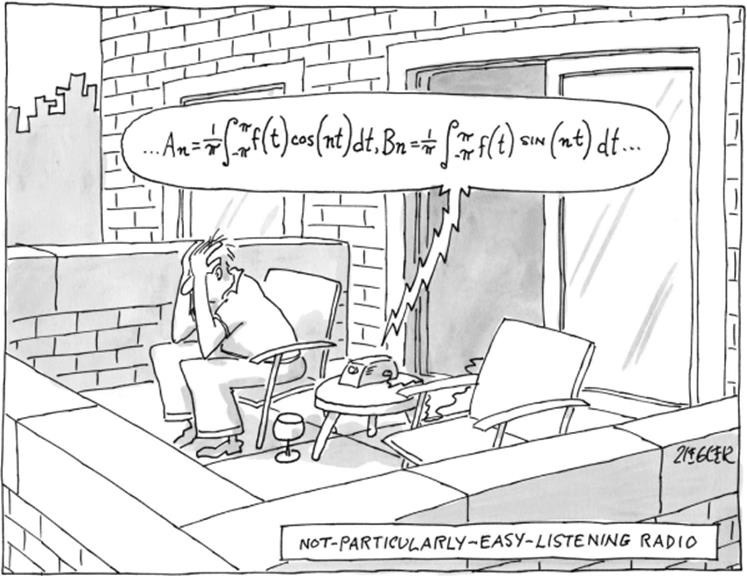
The humor in this cartoon—imagine being given the Fourier series of a symphony and having to do the Fourier inversion in your head in order to interpret the symphony!—is rather esoteric and will be appreciated only by the tiny segment of the population that knows Fourier analysis. For the rest of the public, this cartoon was probably just one more way of saying, “Math is hard.”

1.4 Our Approach to the History of Mathematics

We are going to study the history of mathematics partly for its intrinsic interest. That will lead us to develop a few mathematical skills that will not be of much use outside this course. We do this partly for an ethical reason: to preserve the memory of brilliant people whose contributions to human history should not be forgotten. Except for these excursions into true history, our focus is on the “heritage” aspect of mathematical history. The main aim of this course is to give insight into the way that today's mathematics developed, the motives of its creators, and the social and intellectual context in which they worked. As Santayana said, in so doing, we are to some extent creating literary fiction. But it is useful fiction.

Questions for Reflection

At the end of most of the chapters in this book, there will be a set of mathematical problems testing for understanding of the mathematics discussed in that chapter, followed by a set of questions of historical fact to reinforce the historical narrative of the chapter, in turn followed by a set of questions calling for reflection on the historical and mathematical issues that arise in the chapter. In this introductory chapter, where we have not introduced any mathematics or discussed any systematic development of it, only the third category seems appropriate. The following questions are therefore intended to make you think about general issues such as those raised in the questions listed above.

1.1. In what practical contexts of everyday life are the fundamental operations of arithmetic—addition, subtraction, multiplication, and division—needed? Give at least two examples of the use of each. How do these operations apply to the problems for which the theory of proportion was invented?

1.2. Measuring a continuous object involves finding its ratio to some standard unit. For example, when you measure out one-third of a cup of flour in a recipe, you are choosing a quantity of flour whose ratio to the standard cup is 1 : 3. Suppose that you have a standard cup without calibrations, a second cup of unknown size, and a large bowl. How could you determine the volume of the second cup?

1.3. Units of time, such as a day, a month, and a year, have ratios. In fact you probably know that a year is about days long. Imagine that you had never been taught that fact. How would you—how did people originally—determine how many days there are in a year?

1.4. Why is a calendar needed by an organized society? Would a very small society (consisting of, say, a few dozen families) require a calendar if it engaged mostly in hunting, fishing, and gathering vegetable food? What if the principal economic activity involved following a reindeer herd? What if it involved tending a herd of domestic animals? Finally, what if it involved planting and tending crops?

1.5. Describe three different ways of measuring time, based on different physical principles. Are all three ways equally applicable to all lengths of time?

1.6. In what sense is it possible to know the exact value of a number such as ? Obviously, if a number is to be known only by its whole infinite decimal expansion, nobody does know and nobody ever will know the exact value of this number. What immediate practical consequences, if any, does this fact have? Is there any other sense in which one could be said to know this number exactly? If there are no direct consequences of being ignorant of its exact value, is there any practical value in having the concept of an exact square root of 2? Why not simply replace it by a suitable approximation such as 1.41421? Consider also other “irrational” numbers, such as π, e, and . What is the value of having the concept of such numbers as opposed to approximate rational replacements for them?

1.7. Does the development of personal knowledge of mathematics mirror the historical development of the subject? That is, do we learn mathematical concepts as individuals in the same order in which these concepts appeared historically?

1.8. Topology, which may be unfamiliar to you, studies (among other things) the mathematical properties of knots, which have been familiar to the human race at least as long as most of the subject matter of geometry. Why was such a familiar object not studied mathematically until the twentieth century?

1.9. What function does logic fulfill in mathematics? Is it needed to provide a psychological feeling of confidence in a mathematical rule or assertion? Consider, for example, any simple computer program that you may have written. What really gave you confidence that it worked? Was it your logical analysis of the operations involved, or was it empirical testing on an actual computer with a large variety of different input data?

1.10. Logic enters the mathematics curriculum in high-school geometry. The reason for introducing it at that stage is historical: Formal treatises with axioms, theorems, and proofs were a Greek innovation, and the Greeks were primarily geometers. There is no logical reason why logic is any more important in geometry than in algebra or arithmetic. Yet it seems that without the explicit statement of assumptions, the parallel postulate of Euclid would never have been questioned. Suppose things had happened that way. Does it follow that non-Euclidean geometry would never have been discovered? How important is non-Euclidean geometry, anyway? What other kinds of geometry do you know about? Is it necessary to be guided by axioms and postulates in order to discover or fully understand, say, the non-Euclidean geometry of a curved surface in Euclidean space? If it is not necessary, what is the value of an axiomatic development of such a geometry?

1.11. According to musical theory, the frequency of the major fifth in each scale should be 3/2 of the frequency of the base tone, while the frequency of the octave should be twice the base frequency. If you start at the lowest A on the piano and ascend in steps of a major fifth, twelve steps will bring you to the highest A on the piano. If all these fifths are tuned properly, that highest A should have a frequency of times the frequency of the lowest A. On the other hand, that highest A is seven octaves above the lowest, so that, if all the octaves are tuned properly, the frequency should be 2⁷ times as high. Now obviously, is not the same thing as 2⁷ = 128, since equality of these two quantities would mean 3¹² = 2¹⁹, that is, an odd number would equal an even number. The difference between these two frequency ratios is called the Pythagorean comma. (The Greek word komma means a break or cutoff.) What is the significance of this discrepancy for music? Could you hear the difference between a piano tuned so that all these fifths are exactly right and a piano tuned so that all the octaves are exactly right? In fact, because of the properties of metal strings and the peculiarities of human perception, piano tuning (like music itself) is very much an art or a skill, not reducible to formula.

Notes

1. We may or may not have in the back of our minds a picture of an infinitely precise number that represents the exact volume of water in a jar, for example, but we can meaningfully talk about only a measured volume and say with absolute assurance that the “true” volume lies between two limits. This “true” volume, given with infinite precision, is unknowable. This problem always arises in applications to the physical world. It is meaningful to ask what the 3000th decimal digit of is (it is 2); it does not make sense to ask what the 3000th decimal digit of Planck's constant in MKS units is.

2. Rectangles do not exist in non-Euclidean geometry. There is a Pythagorean theorem in both elliptic and hyperbolic geometry, but it involves trigonometric and hyperbolic functions and is more analytic than geometric in nature.

3. Natural philosophy was the name once given to what we now call the natural sciences.

4. Throughout most of history, however, arithmetic meant what we now call number theory. The modern use of this word to denote the four basic operations on numbers is largely an American innovation, and not a desirable one, in my opinion. Since the word comes from Greek and uses the root arithmos, meaning number, and the suffix -ikos, meaning skilled in, I prefer to translate it as the numerical art.