Basic Math and Pre-Algebra
PART 2. Into the Unknown
CHAPTER 11. Coordinate Graphing
When you learned to solve equations and inequalities, I mentioned that there should only be one variable involved. If there are two variables in the same equation, the solution would have to be not just a number, but a pair of numbers, one for each variable. It turns out that if you have two variables and only one equation, there are infinitely many different pairs of numbers that could be solutions. You can’t settle on just one pair. If you look at an equation like x + y = 5, you could say x is 1 and y is 4, or x is 2 and y is 3, or x is 5 and y is 0. That’s three possible solutions already, and we haven’t begun to talk about negative numbers or fractions yet. A single equation with two variables has many, many possible solutions.
When you learned to solve an inequality, you learned that a picture of the solution set on a number line was helpful in understanding what the solution really meant. In this chapter, you’ll learn a system for picturing the many, many solutions for an equation with two variables. You’ll look at the basic idea of the system and at quick ways to draw the picture for your particular equation. You’ll also see how a few key pieces of information from the picture tell you what equation it represents. Inequalities with two variables can be pictured too, and you’ll see how those pictures compare to the pictures of equations.
The Coordinate Plane
Take a sheet of paper and draw a horizontal number line across the middle of the sheet. Then draw a vertical number line, so that the two lines have their zero in the same spot. With just those two lines in place, you can direct someone to any point on the paper by giving them a number on the horizontal line and one on the vertical line. It’s as if those two numbers were the names of streets, 4thStreet and 5th Avenue, and you wanted to meet someone on the corner.
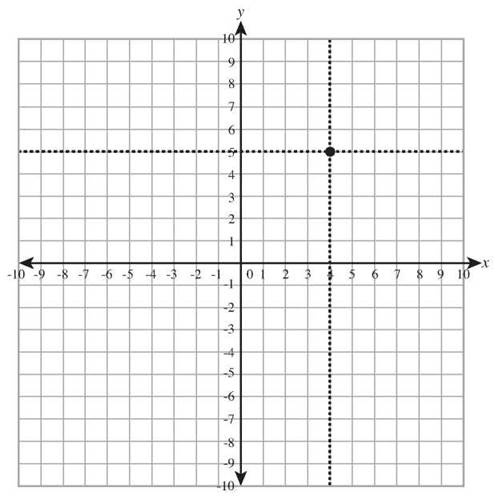
The horizontal number line is called the x-axis, and the vertical one is called the y-axis. Every point is represented by a pair of numbers, (x,y). The point in our example is the point (4,5). The two numbers are called the coordinates of the point. 4 is the x-coordinate, and 5 is the y-coordinate. To locate the point, start at the spot that is 0 on both number lines. This point (0,0) is called the origin. Count left or right according to the first coordinate. In this case, count 4 to the right. Then let the y-coordinate tell you how far up or down to go. In this case, go up 5.
DEFINITION
The Cartesian coordinate system, named for Rene Descartes, is a rectangular coordinate system that locates every point in the plane with an ordered pair of numbers, (x,y). The x-coordinate indicates horizontal movement, and the y-coordinate vertical movement.
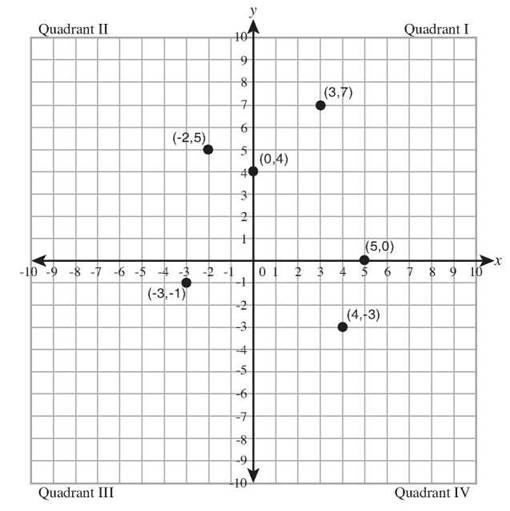
The x-axis and y-axis divide the graphing area into four sections called quadrants. The following graph shows the point (3,7) in the upper right quadrant, which is quadrant I. The point (-2,5) is in the upper left quadrant, quadrant II. In the lower left quadrant, called quadrant III, you can see the point (-3,-1), and in quadrant IV on the lower right, the point (4,-3). The point (5,0) sits on the x-axis, and (0, 4) is on the y-axis.
CHECK POINT
Plot each point in the coordinate plane.
1. (1,8)
2. (-6,2)
3. (3,-6)
4. (-4,-1)
5. (0,2)