Basic Math and Pre-Algebra
PART 3. The Shape of the World
CHAPTER 13. Triangles
Right Triangles
Right triangles are triangles that contain one right angle, and they pop up all over the place. When someone wants to build something that stands up vertically, they’ll often add a slanting support to create a right triangle. Every time you draw an altitude in any triangle, you create two right triangles. The right triangle is the one in which a side can also be an altitude.
In a right triangle, the two sides that meet to form the right angle are called the legs, and the third side, opposite the right angle, is called the hypotenuse. The hypotenuse of a right triangle is always the longest side because it’s opposite the right angle, the largest angle of the triangle.
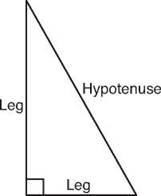
In a right triangle, the two sides that form the right angle are called legs, and the side opposite the right angle is called the hypotenuse.
The Pythagorean Theorem
One of the most famous theorems in mathematics can be applied to right triangles. It’s named for Pythagoras, a sixth century B.C.E. Greek mathematician and philosopher. What Pythagoras actually said about right triangles was probably something like “the square constructed on the hypotenuse of a right triangle contains the squares on the other two sides.” His method of investigating was actually drawing squares, but you can think about it from more of a number point of view.
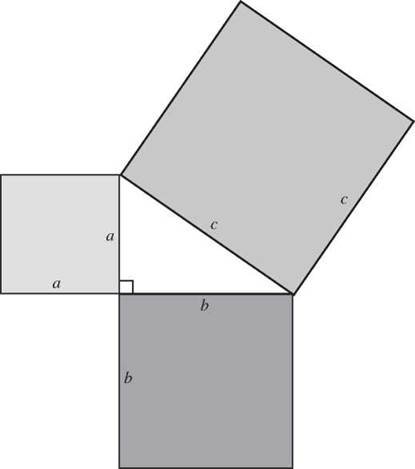
In any right triangle, if you measure all the sides and square those measurements, the square of the length of the hypotenuse will be equal to the sum of the squares of the other two sides. If the legs of a right triangle measure 5 inches and 12 inches, then the hypotenuse is 13 inches; 132 = 169 and 52 + 122 = 25 + 144 = 169.
The easiest way to remember the Pythagorean theorem, and the most common way, is in symbolic form. If the legs of the right triangle are labeled a and b and the hypotenuse is c, then a2 + b2 = c2.
DEFINITION
The Pythagorean theorem states that for any right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides, or if the length of the hypotenuse is c, and the lengths of the legs are a and b, then a2 + b2 = c2.
The importance of the Pythagorean theorem comes from the fact that you can use it to find the length of one side of the right triangle if you know the other two.
Suppose ∆RST is a right triangle with right angle at R. If RS = 7 and RT = 4, you can find the length of side ![]() with the Pythagorean theorem. The right angle is at R, so the sides you know are the legs that make the right angle, and you’re looking for the hypotenuse. Use a2 + b = c2, with a = 7 and b = 4.
with the Pythagorean theorem. The right angle is at R, so the sides you know are the legs that make the right angle, and you’re looking for the hypotenuse. Use a2 + b = c2, with a = 7 and b = 4.
c2 = 49 + 16
c2 = 65
This means TS will be √65, which is slightly more than 8.06.
You’ve probably thought about problems that could be solved with the Pythagorean theorem. Here’s one. Elise walks to school every morning, and on sunny days, she can cut across the football field from corner to corner. On snowy days, she must go around the outside edges of the field. How much shorter is Elise’s walk on sunny days?
The field has a right angle at its corner, and Elise’s path from corner to corner makes the hypotenuse of a right triangle. The field, including the end zones, is 120 yards long and 53.33 yards wide. Use a2 + b2 = c2, with a = 120 and b = 53.33 to find the length of Elise’s shortcut.
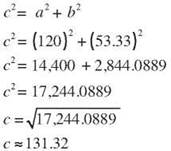
On sunny days, Elise can take the shortcut that’s about 131.32 yards, but on snowy mornings, she’ll have to go around the edges of the field, a total of 120 + 53.33 = 173.33 yards. On sunny days, she saves 173.33 - 131.32 ≈ 42.01 yards. Her sunny day route is about 42 yards shorter.
You can use the Pythagorean theorem to find a leg, too, if you know one leg and the hypotenuse. Suppose you’re shopping for a new TV, and you’re looking at one that is advertised as 55 inch. That’s the diagonal measurement, corner to corner, so it’s the hypotenuse of a right triangle. If that television is 27 inches high, how wide will it be? The height is one leg, and the width you’re looking for is the other leg, so a = 27, b is unknown, and c = 55.
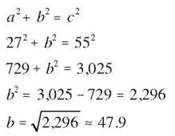
The TV will be about 48 inches, or 4 feet, wide.
You’ve probably notices a lot of “approximately equal to” answers from the Pythagorean theorem. That’s because a lot of those square roots produce irrational numbers, whose decimals go on forever and have to be rounded.
There are some problems that work out to nice whole number answers, and when you work with right triangles, you get to know them. A set of three whole numbers that fits the Pythagorean theorem is called a Pythagorean triple. The most common one is 3-4-5: 32 + 42 = 52. Multiples of Pythagorean triples are also triples, so 6-8-10 and 30-40-50 work as well. Other Pythagorean triples are 5-12-13 and 8-15-17. These sets of numbers come up a lot in right triangle problems, and recognizing the triples can save you some work.
DEFINITION
A Pythagorean triple is a set of three whole numbers a, b, and c that fit the rule a2 + b2 = c2.
CHECK POINT
Find the missing side of each right triangle.
11. In ∆XYZ, ![]() If XY = 15 cm and YZ = 20 cm, find XZ.
If XY = 15 cm and YZ = 20 cm, find XZ.
12. In ∆RST, ![]() If ST = 20 inches and RS = 52 inches, find RT.
If ST = 20 inches and RS = 52 inches, find RT.
13. In ∆PQR, ![]() If PQ = PR = 3 feet, find QR.
If PQ = PR = 3 feet, find QR.
14. In ∆CAT, ![]() If CT = 8 meters and CA = 4 meters, find AT.
If CT = 8 meters and CA = 4 meters, find AT.
15. In ∆DOG, ![]() If DO = 21 cm and DG = 35 cm, find OG.
If DO = 21 cm and DG = 35 cm, find OG.
Special Right Triangles
The Pythagorean triples show up a lot when you’re working with right triangles, and certain families of right triangles tend to show up a lot as well. When an altitude is drawn in an equilateral triangle, it divides the triangle into two right triangles. Do you remember that this altitude is one of those super segments that are altitudes, angle bisectors, and medians all in one? Each of these smaller triangles has a right angle where the altitude meets the base, an angle of 30° where the altitude bisects the vertex angle, and an angle of 60°. These right triangles are often called 30-60-90 right triangles because of their angles.
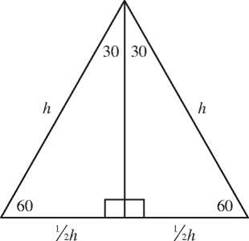
The hypotenuse of the 30°-60°-90° triangle is the side of the original equilateral triangle. The side opposite the 30° angle is half as large because that altitude was also a median, so it divided that side into two congruent segments. Using the Pythagorean theorem, you can find the length of the third side of the right triangle, the side that actually is the altitude. Suppose the hypotenuse is 1 foot long. That means the leg you know is half of that, or 1/2 foot.
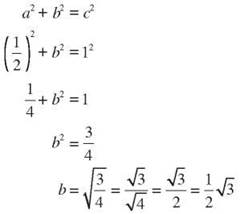
The side opposite the 60° angle must be half the hypotenuse times the square root of 3. So if you know the side of an equilateral triangle in 8 inches long, the altitude will divide the base into two segments, each 4 inches long, and the altitude itself will be 4√3 inches long, or approximately 6.9 inches.
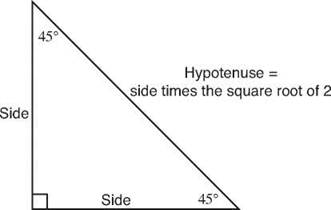
The other special right triangle, another one that shows up a lot, is the 45-45-90 triangle, or the isosceles right triangle. In an isosceles right triangle, the two legs are of equal length. You could pick your favorite number for an example, but let’s say the legs are each 6 inches long. Apply the Pythagorean theorem. The equation a2 + b2 = c2 becomes 62 + 62 = c2, or c2 = 72. Take the square root and c, the length of the hypotenuse, is c = √72 = 6√2. The hypotenuse of an isosceles right triangle is equal to the length of the leg times the square root of 2.
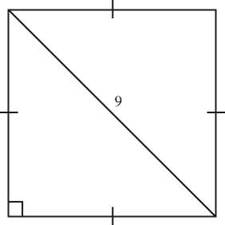
Suppose the diagonal of a square is 9 cm. The diagonal of the square is the hypotenuse of an isosceles right triangle, so if that is equal to 9, and you know it’s supposed to be equal to the side times the square root of 2, you can say s√2 = 9 and s = 9/√2 ≈ 6.4.
CHECK POINT
16. ∆ABC is a 30°-60°-90° right triangle, with hypotenuse 8 cm long. Find the length of the shorter leg.
17. ∆RST is an isosceles right triangle with legs 5 inches long. Find the length of the hypotenuse.
18. ∆ARM is a right triangle with AR = 14 meters, RM = 28 meters, and AM = 14√3 meters. Find the m∠M.
19. ∆LEG is a right triangle with LE = EG and LG = 7√2 inches. Find m∠G.
20. ∆OWL is an isosceles right triangle with OW > OL. Which angle is the right angle?