Basic Math and Pre-Algebra

PART 3. The Shape of the World

CHAPTER 13. Triangles

Area and Perimeter

The perimeter of any figure is the distance around all the edges. In a triangle, that means the sum of the lengths of the three sides. If a right triangle has legs of 3 feet and 4 feet and a hypotenuse of 5 feet, its perimeter is 3 + 4 + 5 = 12 feet.

DEFINITION

The perimeter of a triangle (or any polygon) is the total of the lengths of all its sides.

If you know the perimeter and two of the sides of a triangle, you can work backward to find the length of the other side. If a triangle has a perimeter of 42 inches, and you know that it has an 18-inch side and a 15-inch side, you can add 18 + 15 to find out that the two known sides account for 33 inches of the perimeter, so the third side must be 42 - 33 = 9 inches long.

If you think of the perimeter as the outline of a shape, you can think of the area as the space inside the perimeter. This is true for triangles and for all polygons. When you talk about the size of a lot of land or the size of a rug, you’re talking about area.

DEFINITION

The area of a polygon is the space enclosed within its sides.

To find the area of a triangle, you need to know the measure of the base and the height. Then you can plug these measurements into a simple formula, , where A = area, b = base, and h = height. You can call any one of the sides the base, as long as the height is the length of the altitude drawn from the opposite vertex, perpendicular to the base. This can sometimes cause the altitude to fall outside the triangle. If that happens, extend the side to cross the altitude. The length of the altitude is from the vertex to the point where it crosses the extension, but the length of the base is only the part in the triangle. It doesn’t include the extension.

Suppose you want to find the area of an equilateral triangle with sides 12 inches long. You need to find the length of an altitude, but if you remember the special right triangles, it’s not too bad. The altitude in an equilateral triangle is also a median and an angle bisector, so it creates two 30°-60°-90° triangles. The length of the altitude is half the length of a side times the square root of three, so 6√3 inches. Use the side of 12 inches as the base and the 6√3 inches as the height.

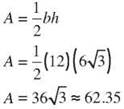
The area of the triangle is approximately 62.35 square inches.

WORLDLY WISDOM

Area is always measured in square units: square inches, square feet, square centimeters, etc. When you multiply feet times feet you get square feet. Meters times meters yields square meters.

Triangle PQR has an area of 24 square centimeters. If the lengths of its sides are 3 centimeters, 6 centimeters, and 8 centimeters, find the length of the longest altitude.

The area of the triangle will be the same no matter which side is called the base, if the altitude is drawn to that base. If we say the base is the 3 centimeter side, then  becomes  and h = 16. If we use the 6 centimeter side as the base, then  becomes  and h = 8. Declare that the base is the 8 centimeter side, then  becomes  and h = 6. The longest altitude is 16 cm.

CHECK POINT

21. Find the area of a triangle with a base 14 cm long and an altitude of 7 cm.

22. If the area of a triangle is 27 square inches, and the altitude measures 6 inches, how long is the base to which that altitude is drawn?

23. Find the perimeter of a right triangle with legs that measure 20 cm and 48 cm.

24. Find the perimeter of an equilateral triangle with an area of 9√3 square inches.

25. The area of a right triangle with legs of 3 cm and 4 cm and hypotenuse of 5 cm is _____ square centimeters, so the altitude from the right angle to the hypotenuse is _____ centimeters long.

The Least You Need to Know

• Equilateral triangles have three sides of equal length, isosceles triangles have two sides of equal length, and scalene triangles have no equal sides.

• Obtuse triangles contain one obtuse angle, right triangles contain one right angle, and acute triangles have three acute angles.

• In any right triangle, the sum of the squares of the lengths of the legs is equal to the square of the length of the hypotenuse.

• In a 30-60-90 right triangle, the shorter leg is half the length of the hypotenuse and the longer leg is half the hypotenuse times the square root of three.

• The perimeter of any polygon is the sum of the lengths of its sides.

• The area of a triangle is one-half the length of the base times the height.