Basic Math and Pre-Algebra
PART 3. The Shape of the World
CHAPTER 14. Quadrilaterals and Other Polygons
Rectangles
A rectangle is a parallelogram in which adjacent sides are perpendicular, and so it has four right angles. Because the rectangle is a parallelogram, it has all the properties of a parallelogram, but also the special property that all four of the angles are the same size. It’s an equiangular parallelogram.

A rectangle is a parallelogram with four right angles.
You’ve probably worked with rectangles before, because there are so many rectangles all around us. You’re reading this on a rectangular page, and you could be sitting in a room built from rectangles.
Every rectangle is a parallelogram, so its opposite sides are parallel and congruent, its consecutive angles are supplementary (90° + 90° = 180°), and its opposite angles are congruent. Those are properties of every parallelogram. The rectangle also has consecutive congruent angles, because all angles are congruent.
In any parallelogram, a diagonal makes two congruent triangles. That’s still true in a rectangle, but those congruent triangles will both be right triangles. In any parallelogram, the diagonals bisect each other, and because the rectangle is a parallelogram, that’s still true. But the rectangle has another special property. The diagonals of a rectangle are congruent. In most parallelograms, which sort of slant to one side, there’s a long diagonal and a short one, but in a rectangle, both diagonals are the same length.
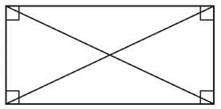
Suppose that in rectangle ABCD, the diagonals intersect at E. If BE = 8, how long is AE? Because the diagonals are congruent and bisect each other, AE = EC = BE = ED. So if BE = 8, AE = 8 as well.