Basic Math and Pre-Algebra
PART 3. The Shape of the World
CHAPTER 15. Circles
Lines and Angles
Radii, diameters, and chords are line segments. They have endpoints that are either on the circle or at the center of the circle. Lines that go on forever can also create angles that interact with the circle.
MATH TRAP
Remember that the center is not a point of the circle. The circle is points that are a set distance from the center.
A secant is a line that contains a chord. It is a line that intersects the circle in two distinct points. A tangent is a line that touches the circle in exactly one point. Just the way tangent circles only touched each other at one point, a tangent line only touches the circle at one point.
Secants and tangents also make angles on or around the circle. Two tangents, each of which touches the circle in just one point, can cross outside the circle, making an angle. It will look like an ice cream cone (the two tangents) holding a scoop of ice cream (the circle.) Two secants can cross outside the circle to form the vertex of the angle, and then each intersects the circle twice, dividing it into four arcs. Or a secant and a tangent can make an angle with its vertex outside the circle, with the secant cutting the circle twice and the tangent just touching the side.
DEFINITION
A secant is a line that intersects the circle it two different points. A tangent is a line that only touches the circle at one point.
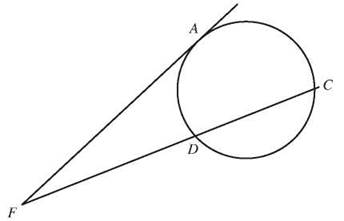
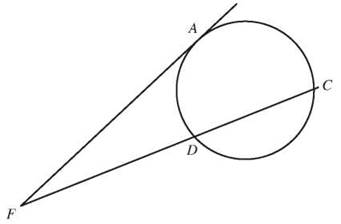
Line FC is a secant. Line FA is a tangent.
When these lines start making angles, there are a lot of angles and a lot of arcs that you can look at, but the good news is that all three kinds of angles come down to the same measuring rule. Let’s look at them one by one, starting with the angle formed by two secants.
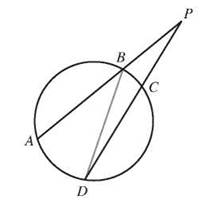
The figure shows two secants that cross at P. One cuts the circle at B and then again at A. The other crosses the circle at C and at D. You want the measurement of ∠P. To create some angles you do know how to measure, draw BD. That will make ∆BDP and exterior angle ∠ABD. m∠ABD = m∠P + m∠BDC, so m∠P = m∠ABD - m∠BDC. Both ∠ABD and ∠BDC are inscribed angles, so each is equal to half its intercepted arc. That means that the measure of ∠P is half of ![]() minus half of
minus half of ![]() , or half of the difference between
, or half of the difference between ![]() and
and ![]() . The measure of an angle formed by two secants is half the difference of the two arcs it intercepts.
. The measure of an angle formed by two secants is half the difference of the two arcs it intercepts.
Angles formed by two secants, a tangent and a secant, or two tangents will intercept two arcs. The arc nearest to the vertex of the angle is the smaller of the two. The two secants, as you can see in the figure, cut off a small arc ![]() the first time they cross the circle and a larger one
the first time they cross the circle and a larger one ![]() the second time they cross the circle. The measure of the angle is one-half the difference of the two arcs it intercepts.
the second time they cross the circle. The measure of the angle is one-half the difference of the two arcs it intercepts.
Two tangents are drawn to the circle from point P, as shown in the figure. Each tangent touches the circle at one point, A or B. The circle is broken into two arcs: the minor arc ![]() and the major arc
and the major arc ![]() The measure of LP will be half the difference between the major arc and the minor arc,
The measure of LP will be half the difference between the major arc and the minor arc, ![]()

When an angle is formed by two tangents, the circle is broken into two arcs. When two secants make the angle, there are four arcs, but only the two that fall between the secants are used in the measurement. When a secant and a tangent team up to make an angle, you get three arcs, ![]() and
and ![]() as shown below. The two you want are the two between the tangent and the secant,
as shown below. The two you want are the two between the tangent and the secant, ![]() and
and![]() The measure of
The measure of ![]()
WORLDLY WISDOM
All the rules about the measurement of angles in and around circles can be boiled down to four rules, according to where the vertex of the angle is. If the vertex is at the center, the angle equals its intercepted arc. If the vertex is on the circle, the angle is half the intercepted arc. If the vertex is inside the circle, the angle is half the sum of the arcs, and if the vertex is outside the circle, the angle is half the difference of the arcs.
One last type of angle that you should know about is the angle that’s formed by a line and a segment, or specifically a tangent and a radius or diameter, or a tangent and a chord. A tangent just touches the circle at one point. When you draw a chord from that point of tangency T to some other point on the circle, like C, you divide the circle into two arcs, minor arc ![]() and major arc
and major arc ![]() and you create two angles, ∠ATC and ∠BTC. The measure of each angle is half its intercepted arc.
and you create two angles, ∠ATC and ∠BTC. The measure of each angle is half its intercepted arc. ![]() and
and ![]() The two arcs add up to the whole circle, or 360°, so half of each one will add to 180°, which sounds just right because the angles are a linear pair and linear pairs are supplementary.
The two arcs add up to the whole circle, or 360°, so half of each one will add to 180°, which sounds just right because the angles are a linear pair and linear pairs are supplementary.

If the chord you drew happened to be a diameter, both intercepted arcs would be 180°, so both angles would be 90°. That means that a diameter drawn to the point of tangency is perpendicular to the tangent line, and because a radius is half the diameter, a radius drawn to the point of tangency is perpendicular to the tangent.
CHECK POINT
11. Two tangents ![]() and
and ![]() meet at point P outside circle O and touch the circle at A and E. Minor arc
meet at point P outside circle O and touch the circle at A and E. Minor arc ![]() measures 140° and major arc
measures 140° and major arc ![]() measures 220°. Find the measure of ∠APE.
measures 220°. Find the measure of ∠APE.
12. Two lines pass through point A, outside circle O. One intersects circle O at points E and F. The other line intersects the circle at 5 and then T. The measure of ![]() is 12°, and the measure of
is 12°, and the measure of ![]() is 52°. Find the measure of ∠FAT.
is 52°. Find the measure of ∠FAT.
13. Tangent ![]() touches circle O at point A and meets chord
touches circle O at point A and meets chord ![]() Minor arc
Minor arc ![]() measures 72°. If m∠RAC > m∠TAC, find the measure of ∠RAC.
measures 72°. If m∠RAC > m∠TAC, find the measure of ∠RAC.
14. Tangent ![]() and secant
and secant ![]() form an angle of 15°. If arc
form an angle of 15°. If arc ![]() measures 160°, find the measure of arc
measures 160°, find the measure of arc ![]()
15. Tangents ![]() and
and ![]() touch circle O at points A and B, dividing the circle into two arcs. The measure of the larger arc is twice that of the smaller arc. Find the measure of ∠P.
touch circle O at points A and B, dividing the circle into two arcs. The measure of the larger arc is twice that of the smaller arc. Find the measure of ∠P.