Basic Math and Pre-Algebra
PART 3. The Shape of the World
CHAPTER 16. Surface Area and Volume
Pyramids
A pyramid is a polyhedron formed by one polygon, called the base, surrounded by triangles that meet at a point. Like prisms, pyramids take their names from the polygon that forms the base. If the base is a pentagon, it’s a pentagonal pyramid. If the base is a square, it’s a square pyramid, and if the base is also a triangle, it’s a triangular pyramid.
DEFINITION
A pyramid is a polyhedron composed of a polygon for a base surrounded by triangles that meet at a point.
Surface Area
Like the prism, the pyramid can be broken down into its parts to find the surface area. If the base has n sides, the surface area is the area of the base plus the area of the n triangles that surround it. You can calculate each of the pieces separately and add them up.
MATH TRAP
You need to have the right slant on measurements in a pyramid. The word “height” gets used in a lot of different ways and doesn't always mean the same thing. In a prism, the height is one of the dimensions of the surrounding rectangles, because those rectangles stand straight up at right angles to the base. In a prism, the surrounding triangles are tilted. Their height, called the slant height, is longer than the height of the pyramid.
Usually when you’re asked to find the surface area of a pyramid with a polygon as its base, that polygon is regular. All its sides are the same length and all its angles are equal. That makes finding its area a little easier. The area of a regular polygon is 1/2 times the apothem times the perimeter.
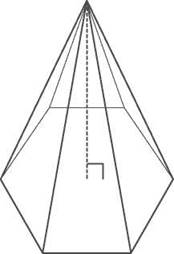
To find the surface area of a hexagonal pyramid, you have to know the area of the hexagon at the base and the area of the six surrounding triangles. If the hexagon is a regular hexagon, you can find the area if you know a side (or the perimeter) and the apothem. To find the area of the triangles, you need to know the side of the hexagon and the height of the triangle, which is the slant height of the pyramid. The lateral area is six times the area of one triangle, or 1/2 times the perimeter times the slant height.
WORLDLY WISDOM
The area of a regular polygon with n sides is the total of the n triangles into which it is broken when you draw the radii from the center to each vertex. That translates to n x 1/2 x apothem x a side. Because n times a side is also the perimeter, the area of a regular polygon is 1/2 x apothem x perimeter.
It sounds like a lot of work, but here’s how it breaks down. You need to know the perimeter (p) of the regular polygon at the base, the apothem (a) of that polygon, and the slant height (l) of the pyramid. Then you can find the surface area.
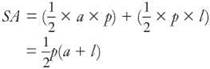
To find the surface area of a hexagonal pyramid with a perimeter of 18 inches, an apothem of 2 inches, and a slant height of 8 inches, plug those numbers into the formula.

The hexagonal pyramid has a surface area of 90 square inches.
CHECK POINT
Find the surface area of each pyramid. ![]()
11. A square pyramid 4 inches on each side with a slant height of 5 inches.
12. A triangular pyramid with a slant of 10 cm, whose base is an equilateral triangle 12 cm on a side, with an area of 62.4 square centimeters.
13. A pyramid with a slant height of 18 cm and regular pentagon as a base. The regular pentagon has a perimeter of 50 cm and an area of 172 square centimeters.
14. A hexagonal pyramid with a slant height of 10 inches. The regular hexagon that forms the base has a perimeter of 60 inches and an area of 260 square inches.
15. A square pyramid with a slant height of 13 inches and a side of 10 inches.
Volume
The volume of a prism is V = Bh. The volume of a pyramid with the same base and the same height must be smaller, because those lateral faces went from rectangles to triangles and tipped inward. The volume of a pyramid is one-third of the area of the base times the height. If B is the area of the base and h is the height, the volume is ![]()
Let’s return to the hexagonal pyramid you looked at earlier, with p = 18 inches, a = 2 inches, and l = 8 inches. To find its volume, we need to know the area of the base and its height. (Remember, the height is different from the slant height.)
The area of its base is ![]() square inches. For this pyramid, the height is about 7.75 inches. Plug those numbers into the formula.
square inches. For this pyramid, the height is about 7.75 inches. Plug those numbers into the formula.
![]()
The volume is about 46.5 cubic inches. Now consider a square pyramid with an edge 12 feet long and a height of 18 feet. If you plug those numbers into the formula, you get ![]()
![]() The pyramid will have a volume of 864 cubic feet.
The pyramid will have a volume of 864 cubic feet.
MATH IN THE PAST
The Great Pyramid of Giza, the largest of the three famous structures, is a square pyramid with a base 756 feet on each side and a height of 455 feet. That gives it a volume of over 86 million cubic feet. However, there isn't actually that much room inside it, because much of the space is occupied by the stones from which it was built.
CHECK POINT
Find the volume of each pyramid. ![]()
16. A square pyramid with a slant height of 13 inches and a side of 10 inches.
17. A square pyramid 4 inches on each side with a slant height of 5 inches.
18. A triangular pyramid with a slant of 10 cm, whose base is an equilateral triangle 12 cm on a side, with an area of 62.4 square centimeters.
19. A pyramid with a slant height of 18 cm and regular pentagon as a base. The regular pentagon has a perimeter of 50 cm and an area of 172 square centimeters.
20. A hexagonal pyramid with a slant height of 10 inches. The regular hexagon that forms the base has a perimeter of 60 inches and an area of 260 square inches.