Numbers: Their Tales, Types, and Treasures.

Chapter 2: Numbers and Psychology

2.7.THE NUMBER LINE

Experiments carried out by Stanislas Dehaene and his coworkers have revealed some interesting effects concerning the processing of numbers in the human brain.

When comparing two numbers according to size, the time it takes to decide which of two numbers is larger depends on their difference. For example, in a reaction test we all need longer to decide whether 6 is larger than 5 than it takes us to decide whether 9 is larger than 4. In the case of two-digit numbers, it takes longer to decide if 81 is larger than 79 than it takes to decide whether 85 is larger than 76 (even if in both cases one could make the decision by only looking at the tens digit). Dehaene calls this the “distance effect.” It is more difficult to sort numbers according to size when they are closer together, and this fact cannot be changed even by extensive training. Another aspect of the distance effect is that 85 and 76 appear to be closer together than 15 and 6, although their difference is the same. This is an effect of the Weber-Fechner Law discussed earlier. When sorting two numbers, one has roughly the same reaction time and error rate when the two numbers differ by the same percentage (such as 9 and 12, versus 60 and 80).

An explanation seems to be that during learning numbers, the brain creates an analog representation of quantity in its neuronal network. This representation is a vague association of numbers with a spatial arrangement. Numbers appear to be arranged in space according to their size, so that 2 is “in between” 1 and 3, and likewise 12 is between 11 and 13. The idea of a spatial arrangement of number is a result of cultural learning. In Western culture, people usually arrange numbers increasing from left to right, the usual direction of Western writing, while people in cultures with another direction of writing also tend to arrange numbers in that direction. It is commonly believed that the activation of that mental number line is automatic and unconscious yet influences our perception of numbers.

The SNARC effect (“spatial numerical association of response codes”), discovered by Dehaene in 1993, provides another indication of the role of the number line in the mental representation of numbers. A testing subject has to answer by pressing a button either on his right side or on his left side, to decide as fast as possible whether a number that appears on a computer screen is even or odd. The response time shows a difference between answers given with the buttons on the right side and answers given on the left side. For small numbers, people are able to give the answer faster on the left side, and for large numbers they are faster on the right side. And this effect does not depend on whether the test takers are right- or left-handed or whether they provide the answer with their arms crossed. It is not the hand with which we give the answer, it is the side of the body where the answer buttons are located that determines the reaction time. On the left side, the side that is associated with small numbers, we are faster to discover properties of small numbers. With larger numbers, we have a shorter reaction time on the right side than on the left side because the right side corresponds better to our inner representation of numbers as being arranged on a mental number line. Dehaene believes that the mental number line is invoked unconsciously during the perception of any number, even when the number line is irrelevant for the task at hand, such as to determine whether a number is even or odd.

The effects of the internal spatial organization of numbers have often been considered. This internal spatial organization cannot be changed by training, and it is independent of the mathematical preparation of the test person. The mental number line is closely related to the ordering of numbers according to size. The placement of cardinal numbers in a spatial arrangement certainly makes it easier for an individual to harmonize the cardinal and ordinal aspects of number. Although the mental number line is deeply ingrained in our neuronal structure, and is often activated without conscious control, it is not innate but a result of cultural learning.

The mental number line as a neuropsychological effect has to be distinguished from the mathematical number line that we learn in school. Young children, if asked to draw the numbers on a line, where the end points are marked as 1 and 10, would produce something like figure 2.4, with 3 in the middle and the higher numbers placed closer together, with overlapping uncertainties.

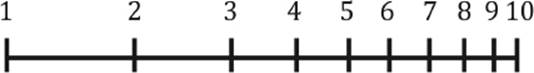
Figure 2.4: Mental number line.

Early in a child's development, the number line contains only vague information about the direction of getting larger. It will perhaps contain discrete points for one, two, and three, corresponding to the exact subitizing range of the innate number sense. For larger numbers, the number line becomes blurred, and the individual numbers appear to be the closer together as they get progressively larger, representing approximate quantities. Later, when the child learns to count, and an understanding of differences in number is developed, a conscious image of the number line is formed and gradually adjusted. When the child can count forward or backward starting from any number, the child begins to develop an understanding for the “same distance” between subsequent numbers. Older children would, therefore, tend to draw the numbers on a line with equal distances between numbers. This, of course, corresponds to the mathematical number line, for which a measuring tape would be a good model (figure 2.5).

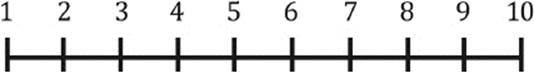
Figure 2.5: Mathematical number line.

The conscious perception of the number line is flexible and adaptive, depends on the cultural situation and on individual preferences, and changes through learning and experience, when the number concept is further developed. Not everybody prefers a linear arrangement. For some purposes, or in some intervals, numbers can be mentally arranged in a nonlinear way—for example, as on the face of a clock or sometimes even with colors as additional attributes.