Numbers: Their Tales, Types, and Treasures.

Chapter 4: Discovering Properties of Numbers

4.4.RECTANGULAR AND SQUARE NUMBERS

Experimenting a little, one sees that many numbers can be arranged to form a rectangle. For example, 15 dots can be arranged as 3 × 5, that is, as a rectangular array with 3 rows and 5 columns of points (see figure 4.3).

Figure 4.3: The rectangular number 15.

We do not regard a 5 × 3 rectangle as being different from a 3 × 5 rectangle, as one can be obtained from the other just by rotating the paper 90°. Sometimes there are several different ways to arrange rectangles. For example, 12 can be arranged as 2 × 6 or as 3 × 4 (see figure 4.4).

Figure 4.4: The rectangular number 12.

A number that can be represented geometrically by points arranged in the form of a rectangle is called a rectangular number. Of course, it is understood that a rectangle has more than one row and more than one column. Usually, 1 was not considered a rectangular number, because it is just a dot. Likewise, 2 is not rectangular, because two points just make a single line (row or column), which is not sufficient for a rectangle. Numbers like 3, 5, 7, and 11 are not rectangular numbers, because they cannot be arranged in rectangular form. A number is rectangular whenever it can be written as a product of two natural numbers other than itself and 1. Rectangular numbers are also often called composite numbers. The numbers greater than 1 that are not rectangular are called prime numbers. We will delve into these fascinating prime numbers later in the book.

A special case among the rectangular numbers is the square numbers. Square numbers can be represented by a rectangle, where the number of rows equals the number of columns. For example, 16 = 4 × 4 (see figure 4.5).

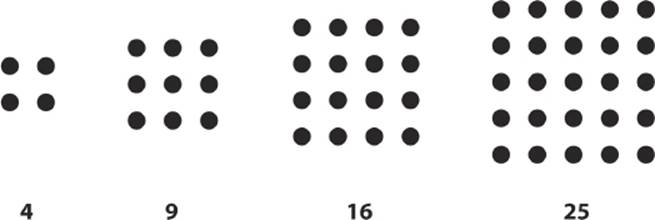
Figure 4.5: The first square numbers.

According to the definition, 1 is not a square number in the geometric sense, because 1 is not a rectangular number. And indeed, in Greek mathematics, 1 was not a number at all, as was explained earlier. Arithmetically, however, 1 is the square of 1, since 1 = 1 × 1, and today the sequence of square numbers usually includes the number 1. Sometimes it even contains 0, because zero is the square of zero: 0 = 0 × 0. The following is the sequence of nonnegative square numbers:

0, 1, 4, 9, 16, 25, 36, 49, 64, 81, 100, 121, 144, 169, 196, 225, 256, 289,…

According to the online encyclopedia of integer sequences, this was the first sequence ever computed by an electronic computer, in the year 1949.

When playing with square numbers, people noticed that in order to produce the next larger square number, one always has to add an odd number of points. So in order to go from a 2 × 2 square to a 3 × 3 square, one has to add five points; and in order to produce the next larger square number, 4 × 4, one has to add another seven points, and so on, as shown in figure 4.6.

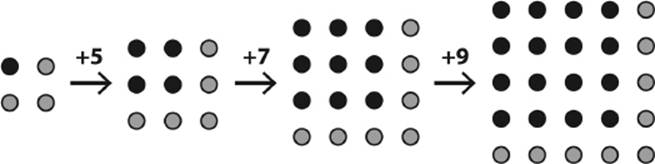
Figure 4.6: Adding odd numbers.

Even our first square number, 4, can be obtained as 1 plus 3. Thus we obtain the following beautiful pattern,

and so on.

Of course, the pattern can be extended indefinitely. The next line would be

1 + 3 + 5 + 7 + 9 + 11 = 6 × 6 = 6²,

which we can further appreciate by visualizing the geometric interpretation in figure 4.7.

Figure 4.7: The sum of the first odd numbers gives a square number.

We see that in order to compute 5 × 5 we have to sum up the first five odd numbers. And 6 × 6 is the sum of the first six odd numbers. This leads to the amazing conjecture that

· the sum of the first n odd numbers equals n × n, where n is any natural number.

When formulating a law that holds for all natural numbers, we have reached a new level of abstraction. The letter n in that statement does not refer to any particular number; rather it represents any number one wishes to substitute for n. It is a “placeholder” that can be replaced by any particular number (like 5, 6, or 273), which would turn the general statement into a statement about this particular number. For example,

· the sum of the first 273 odd numbers equals 273 × 273.

In mathematics, we call n a variable.

The sum of the first n even numbers can be constructed in a quite similar way. We do this in complete analogy to figure 4.7, with the only difference here being that we start with two dots at the beginning. Adding borders, we obtain a sequence of rectangular numbers, where the number of columns exceeds the number of rows by 1 (see figure 4.8). Notice that each of the borders contains an even number of dots.

Figure 4.8: The sum of the first even numbers gives a number of the form n × (n + 1).

From this figure, we find that,

and so on.

We can express this as a general rule:

· the sum of the first n even numbers equals n × (n + 1), where n is any natural number.

The British historian of Greek mathematics T. L. Heath (1861–1940) noted that the rectangles obtained as sums of even numbers in figure 4.8 on the basis of the even number 2 all have different proportions (3:2 is different from 4:3, which is different from 5:4, and so on), while the odd numbers all preserve the same quadratic form as shown in figure 4.7. This gives another interpretation as to why the even numbers were regarded by the Pythagoreans as unlimited and odd numbers as limited.

Obviously, the world of numbers is full of amazing regularities. To the Pythagoreans, the laws of numbers were the origin of the order of the cosmos.