Numbers: Their Tales, Types, and Treasures.

Chapter 5: Counting for Poets

5.6.DISCOVERING COMMON TRAITS OF COUNTING PROBLEMS

One of the fascinating aspects of mathematics is that insight gained for one situation can be reapplied in quite different situations. The counting problem solved in the last section indeed occurs in many different contexts. It can be seen easily that the succession of long and short syllables in speech has much in common with the succession of long and short notes of a piece of music. Figure 5.1 shows all possible bars in six-eight time that consist only of quarter notes and eighth notes.

Figure 5.1: A list of all bars in 6/8 time containing only quarter notes and eighth notes.

We can see that the list of measures in figure 5.1 corresponds exactly to the list of all possible meters with a length of six moras, as shown earlier. And figure 5.2 shows one of the A(16) = 1,597 possible rhythms with a total length of 16 eighth notes, and consisting only of quarter notes and eighth notes:

Figure 5.2: Rhythm with a total length of 16 eighth notes.

The rhythm depicted in figure 5.2 occurs, for example, in Ludwig van Beethoven's Symphony no. 7, second movement. In the literature of India, this corresponds to the meter rukmavati, which has the following sequence of long and short syllables: ¯ ˘ ˘ ¯ ¯ ¯ ˘ ˘ ¯ ¯.

Here are two other examples:

The garden-path problem: You want to lay out a garden path with rectangular slabs. You can either place the slabs perpendicular or parallel to the direction of walking, as shown in figure 5.3. How many patterns are there to lay out sixteen slabs?

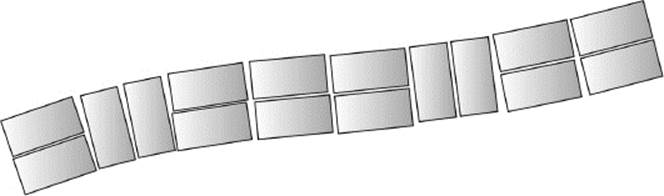
Figure 5.3: A garden path paved with sixteen slabs.

Do you recognize the similarity of this problem with Pingala's problem of arranging long and short syllables? A perpendicular slab would correspond to a single short syllable, and an element of two parallel slabs would correspond to a single long syllable. A long syllable takes the time of two short syllables and a parallel element consists of two slabs. The footpath is an arrangement of perpendicular (one slab) and parallel elements (two slabs), in very much the same way as a verse meter is an arrangement of short (one mora) and long (two moras) syllables. Thus, we conclude by analogy that the number of possible ways to lay out sixteen slabs in an arrangement of perpendicular and parallel elements is again A(16) = 1,597.

The problem of the postman: Every day, the driver of a parcel service has to use the same staircase with sixteen steps to deliver a parcel. Sometimes he climbs the stairs two at a time, sometimes he takes single steps. In order to add variety to his life, he decides to climb the stairs every day with another succession of single and double steps. How many ways of climbing the staircase are there?

A moment of thinking should reveal the similarity of this question with Pingala's first problem of counting rhythmic patterns of short and long syllables, or with the garden-path problem described previously. In all cases, the answer is A(16) = 1,597.