AP Physics B Exam

18 Solutions to the Chapter Review Questions

CHAPTER 2 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. A Traveling once around a circular path means that the final position coincides with the initial position. Therefore, the displacement is zero. The average speed, which is total distance traveled divided by elapsed time, cannot be zero. Since the velocity changed (because its direction changed), there was a nonzero acceleration. Therefore, only Statement I is true.

2. D Section 1 represents a constant positive speed. Section 2 shows an object slowing down, moving in the positive direction. Section 3 represents an object speeding up in the negative direction. Section 4 demonstrates a constant positive speed, and section 5 represents the correct answer: slowing down moving in the negative direction.

3. C Statement I is false since a projectile experiencing only the constant acceleration due to gravity can travel in a parabolic trajectory. Statement II is true: Zero acceleration means no change in speed (or direction). Statement III is false: An object whose speed remains constant but whose velocity vector is changing direction is accelerating.

4. C The baseball is still under the influence of Earth”s gravity. Its acceleration throughout the entire flight is constant, equal to g downward.

5. A Use Big Five #3 with v₀ = 0:

6. D Use Big Five #5 with v₀ = 0 (calling down the positive direction):

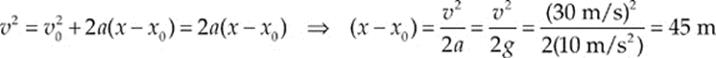
7. C Apply Big Five #3 to the vertical motion, calling down the positive direction:

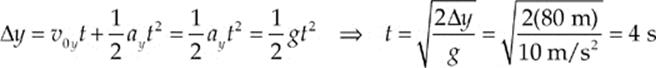
Note that the stone”s initial horizontal speed (v_0x = 10 m/s) is irrelevant.

8. B First we determine the time required for the ball to reach the top of its parabolic trajectory (which is the time required for the vertical velocity to drop to zero).

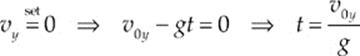
The total flight time is equal to twice this value:

9. C After 4 seconds, the stone”s vertical speed has changed by ∆v_y = a_yt = (10 m/s²)(4 s) = 40 m/s. Since v_0y = 0, the value of v_y at t = 4 is 40 m/s. The horizontal speed does not change. Therefore, when the rock hits the water, its velocity has a horizontal component of 30 m/s and a vertical component of 40 m/s.

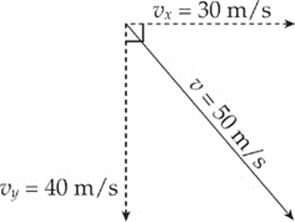
By the Pythagorean theorem, the magnitude of the total velocity, v, is 50 m/s.

10. E Since the acceleration of the projectile is always downward (because it”s gravitational acceleration), the vertical speed decreases as the projectile rises and increases as the projectile falls. Statements (A), (B), (C), and (D) are all false.

SECTION II: FREE RESPONSE

1. (a) At time t = 1 s, the car”s velocity starts to decrease as the acceleration (which is the slope of the given v vs. t graph) changes from positive to negative.

(b) The average velocity between t = 0 and t = 1 s is (v_t=0 + v_t=1) = (0 + 20 m/s) = 10 m/s, and the average velocity between t = 1 and t = 5 is (v_t=1 + v_t=5) = (20 m/s + 0) = 10 m/s. The two average velocities are the same.

(c) The displacement is equal to the area bounded by the graph and the t axis, taking areas above the t axis as positive and those below as negative. In this case, the displacement from t = 0 to t = 5 s is equal to the area of the triangular region whose base is the segment along the t axis from t = 0 to t = 5 s:

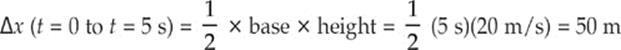
The displacement from t = 5 s to t = 7 s is equal to the negative of the area of the triangular region whose base is the segment along the t axis from t = 5 s to t = 7 s:

Therefore, the displacement from t = 0 to t = 7 s is

(d) The acceleration is the slope of the v vs. t graph. The segment of the graph from t = 0 to t = 1 s has a slope of a = ∆v/∆t = (20 m/s – 0)/(1 s – 0) = 20 m/s², and the segment of the graph from t = 1 s to t = 7 s has a slope of a = ∆v/∆t = (–10 m/s – 20 s)/(7 s – 1 s) = –5 m/s². Therefore, the graph of a vs. t is

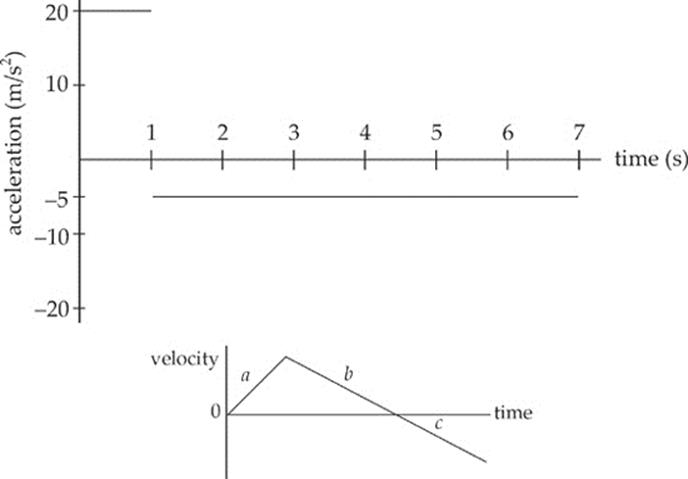
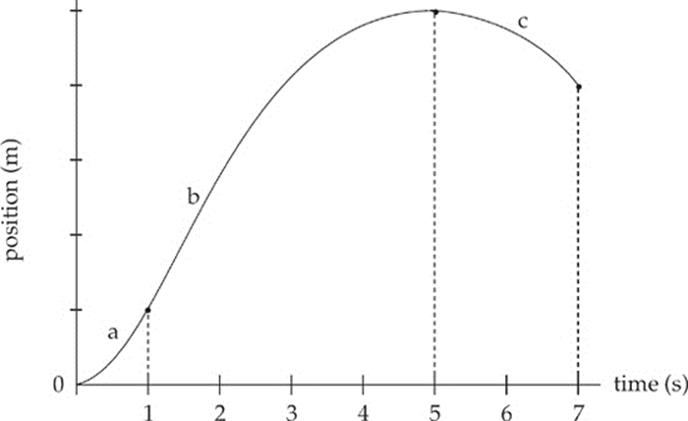
Section a tells us the object is speeding up in the positive direction. Section b tells us the object is slowing down, yet still moving in the positive direction. At five seconds, the object has stopped for an instant. Section c tells us the object is moving in the negative direction and speeding up. The corresponding position-vs.-time graph for each section would look like this:

2. (a) The maximum height of the projectile occurs at the time at which its vertical velocity drops to zero:

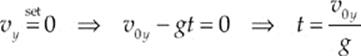
The vertical displacement of the projectile at this time is computed as follows:

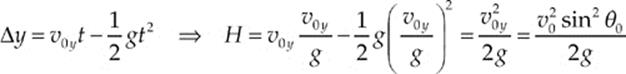
(b) The total flight time is equal to twice the time computed in part (a):

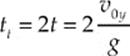
The horizontal displacement at this time gives the projectile”s range:

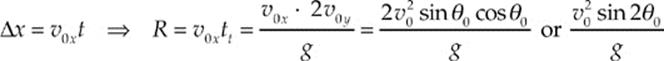
(c) For any given value of v₀, the range,

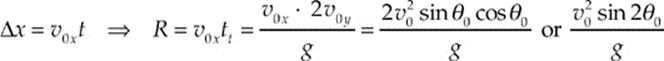
will be maximized when sin 2θ₀ is maximized. This occurs when 2θ₀ = 90°, that is, when θ₀ = 45°.

(d) Set the general expression for the projectile”s vertical displacement equal to h and solve for the two values of t (assuming that g = +10 m/s²):

Applying the quadratic formula, we find that

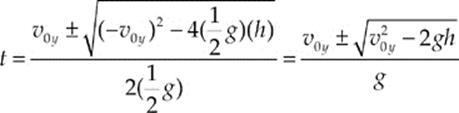
Therefore, the two times at which the projectile crosses the horizontal line at height h are

so the amount of time that elapses between these events is

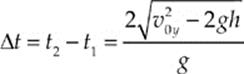
3. (a) The cannonball will certainly reach the wall (which is only 220 m away) since the ball”s range is

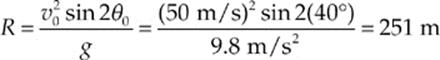
We simply need to make sure that the cannonball”s height is less than 30 m at the point where its horizontal displacement is 220 m (so that the ball actually hits the wall rather than flying over it). To do this, we find the time at which x = 220 m by first writing

Thus, the cannonball”s vertical position can be written in terms of its horizontal position as follows:

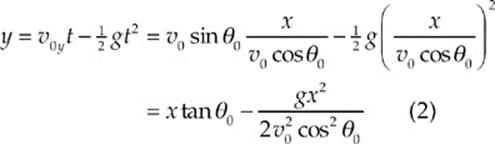
Substituting the known values for x, θ₀, g, and v₀, we get

This is indeed less than 30 m, as desired.

(b) From Equation (1) derived in part (a),

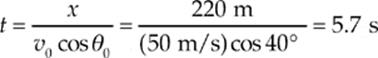
(c) The height at which the cannonball strikes the wall was determined in part (a) to be 23 m.

CHAPTER 3 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. B Because the person is not accelerating, the net force he feels must be zero. Therefore, the magnitude of the upward normal force from the floor must balance that of the downward gravitational force. Although these two forces have equal magnitudes, they do not form an action/reaction pair because they both act on the same object (namely, the person). The forces in an action/reaction pair always act on different objects.

2. D First draw a free-body diagram:

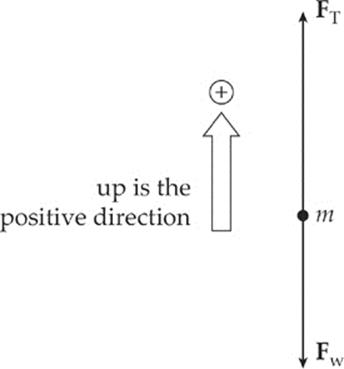
The person exerts a downward force on the scale, and the scale pushes up on the person with an equal (but opposite) force, F_N. Thus, the scale reading is F_N, the magnitude of the normal force. Since F_N – F_w = ma, we have F_N = F_w + ma = (800 N) + [800 N/(10 m/s²)](5 m/s²) = 1200 N.

3. A The net force that the object feels on the inclined plane is mg sin θ, the component of the gravitational force that is parallel to the ramp. Since sin θ = (5 m)/(20 m) = 1/4, we have F_net = (2 kg)(10 N/kg)(1/4) = 5 N.

4. C The net force on the block is F – F_f = F – µ_kF_N = F – µ_kF_w = (18 N) – (0.4)(20 N) = 10 N. Since F_net = ma = (F_w/g)a, we find that 10 N = [(20 N)/(10 m/s²)]a, which gives a = 5 m/s².

5. A The force pulling the block down the ramp is mg sin θ, and the maximum force of static friction is µ_sF_N = µ_smg cos θ. If mg sin θ is greater than µ_smg cos θ, then there is a net force down the ramp, and the block will accelerate down. So, the question becomes, “Is sin θ greater than µ_s cos θ?” Since θ = 30° and µ_s = 0.5, the answer is “yes.”

6. E One way to attack this question is to notice that if the two masses happen to be equal, that is, if M = m, then the blocks won”t accelerate (because their weights balance). The only expression given that becomes zero when M = m is the one given in choice (E). If we draw a free-body diagram,

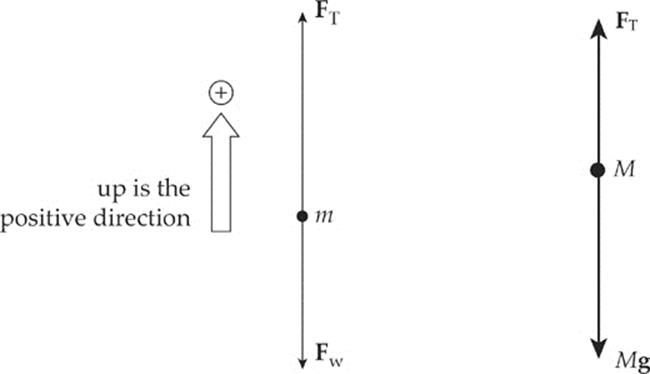
Newton”s Second Law gives us the following two equations:

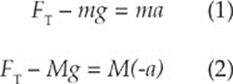
Subtracting these equations yields Mg – mg = ma + Ma = (M + m)a, so

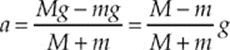
7. E If F_net = 0, then a = 0. No acceleration means constant speed (possibly, but not necessarily, zero) with no change in direction. Therefore, statements (B), (C), and (D) are false, and statement (A) is not necessarily true.

8. D The horizontal motion across the frictionless tables is unaffected by (vertical) gravitational acceleration. It would take as much force to accelerate the block across the table on Earth as it would on the Moon. (If friction were taken into account, then the smaller weight of the block on the Moon would imply a smaller normal force by the table and hence a smaller frictional force. Less force would be needed on the Moon in this case.)

9. D The maximum force which static friction can exert on the crate is µ_sF_N = µ_sF_w = µ_smg = (0.4)(100 kg)(10 N/kg) = 392 N. Since the force applied to the crate is only 344 N, static friction is able to apply that same magnitude of force on the crate, keeping it stationary. [Choice (B) is incorrect because the static friction force is not the reaction force to F; both F and F_{f (static)} act on the same object (the crate) and therefore cannot form an action/reaction pair.]

10. A With Crate #2 on top of Crate #1, the force pushing downward on the floor is greater, so the normal force exerted by the floor on Crate #1 is greater, which increases the friction force. Statements (B), (C), (D), and (E) are all false.

SECTION II: FREE RESPONSE

1. (a) The forces acting on the crate are F_T (the tension in the rope), F_w (the weight of the block), F_N (the normal force exerted by the floor), and F_f (the force of kinetic friction):

(b) First break F_T into its horizontal and vertical components:

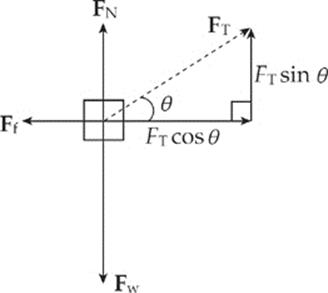
Since the net vertical force on the crate is zero, we have F_N + F_T sin θ = F_w, so F_N = F_w – F_T sin θ = mg – F_T sin θ.

(c) From part (b), we see that the net horizontal force acting on the crate is

so the crate”s horizontal acceleration across the floor is

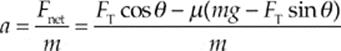
2. (a) The forces acting on Block #1 are F_T (the tension in the string connecting it to Block #2), F_w1 (the weight of the block), and F_N1 (the normal force exerted by the tabletop):

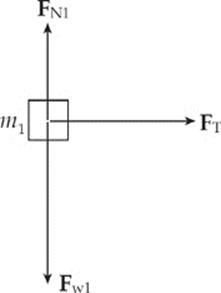
(b) The forces acting on Block #2 are F (the pulling force), F_T (the tension in the string connecting it to Block #1), F_w2 (the weight of the block), and F_N2 (the normal force exerted by the tabletop):

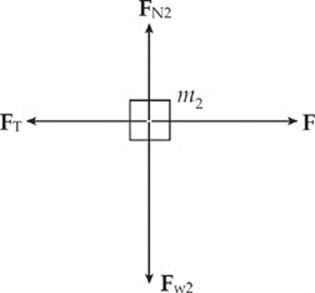
(c) Newton”s Second Law applied to Block #2 yields F – F_T = m₂a, and applied to Block #1 yields F_T = m₁a. Adding these equations, we find that F = (m₁ + m₂)a, so

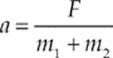
(d) Substituting the result of part (c) into the equation F_T = m₁a, we get

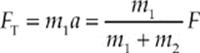
(e) (i) Since the force F must accelerate all three masses—m₁, m, and m₂—the common acceleration of all parts of the system is

(e) (ii) Let F_T1 denote the tension force in the connecting string acting on Block #1, and let F_T2 denote the tension force in the connecting string acting on Block #2. Then, Newton”s Second Law applied to Block #1 yields F_T1 = m₁a and applied to Block #2 yields F – F_T2 = m₂a. Therefore, using the value for a computed above, we get

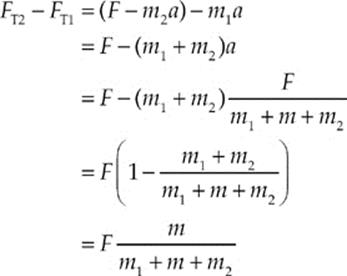
3. (a) First draw free-body diagrams for the two boxes:

Applying Newton”s Second Law to the boxes yields the following two equations:

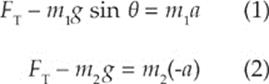
Subtracting the equations allows us to solve for a:

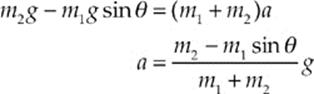
(i) For a to be positive, we must have m₂ – m₁ sin θ > 0, which implies that sin θ < m₂/m₁, or, equivalently, θ < sin^–1(m₂/m₁).

(ii) For a to be zero, we must have m₂ – m₁ sin θ = 0, which implies that sin θ = m₂/m₁, or, equivalently, θ = sin^–1(m₂/m₁).

(b) Including the force of kinetic friction, the force diagram for m₁ is

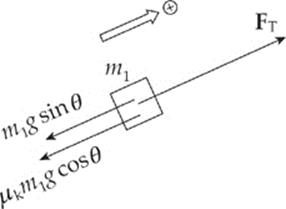
Since F_f = µ_kF_N = µ_km₁g cos θ, applying Newton”s Second Law to the boxes yields these two equations:

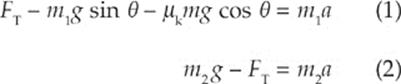
Adding the equations allows us to solve for a:

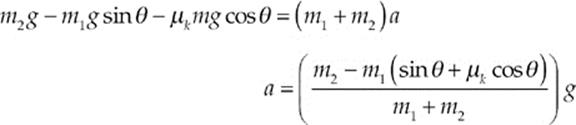
If we want a to be equal to zero (so that the box of mass m₁ slides up the ramp with constant velocity), then

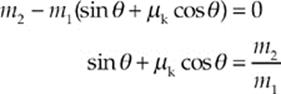
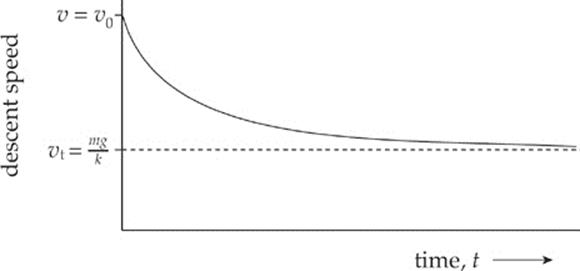
4. (a) The forces acting on the sky diver are F_r, the force of air resistance (upward), and F_w, the weight of the sky diver (downward):

(b) Since F_net = F_w – F_r = mg – kv, the sky diver”s acceleration is

(c) Terminal speed occurs when the sky diver”s acceleration becomes zero, since then the descent velocity becomes constant. Setting the expression derived in part (b) equal to 0, we find the speed v = v_t at which this occurs:

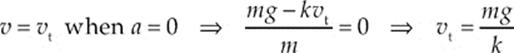
(d) The sky diver”s descent speed is initially v₀ and the acceleration is (close to) g. However, once the parachute opens, the force of air resistance provides a large (speed-dependent) upward acceleration, causing her descent velocity to decrease. The slope of the v vs. t graph (the acceleration) is not constant but instead decreases to zero as her descent speed decreases from v₀ to v_t. Therefore, the graph is not linear.

CHAPTER 4 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. A Since the force F is perpendicular to the displacement, the work it does is zero.

2. B By the work–energy theorem,

3. B Since the box (mass m) falls through a vertical distance of h, its gravitational potential energy decreases by mgh. The length of the ramp is irrelevant here.

4. C Since the centripetal force always points along a radius toward the center of the circle, and the velocity of the object is always tangent to the circle (and thus perpendicular to the radius), the work done by the centripetal force is zero. Alternatively, since the object”s speed remains constant, the work–energy theorem tells us that no work is being performed.

5. A The gravitational force points downward while the book”s displacement is upward. Therefore, the work done by gravity is –mgh = –(2 kg)(10 N/kg)(1.5 m) = –30 J.

6. D The work done by gravity as the block slides down the inclined plane is equal to the potential energy at the top (mgh).

7. D Since a nonconservative force (namely, friction) is acting during the motion, we use the modified Conservation of Mechanical Energy equation.

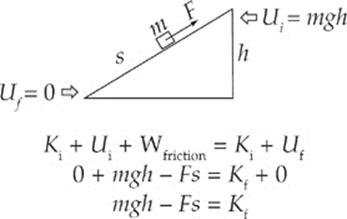
8. E Apply Conservation of Mechanical Energy (including the negative work done by F_r, the force of air resistance):

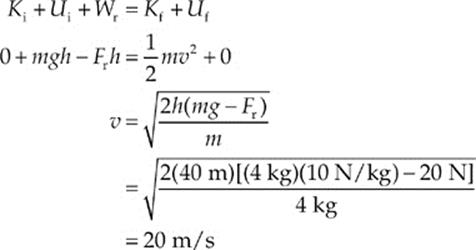
9. E Because the rock has lost half of its gravitational potential energy, its kinetic energy at the halfway point is half of its kinetic energy at impact. Since K is proportional to v², if K_{at halfway point} is equal to K_{at impact}, then the rock”s speed at the halfway point is its speed at impact.

10. D Using the equation P = Fv, we find that P = (200 N)(2 m/s) = 400 W.

SECTION II: FREE RESPONSE

1. (a) Applying Conservation of Energy,

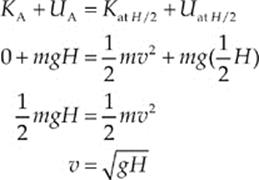
(b) Applying Conservation of Energy again,

(c) By the work–energy theorem, we want the work done by friction to be equal (but opposite) to the kinetic energy of the box at Point B:

Therefore,

(d) Apply Conservation of Energy (including the negative work done by friction as the box slides up the ramp from B to C):

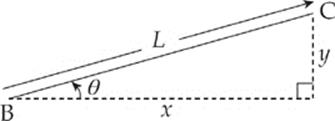
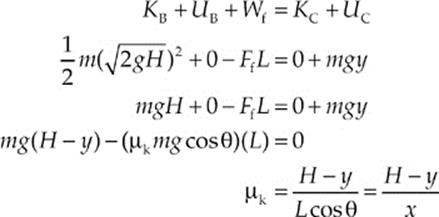
(e) The result of part (b) reads v_B = . Therefore, by Conservation of Mechanical Energy (with the work done by the frictional force on the slide included), we get

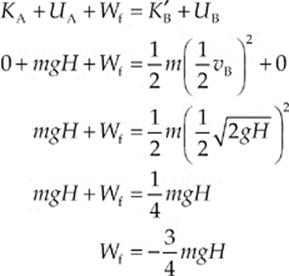
2. (a) The centripetal acceleration of the car at Point C is given by the equation a = v²_C/r, where v_C is the speed of the car at C. To find v_2C, we apply Conservation of Energy:

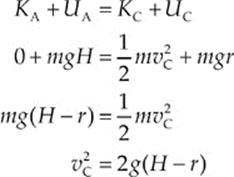
Therefore,

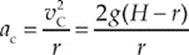
(b) When the car reaches Point D, the forces acting on the car are its weight, F_w, and the downward normal force, F_N, from the track. Thus, the net force, F_w + F_N, provides the centripetal force. In order for the car to maintain contact with the track, F_N must not vanish. Therefore, the cut-off speed for ensuring that the car makes it safely around the track is the speed at which F_N just becomes zero; any greater speed would imply that the car would make it around. Thus,

(c) Using the cut-off speed calculated in part (c), we now apply Conservation of Mechanical Energy:

(d) First, we calculate the car”s kinetic energy at Point B; then, we determine the distance x the car must travel from B to F for the work done by friction to eliminate this kinetic energy. So, applying Conservation of Mechanical Energy, we find

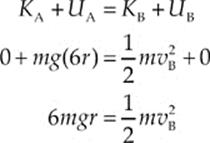
Now, by the work–energy theorem,

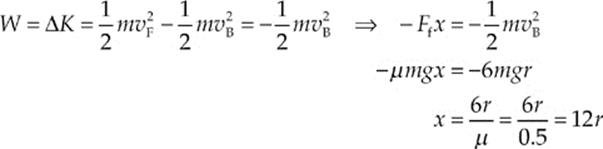
3. (a) Using conservation of energy K_i + U_i = K_f + U_f and v_i = 0 this becomes U_i = K_f + U_f or K_f = U_i – U_f. This is equivalent to mv² = mgh_i − mgh_f, which simplifies to v² − gh_i = −gh_f or h_f = h_i − . Now we can fill in the table on the next page.

(b) The greatest acceleration would occur where there is the greatest change in velocity. This occurs between 0.00 and 0.05 seconds. The acceleration during that time interval is given by 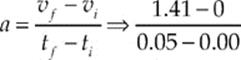or a = 2.8 m/s².

(c) Changing the mass does not effect the time spent falling or the velocity of the object. Thus, a change in mass will not affect the results.

CHAPTER 5 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. C The magnitude of the object”s linear momentum is p = mv. If p = 6 kg · m/s and m = 2 kg, then v = 3 m/s. Therefore, the object”s kinetic energy is K = mv² = (2 kg)(3 m/s)² = 9 J.

2. C The impulse delivered to the ball, J = F∆t, equals its change in momentum. Since the ball started from rest, we have

3. E The impulse delivered to the ball, J = Δt, equals its change in momentum. Thus,

4. D The impulse delivered to the ball is equal to its change in momentum. The momentum of the ball was mv before hitting the wall and m(–v) after. Therefore, the change in momentum is m(–v) – mv = –2mv, so the magnitude of the momentum change (and the impulse) is 2mv.

5. B By definition of perfectly inelastic, the objects move off together with one common velocity, v′, after the collision. By Conservation of Linear Momentum,

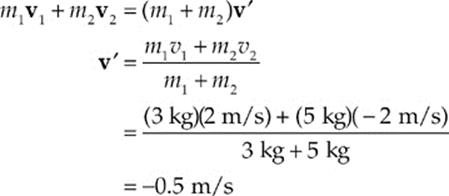
6. D First, apply Conservation of Linear Momentum to calculate the speed of the combined object after the (perfectly inelastic) collision:

Therefore, the ratio of the kinetic energy after the collision to the kinetic energy before the collision is

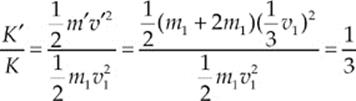
7. C Total linear momentum is conserved in a collision during which the net external force is zero. If kinetic energy is lost, then by definition, the collision is not elastic.

8. E Because the two carts are initially at rest, the initial momentum is zero. Therefore, the final total momentum must be zero.

9. D The linear momentum of the bullet must have the same magnitude as the linear momentum of the block in order for their combined momentum after impact to be zero. The block has momentum MV to the left, so the bullet must have momentum MV to the right. Since the bullet”s mass is m, its speed must be v = MV/m.

10. C In a perfectly inelastic collision, kinetic energy is never conserved; some of the initial kinetic energy is always lost to heat and some is converted to potential energy in the deformed shapes of the objects as they lock together.

SECTION II: FREE RESPONSE

1. (a) First draw a free-body diagram:

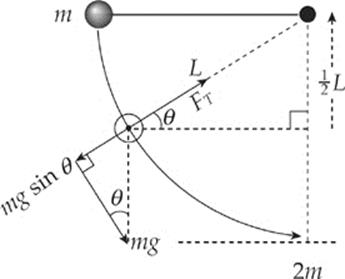
The net force toward the center of the steel ball”s circular path provides the centripetal force. From the geometry of the diagram, we have

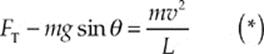
In order to determine the value of mv², we use Conservation of Mechanical Energy:

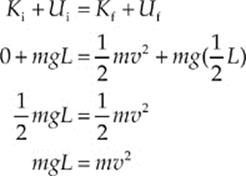
Substituting this result into Equation (*), we get

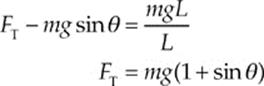
Now, from the free-body diagram we see that sin θ = L/L = , so

(b) Applying Conservation of Energy, we find the speed of the ball just before impact:

We can now use Conservation of Linear Momentum and the fact that kinetic energy is conserved to derive expressions for the speeds of the ball and block immediately after their collision. Since the collision is elastic, head-on, and the target object is at rest, this was done in Example 5.8. The velocity of the block after the collision is

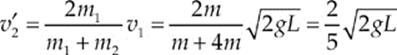
(c) We can quote the result of Example 5.8 to find the velocity of the ball immediately after the collision:

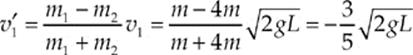
Now, applying Conservation of Mechanical Energy, we find

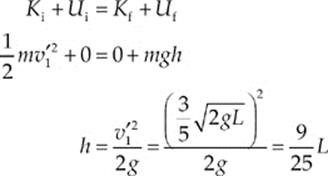
2. (a) By Conservation of Linear Momentum, mv = (m + M)v′, so v′ =

Now, by Conservation of Mechanical Energy,

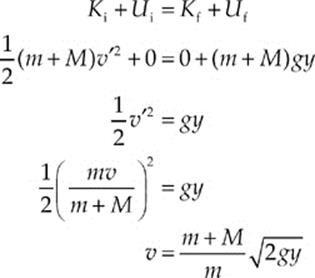
(b) Use the result derived in part (a) to compute the kinetic energy of the block and bullet immediately after the collision:

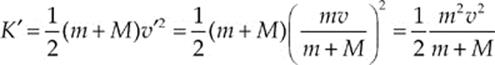
Since K = mv², the difference is

Therefore, the fraction of the bullet”s original kinetic energy that was lost is M/(m + M). This energy is manifested as heat (the bullet and block are warmer after the collision than before), and some was used to break the intermolecular bonds within the wooden block to allow the bullet to penetrate.

(c) From the geometry of the diagram,

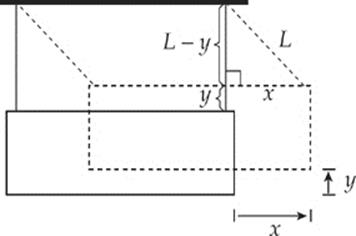
the Pythagorean theorem implies that (L − y)² + x² = L². Therefore,

(where we have used the fact that y² is small enough to be neglected). Substituting this into the result of part (a), we derive the following equation for the speed of the bullet in terms of x and L instead of y:

(d) No; momentum is conserved only when the net external force on the system is zero (or at least negligible). In this case, the block and bullet feel a net nonzero force that causes it to slow down as it swings upward. Since its speed is decreasing as it swings upward, its linear momentum cannot remain constant.

CHAPTER 6 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. E Neither the velocity nor the acceleration is constant because the direction of each of these vectors is always changing as the object moves along its circular path. And the net force on the object is not zero, because a centripetal force must be acting to provide the necessary centripetal acceleration to maintain the object”s circular motion.

2. B When the bucket is at the lowest point in its vertical circle, it feels a tension force F_T upward and the gravitational force F_w downward. The net force toward the center of the circle, which is the centripetal force, is F_T – F_w. Thus,

3. C When the bucket reaches the topmost point in its vertical circle, the forces acting on the bucket are its weight, F_w, and the downward tension force, F_T. The net force, F_w + F_T, provides the centripetal force. In order for the rope to avoid becoming slack, F_T must not vanish. Therefore, the cut-off speed for ensuring that the bucket makes it around the circle is the speed at which F_T just becomes zero; any greater speed would imply that the bucket would make it around. Thus,

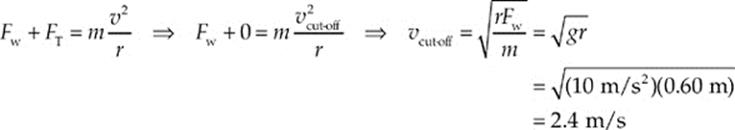
4. D Centripetal acceleration is given by the equation a_c = v²/r. Since the object covers a distance of 2πr in 1 revolution, its speed is 2πr s^–1. Therefore,

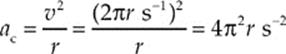
5. D The torque is τ = rF = (0.20 m)(20 N) = 4 N · m.

6. D From the diagram,

we calculate that

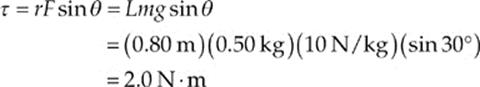
7. B The stick will remain at rest in the horizontal position if the torques about the suspension point are balanced:

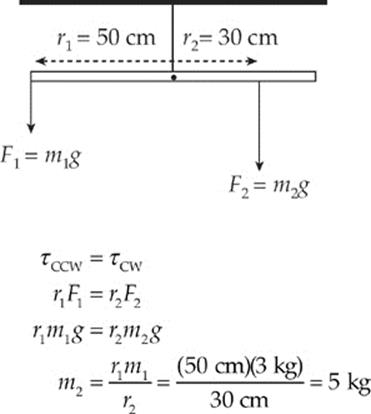
8. A Gravitational force obeys an inverse-square law: F_grav ∝ 1/r². Therefore, if r increases by a factor of 2, then F_grav decreases by a factor of 2² = 4.

9. E Mass is an intrinsic property of an object and does not change with location. This eliminates choices (A) and (C). If an object”s height above the surface of the earth is equal to 2R_E, then its distance from the center of the earth is 3R_E. Thus, the object”s distance from the earth”s center increases by a factor of 3, so its weight decreases by a factor of 3² = 9.

10. C The gravitational force that the Moon exerts on the planet is equal in magnitude to the gravitational force that the planet exerts on the Moon (Newton”s Third Law).

11. D The gravitational acceleration at the surface of a planet of mass M and radius R is given by the equation g = GM/R². Therefore, for the dwarf planet Pluto:

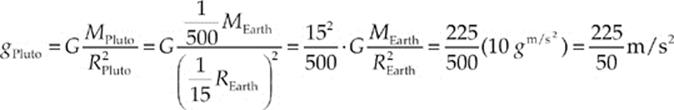
12. B The gravitational pull by the earth provides the centripetal force on the satellite, so GMm/R² = mv²/R. This gives mv² = GMm/2R, so the kinetic energy K of the satellite is inversely proportional to R. Therefore if R increases by a factor of 2, then K decreases by a factor of 2.

13. E The gravitational pull by Jupiter provides the centripetal force on its moon:

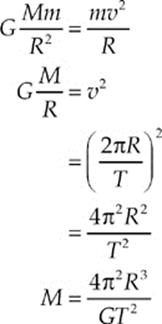
14. E Let the object”s distance from Body A be x; then its distance from Body B is R – x. In order for the object to feel no net gravitational force, the gravitational pull by A must balance the gravitational pull by B. Therefore, if we let M denote the mass of the object, then

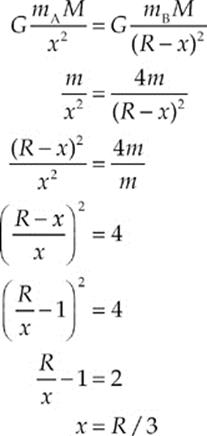
15. B Because the planet is spinning clockwise and the velocity is tangent to the circle, the velocity must point down. The acceleration and force point toward the center of the circle.

16. B If there were no forces or balanced forces in and out the satellite would have a net force of zero. If the net force were zero, the satellite would continue in a straight line and not orbit the planet.

SECTION II: FREE RESPONSE

1. (a) Given a mass of 1 kg, weight F_g = 5N, and diameter = 8 × 10⁶ m (this gives us r = 4 × 10⁶ m), we can fill in this equation:

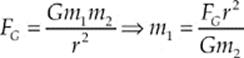
This becomes:

(b)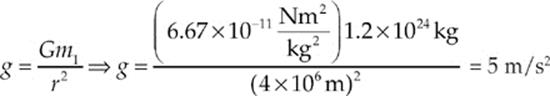

Note that we could have also observed that, because a 1 kg mass (which normally weighs 10 N on the surface of the earth) only weighed 5 N, gravity on this planet must be half the earth”s gravity.

If you want to look at it in terms of g, g is 10 m/s² on Earth, so we can simply convert

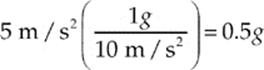
(c) Density is given by mass per unit volume (ρ = ). We also use the equation for the volume of a sphere as V = πr³ to get

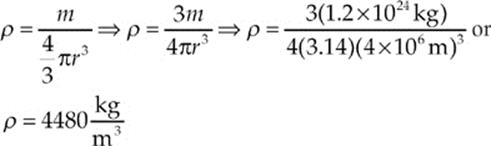
Using Kepler”s Third Law 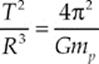, where m_p is the weight of the uncharted planet, we can solve for the period.

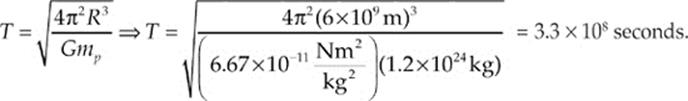
2. (a) The centripetal acceleration is given by the equation a_c = . We also know that for objects traveling in circles (Earth”s orbit can be considered a circle), v = . Substituting this v into the above equation for centripetal acceleration, we get a_c = 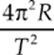. This becomes a_c = 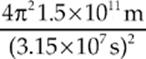= 6.0 × 10⁻³ m/s².

(b) The gravitational force is the force that keeps the earth traveling in a circle around the Sun. More specifically, ma_c = (6 × 10²⁴ kg)6.0 × 10^–3 m/s².

(c) Using Kepler”s Third Law 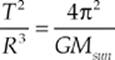, we can solve for the mass of the planet:

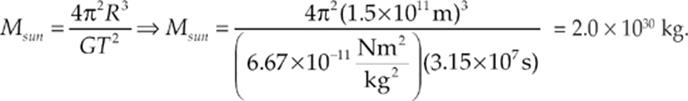
3. (a) The forces acting on a person standing against the cylinder wall are gravity (F_w, downward), the normal force from the wall (F_N, directed toward the center of the cylinder), and the force of static friction (F_f, directed upward):

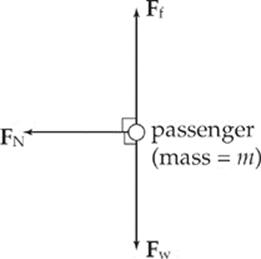
(b) In order to keep the passenger from sliding down the wall, the maximum force of static friction must be at least as great as the passenger”s weight: F_{f (max)} ≥ mg. Since F_{f (max)} = µ_sF_N, this condition becomes

Now, consider the circular motion of the passenger. Neither F_f nor F_w has a component toward the center of the path, so the centripetal force is provided entirely by the normal force:

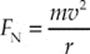
Substituting this expression for F_N into the previous equation, we get

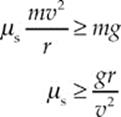
Therefore, the coefficient of static friction between the passenger and the wall of the cylinder must satisfy this condition in order to keep the passenger from sliding down.

(c) Since the mass m canceled out in deriving the expression for µ_s, the conditions are independent of mass. Thus, the inequality µ_s ≥ gr/v² holds for both the adult passenger of mass m and the child of mass m/2.

4. (a) The forces acting on the car are gravity (F_w, downward), the normal force from the road (F_N, upward), and the force of static friction (F_f, directed toward the center of curvature of the road):

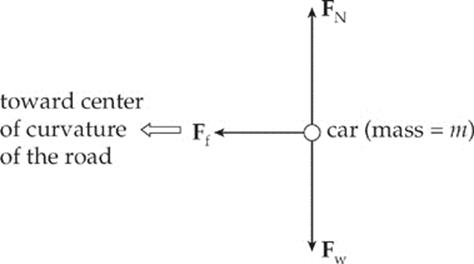
(b) The force of static friction [we assume static friction because we don”t want the car to slide (that is, skid)] provides the necessary centripetal force:

Therefore, to find the maximum speed at which static friction can continue to provide the necessary force, we write

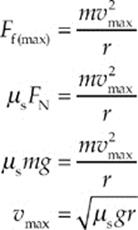
(c) Ignoring friction, the forces acting on the car are gravity (F_w, downward) and the normal force from the road (F_N, which is now tilted toward the center of curvature of the road):

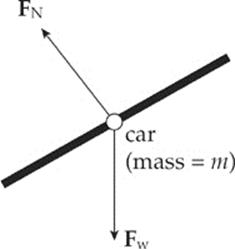
(d) Because of the banking of the turn, the normal force is tilted toward the center of curvature of the road. The component of F_N toward the center can provide the centripetal force, making reliance on friction unnecessary.

However, there”s no vertical acceleration, so we can say that there is no net vertical force. Therefore, F_N cos θ = F_w = mg, so F_N = mg/cos θ. The component of F_N toward the center of curvature of the turn, F_N sin θ, provides the centripetal force:

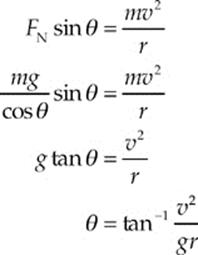
CHAPTER 7 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. D The acceleration of a simple harmonic oscillator is not constant, since the restoring force—and, consequently, the acceleration—depends on position. Therefore Statement I is false. However, both Statements II and III are fundamental, defining characteristics of simple harmonic motion.

2. C The acceleration of the block has its maximum magnitude at the points where its displacement from equilibrium has the maximum magnitude (since a = F/m = kx/m). At the endpoints of the oscillation region, the potential energy is maximized and the kinetic energy (and hence the speed) is zero.

3. E By Conservation of Mechanical Energy, K + U_S is a constant for the motion of the block. At the endpoints of the oscillation region, the block”s displacement, x, is equal to ±A. Since K = 0 here, all the energy is in the form of potential energy of the spring, kA². Because kA² gives the total energy at these positions, it also gives the total energy at any other position.

Using the equation U_S(x) = kx², we find that, at x = A,

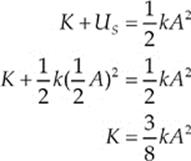
Therefore,

4. C As we derived in Example 7.2, the maximum speed of the block is given by the equation v_max = A. Therefore, v_max is inversely proportional to . If m is increased by a factor of 2, then v_max will decrease by a factor of .

5. D The period of a spring–block simple harmonic oscillator is independent of the value of g. (Recall that T = 2π.) Therefore, the period will remain the same.

6. D The frequency of a spring–block simple harmonic oscillator is given by the equation f = (1/2π). Squaring both sides of this equation, we get f ² = (k/4π²)(1/m). Therefore, if f² is plotted vs. (1/m), then the graph will be a straight line with slope k/4π². (Note: The slope of the line whose equation is y = ax is a.)

7. C For small angular displacements, the period of a simple pendulum is essentially independent of amplitude.

SECTION II: FREE RESPONSE

1. (a) Since the spring is compressed to 3/4 of its natural length, the block”s position relative to equilibrium is x = −L. Therefore, from F_S = –kx, we find

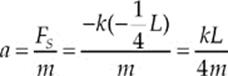
(b) Let v₁ denote the velocity of Block 1 just before impact, and let v′₁ and v′₂ denote, respectively, the velocities of Block 1 and Block 2 immediately after impact. By conservation of linear momentum, we write mv₁ = mv′₁ + mv′₂, or

The initial kinetic energy of Block 1 is mv²₁. If half is lost to heat, then mv²₁ is left to be shared by Block 1 and Block 2 after impact: mv²₁ + mv′²₁ + mv′²₂, or

Square Equation (1) and multiply by 2 to give

then subtract Equation (2) from Equation (1′):

Square Equation (1) again,

and substitute into this the result of Equation (3):

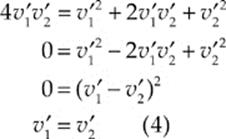
Thus, combining Equations (1) and (4), we find that

(c) When Block 1 reaches its new amplitude position, A′, all of its kinetic energy is converted to elastic potential energy of the spring. That is,

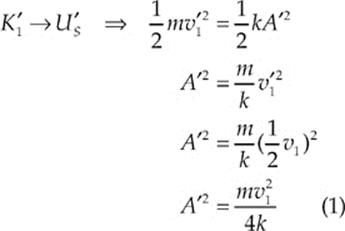
But the original potential energy of the spring, U_S = k(−L)², gave K₁:

Substituting this result into Equation (1) gives

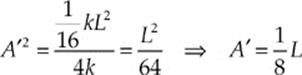
(d) The period of a spring–block simple harmonic oscillator depends only on the spring constant k and the mass of the block. Since neither of these changes, the period will remain the same; that is, T′ = T₀.

(e) As we showed in part (b), Block 2”s velocity as it slides off the table is v₁ (horizontally). The time required to drop the vertical distance H is found as follows (calling down the positive direction):

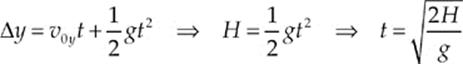
Therefore,

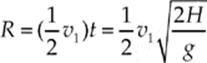
Now, from Equation (2) of part (c), v₁ = , so

2. (a) By conservation of linear momentum,

(b) When the block is at its amplitude position (maximum compression of spring), the kinetic energy it (and the embedded bullet) had just after impact will become potential energy of the spring:

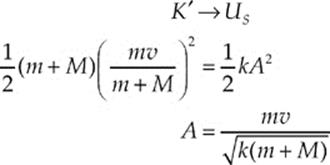
(c) Since the mass on the spring is m + M, f = .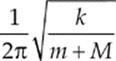

(d) The position of the block is given by the equation x = A sin(ωt + φ₀), where ω = 2πf and A is the amplitude. Since x = 0 at time t = 0, the initial phase, φ₀, is 0. From the results of parts (b) and (c), we have

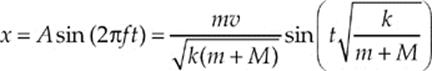
3. (a) By Conservation of Mechanical Energy, K + U = E, so

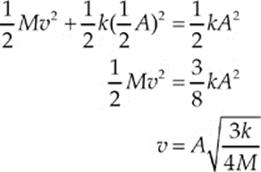
(b) Since the clay ball delivers no horizontal linear momentum to the block, horizontal linear momentum is conserved. Thus,

(c) Applying the general equation for the period of a spring–block simple harmonic oscillator,

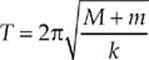
(d) The total energy of the oscillator after the clay hits is kA′², where A′ is the new amplitude. Just after the clay hits the block, the total energy is

Substitute for v′ from part (b), set the resulting sum equal to kA′², and solve for A′.

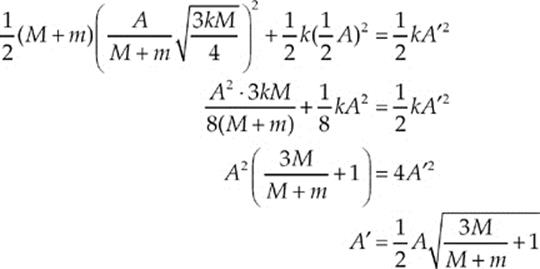
(e) No, since the period depends only on the mass and the spring constant k.

(f) Yes. For example, if the clay had landed when the block was at x = A, the speed of the block would have been zero immediately before the collision and immediately after. No change in the block”s speed would have meant no change in K, so no change in E, so no change in A = .

CHAPTER 8 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. D Since Point X is 5 m below the surface of the water, the pressure due to the water at X, P_X, is ρgh_X = ρg(5 m), where ρ is the density of water. Because Point Y is 4 m below the surface of the water, the pressure due to the water at Y, p_Y, is ρg(4 m). Therefore,

2. C Because the top of the box is at a depth of D – z below the surface of the liquid, the pressure on the top of the box is

The area of the top of the box is A = xy, so the force on the top of the box is

3. C Let P₀ be the pressure of the gas at the surface of the liquid. Then the pressures at points Y and Z are, respectively,

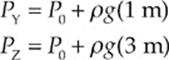
Subtracting the first equation from the second, we get P_Z – P_Y = ρg(2 m). Because we”re given the values of P_Y and P_Z, we know that P_Z – P_Y = 16,000 Pa, so we can write

Substituting this into the equation for P_Y, we find that

4. A The density of the plastic cube is

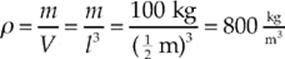
This is 4/5 the density of the water, so 4/5 of the cube”s volume is submerged; this means that 1/5 of the cube”s volume is above the surface of the water.

5. B The buoyant force on the styrofoam block is F_buoy = ρ_LVg, and the weight of the block is F_g = m_Sg = ρ_SVg. Because ρ_L > ρ_S, the net force on the block is upward and has magnitude

Therefore, by Newton”s Second Law, we have

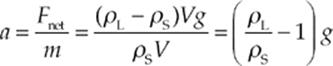
6. B The buoyant force acting on the ball is

The weight of the ball is

Because the upward force on the ball, F_buoy, balances the total downward force, F_g + F_T, the tension in the string is

F_T = F_buoy – F_g = 50 N – 20 N = 30 N

7. A If the object weighs 100 N less when completely submerged in water, the buoyant force must be 100 N; therefore

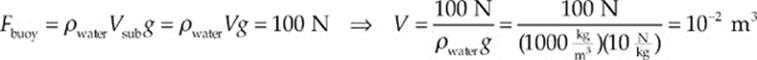
Now that we know the volume of the object, we can figure out its weight:

8. A The cross-sectional diameter at Y is 3 times the cross-sectional diameter at X, so the cross-sectional area at Y is 3² = 9 times that at X. The Continuity Equation tells us that the flow speed, v, is inversely proportional to the cross-sectional area, A. So, if A is 9 times greater at Point Y than it is at X, then the flow speed at Y is 1/9 the flow speed at X; that is, v_Y = (1/9)v_X = (1/9)(6 m/s) = 2/3 m/s.

9. E Each side of the rectangle at the bottom of the conduit is 1/4 the length of the corresponding side at the top. Therefore, the cross-sectional area at the bottom is (1/4)² = 1/16 the cross-sectional area at the top. The Continuity Equation tells us that the flow speed, v, is inversely proportional to the cross-sectional area, A. So, if A at the bottom is 1/16 the value of A at the top, then the flow speed at the bottom is 16 times the flow speed at the top.

10. D We”ll apply Bernoulli”s Equation to a point at the pump (Point 1) and at the nozzle (the exit point, Point 2). We”ll choose the level of Point 1 as the horizontal reference level; this makes y₁ = 0 and y₂ = 1 m. Now, because the cross-sectional diameter decreases by a factor of 10 between Points 1 and 2, the cross-sectional area decreases by a factor of 10² = 100, so flow speed must increase by a factor of 100; that is, v₂ = 100v₁ = 100(0.4 m/s) = 40 m/s. Because the pressure at Point 2 is P_atm, Bernoulli”s Equation becomes

Therefore,

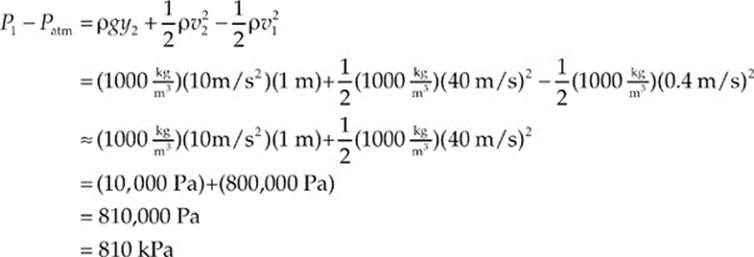
SECTION II: FREE RESPONSE

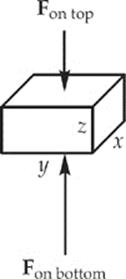
1. (a) The pressure at the top surface of the block is P_top = P_atm + ρ_Lgh. Since the area of the top of the block is A = xy, the force on the top of the block has magnitude

The pressure at the bottom of the block is P_bottom = P_atm + ρ_Lg(h + z). Since the area of the bottom face of the block is also A = xy, the force on the bottom surface of the block has magnitude

These forces are sketched below:

(b) Each of the other four faces of the block (left and right, front and back) is at an average depth of h + z, so the average pressure on each of these four sides is

The left and right faces each have area A = xz, so the magnitude of the average force on this pair of faces is

The front and back faces each have area A = yz, so the magnitude of the average force on this pair of faces is

These forces are sketched below:

(c) The four forces sketched in part (b) add up to zero, so the total force on the block due to the pressure is the sum of F_{on top} and F_{on bottom}; because F_{on bottom} > F_{on top}, this total force points upward and its magnitude is

(d) By Archimedes” Principle, the buoyant force on the block is upward and has magnitude

This is the same as the result we found in part (c).

(e) The weight of the block is

If F_T is the tension in the string, then the total upward force on the block, F_T + F_buoy, must balance the downward force, F_g; that is, F_T + F_buoy = F_g, so

2. (a) See the section on Torricelli”s theorem for the derivation of the efflux speed from the hole; applying Bernoulli”s Equation to a point on the surface of the water in the tank (Point 1) and a point at the hole (Point 2), the assumption that v₁ ≈ 0 leads to the result

(b) The initial velocity of the water, as it emerges from the hole, is horizontal. Since there”s no initial vertical velocity, the time t required to drop the distance y = D – h to the ground is found as follows:

Therefore, the horizontal distance the water travels is

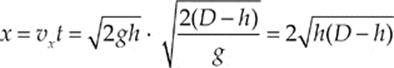
(c) The second hole would be at a depth of h/2 below the surface of the water, so the horizontal distance it travels—from the edge of the tank to the point where it hits the ground—is given by the same formula we found in part (b) except it will have h/2 in place of h; that is,

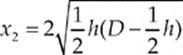
If both streams land at the same point, then the value of x from part (b) is the same as x₂:

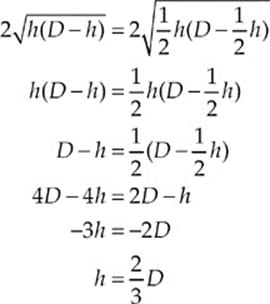
(d) Once again, we apply Bernoulli”s Equation to a point on the surface of the water in the tank (Point 1) and to a point at the hole (Point 2). We”ll choose the ground level as our horizontal reference level; then y₁ = D and y₂ = D – h. If v₁ is the flow speed of Point 1—that is, the speed with which the water level in the tank drops—and v₂ is the efflux speed from the hole, then, by the Continuity Equation, A₁v₁ = A₂v₂, where A₁ and A₂ are the cross-sectional areas at Points 1 and 2, respectively. Therefore, v₁ = (A₂/A₁)v₂. Bernoulli”s Equation then becomes

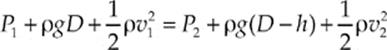
Since P₁ = P₂ = P_atm, these terms cancel out; and substituting v₁ = (A₂/A₁)v₂, we have

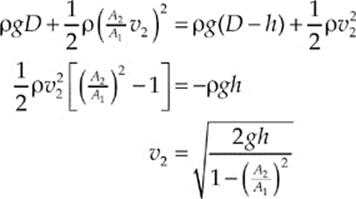
Now, since A₁ = πR² and A₂ = πr², this final equation can be written as

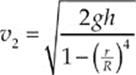
[Note that if r << R, then (r/R)⁴ ≈ 0, and the equation above reduces to v₂ = , as in part (a).]

3. (a) Point X is at a depth of h₁ below Point 1, where the pressure is P₁. Therefore, the hydrostatic pressure at X is P_X = P₁ + ρ_Fgh₁.

(b) Point Y is at a depth of h₂ + d below Point 2, where the pressure is P₂. The column of static fluid above Point Y contains fluid of density of depth h₂ and fluid of density ρ_F of depth d. Therefore, the hydrostatic pressure at Y is P_Y = P₂ + ρgh₂ + ρ_Vgd.

(c) First, notice that Points 1 and 2 are at the same horizontal level; therefore, the heights y₁ and y₂ are the same, and the terms ρ_Fgy₁ and ρ_Fgy₂ will cancel out of the equation. Bernoulli”s Equation then becomes

By the Continuity Equation, we have A₁v₁ = A₂v₂, so v₁ = (A₂/A₁)v₂. Therefore,

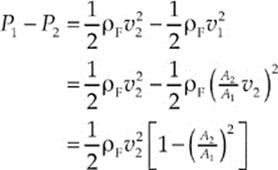
(d) In parts (a) and (b) above, we found that P_X = P₁ + ρ_Fgh₁ and P_Y = P₂ + ρ_Fgh₂+ρ_vgd. Since P_X = P_Y, we have

so

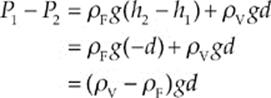
(e) In parts (c) and (d), we found two expressions for P₁ – P₂. Setting them equal to each other gives

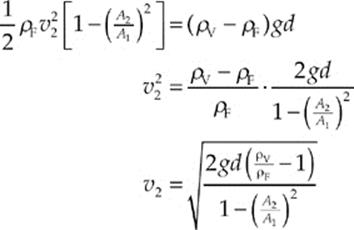
The flow rate in the pipe is

Since

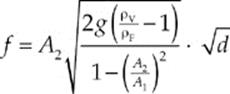
we see that f is proportional to , as desired.

CHAPTER 9 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. C Because the average kinetic energy of a molecule of gas is directly proportional to the temperature of the sample, the fact that the gases are at the same temperature—since they”re in the same container at thermal equilibrium—tells us that the molecules have the same average kinetic energy. The ratio of their kinetic energies is therefore equal to 1.

2. E From the Ideal Gas Law, we know that P = nRT/V. If both T and V are doubled, the ratio T/V remains unchanged, so P remains unchanged.

3. A The work done on the gas during a thermodynamic process is equal to the area of the region in the P–V diagram above the V-axis and below the path the system takes from its initial state to its final state. Since the area below path 1 is the greatest, the work done on the gas during the transformation along path 1 is the greatest.

4. C During an isothermal change, ∆U is always zero.

5. B Because the gas is confined, n remains constant, and because we”re told the volume is fixed, V remains constant as well. Since R is a universal constant, the Ideal Gas Law, PV = nRT, tells us that P and T are proportional. Therefore, if T increases by a factor of 2, then so does P.

6. E Neither choice (A) nor (B) can be correct. Using PV = nRT, both containers have the same V, n is the same, P is the same, and R is a universal constant. Therefore, T must be the same for both samples. Choice (C) is also wrong, since R is a universal constant. The kinetic theory of gases predicts that the rms speed of the gas molecules in a sample of molar mass M and temperature T is

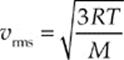
Hydrogen has a smaller molar mass than does helium, so v_rms for hydrogen must be greater than v_rms for helium (because both samples are at the same T).

7. A By convention, work done on the gas sample is designated as positive, so in the First Law of Thermodynamics, ∆U = Q + W, we must write W = +320 J. Therefore, Q = ∆U – W = 560 J – C 320 J = +240 J. Positive Q denotes heat in.

8. C No work is done during the step from state a to state b because the volume doesn”t change. Therefore, the work done from a to c is equal to the work done from b to c. Since the pressure remains constant (this step is isobaric), we find that

W = –P∆V = –(3.0 × 10⁵ Pa)[(10 – 25) × 10^–3 m³] = 4500 J

9. D Statement (A) is wrong because “no heat exchanged between the gas and its surroundings” is the definition of adiabatic, not isothermal. Statement (B) cannot be correct since the step described in the question is isothermal; by definition, the temperature does not change. This also eliminates statement (E) and supports statement (D). Statement (C) is false, because although the heat absorbed is converted completely to work (∆U = 0 since ∆T = 0, so, by the First Law of Thermodynamics, W = –Q_H), the Second Law of Thermodynamics is not violated. If the sample could be brought back to its initial state and have a 100% conversion of heat to work, that would violate the Second Law. The Second Law states that heat cannot be completely converted to work with no other change taking place. In this case, there are changes taking place: The pressure decreases and the volume increases.

10. D Be careful! Make sure that you always use absolute temperatures (in kelvins) when computing the Carnot efficiency, not temperatures in degrees Celsius. Since T_H = 800°C = 1073 K and T_C = 200°C = 473 K, the Carnot efficiency is

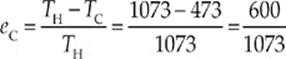
This is a little less than 60%, which best matches 56%.

SECTION II: FREE RESPONSE

1. (a) First, let”s calculate ∆U_acb. Using path acb, the question tells us that Q = +70 J and W = –30 J (W is negative here because it is the system that does the work). The First Law, ∆U = Q + W, then tells us that ∆U_acb = +40 J. Because ∆U_a→b does not depend on the path taken from a to b, we must have ∆U_ab = +40 J, and ∆U_ba = –∆U_ab = –40 J. Thus, –40 J = Q_ba + W_ba, where Q_baand W_ba are the values along the curved path from b to a. Since Q_ba = –60 J, it follows that W_ba = +20 J. Therefore, the surroundings do 20 J of work on the system.

(b) Again, using the fact that ∆U_a→b does not depend on the path taken from a to b, we know that ∆U_adb = +40 J, as computed above. Writing ∆U_adb = QW_adb + W_adb, if W_adb= –10 J, it follows that Q_adb = +50 J. That is, the system absorbs 50 J of heat.

(c) For the process db, there is no change in volume, so W_db = 0. Therefore, ∆U_db= Q_db + W_db = Q_db. Now, since U_ab = +40 J, the fact that U_a = 0 J implies that U_b = 40 J, so ∆U_db = U_b – U_d = 40 J – 30 J = 10 J. Thus, Q_db = 10 J. Now let”s consider the process ad. Since W_adb = W_ad + W_db = W_ad + 0 = W_ad, the fact that W_adb = –10 J [computed in part (c)] tells us that W_ad = –10 J. Because ∆U_ad = U_d – U_a = 30 J, it follows from ∆U_ad = Q_ad + W_ad that Q_ad = ∆U_ad – W_ad = 30 J – (–10 J) = 40 J.

(d) The process adbca is cyclic, so ∆U is zero. Because this cyclic process is traversed counterclockwise in the P–V diagram, we know that W is positive. Then, since ∆U = Q + W, it follows that Q must be negative.

2. (a) (i) Use the Ideal Gas Law:

(a) (ii) Since state b is on the isotherm with state a, the temperature of state b must also be 870 K.

(a) (iii) Use the Ideal Gas Law:

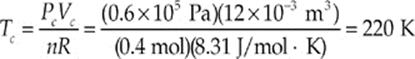
(b) (i) Since step ab takes place along an isotherm, the temperature of the gas does not change, so neither does the internal energy; ∆U_ab = 0.

(b) (ii) By the First Law of Thermodynamics, ∆U_bc = Q_bc + W_bc. Since step bc is isobaric (constant pressure), we have

Next,

Therefore,

(b) (iii) By the First Law of Thermodynamics, ∆U_ca = Q_ca + W_ca. Since step ca is isochoric (constant volume), we have

and W_ca = 0. Therefore, ∆U_ca = Q_ca + W_ca = (5400 J) + 0 = 5400 J.

Note that part (iii) could have been answered as follows:

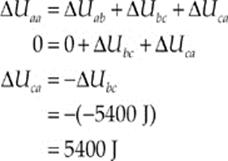
(c) Using the equation given, we find that

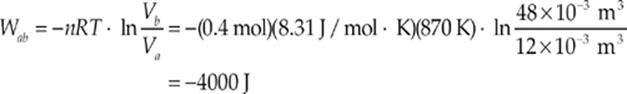
(d) The total work done over the cycle is equal to the sum of the values of the work done over each step:

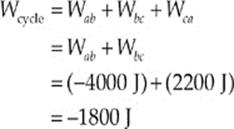
(e) (i) and (ii) By the First Law of Thermodynamics,

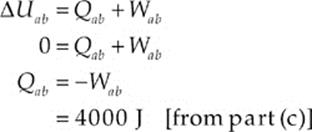
Since Q is positive, this represents heat absorbed by the gas.

(f) The maximum possible efficiency is the efficiency of a Carnot engine:

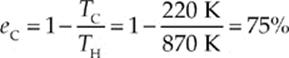
CHAPTER 10 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. D Electrostatic force obeys an inverse-square law: F_E ∝ 1/r². Therefore, if r increases by a factor of 3, then F_E decreases by a factor of 3² = 9.

2. C The strength of the electric force is given by kq²/r², and the strength of the gravitational force is Gm²/r². Since both of these quantities have r² in the denominator, we simply need to compare the numerical values of kq² and Gm². There”s no contest: Since

and

we see that kq² >> Gm², so F_E is much stronger than F_G.

3. C If the net electric force on the center charge is zero, the electrical repulsion by the +2q charge must balance the electrical repulsion by the +3q charge:

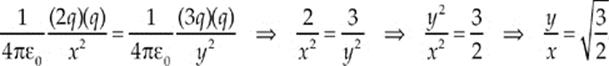
4. E Since P is equidistant from the two charges, and the magnitudes of the charges are identical, the strength of the electric field at P due to +Q is the same as the strength of the electric field at P due to –Q. The electric field vector at P due to +Q points away from +Q, and the electric field vector at P due to –Q points toward –Q. Since these vectors point in the same direction, the net electric field at P is (E to the right) + (E to the right) = (2E to the right).

5. D The acceleration of the small sphere is

As r increases (that is, as the small sphere is pushed away), a decreases. However, since a is always positive, the small sphere”s speed, v, is always increasing.

6. B Since F_E (on q) = qE, it must be true that F_E (on –2q) = –2qE = –2F_E.

7. D All excess electric charge on a conductor resides on the outer surface.

SECTION II: FREE RESPONSE

1. (a) From the figure below, we have F_1-2 = F₁/cos 45°.

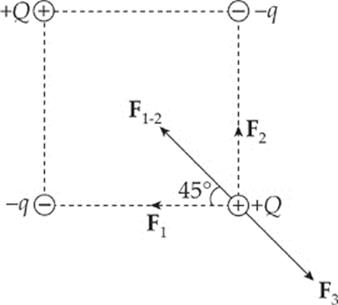
Since the net force on +Q is zero, we want F_1-2 = F₃. If s is the length of each side of the square, then:

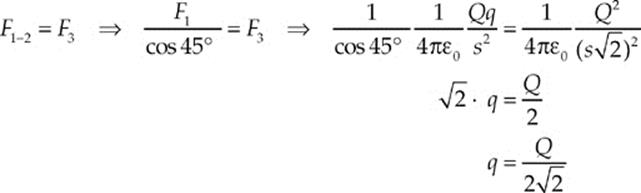
(b) No. If q = Q/2, as found in part (a), then the net force on –q is not zero.

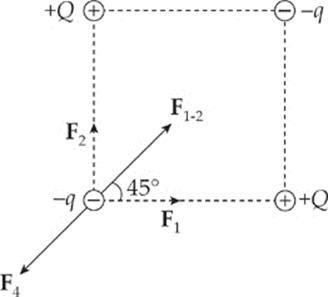
This is because F_1-2 ≠ F₄, as the following calculations show:

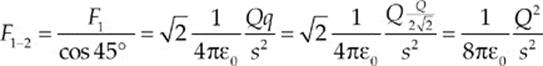
but

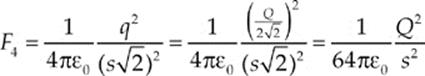
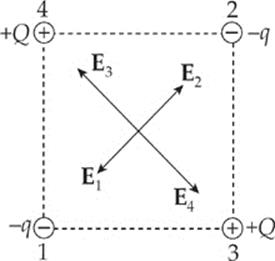
(c) By symmetry, E₁ = E₂ and E₃ = E₄, so the net electric field at the center of the square is zero:

2. (a) The magnitude of the electric force on Charge 1 is

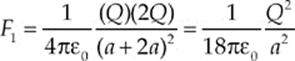
The direction of F₁ is directly away from Charge 2; that is, in the +y direction, so

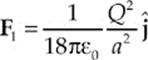
(b) The electric field vectors at the origin due to Charge 1 and due to Charge 2 are

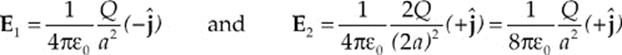
Therefore, the net electric field at the origin is

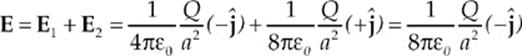
(c) No. The only point on the x axis where the individual electric field vectors due to each of the two charges point in exactly opposite directions is the origin (0,0). But at that point, the two vectors are not equal and thus do not cancel.

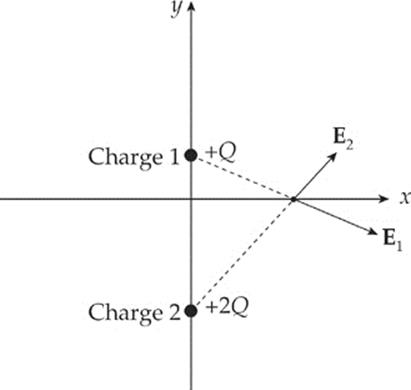
Therefore, at no point on the x axis could the total electric field be zero.

(d) Yes. There will be a Point P on the y axis between the two charges,

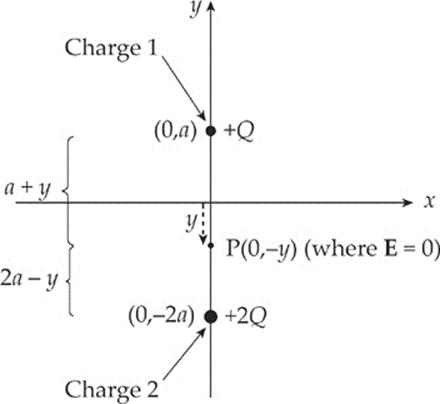
where the electric fields due to the individual charges will cancel each other out.

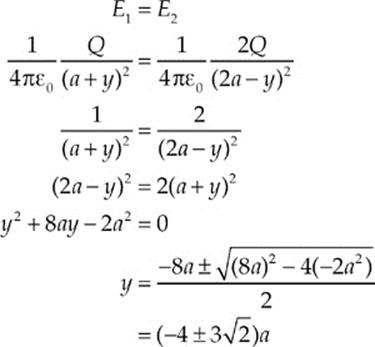
Disregarding the value y = (−4 − 3)a (because it would place the point P below Charge 2 on the y axis, where the electric field vectors do not point in opposite directions), we have that E = 0 at the point P = (0,–y) = (0, (4 − 3)a).

(e) Use the result of part (b) with Newton”s Second Law:

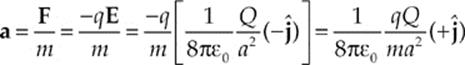
CHAPTER 11 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. E A counterexample for Statement I is provided by two equal positive charges; at the point midway between the charges, the electric field is zero, but the potential is not. A counterexample for Statement II is provided by an electric dipole (a pair of equal but opposite charges); at the point midway between the charges, the electric potential is zero, but the electric field is not. As for Statement III, consider a single positive point charge +Q. Then at a distance r from this source charge, the electric field strength is E = kQ/r² and the potential is V = kQ/r. Thus, V = rE, so V is not inversely proportional to E.

2. C By definition, ∆U_E = –W_E, so if W_E is negative, then ∆U_E is positive. This implies that the potential energy, U_E, increases.

3. B The work required to assemble the configuration is equal to the potential energy of the system:

4. B Use the definition ∆V = –W_E/q. If an electric field accelerates a negative charge doing positive work on it, then W_E > 0. If q < 0, then –W_E/q is positive. Therefore, ∆V is positive, which implies that V increases.

5. E By definition,

6. C Because E is uniform, the potential varies linearly with distance from either plate (∆V = Ed). Since Points 2 and 4 are at the same distance from the plates, they lie on the same equipotential. (The equipotentials in this case are planes parallel to the capacitor plates.)

7. B By definition, W_E = –q∆V, which gives

Note that neither the length of the segment AB nor that of the curved path from A to B is relevant.

8. D

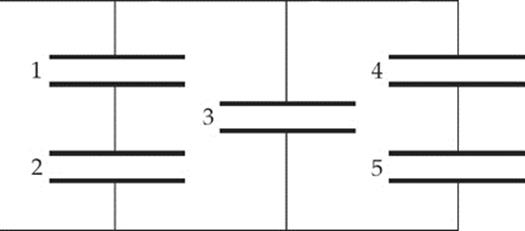
Capacitors 1 and 2 are in series, so their equivalent capacitance is C_1-2 = C/2. (This is obtained from the equation 1/C_1-2 = 1/C₁ + 1/C₂ = 1/C + 1/C = 2/C.) Capacitors 4 and 5 are also in series, so their equivalent capacitance is C_4-5 = C/2. The capacitances C_1-2, C₃, and C_4-5 are in parallel, so the overall equivalent capacitance is (C/2) + C + (C/2) = 2C.

SECTION II: FREE RESPONSE

1. (a) Labeling the four charges as given in the diagram, we get

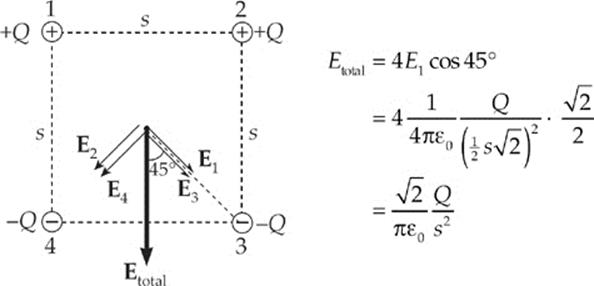
(b) Let E_i denote the electric field at the center of the square due to Charge i. Then by symmetry, E₁ = E₃, E₂ = E₄, and E₁ = E₂ = E₃ = E₄. The horizontal components of the four individual field vectors cancel, leaving only a downward-pointing electric field of magnitude E_total = 4E₁ cos 45°:

(c) The potential at the center of the square is zero:

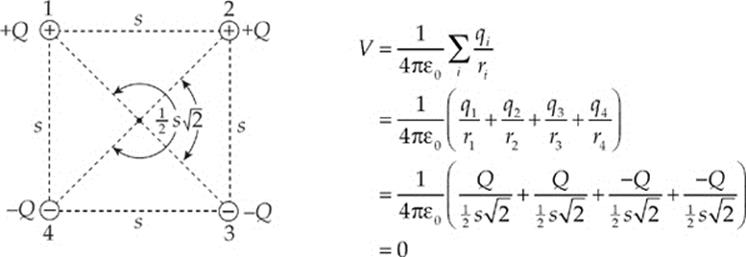
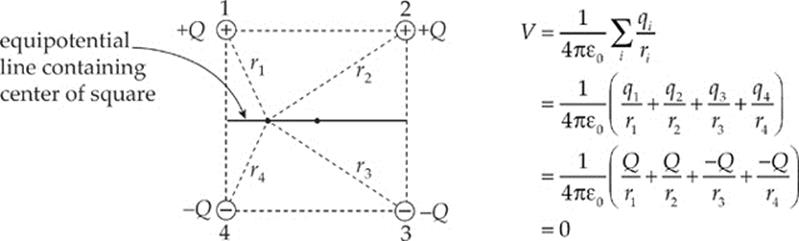
(d) At every point on the center horizontal line shown, r₁ = r₄ and r₂ = r₃, so V will equal zero (just as it does at the center of the square):

(e) The work done by the electric force as q is displaced from Point A to Point B is given by the equation W_E = –q∆V_A→B = –q(V_B – V_A) = –qV_B (since V_A = 0).

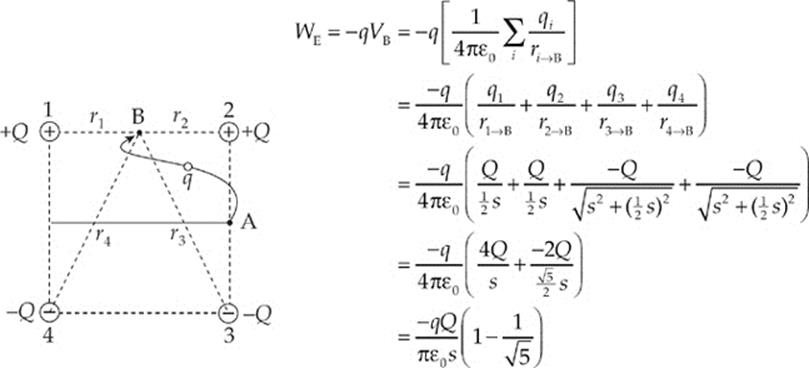
2. (a) The capacitance is C = ε₀A/d. Since the plates are rectangular, the area A is equal to Lw, so C = ε₀Lw/d.

(b) and (c) Since the electron is attracted upward, the top plate must be the positive plate:

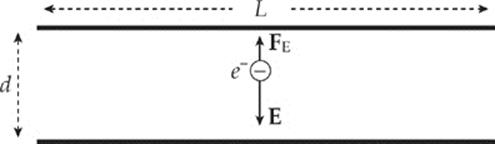
(d) The acceleration of the electron is a = F_E/m = qE/m = eE/m, vertically upward. Therefore, applying Big Five #3 for vertical motion, ∆y = v_0yt + a_yt², we get

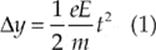
To find t, notice that v_0x = v₀ remains constant (because there is no horizontal acceleration). Therefore, the time necessary for the electron to travel the horizontal distance L is t = L/v₀. In this time, ∆y is d/2, so Equation (1) becomes

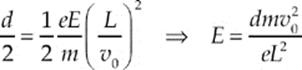
(e) Substituting the result of part (d) into the equation ∆V = Ed gives

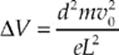
Since Q = C∆V (by definition), the result of part (a) now gives

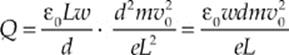
(f) Applying the equation U_E = C(∆V)², we get

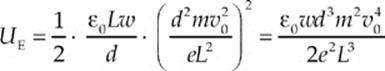
3. (a) Outside the sphere, the sphere behaves as if all the charge were concentrated at the center. Inside the sphere, the electrostatic field is zero:

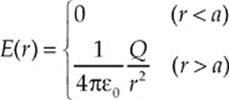
(b) On the surface and outside the sphere, the electric potential is (1/4πε₀)(Q/r). Within the sphere, V is constant (because E = 0) and equal to the value on the surface. Therefore,

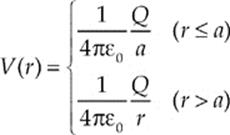
(c) See diagrams:

CHAPTER 12 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. A Let ρ_S denote the resistivity of silver and let A_S denote the cross-sectional area of the silver wire. Then

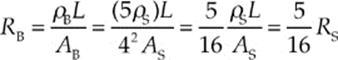
2. D The equation I = V/R implies that increasing V by a factor of 2 will cause I to increase by a factor of 2.

3. C Use the equation P = V²/R:

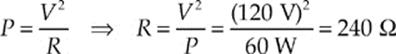
4. B The current through the circuit is

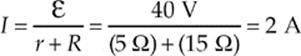
Therefore, the voltage drop across R is V = IR = (2 A)(15 Ω) = 30 V.

5. E The 12 Ω and 4 Ω resistors are in parallel and are equivalent to a single 3 Ω resistor, because 1/(12 Ω) + 1/(4 Ω) = 1/(3 Ω). This 3 Ω resistor is in series with the top 3 Ω resistor, giving an equivalent resistance in the top branch of 3 + 3 = 6 Ω. Finally, this 6 Ω resistor is in parallel with the bottom 3 Ω resistor, giving an overall equivalent resistance of 2 Ω, because 1/(6 Ω) + 1/(3 Ω) = 1/(2 Ω).

6. D If each of the identical bulbs has resistance R, then the current through each bulb is ε/R. This is unchanged if the middle branch is taken out of the parallel circuit. (What will change is the total amount of current provided by the battery.)

7. B The three parallel resistors are equivalent to a single 2 Ω resistor, because 1/(8 Ω) + 1/(4 Ω) + 1/(8 Ω) = 1/(2 Ω). This 2 Ω resistance is in series with the given 2 Ω resistor, so their equivalent resistance is 2 + 2 = 4 Ω. Therefore, three times as much current will flow through this equivalent 4 Ω resistance in the top branch as through the parallel 12 Ω resistor in the bottom branch, which implies that the current through the bottom branch is 3 A, and the current through the top branch is 9 A. The voltage drop across the 12 Ω resistor is therefore V = IR = (3 A)(12 Ω) = 36 V.

8. E Since points a and b are grounded, they”re at the same potential (call it zero).

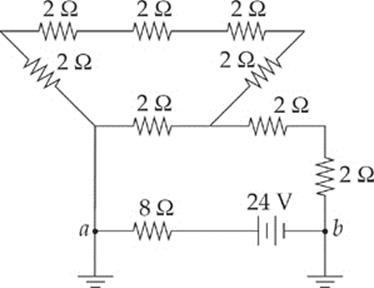
Traveling from b to a across the battery, the potential increases by 24 V, so it must decrease by 24 V across the 8 Ω resistor as we reach point a. Thus, I = V/R = (24 V)/(8 Ω) = 3 A.

9. D The equation P = I²R gives

Therefore, in 20 s, the energy dissipated as heat is

SECTION II: FREE RESPONSE

1. (a) The two parallel branches, the one containing the 40 Ω resistor and the other a total of 120 Ω, is equivalent to a single 30 Ω resistance. This 30 Ω resistance is in series with the three 10 Ω resistors, giving an overall equivalent circuit resistance of 10 + 10 + 30 + 10 = 60 Ω. Therefore, the current supplied by the battery is I = V/R = (120V)/(60) = 2 A, so it must supply energy at a rate of P = IV = (2 A)(120 V) = 240 W.

(b) Since three times as much current will flow through the 40 Ω resistor as through the branch containing 120 Ω of resistance, the current through the 40 Ω resistor must be 1.5 A.

(c) (i) V_a – V_b = IR₂₀ + IR₁₀₀ = (0.5 A)(20 Ω) + (0.5 A)(100 Ω) = 60 V.

(ii) Point a is at the higher potential (current flows from high to low potential).

(d) Because energy is equal to power multiplied by time, we get

(e) Using the equation R = ρL/A, with A = πr², we find

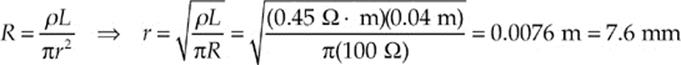
2. (a) There are many ways to solve this problem. If you notice that each of the three branches of the parallel section is 60 Ω, then they must all have the same current flowing through them. The currents through the 20 Ω, 40 Ω, and other 60 Ω resistor are all 0.5A.

If you had not noticed this, you would have used Ohm”s Law to determine the voltage across the resistors and proceeded from there (see part b below). Because 0.5A goes through each of the three pathways, Kirchhoff”s Junction Rule tells us that the current that must have come though the 10Ω resistor is 1.5A.

(b) The voltage across the 60 Ω resistor is given by Ohm”s Law. Because V = IR, V = (0.5 A)(60 Ω) = 30V. All three parallel branches must have the same voltage across them, so the other 60 Ω resistor also has 30V across it and the combination of the 20 Ω and 60 Ω resistor must also be 30 V. To determine the voltage across the 20 Ω and 40 Ω resistor you can rely on the previously solved currents of 0.5A and Ohm”s Law to yield V = IR = (0.5 A)(20 Ω) = 10 V and V = (0.5A)(40 Ω) = 20 V.

You also could have used the ratio of the resistors. That is, we know the two voltages must sum to 30 V and the voltage drop across the 40 Ω must be twice the amount across the 20 Ω. The voltage across the 10 Ω resistor can be found using Ohm”s Law: V = (1.5 A)(10 Ω) = 15 V.

(c) The equivalent resistance of the circuit can be solved either by adding the resistances or by using Ohm”s Law.

If you want to add resistances, start by summing the 20 Ω and 40 Ω resistors to get 60 Ω. Then add the three parallel branches using 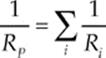or 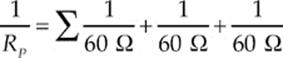, which becomes 20 Ω. Then adding this section in series to the 10 Ω resistor to get R_p = R₁+R₂ = 10 Ω + 20 Ω = 30 Ω.

You could have also realized that the total voltage drop across the battery is 45 V (15 V across the 10 Ω resistor and 30 V across the parallel branch). Using Ohm”s Law again R_eq = or R_eq = 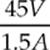= 30 Ω.

CHAPTER 13 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. D Statement I is false: The magnetic field lines due to a current-carrying wire encircle the wire in closed loops. Statement II is also false: Since the magnetic force is always perpendicular to the charged particle”s velocity vector, it cannot do work on the charged particle; therefore, it cannot change the particle”s kinetic energy. Statement III, however, is true: If the charged particle”s velocity is parallel (or antiparallel) to the magnetic field lines, then the particle will feel no magnetic force.

2. C The magnitude of the magnetic force is F_B = qvB, so the acceleration of the particle has magnitude

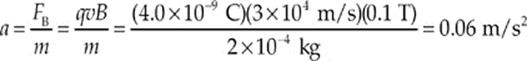
3. D By the right-hand rule, the direction of v × B is into the plane of the page. Since the particle carries a negative charge, the magnetic force it feels will be out of the page.

4. D Since F_B is always perpendicular to v, v cannot be upward or downward in the plane of the page; this eliminates choices (B) and (C). The velocity vector also cannot be to the right (choice (A)), since then v would be antiparallel to B, and F_B would be zero. Because the charge is positive, the direction of F_B will be the same as the direction of v × B. In order for v × B to be downward in the plane of the page, the right-hand rule implies that v must be out of the plane of the page.

5. A The magnetic force provides the centripetal force on the charged particle. Therefore,

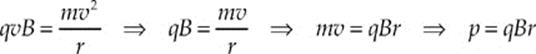
6. D The strength of the magnetic field at a distance r from a long, straight wire carrying a current I is given by the equation B = (µ₀/2π)(I/r). Therefore,

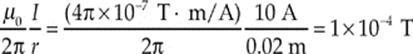
7. D By Newton”s Third Law, neither choice (A) nor choice (B) can be correct. Also, as we learned in Example 13.9, if two parallel wires carry current in the same direction, the magnetic force between them is attractive; this eliminates choices (C) and (E). Therefore, the answer must be (D). The strength of the magnetic field at a distance r from a long, straight wire carrying a current I₁ is given by the equation B₁ = (µ₀/2π)(I₁/r). The magnetic force on a wire of length carrying a current I through a magnetic field B is I( × B), so the force on Wire #2 (F_B2) due to the magnetic field of Wire #1 (B₁) is

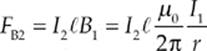
which implies

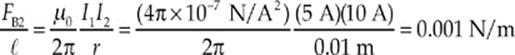
8. E The strength of the magnetic field at a distance r from a long, straight wire carrying a current I is given by the equation B = (µ₀/2π)(I/r). Therefore, the strength of the magnetic field at Point P due to either wire is B = (µ₀/2π)(I/d). By the right-hand rule, the direction of the magnetic field at P due to the top wire is into the plane of the page and the direction of the magnetic field at P due to the bottom wire is out of the plane of the page. Since the two magnetic field vectors at P have the same magnitude and opposite directions, the net magnetic field at Point P is zero.

9. C Use the right-hand rule for wires. If you point your thumb to the right and wrap your fingers along the wire, you will note that the magnetic field goes into the page when you are below the wire and comes out of the page above the wire. This allows us to eliminate choices (A) and (B). Because 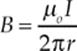, the closer we are to the wire the stronger the magnetic field. Choice (C) is closer, so it is the correct answer. We could ignore choice (E), because point E is in line with the wire, so there is no radial distance and B becomes undefined there.

10. D Magnetic fields point from north to south. Therefore, the magnetic field between the two magnets is toward the right of the page. Use the right-hand rule. Because the B field is to the right and the charges through the wire flow to the bottom of the page, the force must be out of the page.

SECTION II: FREE RESPONSE

1. (a) The acceleration of an ion of charge q is equal to F_E/m. The electric force is equal to qE, where E = V/d. Therefore, a = qV/(dm).

(b) Using a = qV/(dm) and the equation v² = v²₀ = 2ad = 2ad, we get

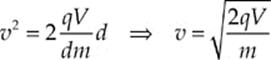
As an alternate solution, notice that the change in the electrical potential energy of the ion from the source S to the entrance to the magnetic-field region is equal to qV; this is equal to the gain in the particle”s kinetic energy.

Therefore,

(c) (i) and (ii) Use the right-hand rule. Since v points to the right and B is into the plane of the page, the direction of v × B is upward. Therefore, the magnetic force on a positively charged particle (cation) will be upward, and the magnetic force on a negatively charged particle (anion) will be downward. The magnetic force provides the centripetal force that causes the ion to travel in a circular path. Therefore, a cation would follow Path 1 and an anion would follow Path 2.

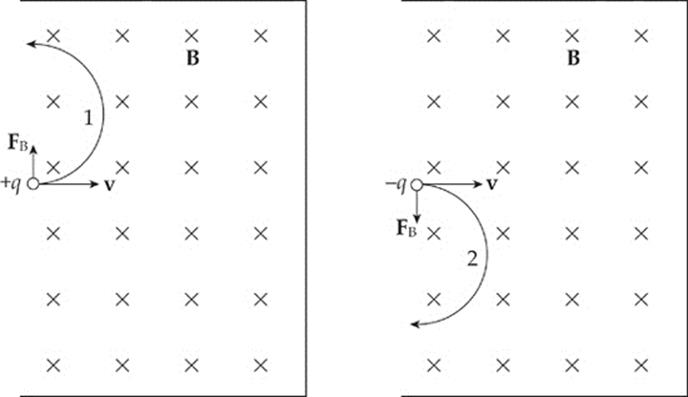
(d) Since the magnetic force on the ion provides the centripetal force,

Now, by the result of part (b),

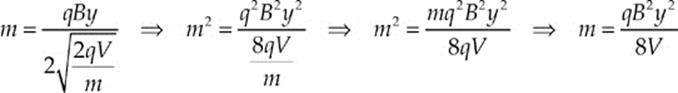
(e) Since the magnetic force cannot change the speed of a charged particle, the time required for the ion to hit the photographic plate is equal to the distance traveled (the length of the semicircle) divided by the speed computed in part (b):

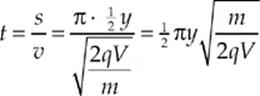
(f) Since the magnetic force F_B is always perpendicular to a charged particle”s velocity vector v, it can do no work on the particle. Thus, the answer is zero.

2. (a) Because a photon has no charge, it will experience no force and therefore travel in a straight line.

(b) From r = , we get r = 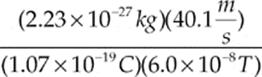= 13.9 m.

(c) From r = , we get q = . So q = = 5.58 × 10^–20C. To determine the sign of the charge, use the right-hand rule. Because the magnetic field is into the page and the charge spins in a clockwise manner, it would not obey the right-hand rule that works for all positive charges. Therefore, the charge must be negative.

CHAPTER 14 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. E Since v is upward and B is out of the page, the direction of v × B is to the right. Therefore, free electrons in the wire will be pushed to the left, leaving an excess of positive charge at the right. Therefore, the potential at Point b will be higher than at Point a, by ε = vBL (motional emf).

2. A As shown in Example 14.3, the magnitude of the emf induced between the ends of the rod is ε = BLv = (0.5 T)(0.2 m)(3 m/s) = 0.3 V. Since the resistance is 10 Ω, the current induced will be I = V/R = (0.3 V)/(10 Ω) = 0.03 A. To determine the direction of the current, we can note that since positive charges in the rod are moving to the left and the magnetic field points into the plane of the page, the right-hand rule tells us that the magnetic force, qv × B, points downward. Since the resulting force on the positive charges in the rod is downward, so is the direction of the induced current.

3. A The magnetic field through the loop is B = µ₀nI. Since its area is A = πr², the magnetic flux through the loop is Φ_B = BA = (µ₀nI)(πr²). If the current changes (with ∆I/∆t = –a), then the magnetic flux through the loop changes, which, by Faraday”s Law, implies that an emf (and a current) will be induced. We get

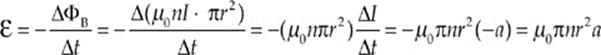
Since the magnetic flux into the page is decreasing, the direction of the induced current will be clockwise (opposing a decreasing into-the-page flux means that the induced current will create more into-the-page flux).

4. C By definition, magnetic field lines emerge from the north pole and enter at the south pole. Therefore, as the north pole is moved upward through the loop, the upward magnetic flux increases. To oppose an increasing upward flux, the direction of the induced current will be clockwise (as seen from above) to generate some downward magnetic flux. Now, as the south pole moves away from the center of the loop, there is a decreasing upward magnetic flux, so the direction of the induced current will be counterclockwise.

5. E Since the current in the straight wire is steady, there is no change in the magnetic field, no change in magnetic flux, and, therefore, no induced emf or current.

SECTION II: FREE RESPONSE

1. (a) ε = Bv ⇒ or 0.8V.

(b) V = IR becomes I = or I = 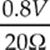= 0.04 A. The direction is given by Lenz”s Law. Current flows in order to oppose the change in the magnetic flux. Because there is suddenly a new flux “in,” current flows to produce an outward flux. This would be in the counterclockwise direction.

(c) ε = Bv ⇒ (2T)(0.2m)(1 m/s) or 0.4 V

(d) In order to get the 0.8 V you would need v = 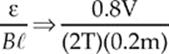or v = 2.0 m/s. You also could have noticed that if you cut the length in half, you have to compensate by doubling the speed.

CHAPTER 15 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. D From the equation λf = v, we find that

2. C The speed of a transverse traveling wave on a stretched rope is given by the equation v = . Therefore,

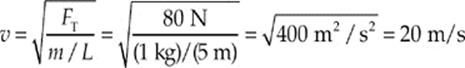
3. D The time interval from a point moving from its maximum displacement above y = 0 (equilibrium) to its maximum displacement below equilibrium is equal to one-half the period of the wave. In this case,

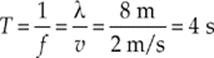
so the desired time is (4 s) = 2 s.

4. D The standard equation for a transverse traveling wave has the form y = A sin (ωt ± κx), where ω is the angular frequency and κ is the angular wave number. Here, κ = π cm^–1. Since, by definition, κ = 2π/λ, we have

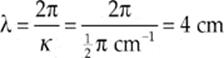
5. E The distance between successive nodes is always equal to λ. If a standing wave on a string fixed at both ends has a total of 4 nodes, the string must have a length L equal to 3(λ). If L = 6 m, then λ must equal 4 m.

6. B We found in the previous question that λ = 4 m. Since v = 40 m/s, the frequency of this standing wave must be

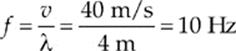
7. A In general, sound travels faster through solids than through gases. Therefore, when the wave enters the air from the metal rod, its speed will decrease. The frequency, however, will not change. Since v = λf must always be satisfied, a decrease in v implies a decrease in λ.

8. A The distance from S₂ to P is 5 m (it”s the hypotenuse of 3-4-5 triangle), and the distance from S₁ to P is 4 m. The difference between the path lengths to Point P is 1 m, which is half the wavelength. Therefore, the sound waves are always exactly out of phase when they reach Point P from the two speakers, causing destructive interference there. By contrast, since Point Q is equidistant from the two speakers, the sound waves will always arrive in phase at Q, interfering constructively. Since there”s destructive interference at P and constructive interference at Q, the amplitude at P will be less than at Q.

9. E The intensity (power per unit area) is proportional to 1/r², where r is the distance between the source and the detector. If r increases by a factor of 10, the intensity decreases by a factor of 100. Because the decibel scale is logarithmic, if the intensity decreases by a factor of 100 = 10², the decibel level decreases by 10 log (10²) = 20 dB.

10. B An air column (such as an organ pipe) with one closed end resonates at frequencies given by the equation f_n = nv/(4L) for odd integers n. The fundamental frequency corresponds, by definition, to n = 1. Therefore,

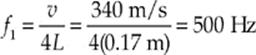
11. B The speed of the chirp is

If the distance from the bat to the tree is d, then the wave travels a total distance of d + d = 2d (round-trip distance). If T is the time for this round-trip, then

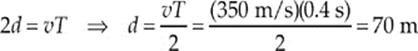
12. A Since the car is traveling away from the stationary detector, the observed frequency will be lower than the source frequency. This eliminates choices B and C. Using the Doppler effect equation, we find that

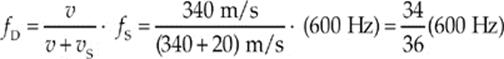
SECTION II: FREE RESPONSE

1. (a) The speed of a transverse traveling wave on a stretched rope is given by the equation v = . Therefore,

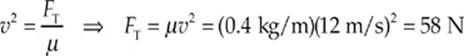
(b) Use the fundamental equation λf = v:

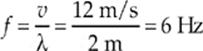
(c) (i) Because higher harmonic numbers correspond to shorter wavelengths, the harmonic number of the 3.2 m standing wave must be higher than that of the 4 m standing wave. We”re told that these harmonic numbers are consecutive integers, so if n is the harmonic number of the 4 m standing wave, then n + 1 is the harmonic number of the 3.2 m wave. Therefore,

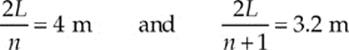
The first equation says that 2L = 4n, and the second one says that 2L = 3.2(n + 1). Therefore, 4n must equal 3.2(n + 1); solving this equation gives n = 4. Substituting this into either one of the displayed equations then gives L = 8 m.

(c) (ii) Because µ = 0.4 kg/m, the mass of the rope must be

(d) We determined this in the solution to part (c) (i). The 4 m standing wave has harmonic number n = 4.

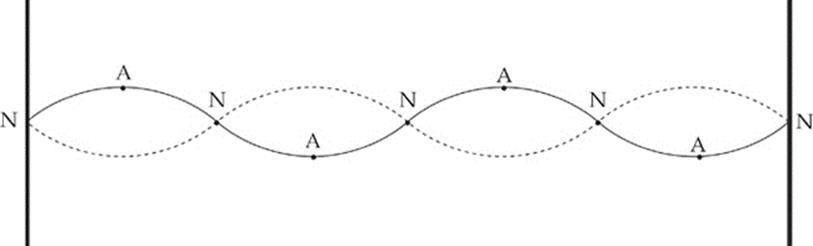
(e)

2. (a) For a stationary observer and a source moving away, the Doppler effect equation predicts that

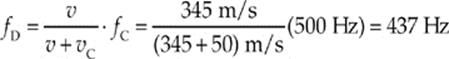
(b) Yes. If the source is stationary and the observer moves away, the Doppler effect equation now gives

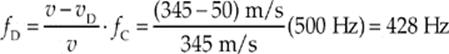
(c) With θ = 60°, the given equation becomes

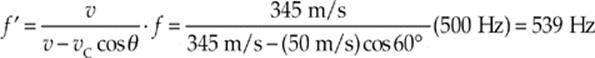
Note that the observed frequency is higher than the source frequency, which we expect since the car is traveling toward the students.

(d) With θ = 120°, the given equation becomes

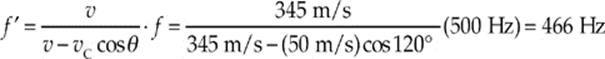
Note that the observed frequency is now lower than the source frequency, which we expect since the car is traveling away from the students.

(e) When the car is far to the left of the students” position, θ is very small (approaches 0°). Therefore, for large negative x, the observed frequency should approach

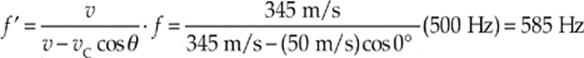
When the car is far to the right of the students” position, θ approaches 180°. Therefore, for large positive x, the observed frequency should approach

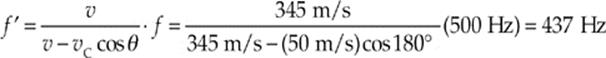
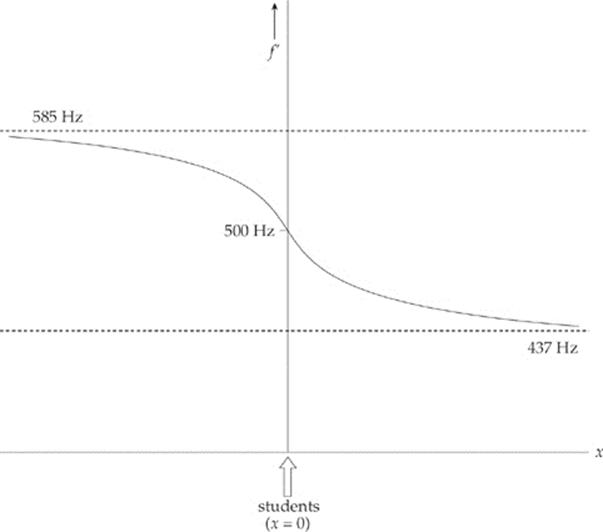
When the car is directly in front of the students—that is, when x = 0 (and θ = 90°)—the equation given predicts that f′ will equal 500 Hz. The graph of f′ versus x should therefore have the following general shape:

CHAPTER 16 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. B From the equation λf = c, we find that

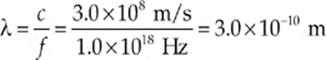
2. D Since the fringe is bright, the waves must interfere constructively. This implies that the difference in path lengths must be a whole number times the wavelength, eliminating choices (B), (C), and (E). The central maximum is equidistant from the two slits, so ∆ = 0 there. At the first bright fringe above the central maximum, we have ∆ = λ.

3. C First, eliminate choices (A) and (B): The index of refraction is never smaller than 1. Refer to the following diagram:

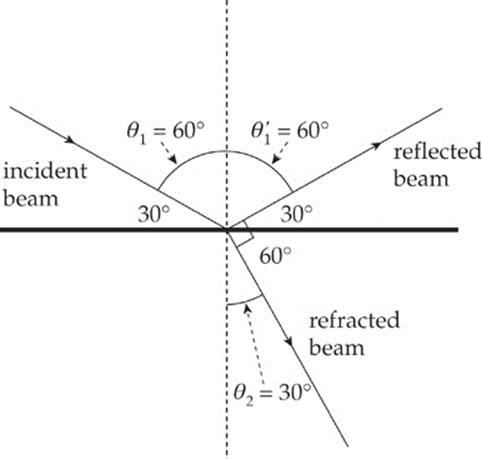
Since the reflected and refracted beams are perpendicular to each other, we have θ₂ = 30°. Snell”s Law then becomes

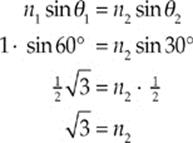
4. A The frequency is unchanged, but because the speed of light in diamond is less than in air, the wavelength of the light in diamond is shorter than its wavelength in air:

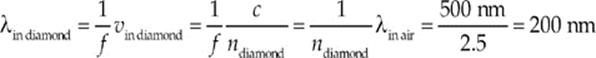
5. A If the speed of light is less in Medium 2 than in Medium 1, then Medium 2 must have the higher index of refraction; that is, n₂ > n₁. Snell”s Law then implies that θ₂ < θ₁: The beam will refract toward the normal upon transmission into Medium 2.

6. A The critical angle for total internal reflection is computed as follows:

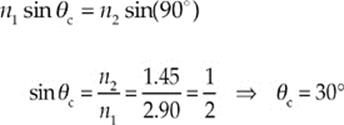
Total internal reflection can happen only if the incident beam originates in the medium with the higher index of refraction and strikes the interface of the other medium at an angle of incidence greater than the critical angle.

7. D If s_o = 60 cm and f = 40 cm, the mirror equation tells us that

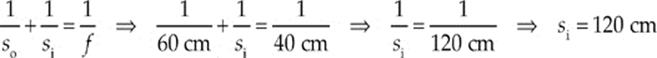
And, since s_i is positive, the image is real.

8. D Because the image is virtual, we must write the image distance, s_i, as a negative quantity: s_i = –20 cm. Now, with s_o = 60 cm, the mirror equation gives

The focal length is half the radius of curvature, so

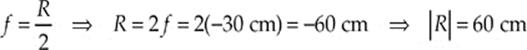
9. C Since the image is projected onto a screen, it must be real, and therefore inverted. The magnification must be negative, so

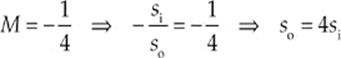
Because s_i = 60 cm, the object distance, s_o, must be 240 cm. Therefore,

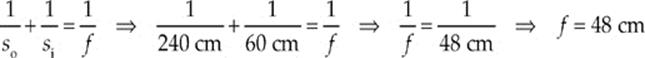
10. B A bi-concave lens is a diverging lens. Diverging lenses (like convex mirrors) have negative focal lengths and therefore cannot form real images. (Note that statement (D) is false; diverging lenses and convex mirrors always form diminished, virtual images, as you can verify using the mirror and magnification equations.)

SECTION II: FREE RESPONSE

1. (a) Interference effects can be observed only if the light is coherent. Using two independent light sources at the slits in Barrier 2 would generate incoherent light waves. The set-up shown guarantees that the light reaching the two slits will be coherent.

(b) Maxima are located at positions given by the equation x_m = mλL/d, where m is an integer (y₀ = 0 is the central maximum). The first-order maximum for red light occurs at

and the first-order maximum for violet light occurs at

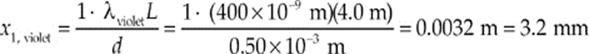
Therefore, the vertical separation of these maxima on the screen is ∆x = 6.0 mm – 3.2 mm = 2.8 mm.

(c) Maxima are located at positions given by the equation x_m = mλL/d, where m is an integer. We therefore want to solve the equation

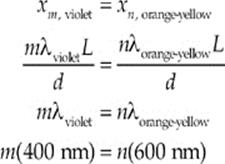
The smallest integers that satisfy this equation are m = 3 and n = 2. That is, the third-order maximum for violet light coincides with the second-order maximum for orange-yellow light. The position on the screen (relative to the central maximum at x = 0) of these maxima is

(d) Within the glass, the wavelength is reduced by a factor of n = 1.5 from the wavelength in air. Therefore, the difference in path lengths, d sin θ, must be equal to m(λ/n) in order for constructive interference to occur. This implies that the maxima are located at positions given by x_m = mλL/(nd). The distance between adjacent bright fringes is therefore

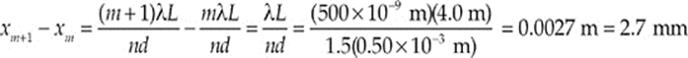
2. (a) Beam 1 undergoes a 180° phase change when it reflects off the soap film. The refracted portion of the incident beam does not undergo a phase change upon reflection at the film/air boundary, because it strikes the boundary to a medium whose index is lower; therefore, Beam 2 does not suffer a 180° phase change.

(b) We”re told that the criterion for destructive interference in this case is ∆ = 2T = m(λ/n), where n is the refractive index of the soap film and m is a whole number. Therefore, the criterion for constructive interference must be

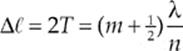
Alternatively, Δ = 2T = 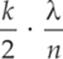where k is an odd whole number.

3. (a) Since the image is formed behind the mirror, it is virtual.

(b) Virtual images are upright.

(c) Writing s_i = –72 cm (negative because the image is virtual), the magnification is

so the height of the image is h_i = |m|·h_o = 1.8(5 cm) = 9 cm.

(d) The mirror equation can be used to solve for the mirror”s focal length:

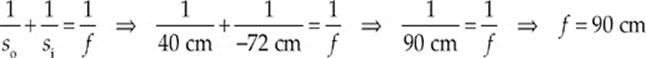
Therefore, the mirror”s radius of curvature is R = 2f = 2(90 cm) = 180 cm.

(e)

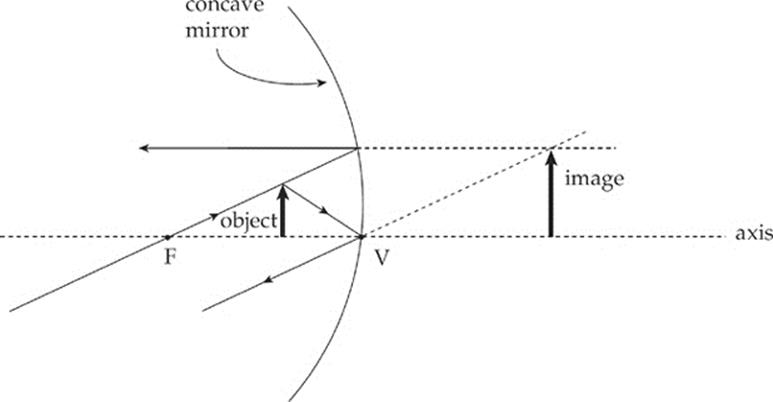
CHAPTER 17 REVIEW QUESTIONS

SECTION I: MULTIPLE CHOICE

1. B Combining the equation E = hf with f = c/λ gives us

2. D The energy of the incident photons is

Since E > φ, photoelectrons will be produced, with maximum kinetic energy

3. A If the atom”s ionization energy is 25 eV, then the electron”s ground-state energy must be –25 eV. Making a transition from the –16 eV energy level to the ground state will cause the emission of a photon of energy

∆E = (–16 eV) – (–25eV) = 9 eV

4. E The gap between the ground-state and the first excited state is

–10 eV – (–40 eV) = 30 eV

Therefore, the electron must absorb the energy of a 30 eV photon (at least) in order to move even to the first excited state. Since the incident photons have only 15 eV of energy, the electron will be unaffected.

5. C The de Broglie wavelength of a particle whose momentum is p is λ = h/p. For this proton, we find that

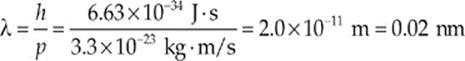
6. E If the atom begins in the n = 3 state, it could lose energy by making any of the following transitions: 3 → 2, 3 → 1, or 3 → 2 → 1. The 3 → 2 transition would result in the emission of –5 eV – (–8 eV) = 3 eV; the 3 → 1 transition would emit a –5 eV – (–12 eV) = 7 eV photon; and the 2 → 1 transition would result in the emission of –8 eV – (–12 eV) = 4 eV. Therefore, if the atom is initially in the n = 3 state, it could emit photons of energy 3 eV, 4 eV, or 7 eV.

7. D The energy of the nth level is given by the equation E_n = E₁/n², where E₁ is the ground-state energy. Therefore, the energy of level n = 2 (the next level above the ground state) is E₂ = (–54.4 eV)/4 = –13.6 eV. Therefore, the difference in energy between the n = 1 and n = 2 levels is ∆E = –13.6 eV – (–54.4 eV) = 40.8 eV.

8. C The energy of a photon is given by the equation E = hf, or equivalently by E = hc/λ. Therefore, E is inversely proportional to λ. If λ decreases by a factor of 2, then E will increase by a factor of 2.

9. A The equation that relates the mass difference m and the disintegration energy Q is Einstein”s mass–energy equivalence formula, Q = mc². Because c² is a constant, we see that Q is proportional to m. Therefore, if m decreases by a factor of 4, then so will Q.

10. B In order to balance the mass number (the superscripts), we must have 2 + 63 = 64 + A, so A = 1. In order to balance the charge (the subscripts), we need 1 + 29 = 30 + Z, so Z = 0. A particle with a mass number of 1 and no charge is a neutron, .

11. E In order to balance the mass number (the superscripts), we must have 196 + 1 = 197 + A, so A = 0. In order to balance the charge (the subscripts), we need 78 + 0 = 78 + Z, so Z = 0. The only particle listed that has zero mass number and zero charge is a gamma-ray photon, .

SECTION II: FREE RESPONSE

1. (a) If ionizing lithium twice leaves the atom with just one electron, then it must have originally had three electrons. Since neutral atoms have the same number of protons as electrons, Z must be 3 for lithium.

(b) First, use the formula given for E_n to determine the values of the first few electron energy levels:

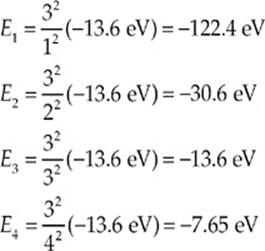
Differences between electron energy levels equal the energies of the emitted photons. Since E = hf = hc/λ, we have λ = hc/E. Since the E”s are in electronvolts and the wavelengths in nanometers, it is particularly helpful to note that

The goal here is to match the energy-level differences with the given wavelengths, using the equation λ = (1240 eV·nm)/E.

Using this diagram, the four wavelengths given correspond to the following photon energies and energy-level transitions:

(c) No. In order for a spectral line to have a wavelength between 54.0 nm and 72.9 nm, there must be an energy-level transition with an energy between 17 eV and 23 eV. As the diagram above shows, no such intermediate transition is possible.

(d) The 11.4 nm line corresponds to a photon energy of 109 eV. Therefore, the next shortest wavelength must correspond to the next highest possible photon energy. According to the diagram, the next highest available transition energy after 109 eV is 115 eV. This wavelength of the photon emitted in this (4 → 1) transition is