SAT Physics Subject Test
Chapter 6 Curved and Rotational Motion
Chapter 6 Review Questions
See Chapter 17 for solutions.
1. An object of mass 0.5 kg, moving in a circular path of radius 0.25 m, experiences a centripetal acceleration of constant magnitude 9 m/s2. What is the object”s angular speed?
(A) 2.3 rad/s
(B) 4.5 rad/s
(C) 6 rad/s
(D) 12 rad/s
(E) Cannot be determined from the information given
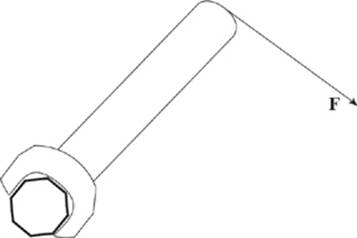
2. In an effort to tighten a bolt, a force F is applied as shown in the figure above. If the distance from the end of the wrench to the center of the bolt is 20 cm and F = 20 N, what is the magnitude of the torque produced by F ?
(A) 0 N × m
(B) 1 N × m
(C) 2 N × m
(D) 4 N × m
(E) 10 N × m
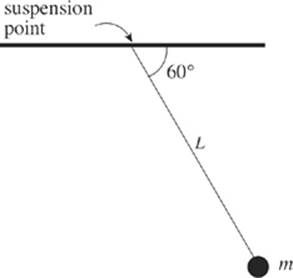
3. In the figure above, what is the torque about the pendulum”s suspension point produced by the weight of the bob, given that the mass is 40 cm below the suspension point, measured vertically, and m = 0.50 kg ?
(A) 0.49 N × m
(B) 0.98 N × m
(C) 1.7 N × m
(D) 2.0 N × m
(E) 3.4 N × m

4. A uniform meter stick of mass 1 kg is hanging from a thread attached at the stick”s midpoint. One block of mass m = 3 kg hangs from the left end of the stick, and another block, of unknown mass m, hangs below the 80 cm mark on the meter stick. If the stick remains at rest in the horizontal position shown above, what is m ?
(A) 4 kg
(B) 5 kg
(C) 6 kg
(D) 8 kg
(E) 9 kg
5. An object moves at constant speed in a circular path. True statements about the motion include which of the following?
I. The velocity is constant.
II. The acceleration is constant.
III. The net force on the object is zero since its speed is constant.
(A) II only
(B) I and III only
(C) II and III only
(D) I and II only
(E) None of the above
6. Three thin, uniform rods each of length L are arranged in the shape of an inverted U.

The two rods on the arms of the U each have mass m; the third rod has mass 2m. How far below the midpoint of the horizontal rod is the center of mass of this assembly?
(A) ![]()
(B) ![]()
(C) ![]()
(D) ![]()
(E) ![]()
7. A satellite is currently orbiting Earth in a circular orbit of radius R; its kinetic energy is K1. If the satellite is moved and enters a new circular orbit of radius 2R, what will be its kinetic energya?
(A) ![]()
(B) ![]()
(C) K1
(D) 2K1
(E) 4K1
8. A moon of Jupiter has a nearly circular orbit of radius R and an orbit period of T. Which of the following expressions gives the mass of Jupiter?
(A) ![]()
(B) 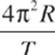
(C) 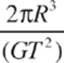
(D) 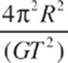
(E) 
9. The mean distance from Saturn to the sun is 9 times greater than the mean distance from Earth to the sun. How long is a Saturn year?
(A) 18 Earth years
(B) 27 Earth years
(C) 81 Earth years
(D) 243 Earth years
(E) 729 Earth years
10. Two satellites orbit the earth in circular orbits, each traveling at a constant speed. The radius of satellite A”s orbit is R, and the radius of satellite B”s orbit is 3R. Both satellites have the same mass. How does FA, the centripetal force on satellite A, compare with FB, the centripetal force on satellite B ?
(A) FA = 9FB
(B) FA = 3FB
(C) FA = FB
(D) FB = 3FA
(E) FB = 9FA
Keywords
uniform circular motion
centripetal acceleration
centripetal force
center of mass
translation
rotation
rotational inertia (moment of inertia)
torque
line of action
lever arm (moment arm)
moment
net torque
translational equilibrium
rotational equilibrium
static equilibrium
angular momentum
conservation of angular momentum
angular displacement
rigid body
average angular velocity (or speed)
average angular acceleration
radian
focus/foci
Summary
· Uniform circular motion considers an object”s circular path at a constant speed. The velocity is not constant because the direction is always changing. Because velocity changes, acceleration changes as well.
· In uniform circular motion, velocity is directed tangent to the circle and acceleration is directed toward the center.
· Center of mass is the point where all of the mass of an object can be considered to reside. For a homogeneous body, the center of mass is at the geometric center of the object. For a group of objects, establish an x/y coordinate system, multiply the position value of each object by its mass and get the sum for all the particles. Divide this sum by the total mass. The resulting value is the center of mass in terms of x- and y-coordinates. (Treat the x-value and y-value separately.)
· In an isolated system the center of mass will not accelerate.
· Rotational dynamics involves describing the acceleration of an object in terms of its mass (inertia) and the forces that act on it: Fnet = ma.
· Torque is the quantity that measures how effectively a force causes rotation. The greater the distance from the axis of rotation (the pivot) where force is applied, the greater the torque will be.
· Equilibrium refers to the state of an object when the sum of the forces and torque acting on it is zero.
· Angular momentum is the rotational analog for linear momentum. It is the product of mass and velocity and the distance from the axis of rotation. It is symbolized by L. Use the formula L = rmv⊥.
· Conservation of angular momentum states that if the torques on a body balance so that the net torque is zero, then the body”s angular momentum cannot change.
· Rotational kinematics has symbols and concepts that are analogous to those of linear kinematics.
· When dealing with rotational kinematics, remember to use the Big Five to find the value of the missing variable.
· Kepler”s first law: Every planet moves in an elliptical orbit with the sun at one focus of both of them in an ellipse.
· Kepler”s second law: A planet moves faster when it is closer to the sun than when it is further away.
· Kepler”s third law: The ratio T2/a3 is the same for all the planets, where T is the time it takes the planet to make one orbit (the period) and a is the length of the semimajor axis of a planet”s orbit.