Pre-Calculus For Dummies, 2nd Edition (2012)
Part II. The Essentials of Trigonometry
Chapter 9. Advanced Identities: Your Keys to Pre-Calc Success
In This Chapter
![]() Applying the sum and difference formulas of trig functions
Applying the sum and difference formulas of trig functions
![]() Utilizing double-angle formulas
Utilizing double-angle formulas
![]() Cutting angles in two with half-angle formulas
Cutting angles in two with half-angle formulas
![]() Changing from products to sums and back
Changing from products to sums and back
![]() Tossing aside exponents with power-reducing formulas
Tossing aside exponents with power-reducing formulas
Prior to the invention of calculators (not as long ago as you may imagine), people had only one way to calculate the exact trig values for angles not shown on the unit circle: using advanced identities. Even now, most calculators give you only an approximation of the trig value, not the exact one. Exact values are important to trig calculations and to their applications (and to teachers, of course). Engineers designing a bridge, for example, don’t want an almost correct value — and neither should you, for that matter.
This chapter is the meat and potatoes of pre-calc identities: It contains the bulk of the formulas that you need to know for calculus. It builds on the basic identities we discuss in Chapter 8. Advanced identities provide you with opportunities to calculate values that you couldn’t calculate before — like finding the exact value of the sine of 15°, or figuring out the sine or cosine of the sum of angles without actually knowing the value of the angles. This information is truly helpful when you get to calculus, which takes these calculations to another level (a level at which you integrate and differentiate by using these identities).
Finding Trig Functions of Sums and Differences
Long ago, some fantastic mathematicians found identities that hold true when adding and subtracting angle measures from special triangles (30°-60°-90° right triangles and 45°-45°-90° right triangles; see Chapter 6). The focus is to find a way to rewrite an angle as a sum or difference. Those mathematicians were curious; they could find the trig values for the special triangles but wanted to know how to deal with other angles that aren’t part of the special triangles on the unit circle. They could solve problems with multiples of 30° and 45°, but they knew nothing about the so many other angles that could be formed!
Constructing these angles was simple; however, evaluating trig functions for them proved to be a bit more difficult. So they put their collective minds together and discovered the identities we discuss in this section. Their only problem was that they still couldn’t find plenty of other trig values by using the sum (a + b) and difference (a – b) formulas.
This section takes the information that we cover in earlier chapters, such as calculating trig values of special angles, to the next level. We introduce you to advanced identities that allow you to find trig values of angles that are multiples of 15°.
Note: You’ll never be asked to find the sine of 87°, for example, without a calculator in this section, because it can’t be written as the sum or difference of special angles. The 30°-60°-90° and the 45°-45°-90° triangles can always be boiled down to the same special ratios of their sides, but other triangles cannot. So if you can break down the given angle into the sum or difference of two known angles, you have it made in the shade because you can use the sum or difference formula to find the trig value you’re looking for. (If you can’t express the angle as the sum or difference of special angles, you have to find some other way to solve the problem.)
 For the most part, when you’re presented with advanced identity problems in pre-calculus, you’ll be asked to work with angles in radians. Of course, sometimes you’ll have to work with degrees as well. We start with calculations in degrees because they’re easier to manipulate. We then switch to radians and show you how to make the formulas work with them, too.
For the most part, when you’re presented with advanced identity problems in pre-calculus, you’ll be asked to work with angles in radians. Of course, sometimes you’ll have to work with degrees as well. We start with calculations in degrees because they’re easier to manipulate. We then switch to radians and show you how to make the formulas work with them, too.
Searching out the sine of (a ± b)
Using the special right triangles (see Chapter 6), which have points on the unit circle that are easy to identify, you can find the sine of 30° and 45° angles (among others). However, no point on the unit circle allows you to find trig values at angle measures that aren’t special (such as the sine of 15°) directly. The sine does exist for such an angle (meaning it’s a real number value) at a point on the circle; it just isn’t one of the nicely labeled points. Don’t despair, because this is where advanced identities help you out.
 If you look really closely, you’ll notice that 45° – 30° = 15°, and 45° + 30° = 75°. For the angles you can rewrite as the sum or difference of special angles, here are the sum and difference formulas for sine:
If you look really closely, you’ll notice that 45° – 30° = 15°, and 45° + 30° = 75°. For the angles you can rewrite as the sum or difference of special angles, here are the sum and difference formulas for sine:
![]() sin(a + b) = sin a · cos b + cos a · sin b
sin(a + b) = sin a · cos b + cos a · sin b
![]() sin(a – b) = sin a · cos b – cos a · sin b
sin(a – b) = sin a · cos b – cos a · sin b
 You can’t rewrite the sin(a + b) as sin a + sin b. You can’t distribute the sine into the values inside the parentheses, because sine isn’t a multiplication operation; therefore, the distributive property doesn’t apply (like it does to real numbers). Sine is a function, not a number or variable.
You can’t rewrite the sin(a + b) as sin a + sin b. You can’t distribute the sine into the values inside the parentheses, because sine isn’t a multiplication operation; therefore, the distributive property doesn’t apply (like it does to real numbers). Sine is a function, not a number or variable.
 You have more than one way to combine unit circle angles to get a requested angle. You can write sin 75° as sin(135° – 60°) or sin(225° – 150°). After you find a way to rewrite an angle as a sum or difference, roll with it. Use the one that works for you!
You have more than one way to combine unit circle angles to get a requested angle. You can write sin 75° as sin(135° – 60°) or sin(225° – 150°). After you find a way to rewrite an angle as a sum or difference, roll with it. Use the one that works for you!
Calculating in degrees
Measuring angles in degrees for the sum and difference formulas is easier than measuring in radians, because adding and subtracting degrees is much easier than adding and subtracting radians. Adding and subtracting angles in radians requires finding a common denominator. Moreover, evaluating trig functions requires you to work backward from a common denominator to split the angle into two fractions with different denominators. (If the angle in the problem given to you is in radians, we show you the way in the next section.)
For example, follow these steps to find the sine of 135°:
1. Rewrite the angle, using the special angles from right triangles (see Chapter 6).
One way to rewrite 135° is 90° + 45°.
2. Choose the appropriate sum or difference formula.
The example from Step 1 uses addition, so you want to use the sum formula, not the difference formula:
sin(a + b) = sin a · cos b + cos a · sin b
3. Plug the information you know into the formula.
You know that sin 135° = sin(90° + 45°). Therefore, a = 90° and b = 45°. The formula gives you sin 90° cos 45° + cos 90° sin 45°.
4. Use the unit circle (see Chapter 6) to look up the sine and cosine values you need.
You now have ![]() .
.
5. Multiply and simplify to find the final answer.
You end up with ![]() .
.
Calculating in radians
You can put the concept of sum and difference formulas to work using radians. This process is different than solving equations because here you’re asked to find the trig value of a specific angle that isn’t readily marked on the unit circle (but still is a multiple of 15° or π/12 radians). Prior to choosing the appropriate formula (Step 2 from the previous section), you simply break the angle into either the sum or the difference of two angles from the unit circle. Refer to the unit circle and notice the angles in radians in Figure 9-1. You see that all the denominators are different, which makes adding and subtracting them a nightmare. You must find a common denominator so that adding and subtracting is a dream. The common denominator is 12, as you can see in Figure 9-1.
Figure 9-1: The unit circle showing angles in radians with common denominators.
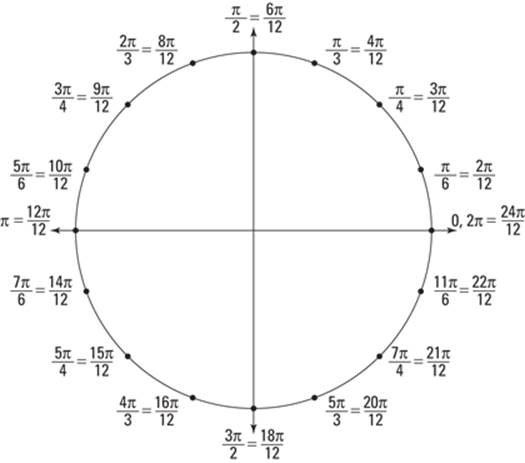
 Figure 9-1 comes in handy only for sum and difference formulas, because finding a common denominator is something you do only when you’re adding or subtracting fractions.
Figure 9-1 comes in handy only for sum and difference formulas, because finding a common denominator is something you do only when you’re adding or subtracting fractions.
For example, follow these steps to find the exact value of sin(π/12):
1. Rewrite the angle in question, using the special angles in radians with common denominators.
From Figure 9-1, you want a way to add or subtract two angles so that, in
the end, you get π/12. In this case, you can rewrite π/12 as ![]() .
.
2. Choose the appropriate sum/difference formula.
Because we rewrote the angle with subtraction, you need to use the difference formula.
3. Plug the information you know into the chosen formula.
You know the following equality:
![]()
Substitute as follows into the difference formula:
![]() and
and ![]()
Which gives you
![]()
4. Reduce the fractions in the formula to ones you’re more comfortable with.
In our example, you can reduce to
![]()
Now you’ll have an easier time referring to the unit circle to get your equation.
5. Use the unit circle to look up the sine and cosine values that you need.
You now have
![]()
6. Multiply and simplify to get your final answer.
You end up with the following answer:
![]()
Applying the sine sum and difference formulas to proofs
The goal when dealing with trig proofs in this chapter is the same as the goal when dealing with them in Chapter 8: You need to make one side of a given equation look like the other. You can work on both sides to get a little further if need be, but make sure you know how your teacher wants the proof to look. This section contains info on how to deal with sum and difference formulas in a proof.
When asked to prove sin(x + y) + sin(x – y) = 2 sin x · cos y, for example, follow these steps:
1. Look for identities in the equation.
In our example, you can see the sum identity, sin(a + b) = sin a · cos b + cos a · sin b, and the difference identity, sin(a – b) = sin a · cos b – cos a · sin b for sin.
2. Substitute for the identities.
sin x · cos y + cos x · sin y + sin x · cos y – cos x · sin y = 2 sin x · cos y
3. Simplify to get the proof.
Two terms cancel, leaving you with this equation:
sin x · cos y + sin x · cos y = 2 sin x · cos y
Combine the like terms to get the answer:
2 sin x · cos y = 2 sin x · cos y
Calculating the cosine of (a ± b)
After you familiarize yourself with the sum and difference formulas for sine, you can easily apply your newfound knowledge to calculate the sums and differences of cosines, because the formulas look very similar to each other. When working with sums and differences for sines and cosines, you’re simply plugging in given values for variables. Just make sure you use the correct formula based on the information you’re given in the question.
 Here are the formulas for the sum and difference of cosines:
Here are the formulas for the sum and difference of cosines:
![]() cos(a + b) = cos a · cos b – sin a · sin b
cos(a + b) = cos a · cos b – sin a · sin b
![]() cos(a – b) = cos a · cos b + sin a · sin b
cos(a – b) = cos a · cos b + sin a · sin b
Applying the formulas to find the sum or difference of two angles
The sum and difference formulas for cosine (and sine) can do more than calculate a trig value for an angle not marked on the unit circle (at least for angles that are multiples of 15°). They can also be used to find the sum or difference of two angles based on information given about the two angles. For such problems, you’ll be given two angles (we’ll call them A and B), the sine or cosine of A and B, and the quadrant(s) in which the two angles are located.
Use the following steps to find the exact value of cos(A + B), given that cos A = –3/5, with A in quadrant II of the coordinate plane, and sin B = –7/25, with B in quadrant III:
1. Choose the appropriate formula and substitute the information you know to determine the missing information.
If cos(A + B) = cos A · cos B – sin A · sin B, then substitutions result in this equations:
cos(A + B) = (–3/5) · cos B – sin A · (–7/25)
To proceed any further, you need to find cos B and sin A.
2. Draw pictures representing right triangles in the quadrant(s).
You need to draw one triangle for angle A in quadrant II and one for angle B in quadrant III. Using the definition of sine as opp/hyp and cosine as adj/hyp, Figure 9-2 shows these triangles. Notice that the value of a leg is missing in each triangle.
3. To find the missing values, use the Pythagorean theorem (once for each triangle; see Chapter 6).
The missing leg in Figure 9-2a is 4, and the missing leg in Figure 9-2b is –24.
Figure 9-2:Drawing pictures helps you visualize the missing pieces of info.
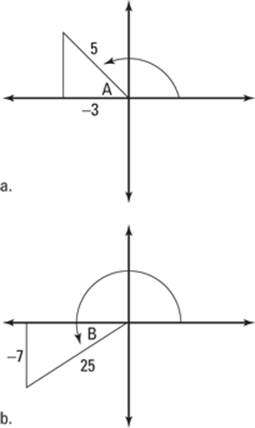
4. Determine the missing trig ratios to use in the sum/difference formula.
You use the definition of cosine to find that cos B = –24/25 and the definition of sine to find that sin A = 4/5.
5. Substitute the missing trig ratios into the sum/difference formula and simplify.
You now have this equation:
cos(A + B) = (–3/5) · (–24/25) – (4/5) · (–7/25)
Follow the order of operations to get this answer:
cos(A + B) = (72/125) – (–28/125) = (72 + 28)/125 = 100/125
This equation simplifies to cos(A + B) = 4/5.
Applying the cosine sum and difference formulas to proofs
You can prove the co-function identities from Chapter 8 by using the sum and
difference formulas for cosine. For example, to prove ![]() , follow these steps:
, follow these steps:
1. Outline the given information.
You start with ![]() .
.
2. Look for sum and/or difference identities for cosine.
In this case, the left side of the equation is the difference formula for cosine. Therefore, you can break up the first term by using the difference formula for cosines:
![]()
3. Refer to the unit circle and substitute all the information you know.
Using the unit circle, the previous equation simplifies to 0 · cos x + 1 · sin x, which equals sin x on the left-hand side. Your equation now says sin x = sin x. Ta-dah!
Taming the tangent of (a ± b)
As with sine and cosine (see the previous sections of this chapter), you can rely on formulas to find the tangent of a sum or a difference of angles. The main difference is that you can’t read tangents directly from the coordinates of points on the unit circle, as you can with sine and cosine, because each point represents (cos θ, sin θ); we explain more about this topic in Chapter 6.
All hope isn’t lost, however, because tangent is defined as sin/cos. Because the sine of the angle is the y-coordinate and the cosine is the x-coordinate, you can express the tangent in terms of x and y on the unit circle as y/x.
 Here are the formulas you need to find the tangent of a sum or difference of angles:
Here are the formulas you need to find the tangent of a sum or difference of angles:
![]()
![]()
![]()
![]()
We suggest that you memorize these sweet little formulas, because then you won’t have to use the sum and difference formulas for sine and cosine in the middle of a tangent problem, saving you time in the long run. If you choose not to memorize these two formulas, you can derive them by remembering these equations:
![]()
![]()
![]()
![]()
Applying the formulas to solve a common problem
The sum and difference formulas for tangent work in similar ways to the sine and cosine formulas. You can use the formulas to solve a variety of problems. In this section, we show you how to find the tangent of an angle that isn’t marked on the unit circle. You can do so as long as the angle can be written as the sum or difference of special angles.
For example, to find the exact value of tan 105°, follow these steps (Note: We don’t mention the quadrant, because the angle 105° is in quadrant II. In the previous example, the angle wasn’t given; a trig value was given, and each trig value has two angles on the unit circle that yield the value, so you need to know which quadrant the problem is talking about):
1. Rewrite the given angle, using the information from special right-triangle angles (see Chapter 6).
Refer to the unit circle in Chapter 6, noting that it’s built from the special right triangles, to find a combination of angles that add or subtract to get 105°. You can choose from 240° – 135°, 330° – 225°, and so on. In this example, we choose 60° + 45°. So tan(105°) = tan(60° + 45°).
Because we rewrote the angle with addition, you need to use the sum formula for tangent.
2. Plug the information you know into the appropriate formula.
![]()
3. Use the unit circle to look up the sine and cosine values that you need.
To find tan 60°, you must locate 60° on the unit circle and use the sine and cosine values of its corresponding point to calculate the tangent:
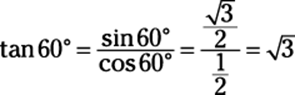
Follow the same process for tan 45°:
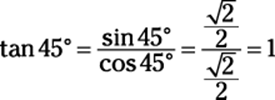
4. Substitute the trig values from Step 4 into the formula.
This step gives you
![]()
which simplifies to
![]()
5. Rationalize the denominator.
You can’t leave the square root on the bottom of the fraction. Because the denominator is a binomial (the sum or difference of two terms), you must multiply by its conjugate. The conjugate of a + b is a – b, and vice versa. So the conjugate of ![]() is
is ![]() :
:
![]()
FOIL (see Chapter 4) both binomials to get
![]()
6. Simplify the rationalized fraction to find the exact value of tangent.
Combine like terms to get
![]()
Make sure you fully simplify this fraction to get ![]() .
.
Applying the sum and difference formulas to proofs
The sum and difference formulas for tangent are very useful if you want to prove a few of the basic identities from Chapter 8. For example, you can prove the co-function identities by using the difference formula and the periodicity identities by using the sum formula. If you see a sum or a difference inside a tangent function, you can try the appropriate formula to simplify things.
For instance, you can prove this identity with the following steps:
![]()
1. Look for identities for which you can substitute.
On the left side of the proof is the sum identity for tangent:
![]()
Working on the left side only gives you the following equation:
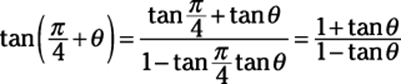
2. Use any applicable unit circle values to simplify the proof.
From the unit circle (see Chapter 6), you see that tan π/4 = 1, so you can plug in that value to get this:
![]()
From there, simple multiplication gives you this result:
![]()
Doubling an Angle’s Trig Value without Knowing the Angle
You use a double-angle formula to find the trig value of twice an angle. Sometimes you know the original angle; sometimes you don’t. Working with double-angle formulas comes in handy when you need to solve trig equations or when you’re given the sine, cosine, tangent, or other trig function of an angle and need to find the exact trig value of twice that angle without knowing the measure of the original angle. Isn’t this your happy day?
Note: If you know the original angle in question, finding the sine, cosine, or tangent of twice that angle is easy; you can look it up on the unit circle or use your calculator to find the answer. However, if you don’t have the measure of the original angle and you must find the exact value of twice that angle, the process isn’t that simple. Read on!
Finding the sine of a doubled angle
To fully understand and be able to stow away the double-angle formula for sine, you should first understand where it comes from. (The double-angle formulas for sine, cosine, and tangent are extremely different from one another, although they can all be derived by using the sum formula.)
1. To find sin 2x, you must realize that it’s the same as sin(x + x).
2. Use the sum formula for sine (see the section “Searching out the sine of (a ± b)”) to get sin x · cos x + cos x · sin x.
 3. Simplify to get sin 2x = 2 sin x · cos x.
3. Simplify to get sin 2x = 2 sin x · cos x.
This process is called the double-angle formula for sine. If you’re given an equation with more than one trig function and asked to solve for the angle, your best bet is to express the equation in terms of one trig function only. You often can achieve this by using the double-angle formula.
To solve 4 sin 2x · cos 2x = 1, notice that it doesn’t equal 0, so you can’t factor it. Even if you subtract 1 from both sides to get 0, it can’t be factored. So there’s no solution, right? Not quite. You have to check the identities first. The double-angle formula, for instance, says that 2 sin x · cos x = sin 2x. You can rewrite some things here:
1. List the given information.
You have 4 sin 2x · cos 2x = 1.
2. Rewrite the equation to find a possible identity.
We go with 2 · (2 sin 2x · cos 2x) = 1.
3. Apply the correct formula.
The double-angle formula for sine gives you 2 · [sin(2 · 2x)] = 1.
4. Simplify the equation and isolate the trig function.
Break it down to 2 · sin 4x = 1, which becomes sin 4x = 1/2.
5. Find all the solutions for the trig equation.
This step gives you ![]() and
and ![]() where k is an integer.
where k is an integer.
This equation tells you that each reference angle has four solutions, and you use the notation + 2πk to represent the circle. Then you can divide everything (including the 2πk) by 4, which gives you the solutions:
• ![]()
• ![]()
These solutions are the general ones, but sometime you may have to use this information to get to a solution on an interval.
Finding the solutions on an interval is a curveball thrown at you in pre-calc. For the previous problem, you can find a total of eight angles on the interval [0, 2π). Because a coefficient was in front of the variable, you’re left with, in this case, four times as many solutions, and you must state them all. You have to find the common denominator to add the fractions. In this case, π/2 becomes (12π)/24:
The first solution: ![]()
The second one: ![]()
The third one: ![]()
The fourth one: ![]()
Doing this one more time gets you (49π)/24, which is really where you started (because you moved (48π)/24 from the original, which really is 2π — the period of the sine function). Meanwhile,
(5π)/24 is another solution.
![]() gives you
gives you ![]() .
.
![]() is
is ![]() .
.
![]() is
is ![]() .
.
![]() is
is ![]() .
.
You stop there, because one more would get you back to the beginning again.
Calculating cosines for two
You can use three different formulas to find the value for cos 2x — the double-angle of cosine — so your job is to choose which one best fits into the problem. The double-angle formula for cosine comes from the sum formula, just like the double-angle formula for sine. If you can’t remember the double-angle formula but you can remember the sum formula, just simplify cos(2x), which is the same as cos(x + x). Because using the sum formula for cosine yields cos 2x = cos2 x – sin2 x, you have two additional ways to express this by using Pythagorean identities (see Chapter 8):
![]() You can replace sin2 x with (1 – cos2 x) and simplify.
You can replace sin2 x with (1 – cos2 x) and simplify.
![]() You can replace cos2 x with (1 – sin2 x) and simplify.
You can replace cos2 x with (1 – sin2 x) and simplify.
 Following are the possible formulas for the double-angle of cosine:
Following are the possible formulas for the double-angle of cosine:
![]() cos 2x = cos2 x – sin2 x
cos 2x = cos2 x – sin2 x
![]() cos 2x = 2 cos2 x – 1
cos 2x = 2 cos2 x – 1
![]() cos 2x = 1 – 2 sin2 x
cos 2x = 1 – 2 sin2 x
Looking at what you’re given and what you’re asked to find usually will lead you toward the right formula. And hey, if you don’t pick the right one at first, you have two more to try!
Here’s an example problem: If sec x = –15/8, find the exact value of cos 2x if x is in quadrant II of the coordinate plane. Follow these steps to solve:
1. Use the reciprocal identity (see Chapter 8) to change secant to cosine.
Because secant doesn’t appear in any of the possible formula choices, you have to complete this step first. Therefore,
cos x = –8/15
2. Choose the appropriate double-angle formula.
Because you now know the cosine value, you should choose the second double-angle formula for this problem:
cos 2x = 2 cos2 x – 1
3. Substitute the information you know into the formula.
You can plug cosine into the equation:
cos 2x = 2 · (–8/15)2 – 1
4. Simplify the formula to solve.
cos 2x = 2 · (64/225) – 1 = (128/225) – 1 = –97/225
Squaring your cares away
We’ve said it before, and we’ll say it again: When a square root appears inside a trig proof, you have to square both sides at some point to get where you
need to go. For example, say you have to prove ![]() .
.
The square root on the right means that you should try squaring both sides:
1. Square both sides.
You have
![]()
This equation gives you
4 sin4 x – 4 sin2 x + 1 = 1 – sin2 2x
2. Look for identities.
You can see a double angle on the right side:
sin2 2x = (sin 2x)2
That gives you
1 – (2 sin x cos x)2
which is the same as
4 sin4 x – 4 sin2 x + 1 = 1 – 4 sin2 x cos2 x
3. Change all sines to cosines or vice versa.
Because you have more sines, change the cos2 x by using the Pythagorean identity to get this equation:
4 sin4 x – 4 sin2 x + 1 = 1 – 4 sin2 x(1 – sin2 x)
4. Distribute the equation.
You end up with
4 sin4 x – 4 sin x + 1 = 1 – 4 sin2 x + 4 sin4 x
Using the commutative and associative properties of equality (from Chapter 1), you get
4 sin4 x – 4 sin x + 1 = 4 sin4 x – 4 sin x + 1
Having twice the fun with tangents
Unlike the formulas for cosine (see the “Calculating cosines for two” section), tangent has just one double-angle formula. That should make you happy, because you have fewer places to get confused. The double-angle formula for tangent is used less often than the double-angle formulas for sine or cosine; however, you shouldn’t overlook it just because it isn’t as popular as its cooler counterparts! (Be advised, though, that instructors drill more heavily on sine and cosine.)
The double-angle formula for tangent is derived by simplifying tan(x + x) with the sum formula. However, the simplification process is much more complicated here because it involves fractions. So we advise you to just memorize the formula.
 The double-angle identity for tangent is
The double-angle identity for tangent is ![]() .
.
 When solving equations for tangent, remember that the period for the tangent function is π. This detail is important — especially when you have to deal with more than one angle in an equation — because you usually need to find all the solutions on the interval [0, 2π). Double-angle equations have twice as many solutions in that interval as single-angle equations do.
When solving equations for tangent, remember that the period for the tangent function is π. This detail is important — especially when you have to deal with more than one angle in an equation — because you usually need to find all the solutions on the interval [0, 2π). Double-angle equations have twice as many solutions in that interval as single-angle equations do.
Follow these steps to find the solutions for 2 tan 2x + 2 = 0 on the interval [0, 2π):
1. Isolate the trig function.
Subtract 2 from both sides to get 2 tan 2x = –2. Divide both sides of the equation by 2 next: tan 2x = –1.
2. Solve for the double-angle by using inverse trig functions.
On the unit circle, the tangent is negative in the second and fourth
quadrants. Moreover, the tangent is –1 at ![]() and
and ![]() , where k is an integer.
, where k is an integer.
Note: You have to add π · k to each solution to find all the solutions of the equation (see the earlier section “Finding the sine of a doubled angle”).
3. Isolate the variable.
Divide both sides of the equation by 2 to find x. (Remember that you have to divide both the angle and the period by 2.) This step gives you
![]() and
and ![]() .
.
4. Find all the solutions on the required interval.
Adding π/2 to π/8 and (7π)/8 until you repeat yourself gives you all the solutions to the equation. Of course, first you must find a common denominator — in this case, 8:
• ![]()
• ![]()
• ![]()
• ![]()
However, (19π)/8 is co-terminal with (3π)/8, so you’re right back where you started. Now you’ve found all the solutions.
Taking Trig Functions of Common Angles Divided in Two
Some time ago, those pesky trigonometricians (we made up that word, by the way) found ways to calculate half of an angle with an identity. As you find out how to do with the sum and difference identities earlier in this chapter, you can use half-angle identities to evaluate a trig function of an angle that isn’t on the unit circle by using one that is. For example, 15°, which isn’t on the unit circle, is half of 30°, which is on the unit circle. Cutting special angles on the unit circle in half gives you a variety of new angles that can’t be achieved by using the sum and difference formulas or the double-angle formulas. Although the half-angle formulas won’t give you all the angles of the unit circle, they certainly get you closer than you were before.
 The trick is knowing which type of identity serves your purpose best. Half-angle formulas are the better option when you need to find the trig values for any angle that can be expressed as half of another angle on the unit circle. For example, to evaluate a trig function of π/8, you can use the half-angle formula of π/4. Because no combination of sums or differences of special angles gets you π/8, you know to use a half-angle formula.
The trick is knowing which type of identity serves your purpose best. Half-angle formulas are the better option when you need to find the trig values for any angle that can be expressed as half of another angle on the unit circle. For example, to evaluate a trig function of π/8, you can use the half-angle formula of π/4. Because no combination of sums or differences of special angles gets you π/8, you know to use a half-angle formula.
You also can find the values of trig functions for angles like π/16 or π/12, each of which are exactly half of angles on the unit circle. Of course, these angles aren’t the only types that the identities work for. You can continue to halve the trig-function value of half of any angle on the unit circle for the rest of your life (if you have nothing better to do). For example, 15° is half of 30°, and 7.5° is half of 15°.
 The half-angle formulas for sine, cosine, and tangent are as follows:
The half-angle formulas for sine, cosine, and tangent are as follows:
![]()
![]()
![]()
![]()
![]()
![]()
 In the half-angle formula for sine and cosine, notice that ± appears in front of each radical (square root). Whether your answer is positive or negative depends on which quadrant the new angle (the half angle) is in. The half-angle formula for tangent doesn’t have a ± sign in front, so the above doesn’t apply to tangent.
In the half-angle formula for sine and cosine, notice that ± appears in front of each radical (square root). Whether your answer is positive or negative depends on which quadrant the new angle (the half angle) is in. The half-angle formula for tangent doesn’t have a ± sign in front, so the above doesn’t apply to tangent.
For example, to find sin 165°, follow these steps:
1. Rewrite the trig function and the angle as half of a unit circle value.
First realize that 165° is half of 330°, so you can rewrite the sine function as sin(330/2).
2. Determine the sign of the trig function.
Because 165° is in quadrant II of the coordinate plane, its sine value should be positive.
3. Substitute the angle value into the right identity.
The angle value 330° plugs in for x in the positive half-angle formula for sine. This gives you
![]()
4. Replace cos x with its actual value.
Use the unit circle to find the cos 330°. Substituting that value into the equation gives you
![]()
5. Simplify the half-angle formula to solve.
This approach has three steps:
a. Find the common denominator for the two fractions on top (including 1/1) to get
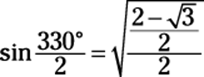
b. Use the rules for dividing fractions to get
![]()
c. Finally, the square of the bottom simplifies to 2, and you end up with
![]()
A Glimpse of Calculus: Traveling from Products to Sums and Back
You’ve now reached the time-travel portion of the chapter, because all the information from here on comes into play mainly in calculus. In calc, you’ll have to integrate functions, which is much easier to do when you’re dealing with sums rather than products. The information in this section helps you prepare for the switch. Here we show you how to express products as sums and how to transport from sums to products.
 The information in this section is theoretical and applies specifically to calculus. We wish we had some really great real-world examples relating to this topic, but alas, it’s just theory that you need to know to get ready for calc.
The information in this section is theoretical and applies specifically to calculus. We wish we had some really great real-world examples relating to this topic, but alas, it’s just theory that you need to know to get ready for calc.
Expressing products as sums (or differences)
Integration of two things being multiplied together is extremely difficult, especially when you must deal with a mixture of trig functions. If you can break up a product into the sum of two different terms, each with its own trig function, doing the math becomes much easier. But you don’t have to worry about any of that right now. In pre-calculus, problems of this type usually say “express the product as a sum or difference.” For the time being, you’ll make the conversion from a product, and that will be the end of the problem.
You have three product-to-sum formulas to digest: sine · cosine, cosine · cosine, and sine · sine. The following list breaks down these formulas:
![]() Sine · cosine:
Sine · cosine: ![]()
Suppose that you’re asked to find 6 cos q sin 2q as a sum. Rewrite this expression as 6 sin 2q cos q (thanks to the commutative property) and then plug what you know into the formula to get
![]()
![]() Cosine· cosine:
Cosine· cosine: ![]()
For example, to express cos 6θ cos 3θ as a sum, rewrite it as the following:
![]()
![]() Sine · sine:
Sine · sine: ![]()
To express sin 5x cos 4x as a sum, rewrite it as the following:
![]()
Transporting from sums (or differences) to products
On the flip side of the previous section, you need to familiarize yourself with a set of formulas that change sums to products. Sum-to-product formulas are useful to help you find the sum of two trig values that aren’t on the unit circle. Of course, these formulas work only if the sum or difference of the two angles ends up being an angle from the special triangles from Chapter 6.
Here are the sum/difference-to-product identities:
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
For example, say you’re asked to find sin 105° + sin 15° without a calculator. You’re stuck, right? Well, not exactly. Because you’re asked to find the sum of two trig functions whose angles aren’t special angles, you can change this to a product by using the sum to product formulas. Follow these steps:
1. Change the sum to a product.
Because you’re asked to find the sum of two sine functions, use this equation:
![]()
This step gives you
![]()
2. Simplify the result.
Combining like terms and dividing gives you 2 sin 60° cos 45°. Those numbers are unit circle values, so continue to the next step.
3. Use the unit circle to simplify further.
![]() and
and ![]()
Substituting those values in, you get
![]()
![]()
Eliminating Exponents with Power-Reducing Formulas
Power-reducing formulas allow you to get rid of exponents on trig functions so you can solve for an angle’s measure. This ability will come in very handy when you get to calculus. (You’re just going to have to trust us that you need to know this information!)
 In the future, you’ll definitely be asked to rewrite an expression using only the first power of a given trig function — either sine, cosine, or tangent — with the help of power-reducing formulas, because exponents can really complicate trig functions in calculus when you’re attempting to integrate functions. In some cases, when the function is raised to the fourth power or higher, you may have to apply the power-reducing formulas more than once to eliminate all the exponents. You can use the following three power-reducing formulas to accomplish the elimination task:
In the future, you’ll definitely be asked to rewrite an expression using only the first power of a given trig function — either sine, cosine, or tangent — with the help of power-reducing formulas, because exponents can really complicate trig functions in calculus when you’re attempting to integrate functions. In some cases, when the function is raised to the fourth power or higher, you may have to apply the power-reducing formulas more than once to eliminate all the exponents. You can use the following three power-reducing formulas to accomplish the elimination task:
![]()
![]()
![]()
![]()
![]()
![]()
For example, follow these steps to express sin4 x without exponents:
1. Apply the power-reducing formula to the trig function.
First, realize that sin4 x = (sin2 x)2. Because the problem requires the reduction of sin4 x, you must apply the power-reducing formula twice. The first application gives you the following:
![]()
2. Foil the numerator.
![]()
3. Apply the power-reducing formula again (if necessary).
Because the equation contains cos2 2x, you must apply the power- reducing formula for cosine.
Because writing a power-reducing formula inside a power-reducing formula is very confusing, find out what cos2 2x is by itself first and then plug it back in:
![]()
![]()
4. Simplify to get your result.
Factor out 1/2 from everything inside the brackets so that you don’t have fractions both outside and inside the brackets. This step gives you
![]()
Combine like terms to get
![]()