Cracking the AP Chemistry Exam

Part IV

Content Review for the AP Chemistry Exam

Chapter 8

Big Idea #6: Equilibrium, Acids and Bases, Titrations, and Solubility

WEAK ACIDS

When a weak acid (often symbolized with HA) is placed in water, a small fraction of its molecules will dissociate into hydrogen ions (H⁺) and conjugate base ions (A⁻). Most of the acid molecules will remain in solution as undissociated aqueous particles.

The dissociation constants, K_a and K_b, are measures of the strengths of weak acids and bases. K_a and K_b are just the equilibrium constants specific to acids and bases.

Acid Dissociation Constant

K_a =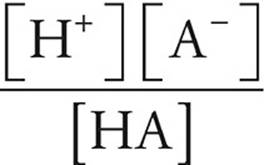

[H⁺]=concentration of hydrogen ions (M)

[A⁻]=concentration of conjugate base ions (M)

[HA]=concentration of undissociated acid molecules (M)

Base Dissociation Constant

K_b =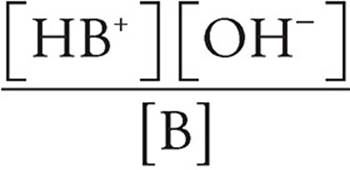

[HB⁺]=concentration of conjugate acid ions (M)

[OH⁻]=concentration of hydroxide ions (M)

[B]=concentration of unprotonated base molecules (M)

The greater the value of K_a, the greater the extent of the dissociation of the acid and the stronger the acid. The same thing goes for K_b, but in the case of K_b, the base is not dissociating. Instead, it is accepting a hydrogen ion (proton) from an ion. So, a base does not dissociate- it protonates (or ionizes).

If you know the K_a for an acid and the concentration of the acid, you can find the pH. For instance, let’s look at 0.20-molar solution of HC₂H₃O₂, with K_a=1.8 × 10⁻⁵.

First we set up the K_a equation, plugging in values.

HC₂H₃O₂→H⁺+C₂H₃O₂⁻

K_a =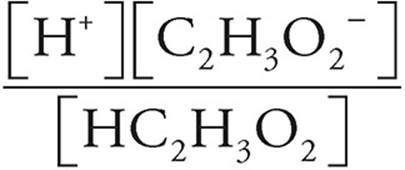

Therefore, the ICE (Initial, Change, Equilibrium) table for the above problem is as follows:

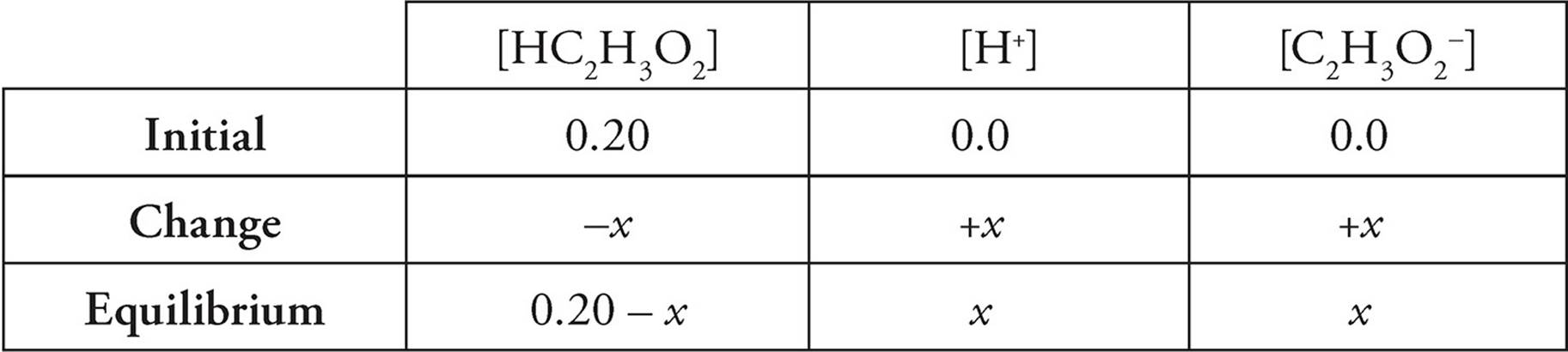
Because every acid molecule that dissociates produces one H⁺ and one C₂H₃O₂⁻.

[H⁺]=[C₂H₃O₂⁻]=x

and because, strictly speaking, the molecules that dissociate should be subtracted from the initial concentration of HC₂H₃O₂, [HC₂H₃O₂] should be (0.20 M − x). In practice, however, x is almost always insignificant compared with the initial concentration of acid, so we just use the initial concentration in the calculation.

[HC₂H₃O₂]=0.20 M

Now we can plug our values and variable into the K_a expression.

1.8 × 10⁻⁵ =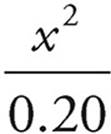

Solve for x.

x=[H⁺]=1.9 × 10⁻³

Now that we know [H⁺], we can calculate the pH.

pH =−log [H⁺]=−log (1.9 × 10⁻³)=2.7

This is the basic approach to solving many of the weak acid/base problems that will be on the test.