CHEMICAL BIOLOGY
Passive Diffusion Across Membranes
Martin Brandl, Goril Eide Flaten and Annette Bauer-Brandl, University of Tromso, Drug Transport & Delivery Group, Breivika, Tromso, Norway
doi: 10.1002/9780470048672.wecb432
This article deals with the passive transport of electrolytes, water, and small organic molecules across biologic membranes (cell membranes and membranes that confine cellular compartments). Passive transport may be classified into diffusion, facilitated diffusion, and bulk flow. The focus in the current article is on simple diffusion, a process described by the random movement of solutes that results in the net transport (flux) along a concentration gradient. This process, at first, may be described by the first law of Fick. More detailed models take into account the partitioning of solutes between the aqueous media and the barrier itself, as well as the flux resistance that arises from water layers adherent to the barrier, which results in the second law of Fick. The actual extent of the diffusion of molecules across biologic membranes is caused by the interaction between the composition and the structural arrangement of the membrane and by the physico-chemical characteristics of the permeant. For the sake of simplification, the primary structural element of a biomembrane, which is a phospholipid bilayer, can be considered a continuous, lipophilic phase between two aqueous compartments. Commonly used permeability screening approaches include in silico modeling, liposome-based experimental models, cell culture models, and in situ perfusion.
The permeability of compounds through cell membranes is of great interest and importance for the elucidation of many biologic cell functions. Most metabolically important substances are transported across membranes by active transport. Many other intrinsic compounds, as well as most drugs, are known to pass the membrane by passive diffusion.
Biologic Background
Biologic membranes as transport barriers
The most prominent function of biologic membranes is to control selectively the molecular transport into and out of cells. The ability of certain molecules to traverse such biologic barriers depends on their composition as well as their structural and functional features. These features are discussed in more detail on a molecular level elsewhere in this encyclopedia. In brief, the plasma membrane of a cell, as well as any other biologic membrane, is organized basically as a bilayer structure of two sheets composed of phospholipids and neutral lipids, like cholesterol, in which membrane proteins are embedded. The polar head-group of the phospholipids is facing toward the aqueous phases, which are the cytosol on the one side and the medium around the cell on the other, whereas the lipophilic chains of the sheets face to one another to form the center of the membrane.
At first glance, such a membrane might be assumed to be a tight barrier that separates the cell interior from its surrounding or cellular compartments from each other, not allowing for any transport of compounds across this barrier. However, particular molecules need to pass through cell barriers to maintain continuously the basic functionality of the cells; they need to introduce and load substrates as well as remove and discharge toxic compounds. Furthermore, overall cell volume, osmotic pressure, intracellular pH, and ionic composition are controlled by the passage of molecules through the cell membrane (1).
Types of transport processes across cell membranes
Transport of molecules across biomembranes can be classified mechanistically into the following types: 1) active transport via carriers, 2) passive transport, which comprises a) simple diffusion and b) facilitated diffusion, and 3) endocytosis/transcytosis.
Active transport consumes energy through coupling with cell metabolism. It is accomplished typically via substrate-specific carrier proteins. Therefore, active transport can be maintained against a concentration gradient, which means, for example, certain substances selectively can be picked up independently of their concentration in the cell. Also, a toxic substance may be removed completely from the cell while an appreciable concentration of the same substance still exists in the surrounding medium.
In contrast to active transport, passive transport as a whole does not involve energy consumption and, therefore, only can work down a concentration gradient (or other types of gradients, such as electrochemical potential, thermal, or pressure gradients). In other words, passive transport of molecules equalizes their chemical potential on both sides of the membrane. The process of passive transport can be subdivided into two different mechanisms: passive diffusion and facilitated transport. Passive diffusion is a physico-chemical process, whereas in facilitated transport, molecules pass through the membrane via special channels or are translocated via carrier proteins. Both passive diffusion and facilitated transport, in contrast to active transport, follow a gradient, where facilitation merely lowers the activation energy for the transport process.
In contrast to the above-discussed molecular transport mechanisms, endocytosis, exocytosis, and transcytosis represent the transport of compounds across plasma membranes along with the bulk of their surrounding aqueous medium through vesicle formation (invagination), translocation of the vesicle, and subsequent membrane fusion, a process that also requires energy. These topics are not covered in this article.
Transport across epithelial barriers
Transport across epithelial barriers, such as the gastrointestinal (GI) wall or the blood/brain-barrier, is the result of a series of different, sequential, and parallel processes. Nevertheless, they may be modelled in many cases in the same way as transport across a single membrane.
As a class of tissue, epithelia demarcate body entry points, predisposing a general barrier function with respect to solute entry and translocation. The intestine is lined with enterocytes, which are polarized cells with their apical membrane facing the intestinal lumen that is separated by tight junctions from the basolateral membrane that faces the subepithelial tissues. In addition to their barrier function, the epithelia that line the GI tract serve specialized functions that promote efficient nutrient digestion and absorption and support other organs of the body in water, electrolyte, and bile salt homeostasis. The homeostatic demand on GI tissue that results from this dual function may pose special transport consideration compared with solute translocation across biologically inert barriers.
Passively absorbed compounds diffuse either through the cell itself (transcellular pathway) or in between cells (paracellular pathway). The lipid bilayers of which the mucosal and basolateral epithelial cell membranes are composed of, define the primary transcellular diffusion resistance to solute transport across the intestinal barrier. Transcellular permeability, particularly of lipophilic solutes, depends on their partitioning between intestinal membrane and aqueous compartments (Fig. 1).

Figure 1. Pathways of the intestinal barrier. A: paracellular passive diffusion, B: transcellular passive diffusion, CF: influx/efflux facilitated transport facilitated by membrane proteins, G: transcytosis, and H: endocytosis (reprinted from Reference 2 with kind permission from Dr. Jon Vaben and Dr. Roy Lysaa).
Physico-Chemical Approach to Passive Transport
Passive transport is the process of mass transfer across any separating barrier, diaphragm, membrane, or partition “wall” toward equilibrating any differences in chemical potential because of gradients of concentration, electrostatic potential, thermal, or (partial) pressure gradient.
Individual molecules and/or ions (if we, for the moment, disregard the solvation shell) traverse the barrier to equilibrate the gradient. The velocity of the passage of individual molecules affects the overall kinetics of transport with respect to both the lag time (time until steady-state of transport is reached), the time period after which the equilibrium is reached, and—in the case of nonsymmetrical systems like cell membranes—the equilibrium state. Properties of both the barrier (membrane) and the diffusant (traversing molecule) are of importance for the mechanism of passage, particularly their interaction on the molecular level. Here, solvation shells and energies of solvation/resolvation play a major role. Solvation states and intermediate states affect activation energies for the passage of the molecules.
Quantitative description of the diffusion process
Let us consider diffusion of molecules between two compartments in the first place, for the sake of simplicity, without a (rate-influencing) barrier between them (Fig. 2, Scheme 2a). The donor compartment contains a higher concentration of diffusant (CD) compared with the concentration in the acceptor compartment (CA); in other words, a concentration gradient exists. Furthermore, also for the sake of simplicity, we consider transport along a line, for example, in one dimension only.

Figure 2. Schematic illustration of concentration gradients. CD concentration in the donor compartment; CA concentration in the acceptor compartment. Scheme 2a: without a physical barrier; Scheme 2b: lipid barrier-controlled; Scheme 2c: water layer-controlled; and Scheme 2d: combined lipid- and water layer-controlled.
Definitions
Flux (J) of a species is the mass (M) (or number of molecules of this species) transported per unit time across the barrier, normalized by the cross-sectional surface area A of the barrier:
![]()
(dimension of J: g sec-1 cm-2)
A plot of the mass transported versus time is linear if the donor concentration is virtually constant and the acceptor concentration is virtually zero; the flux is constant when the system has reached the equilibrium called steady state (i.e., the linear part in Fig. 3):
![]()
The slope of the curve corresponds to J ∙ A. In most experimental setups, the surface area A is known and flux J can be derived. Constant flux is maintained as long as CD is much larger than CA and as long as both are practically unchanged. In biologic systems, a good example for such conditions may be the uptake of xenobiotics from the GI-tract, where the GI tract acts as a reservoir for the molecules, which are transported through the intestinal epithelium into the blood, which acts as a sink.

Figure 3. Diagram illustrating mass transport versus time, lag time, and steady-state flux.
The quantitative value of flux is widely dependent on the concentration in the donor compartment CD (figuratively spoken, the pressure). Ideally, CD should not change over the period of observation; if CD is not constant, its change with time needs to be considered, which in many cases applies to real situations. However, the concentration in the acceptor compartment should be kept neglectably small (sink conditions) to make calculations easier. Fortunately, in biologic systems, this scenario is very often the case and experimental conditions in most cases can be chosen accordingly. It is handy to define permeability P as the inverse of the resistance of the membrane, which is the flux normalized by the concentration in the donor compartment.
![]()
Permeability, therefore, comes in the dimension of cm/sec, a self-explanatory unit.
Combining Equation 1 and 2 reveals:
![]()
In cases where CD is not constant over time, the flux J also changes with time. Exchanging mass by concentration, M = CD ∙ V, where V is the concentration of the donor phase, reveals:
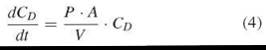
Setting k = P*A/V, integration of Equation 4
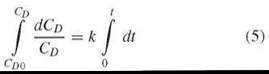
reveals
![]()
The sigma minus plot (i.e., plotting ln CD0 — ln CD vs. time) yields permeability as the slope k, where still k = P*A/V.
A similar general expression also can be used under nonsink conditions. Here it is useful to describe the velocity of transport (i.e., flux J) expressing the effect of the concentration gradient (∆C) and the length of the dimension along the line (∆x), as well as to introduce the diffusion coefficient as the proportionality constant (the Fick law).
![]()
This description is particularly useful because the diffusion coefficient is reasonably well defined in aqueous solutions, it is related to molecular properties in aqueous solutions, and it can be predicted. However, in biologic systems, the observed length x—for a single transport step—will be widely unchanged. Preferably, steady state and sink conditions are studied, which simplifies the Fick law and focuses on permeability as stated above.
Let us now consider a barrier between the donor and the acceptor compartment, where the barrier has different properties in terms of the solvation of the traversing molecules, as a simplification of a biomembrane. Such a model is shown in Fig. 2, beginning with Scheme 2b, where a homogeneous lipid barrier between the donor and the acceptor compartment is introduced. The permeability also is defined in this case as described above and as indicated in the Scheme. Looking closer into the system, the diffusant will partition into the lipid barrier according to the ratio of solubility both in the aqueous phases and in the lipid phase (the partition coefficient, i.e., the ratio of chemical potentials in the two phases). The concentration ratios in the two interfaces will be equal, but not the absolute concentrations, as the concentration in the donor compartment is higher than in the acceptor compartment. The gradient between the two interfaces will make the diffusion occur (see Fig. 2).
The diffusion coefficient in the aqueous phases (donor and acceptor phase) would not play a role if practically no concentration gradient exists within these compartments, in other words, if the diffusion in the aqueous compartments is not rate determining. The partition coefficient between the lipid phases and aqueous phases, K, determines whether CmD and CmA, are higher or lower than CDand CA, respectively.
If the diffusion of the permeant in the lipid barrier phase is rate determining, then the thickness of the barrier has to be taken into account as well. Here, the permeability coefficient is proportional to its diffusion coefficient D in the membrane (slow diffusion in the membrane).
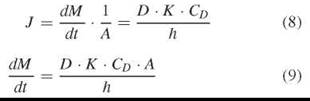
Again, given a practically unchanged CD, the mass transport still reveals the simple form:
![]()
Also in this example, flux J is constant during the steady state and permeability P can be revealed. However, here we have to consider a lag time tL. For a constant flux, we have to wait until the lipid barrier is saturated and shows a constant gradient of the diffusant according to the partition coefficient. The lag time that represents the intercept with the time axis is found by extrapolating the linear part of the curve (Fig. 3).
![]()
Lag time tL would be a measure of membrane thickness h and the diffusion coefficient (diffusion velocity) in the membrane.
The barrier models can be increasingly more complex with respect to the structure of the barriers:
• Adjacent water layers on both sides of the lipid barrier (Fig. 2, Schemes 2c and 2d).
• Nonhomogeneous barriers (as are cell membranes)
• Nonsymmetrical barriers (as are cell membranes)
Any of the components of the barrier may be rate determining, and in some cases, several of them are important for the diffusion process (Fig. 2, Scheme 2d; combined control). In any case, it is most straightforward to look at the overall permeability of the entire barrier. The overall permeability (apparent permeability)—as the reciprocal of the overall resistance—is the sum of the resistances of all the layers involved. One should keep in mind that permeability is a material property of the system with respect to the interaction of the solute, barrier, temperature, solution composition, and other factors.
Furthermore, molecules at each step would be in a solvated state. However, these solvation states cannot be directly experimentally measured, easily predicted, nor modeled. The most simple and effective mathematical approach, therefore, is to look at the entire barrier as a whole and to stick to the evaluation of the overall flux and permeability, respectively, as stated above, keeping in mind that it is the interaction on the molecular level that explains these conditions. The overall transport is described, then—as it is not physico-chemically consistent—in the terminology of “apparent permeability,” Papp.
Nonsink conditions are less appropriate for experimental work on transport through barriers and, thus, are not discussed more here.
Diffusion with respect to physico-chemical characteristics of the permeate
In the beginning of the last century, it was observed that the permeability of a substance across a cell membrane is proportional to the relative partition coefficient of the permeating substance between phases of oil and water. In later years, it was found that the product of the permeability coefficient P and the square root of the molecular weight of a permeant shows a better correlation with the partition coefficient than does permeability alone. This correlation has lead to the idea that permeation is limited not only by the lipid solubility of the permeant but also by a “screen-like” property of the membrane because small molecules penetrate faster than would be predicted from their oil-water partition coefficients. For larger molecules, however, one would expect a product of permeability with the cube root, rather than the square root, of the molecular weight to be more closely correlated with the oil-water partition coefficient. Likewise, the apparent partition coefficient to the membrane plays a major role for the transport processes across the membrane. In strict physico-chemical terms, a membrane is not a phase, but it is most common to treat it as such anyway.
Partitioning of a substance between two phases (e.g., the aqueous phase and the membrane) may be expressed by the difference in Gibbs free energy between the two phases. Major energy differences of the permeant between the two phases are because of the hydrogen-bonding abilities of the substance and the energy of hydration and/or solvation. In addition, nonpolar molecular interactions and entropy terms also contribute to the energy difference. A plot of the logarithm of the partition coefficient versus the number of hydrogen bond donors and acceptor of the permeant gives a straight line (3, 4). The partitioning of a molecule at the water-membrane interface is greatly dependent on its polar nature. The more polar a substance, the higher are the energy expenses for the substance to enter into the membrane phase. For example, the permeability and partition into the membrane decrease approximately exponentially with the number of OH groups of the permeating molecule (3, 4).
Membrane uptake of the noncharged form of a solute is favored over its charged form. As a result, solute membrane permeability versus pH curves is shifted toward the right for weak acids and toward the left for weak bases (5). This condition also explains the irregular permeability behavior of ion pairs.
Passive transport of water and protons
The movement of water and protons/hydroxyl ions across the cell membrane is of particular importance for the existence of the cell (6).
Two passive processes move water through membranes: diffusion and hydrodynamic flow. A semipermeable membrane is a membrane that will allow certain molecules (i.e., water) or ions to pass through it by diffusion. If the concentrations of a substance for which the membrane is impermeable are different on the two sides of the membrane, then water will be transported to equilibrate concentrations, for example, a stream of water toward the higher concentration is driven by osmotic pressure.
For the permeation of protons and hydroxyl ions, it has been shown that a prerequisite is the formation of membrane spanning water aggregates in which just the electrons are switched from one membrane side to the other (6). This prerequisite is in contrast to what is found for other ions.
Simple diffusion of nonelectrolytes
In the case of nonequilibrium spatial distribution of a certain species, characterized by a nonuniform distribution of chemical potential, diffusion of this species will occur in accordance with the gradient of its chemical potential. If a gradient exists in the chemical potential for one of the species, then a statistical force will be exerted on the particle distribution. The average velocity of a particle will be given by the product of its mobility and the sum of the forces acting on it (4). During the transport of a substance across a membrane, it must move through phase boundaries, such as aqueous phase/membrane/aqueous phase. As mentioned before, the substance has different affinities to each phase encountered. In most cases, the diffusion within the membrane is much slower than in the liquid phase.
So, permeability is proportional to the diffusion coefficient in the membrane and the partition coefficient between the membrane and aqueous phases, while being inversely proportional to the thickness of the membrane. The molecular weight and shape of the permeant are related closely to all of those characteristics.
It is reasonable to expect that nonelectrolytes normally have to take off their tightly bound hydration shell, at least partly, before they passively permeate a lipid bilayer. This mechanism has been studied for glucose, where it was assumed that the number of bound water molecules correlates with the activation energy of the permeation process (7).
Facilitated transport of electrolytes and nonelectrolytes
In contrast to small uncharged molecules that can diffuse across cell membranes, hydrophilic molecules and ions do not partition readily into the lipid barrier. Biologic fluids contain a variety of such charged species that originate from the dissociation of salts, acids, and bases. Another kind of transport, facilitated diffusion, is prevalent for them because their permeation via simple diffusion is rather limited because of limited partitioning into the biomembrane. Facilitated diffusion is a process where permeants diffuse across membranes with the aid of transport proteins. Water-filled pores in the membrane formed by proteins are the main pathway for ions. These pores may be gated so that they can open and close, thus regulating the ion flux. Another kind of membrane proteins, carrier proteins that change shape as the molecules are being carried through, are responsible for the facilitated transport of larger molecules like glucose and amino acids. In contrast to active transport, facilitated diffusion does not require energy and only can carry molecules or ions down a concentration gradient.
The transport proteins involved are intrinsic, that is, they span the membrane. They are solute-specific, for example, they have binding sites for the specific molecule or ion that they transport. They also have a defined capacity of how many solutes they can transport. An example of facilitated diffusion is glucose that permeates through cell membranes rather slowly via conventional diffusion because it is not soluble in the membrane. However, glucose passes quickly across a cell membrane via facilitated diffusion.
Tools and Techniques to Study Diffusion Across Membranes
Both the prediction and the studying of transport processes through biologic membranes are of great interest in many fields. For example, in drug development, permeability across the intestinal barrier is of essential importance for the destiny of a drug candidate and in molecular biology, knowledge about the pathways in cells of endogenous compounds is of interest. Many different techniques to study such phenomena, thus, have been developed during the years. The complexity of the used techniques ranges from chromatographic systems with covalently bound membrane phospholipid to in situ studies with segments of animals.
Prediction of diffusion based on experimentally determined physico-chemical characteristics
Chromatographic methods
Chromatography also can be used to predict permeability. The most widely used methods are immobilized artificial membrane chromatography (IAM) and immobilized liposome chromatography (ILC).
Immobilized artificial membrane chromatography (IAM)
IAM chromatography is a reverse-phase liquid chromatography method that emulates the lipid environment of cell membranes by using a chromatographic surface prepared by covalently immobilized cell membrane phospholipids. The technique is experimentally simple and can screen many compounds within a short time. The predominant factor that regulates the passive diffusion across a membrane is its ability to pass through the lipid cell layer, and the capacity factor log Ks determined by this technique shows reasonable correlation with permeability across cell monolayers and partitioning into liposomes (8, 9).
Immobilized liposome chromatography (ILC)
Compared with IAM, which uses a monolayer of phospholipid, the liposomal phospholipid bilayers in ILC have the advantage of closely resembling biologic membrane bilayers and constitute a 2-D fluid in which lipid molecules and other components are free to diffuse (10). With this technique, the phospholipid composition can be changed to mimic the membrane of interest. Membrane lipids extracted from human cells also can be used; the technique then is called immobilized biomembrane chromatography (IBC) (11).
Partitioning and distribution (log P/log D)
The lipophilicity of a drug is the most-used single physicochemical property to predict permeability across biologic membranes (12). The most common distribution model is octanol/ water (log P) or octanol/buffer (log D, at fixed pH).
Several experiments have demonstrated that a correlation exists between log P and the degree of transcellular absorption of a homologous series of compounds. However, for structurally different compounds, such correlation could not be shown. The liposome partitioning system is employed increasingly as an alternative to the octanol approach (13, 14).
In vitro artificial membrane methods
Phospholipid vesicle-based methods
Liposomes
Liposomes can be used to investigate the passive diffusion of compounds across a membrane.
Unilamellar liposomes (Fig. 4) can be loaded passively with the compound of interest, the external water phase replaced by an acceptor medium, and the concentration of this compound measured in the medium as a function of time. Liposome studies allow a complete manipulation of solute environment both inside and outside of the vesicles, thus making it a suitable system for passive mechanistic absorption studies. The so-called “stopped-flow technique” can be used to study permeability through liposome bilayers. The major advantage with this technique is that even very rapid permeation processes can be measured.

Figure 4. Schematic drawing of a unilamellar liposome (reprinted from Reference 15).
The distribution coefficient between a liposome phase (see above about the membrane phase) and a water phase also has been used instead of an octanol/water partition coefficient (log P). The liposome phase provides a biomimetic environment to a much larger degree compared with the octanol phase and has shown to the ability to predict in vivo permeability more precisely (13). Lipid bilayer-containing partition systems thus have been considered to model the hydrogen-bonding ability of biologic membranes better than bulk solvents. Biologic lipid membranes are composed of charged lipids that provide a polar environment at the membrane surface, which in biologic systems, often holds a negative charge. A strong attraction of positively charged molecules, thus, is observed. Liposome partitioning, in contrast to octanol/water partitioning, can take into account hydrogen bonding and electrostatic effects. However, the partition coefficient measured in liposome systems may not reflect permeation always. In some cases, it may reflect more drug interactions with the membrane surface (10, 12).
The phospholipid vesicle-based barrier
The phospholipid vesicle-based barrier is a novel predictive medium-throughput screening method for drug permeability, with the use of a tight barrier of liposomes on a filter support (Fig. 5). The liposomes are deposited into the pores and onto the surface of a filter support by centrifugation. Solvent evaporation and freeze-thaw cycling then are used to promote the fusion of liposomes, and a tight barrier is obtained as shown with calcein permeability and electrical resistance (16). The technique is much less labor intensive than comparable cell-based methods and seems, therefore, appropriate for screening of a larger number of drug candidates in earlier stages of drug development. The advantage of this model compared with the parallel artificial membrane permeability assay (PAMPA) models is that the use of liposomes instead of a mixture of phospholipids in an organic solvent leads to a model that resembles the biologic membrane to a larger extent.

Figure 5. Schematic drawing of the experimental setup used in the permeation studies with the phospholipid vesicle-based barrier (reprinted from Reference 15).
Parallel artificial membrane permeability assay (PAMPA)
The PAMPA model is a simple model for fast determination of transcellular in vivo drug absorption. Coating a hydrophobic filter material with a mixture of lecithin and inert organic solvent creates an artificial lipid membrane (17). The use of 96-well microplates coupled with the rapid analysis using a spectrophotometric plate reader makes this system compatible with automated procedures and, hence, an attractive model for screening many compounds (9). Different variants of this model have been reported in the last 10 years with different lipid compositions: “n-hexadecane” HDM-PAMPA (18), “bio-mimetic” BM-PAMPA (17), and “Double-Sink” DS-PAMPA (19). A schematic drawing of the PAMPA barrier with dissolved phospholipid in an organic solvent on a filter support separating the donor and acceptor chamber is shown in Fig. 6.
What is common for the PAMPA techniques and the phospholipids vesicle-based barrier is that they are much less labor intensive than many cell-based assays, they are versatile and cost efficient, and they are being claimed to be a helpful stand-in for cellular models (20, 21). They also give access to a wider pH range compared with cell-based models, are easier to automate, and give a higher throughput. The limitations of these models are that they underestimate the permeability of actively transported drugs and hydrophilic molecules with low molecular weight and that the use of UV detection excludes drug compound that do not display UV absorbance (8, 9, 20).

Figure 6. Schematic drawing of the PAMPA permeation cell (reprinted from Reference 15).
Cell-based tools
Isolated membrane vesicles
Cell homogenates precipitated by centrifugation and resuspended in a buffer result in the formation of vesicles, which are mixed with the permeant in the buffer and filtrated after a fixed time. The amount of permeant taken up by the vesicles then is determined. Vesicles offer a unique opportunity to study the properties of drug and nutrient transport at the cellular level. In addition to offering a possibility for separating the brush border and basolateral membranes, membrane vesicles studies allow a complete manipulation of the solute environment both inside and outside the vesicle, thus making it an ideal system for mechanistic absorption studies. Uptake studies can be performed with a small amount of the substance to be tested. A unique advantage for this technique is that the vesicles can be cryopreserved and used for a long duration. The drawbacks are that membrane vesicles are used primarily for studying concentrative processes, for example, active carrier-mediated transport, the need for radiolabeled compounds, and day-to-day variations in the preparation (8, 22, 23).
In vitro cell models
In vitro techniques for the measuring of permeability are less labor- and cost-intensive compared with the more complex in situ and in vivo models. In addition, they are more suitable as screening models because they require smaller quantities of test compound. The capacity of an in vitro system to predict permeability across a biologic barrier depends on how closely the model resembles the in vivo system (8, 23).
Single-cell studies
Cells from animal or human origin can be isolated from different tissue and used as uptake systems. Normally, the isolated cells are suspended in a buffer under O2/CO2 in the presence of the permeant and shaken well. After a certain time period, the cells are separated from the buffer and extracted to determine the amount of permeant inside the cells. Because of the low volume of the cells, the assay is based mostly on use of radiolabeled compounds (23). Cells can be isolated from different origin, and one animal can give rise to experiments with many different compounds or conditions. However, many disadvantages exist as well, like the lack of reproducibility with large variations in viability, because of the chemical or mechanical stress during isolation. This method also only allows for uptake, not transport or permeability, to be studied, but the polarity of the uptake; whether a compound is taken up on the apical or basolateral side cannot be decided (22).
Cell monolayer studies
Several different cell monolayer models that mimic in vivo barriers have been developed and are very popular models in industry as well as academia. The ideal model would be a monolayer of polarized normal human enterocytes, but attempts to isolate and grow them have failed because of the low viability and the complicated requirement for attachment, monolayer formation, and differentiation. However, tumor cells grow rapidly into confluent monolayers followed by spontaneous differentiation, which provides a good system for transport studies (22, 23).
Caco-2 cells have been the most extensively characterized and the most used of all cell models, especially in the field of drug permeability and absorption; this model has been considered the “gold standard” used to validate other permeability assays. This cell line originates from the human colon adenocarcinoma, differentiates spontaneously, and forms polarized monolayers with a well-defined brush border on the apical side as well as tight junctions. Other commonly used culture models are: HT-29, T-84, MDCK, LLC-PK1, and 2/4/A1. The HT-29 and T-84 are like the Caco-2 cells, human colon cancer cell lines. MDCK, LLC-PK1, and 2/4/A1 originate in animals. The first two come from kidneys, whereas the last one is derived from fetal rat intestine. The 2/4/A1 can be used to estimate the passive permeability of the human small intestine, especially with regard to paracellular transport, because the tightness of the tight junctions better correlates with the situation in vivo; however, this cell line does not express functional active transport and efflux systems (8, 12, 24, 25).
The use of cell monolayers, instead of single cells or isolated membrane vesicles, provides the opportunity to investigate the transport of drugs across the intestinal epithelial instead of just looking at the uptake of the drug into the cell or vesicle. Sampling on both the apical end basolateral side of the epithelium is possible, and crossover studies can be performed. Another advantage is that the cells are of human origin, different from many tissue-based techniques where animal tissue is used. The system also is amenable to automation. The major drawback of cell monolayer models is the intrinsic variability that can be seen in permeability data. The results obtained in several laboratories can differ by more than an order of magnitude, probably because of the underlying variability in, for example, culturing techniques, genetic drift, and variable expression of transporters. Many cell lines are originations from colon cancer tissue and by that do not resemble the situation in the normal small intestine perfectly, for example, the tight junctions are tighter and thereby can result in underestimation of the permeability of hydrophilic compounds. Caco-2 assays are relatively accessible and more amenable to higher throughput than tissue models, but their culture can be labor-intensive and costly (8, 9, 22-25). A schematic drawing of a cell monolayer assay is shown in Fig. 7.

Figure 7. Schematic drawing of a cell monolayer assay with the cell monolayer on a filter; support separates the apical and basolateral chamber from each other (reprinted from Reference 15).
Tissue models
It is extremely difficult to obtain viable human tissues for permeability studies on a regular basis. Because animal intestinal tissues also are made up of essentially the same kind of endothelial cells, permeability screening for drug discovery purposes routinely is carried out using various animal species. However, the viability of the existing tissues is difficult to maintain because the tissues are devoid of direct blood supply and need constant oxygenation. The everted gut technique, intestinal rings, and the Ussing chamber are the most widely used methods that use tissue for studying absorption and permeability (22). The Ussing chamber can be used to look into the permeability of many different membranes/barriers. Using this technique, the tissue architecture is preserved and closely resembles the in vivo situation and the viability of the tissue can be monitored closely by electrical parameters during the experiment. However, because these techniques also involve the determination of the active transport and efflux mechanisms, we leave these topics to other parts of the encyclopedia.
In vivo and in situ models
In vivo studies are performed in unanesthetized animals or humans. Epithelial permeability has been studied in vivo mainly by perfusion techniques such as double balloon catheter. In situ perfusion methods involve a surgical procedure on anesthetized animals whereby a segment of the intestine is isolated and perfused with drug solution. In situ perfusion of intestinal segments frequently is used to study the permeability and absorption kinetics of drugs. The biggest advantage of the in situ system compared with the in vitro tissue systems is the presence of an intact blood and nerve supply in the experimental animals (8). But because these models are dealing with active transport mechanisms, a more detailed presentation would be beyond the scope of this article.
References
1. Ti Tien H, Ottova-Leitmannova A, Membrane biophysics as viewed from experimental bilayer lipid membranes. In: Membrane Science and Technology. 2000. Elsevier, Amsterdam. pp. 221-282.
2. Vabenp J. Dipeptidomimetics as Pro-Moieties for hPEPT1 Targete Prodrugs. In: Department of Pharmacy, University of Tromso, 2004.
3. Conradi RA, Burton PS, Borchardt RT. Physico-chemical and biological factors that influence a drug’s cellular permeability by passive diffusion: methods and principles in medicinal chemistry. Lipophil. Drug Action Toxicol. 1996; 4:233-252.
4. Ohki S, Sprangler RA. Passive and facilitated transport. In: The Structure of Biological Membranes. Yeagle PL, ed. 2005. CRC Press, Boca Raton, FL. pp. 329-387.
5. Fleisher D, Biological transport phenomena in the gastrointestinal tract: cellular mechanisms: Review article. Drugs and the Pharmaceutical Sciences. Transport Proc. Pharmaceut. Sys. 2000; 102:147-184.
6. Blume A, Lipid phase transitions: water and proton permeability. Methods Enzymol. 1986; 127:480-486.
7. Bresseleers GJ, Goderis HL, Tobback PP. Measurement of the glucose permeation rate across phospholipid bilayers using small unilamellar vesicles. Effect of membrane composition and temperature. Biochim. Biophys. Acta. 1984; 772:374-382.
8. Balimane PV, Chong S, Morrison RA. Current methodologies used for evaluation of intestinal permeability and absorption. J. Pharmacolog. and Toxicol. Methods 2000; 44:301-312.
9. Hamalainen MD, Frostell-Karlsson A. Predicting the intestinal absorption potential of hits and leads. Drug Disc. Today 2004; 1:397-405.
10. Zhang YX, Zeng CM, Li YM, Hjerten S, Lundahl P, Immobilized liposome chromatography of drugs on capillary continuous beds for model analysis of drug-membrane interactions. J. Chromatogr. A. 1996; 749:13-18.
11. Beigi F, Gottschalk I, Lagerquist Hagglund C, Haneskog L, Brekkan E, Zhang Y, Osterberg T, Lundahl P, Immobilized liposome and biomembrane partitioning chromatography of drugs for prediction of drug transport. Int. J. Pharm. 1998; 164:129-137.
12. Malkia A, Murtomaki L, Urtti A, Kontturi K, Drug permeation in biomembranes; In vitro and in silico prediction and influence of physicochemical properties. Eur. J. Pharm. Sci. 2004; 23:13-47.
13. Hellwich U, Schubert R, Concentration-dependent binding of the chiral beta-blocker oxprenolol to isoelectric or negatively charged unilamellar vesicles. Biochem. Pharmacol. 1995; 49:511-517.
14. Kramer SD. Liposome/water partitioning: theory, techniques and applications. In: Pharmacokinetic Optimization in Drug Research. B. Testa, et al., eds. 2001. Wiley-VCH, Zurich.
15. Sugano K, Hamada H, Machida M, Ushio H, High throughput prediction of oral absorption: improvement of the composition of the lipid solution used in parallel artificial membrane permeation assay. J. Biomol. Screen. 2001; 6:189-196.
16. Flaten GE, Dhanikula AB, Luthman K, Brandl M. Drug permeability across a phospholipid vesicle based barrier: A novel approach for studying passive diffusion. Eur. J. Pharm. Sci. 2006; 27:80-90.
17. Kansy M, Senner F, Gubernator K, Physicochemical high throughput screening: parallel artificial membrane permeation assay in the description of passive absorption processes. J. Med. Chem. 1998; 41:1007-1010.
18. Wohnsland F, Faller B. High-throughput permeability pH profile and high-throughput alkane/water log P with artificial membranes. J Med Chem. 2001; 44:923-930.
19. Avdeef A. Absorption and Drug Development; Solubilty, Permeability and Charge State. 1st ed. 2003. Wiley-Interscience, NJ. pp. 116-246.
20. Flaten GE. The phospholipid vesicle-based barrier; A novel method for passive drug permeability screening. In: Department of Pharmacy, University of Tromso, 2007.
21. Kansy M, Avdeef A, Fischer H. Advances in screening for membrane permeability: high-resolution PAMPA for medicinal chemists: Review article. Drug Disc. Today 2004; 1:349-355.
22. Hillgren KM, Kato A, Borchardt RT. In-vitro systems for studying intestinal drug absorption. Medic. Res. Revs. 1995; 15:83-109.
23. Tukker JJ. In vitro methods for the assessment of permeability: drugs and the pharmaceutical sciences. Oral Drug Absorp. 2000; 106:51-72.
24. Artursson P. Cell cultures as models for drug absorption across the intestinal mucosa. Crit. Rev. Therap. Drug Carrier Sys. 1991; 8:305-330.
25. Matsson P, Bergstrom CAS, Nagahara N, Tavelin S, Norinder U, Artursson P. Exploring the role of different drug transport routes in permeability screening. J. Med. Chem. 2005; 48:604-613.
Further Reading
Nielsen PE, Avdeef A. PAMPA—a drug absorption in vitro model—8. Apparent filter porosity and the unstirred water layer. Eur. J. Pharm. Sci. 2004; 22:33-41.
Ruell JA, Tsinman KL, Avdeef A. PAMPA—a drug absorption in vitro model 5. Unstirred water layer in iso-pH mapping assays and pK(a)(flux)—optimized design (pOD-PAMPA. Eur. J. Pharm. Sci. 2003; 20:393-402.
Sugano K, Hamada H, Machida M, Ushio H, Saitoh K, Terada K. Optimized conditions of bio-mimetic artificial membrane permeation assay. Int. J. Pharm. 2001; 228:181-188.
Sugano K, Takata N, Machida M, Saitoh K, Terada K. Prediction of passive intestinal absorption using bio-mimetic artificial membrane permeation assay and the paracellular pathway model. Int. J. Pharm. 2002; 241:241-251.
Ungell AL. In vitro absorption studies and their relevance to absorption from the GI tract. Drug Dev. Ind. Pharm. 1997; 3:879-892.
See Also
Drug Transport
Ion Channels and Pores, Chemical Biology of
Small Molecule Transport
Transport in Cells, Mechanisms of
Water Channels