MCAT General Chemistry Review
Chapter 8: The Gas Phase
8.2 Ideal Gases
When we examine the behavior of gases under varying conditions of temperature and pressure, we assume that the gases are ideal. An ideal gas represents a hypothetical gas with molecules that have no intermolecular forces and occupy no volume. Although real gases deviate from this ideal behavior at high pressures (low volumes) and low temperatures, many compressed real gases demonstrate behavior that is close to ideal.
KEY CONCEPT
An ideal gas follows the gas laws we will discuss at all pressures and temperatures. A real gas deviates from these laws at high pressures (low volumes) and low temperatures because of intermolecular forces or volume effects.
IDEAL GAS LAW
The ideal gas law was first stated in 1834 by Benoît Paul Émile Clapeyron, more than 170 years after Sir Robert Boyle performed his experimental studies on the relationship between pressure and volume in the gas state. In fact, by the time the ideal gas law found its expression, Boyle’s law, Charles’s law, and Dalton’s law had already been well-established. Historical considerations aside, it will benefit us to examine the ideal gas law first so that we can then understand the other laws, which had been identified earlier, to be only special cases of the ideal gas law.
The ideal gas law shows the relationship among four variables that define a sample of gas:
PV = nRT
Equation 8.1
where P is the pressure, V is the volume, n is the number of moles, and T is the temperature. R represents the ideal gas constant, which has a value of 
Be aware that the gas constant can be expressed in other units. On the MCAT, you may also encounter R given as 
which is derived when SI units of pascal (for pressure) and cubic meters (for volume) are substituted into the ideal gas law. Although the relevant values for R will be provided on Test Day if needed, it is important to recognize the appropriate value for R based on the units of the variables given in a passage or question stem.
The ideal gas law is used to determine the missing term when given all of the others. It can also be used to calculate the change in a term while holding two of the others constant. It is most commonly used to solve for volume or pressure at any given temperature and number of moles; Figure 8.2 shows graphs of P‒V relationships at increasing temperatures.
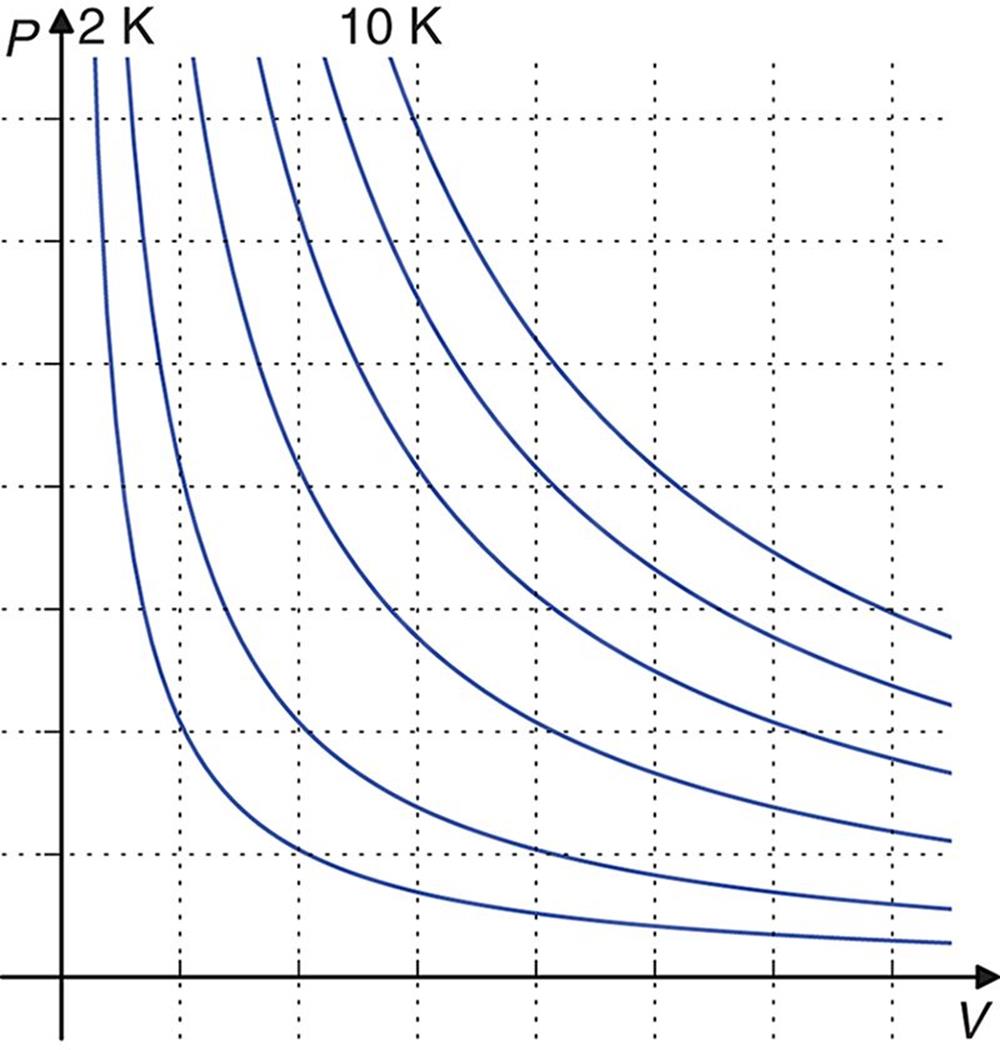Figure 8.2. Ideal Gas Isothermal Curves When n, R, and T are held constant, one can easily analyze the relationship between pressure and volume.
Example:
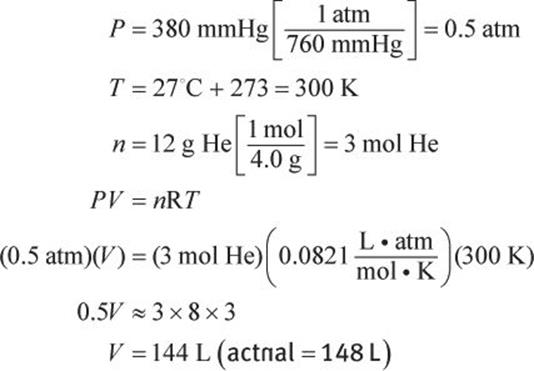
What volume would 12 g of helium occupy at 27°C and a pressure of 380 mmHg?
Solution:
The ideal gas law can be used, but first, all of the variables must be converted to units that will correspond to the expression of the gas constant as 
BRIDGE
Round numbers to speed up your arithmetic on Test Day. For instance, constants such as 0.0821 can be rounded to 0.08. Choices will be sufficiently different so that your estimated answer will be nearly identical to the true answer choice. Arithmetic and math strategies are discussed in Chapter 10 of MCAT Physics and Math Review.
The ideal gas law is useful not only for standard calculations of pressure, volume, or temperature of a gas under a given set of conditions, but also for determinations of gas density and molar mass.
Density
We define density (ρ) as the ratio of the mass per unit volume of a substance. The densities of gases are usually expressed in units of grams per liter. The ideal gas law contains variables for volume and number of moles, so we can rearrange the law to calculate the density of any gas:
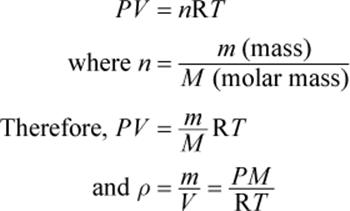
Equation 8.2
A different approach could start with the fact that a mole of an ideal gas at STP occupies 22.4 L. We can then calculate the effect of changes in pressure and temperature when they differ from STP conditions, predicting the volume of the gas. Finally, we’ll calculate the density by dividing the mass by the predicted volume. The following equation, the combined gas law, is an amalgam of some of the special cases we will discuss in the following section. It can be used to relate changes in temperature, volume, and pressure of a gas:
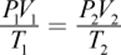
Equation 8.3
where the subscripts 1 and 2 refer to the two states of the gas (at STP and at the conditions of actual temperature and pressure, for example). This equation assumes the number of moles stays constant.
To calculate a change in volume, the equation is rearranged as follows:
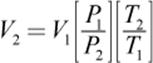
V2 is then used to find the density of the gas under nonstandard conditions:
On Test Day, it may be helpful to visualize how the changes in pressure and temperature affect the volume of the gas, and this can serve as a check to avoid accidentally switching the values of pressure and temperature in the numerator and denominator. For example, one could predict that doubling the temperature of a gas would result in doubling its volume, and doubling the pressure of a gas would result in halving the volume, so doubling both the temperature and pressure at the same time results in a final volume that is equal to the original volume.
Example:
What is the density of CO2 gas at 2 atm and 273°C?
Solution:
At STP, a mole of gas occupies 22.4 L. Because the increase in pressure to 2 atm decreases volume, 22.4 L must be multiplied by 
Because the increase in temperature increases volume, the temperature factor will be 
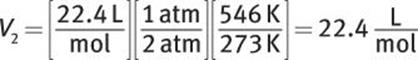
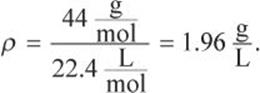
Molar Mass
Sometimes the identity of a gas is unknown, and the molar mass, discussed in Chapter 4 of MCAT General Chemistry Review, can be determined in order to identify it. Using the equation for density derived from the ideal gas law, we can calculate the molar mass of a gas experimentally in the following way:
The pressure and temperature of a gas contained in a bulb of a given volume are measured, and the mass of the bulb with the sample is measured. Then, the bulb is evacuated—the gas is removed—and the mass of the empty bulb is determined. The mass of the bulb with the sample minus the mass of the evacuated bulb gives the mass of the sample. Finally, the density of the sample is determined by dividing the mass of the sample by the volume of the bulb. This gives the density at the given temperature and pressure.
Using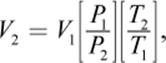 
we then calculate the volume of the gas at STP, substituting 273 K for T2 and 1 atm for P2. The ratio of the sample mass divided by V2 gives the density of the gas at STP. The molar mass can then be calculated as the product of the gas’s density at STP and the STP volume of one mole of gas, 
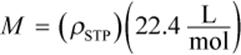
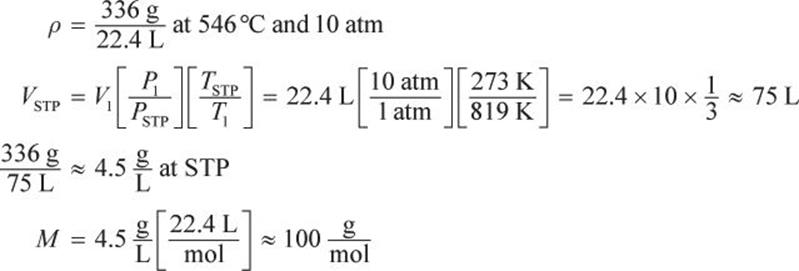
Example:
What is the molar mass of a 22.4 L sample of gas that has a mass of 336 g at a temperature of 546°C and a pressure of 10 atm?
Solution:
SPECIAL CASES
Now that we have considered the ideal gas law as the mathematical relationship between four variables that define the state of a gas (pressure, volume, temperature, and moles of gas), we can examine the other laws that preceded its discovery. Even though the following laws were developed before the ideal gas law, it is conceptually helpful to think of them as special cases of the more general ideal gas law.
Avogadro’s Principle
One important discovery that preceded Clapeyron’s formulation of the ideal gas law was Avogadro’s principle, which states that all gases at a constant temperature and pressure occupy volumes that are directly proportional to the number of moles of gas present. Equal amounts of all gases at the same temperature and pressure will occupy equal volumes. As discussed above, one mole of any gas, irrespective of its chemical identity, will occupy 22.4 liters at STP.
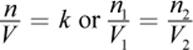
Equation 8.4
where k is a constant, n1 and n2 are the number of moles of gas 1 and gas 2, respectively, and V1 and V2 are the volumes of the gases, respectively. This can be summarized in the following statement: as the number of moles of gas increases, the volume increases in direct proportion.
Example:
A 2.0 L sample at 100°C and 20 atm contains 5 moles of a gas. If an additional 25 moles of gas at the same pressure and temperature are added, what is the final volume of the gas?
Solution:
If pressure and temperature are held constant, the ideal gas law reduces to Avogadro’s principle:
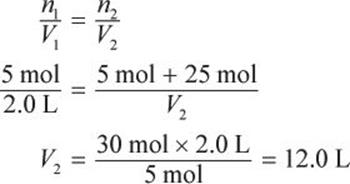
Boyle’s Law
Robert Boyle conducted a series of experimental studies in 1660 that led to his formulation of a law that now bears his name: Boyle’s law. His work showed that, for a given gaseous sample held at constant temperature (isothermal conditions), the volume of the gas is inversely proportional to its pressure:
PV = k or P1V1 = P2V2
Equation 8.5
where k is a constant, and the subscripts 1 and 2 represent two different sets of pressure and volume conditions. Careful examination of Boyle’s law shows that it is, indeed, simply the special case of the ideal gas law in which n and T are constant.
KEY CONCEPT
Boyle’s law is a derivation of the ideal gas law and states that pressure and volume are inversely related: when one increases, the other decreases.
A plot of volume vs. pressure for a gas—the inverse of the curves in Figure 8.2—is shown in Figure 8.3.
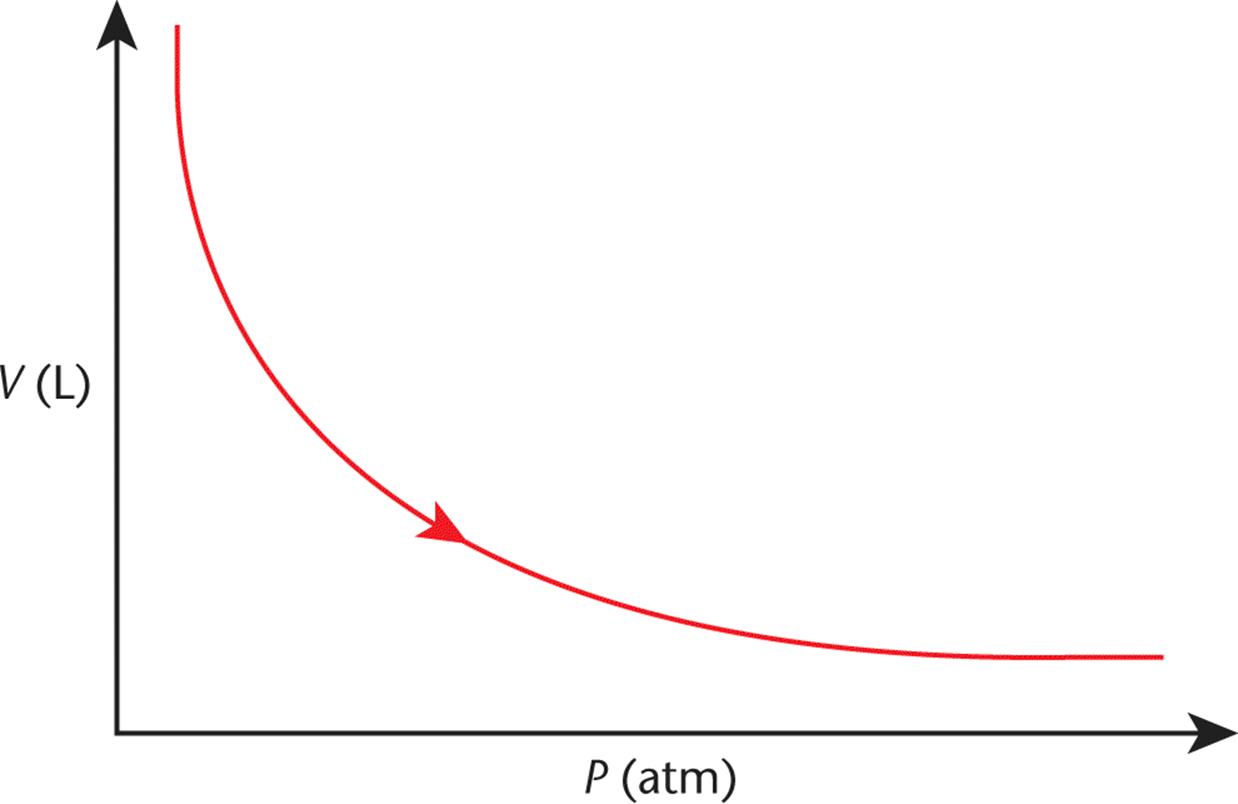Figure 8.3. Boyle’s Law (Isothermal Compression) As pressure increases, volume decreases.
MCAT EXPERTISE
Sometimes it is easier to remember the shape of the graph to help you recall the variables’ relationship on Test Day. Here we can see that, as pressure increases, the volume decreases, and vice-versa. These ratios and relationships will often allow you to answer questions on the MCAT without having to do any math.
Example:
What would be the volume of a 1 L sample of helium if its pressure is changed from 12 atm to 4 atm under isothermal conditions?
Solution:
If the number of moles of gas and temperature are held constant, the ideal gas law reduces to Boyle’s law:
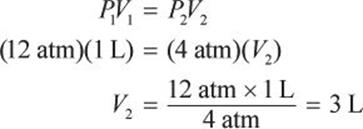
Charles’s Law
In the early 19th century, Joseph Louis Gay-Lussac published findings based, in part, on earlier unpublished work by Jacques Charles; hence, the law of Charles and Gay-Lussac is more commonly known simply as Charles’s law. The law states that, at constant pressure, the volume of a gas is proportional to its absolute temperature, expressed in kelvin. Expressed mathematically, Charles’s law is
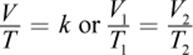
Equation 8.6
where, again, k is a proportionality constant and the subscripts 1 and 2 represent two different sets of temperature and volume conditions. Careful examination of Charles’s law shows that it is another special case of the ideal gas law in which n and P are constant.
KEY CONCEPT
Charles’s law is also a derivation of the ideal gas law and states that volume and temperature are directly proportional: when one increases, the other increases in direct proportion.
A plot of temperature vs. volume is shown in Figure 8.4. Note that if one extrapolates the V vs. T plot for a gas back to where T = 0 (absolute zero), we find that V = 0!
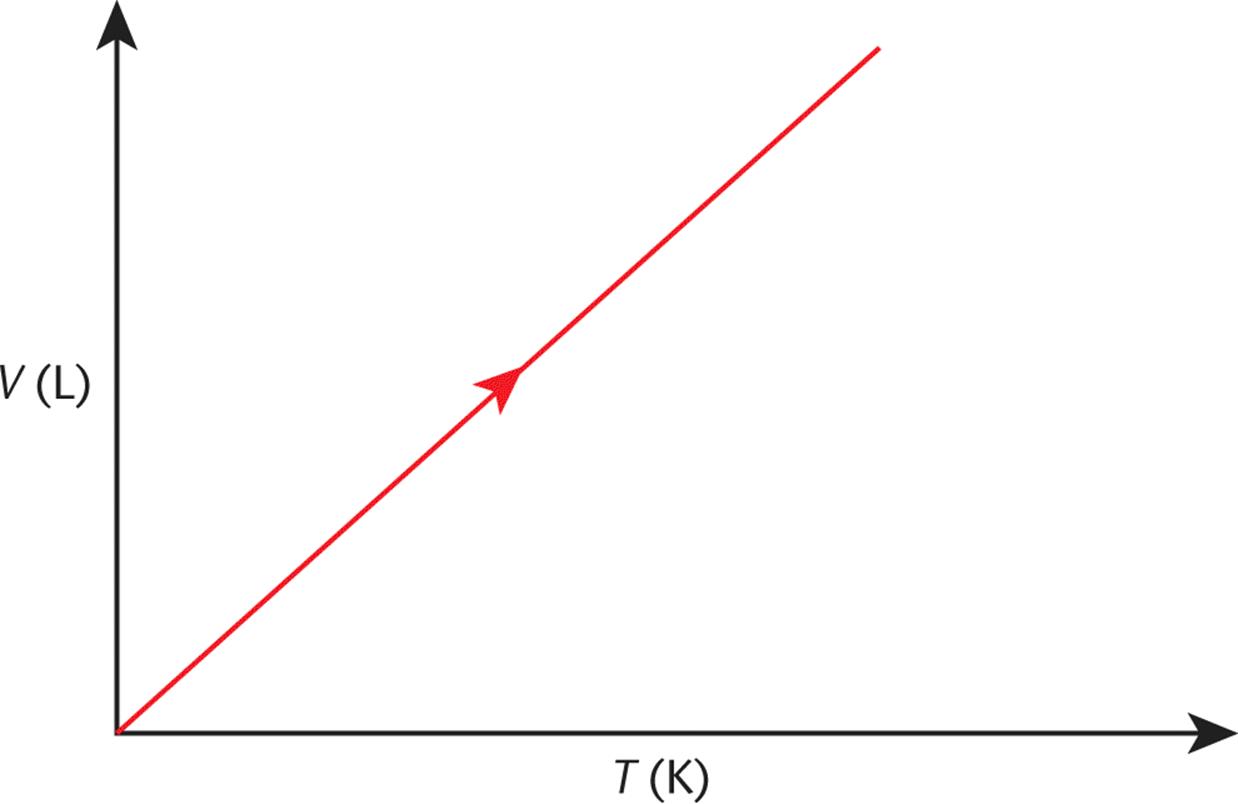Figure 8.4. Charles’s Law (Isobaric Expansion) As temperature increases, volume increases.
REAL WORLD
While the temperature of 0 K cannot be physically attained, curves such as Charles’s law were originally used to figure out its value.
Example:
If the temperature of 2 L of gas at constant pressure is changed from 283 K to 566 K, what would be its final volume?
Solution:
If the number of moles of gas and pressure are held constant, the ideal gas law reduces to Charles’s law:
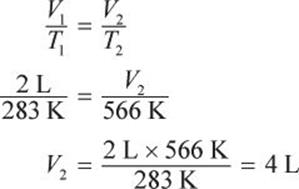
Gay-Lussac’s Law
Gay-Lussac’s law is complementary to Charles’s Law. It utilizes the same derivation from the ideal gas law, but it relates pressure to temperature instead. Expressed mathematically, Gay-Lussac’s law is
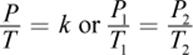
Equation 8.7
where, again, k is a proportionality constant, and the subscripts 1 and 2 represent two different sets of temperature and pressure conditions. Careful examination of Gay-Lussac’s law shows that it is another special case of the ideal gas law in which n and V are constant.
Figure 8.5 graphs this concept, which is nearly identical to Charles’s law. Again, an increase in temperature will increase the pressure in direct proportion.
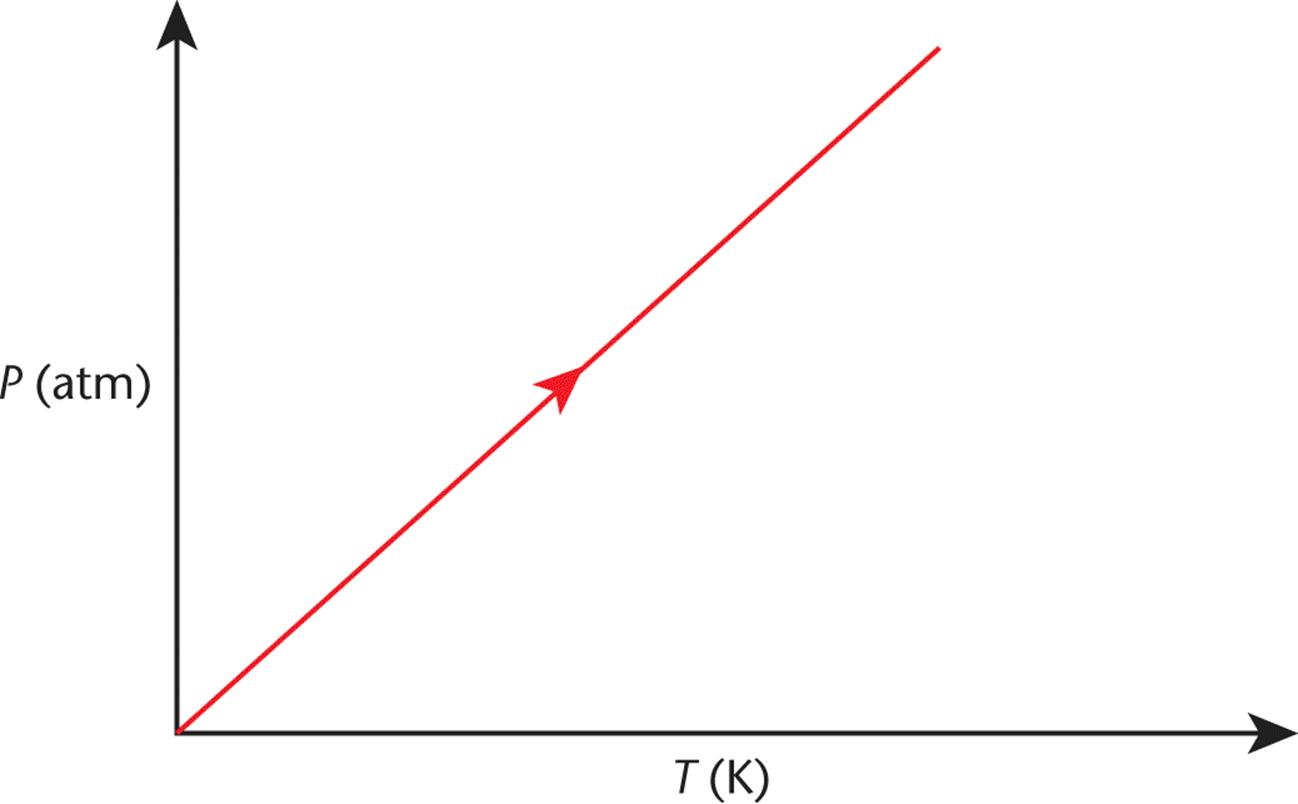Figure 8.5. Gay-Lussac’s Law (Isovolumetric Heating) As temperature increases, pressure increases.
Example:
If the pressure of a sample of gas with a temperature of 273 K changes from 2 atm to 5 atm during heating, what would be the final temperature if volume is held constant?
Solution:
If the number of moles of gas and volume are held constant, the ideal gas law reduces to Gay-Lussac’s law:
Combined Gas Law
As discussed earlier, the combined gas law (Equation 8.3) was a combination of many of the preceding laws. This law relates pressure and volume (Boyle’s law) in the numerator, and relates the variations in temperature to both volume (Charles’s law) and pressure (Gay-Lussac’s law) simultaneously. When using this equation, take care to place all of the variables in the right place.
MCAT EXPERTISE
Understanding how the combined gas law functions helps avoid the need to memorize every other special case of the ideal gas law. Read the question stem or passage with an eye toward the quantities that remain constant to know when assumptions can be made.
DALTON’S LAW OF PARTIAL PRESSURES
When two or more gases that do not chemically interact are found in one vessel, each gas will behave independently of the others. That is, each gas will behave as if it were the only gas in the container. Therefore, the pressure exerted by each gas in the mixture will be equal to the pressure that the gas would exert if it were the only one in the container. The pressure exerted by each individual gas is called the partial pressure of that gas. In 1801, John Dalton derived an expression, now known as Dalton’s law of partial pressures, which states that the total pressure of a gaseous mixture is equal to the sum of the partial pressures of the individual components. The equation for Dalton’s law is
PT = PA + PB + PC + …
Equation 8.8
where PT is the total pressure in the container, and PA, PB, and PC are the partial pressures of gases A, B, and C, respectively.
KEY CONCEPT
When more than one gas is in a container, each contributes to the whole as if it were the only gas present. Add up all of the pressures of the individual gases and you get the whole pressure of the system.
The partial pressure of a gas is related to its mole fraction and can be determined using the following equation:
Equation 8.9
Example:
A vessel contains 0.75 mol of nitrogen, 0.20 mol of hydrogen, and 0.05 mol of fluorine at a total pressure of 2.5 atm. What is the partial pressure of each gas?
Solution:
First calculate the mole fraction of each gas.
Then calculate the partial pressure.
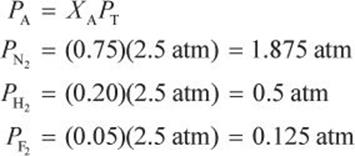
HENRY’S LAW
The difference in gas solubility between fluids was explained by William Henry in 1803. What Henry noticed was that, at various applied pressures, the concentration of a gas in a liquid increased or decreased. This was a characteristic of a gas’s vapor pressure. Vapor pressure is the pressure exerted by evaporated particles above the surface of a liquid. Evaporation, as discussed in Chapter 7 of MCAT General Chemistry Review, is a dynamic process that requires the molecules at the surface of a liquid to gain enough energy to escape into the gas phase.
Vapor pressure from the evaporated molecules forces some of the gas back into the liquid phase, and equilibrium is reached between evaporation and condensation. Mathematically, this is expressed as
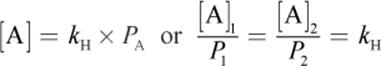
Equation 8.10
KEY CONCEPT
The solubility of a gas will increase with increasing partial pressure of the gas.
where [A] is the concentration of A in solution, kH is Henry’s constant, and PA is the partial pressure of A. The value of Henry’s constant depends on the identity of the gas.
According to this relationship, solubility (concentration) and pressure are directly related. In biology, this is a critically important relationship for gas and nutrient exchange. As discussed in Chapter 6 of MCAT Biology Review, lung tissue—at the microscopic level—is organized into grapelike clusters of sacs called alveoli. These sacs are perfused by capillaries that allow for the exchange of carbon dioxide and oxygen, as shown in Figure 8.6. If the atmospheric pressure changes, as it does from sea level to high altitude, then the partial pressure of oxygen in the atmosphere also changes (as explained by Dalton’s law), and the amount of gas exchanged is altered accordingly; if the partial pressure of a particular gas is elevated, such as when giving hyperbaric oxygen, the amount of that gas dissolved in the blood is also elevated.
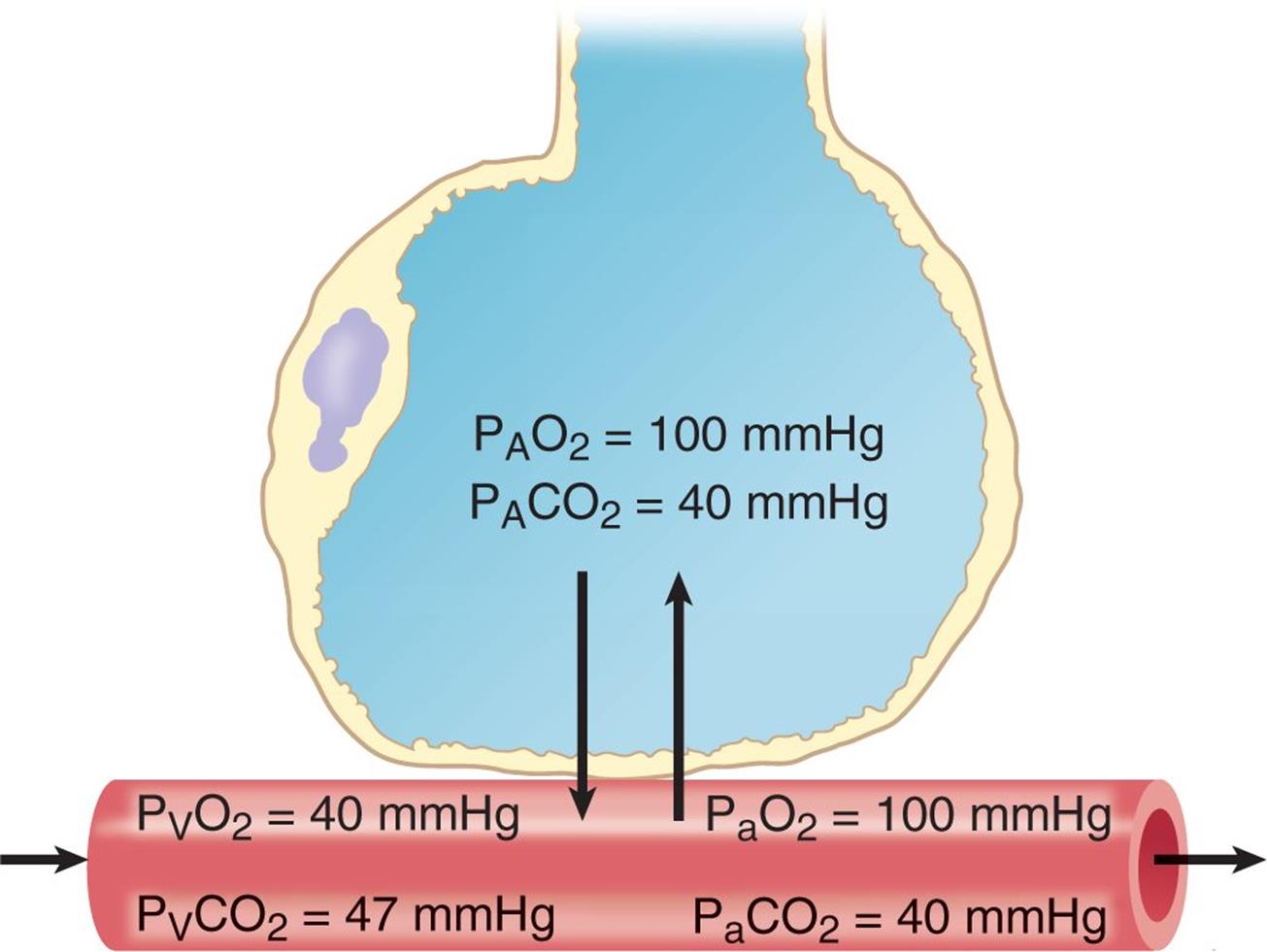Figure 8.6. Alveolar Capillary Gas Exchange In medicine, A represents alveolar concentrations, V represents venous concentrations, and a represents arterial concentrations.
Example:
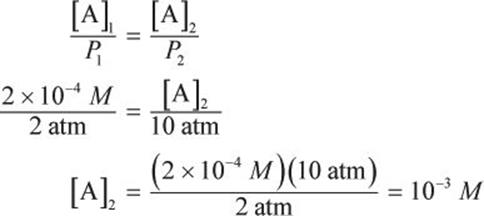
If 4 × 10−4 moles of gas are dissolved in 2 L of solution under an ambient pressure of 2 atm, what will be the molar concentration of the gas under 10 atm?
Solution:
Start by determining the initial concentration of the gas in solution.
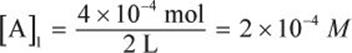
Next, utilize the direct relationship between solubility and pressure according to Henry’s law.
MCAT Concept Check 8.2:
Before you move on, assess your understanding of the material with these questions.
1. A container with 4 moles of a gas at a pressure of 6 atm has a volume of 12 liters. What is its temperature? (Note:
)
2. What is the density of argon gas at 4 atm and 136.5°C?
3. A 20 L sample at 300°C and 5 atm of pressure contains 2 moles of a gas. If an additional 3 moles of gas at the same pressure and temperature are added, what is the final total volume of the gas?
4. What would be the volume of a 2 L sample of neon if its pressure is changed from 1 atm to 40 atm under isothermal conditions?
5. If the temperature of 7.5 L of gas at constant pressure is changed from –136.5°C to 136.5°C, what would be its final volume?
6. If the pressure of a sample of gas with temperature of 273°C is changed from 5 atm to 2 atm during cooling, what would be the final temperature?
7. A vessel contains 8 mol O2, 3 mol CH4, and 1 mol CO2 at a total pressure of 240 atm. What is the partial pressure of each gas?
8. How can the concentration of carbon dioxide in sodas or other carbonated beverages can be so much higher than that of atmospheric carbon dioxide?