Master AP Calculus AB & BC
Part II. AP CALCULUS AB & BC REVIEW
CHAPTER 3. Limits and Continuity
OVERVIEW
• Hands-On Activity 3.1: What is a limit?
• Evaluating limits analytically
• Continuity
• Hands-On Activity 3.2: The extreme value theorem
• Hands-On Activity 3.3: The intermediate value theorem
• Limits involving infinity
• Special limits
• Technology: Evaluating limits with a graphing calculator
• Summing it up
The concepts of limits stymied mathematicians for a long, long time. In fact, the discovery of calculus hinged on these wily little creatures. Limits allow us to do otherwise illegal things like divide by zero. Since, technically, it is never acceptable to divide by zero, limits allow uptight math people to say that they are dividing by “basically” zero or “essentially” zero. Limits are like fortune tellers—they know where you are heading, even though you may not ever get there. Unlike fortune tellers, however, the advice of limits is always free, and limits never have bizarre names like “Madame Vinchense.”
HANDS-ON ACTIVITY 3.1: WHAT IS A LIMIT?
By completing this activity, you will discover what a limit is, when it exists, and when it doesn’t exist. As in previous Hands-On Activities, spend quality time trying to answer the questions before you break down and look up the answers.
1. Let ![]() What is the domain of f(x)? Graph f(x).
What is the domain of f(x)? Graph f(x).
2. The table below gives x-values that are less than but increasingly closer and closer to —1. These values are said to be approaching —1 from the left. Use your calculator to fill in the missing values of f(x) for each x.

TIP. Limits can help you understand the behavior of points not in the domain of a function, like the value you described in Number 1.
3. The y-value (or height) you are approaching as you near the x value of —1 in the table above is called the left-hand limit of — 1 and is written ![]() What is the left-hand limit of f(x)?
What is the left-hand limit of f(x)?
_____________________________________________________
4. The table below gives x-values that are greater than but increasingly closer to —1. These values are approaching —1 from the right. Use your calculator to fill in the missing values, as you did in Number 2.

5. The y-value (or height) you are approaching as you near the x value of —1 in the table above is called the right-hand limit of —1 and is written ![]() What is the right-hand limit of f(x)?
What is the right-hand limit of f(x)?
_____________________________________________________
ALERT! Just because the general limit may exist at one x value, that does not guarantee that it exists for all x in the function!
6. Graph f(x) below, and draw the left- and right-hand limits as arrows on the graph.
7. When the left- and right-hand limits as x approaches —1 both exist and are equal, the general limit at x = —1 exists and is written ![]() Does the general limit exist at x = —1? If so, what is it?
Does the general limit exist at x = —1? If so, what is it?
8. Write a few sentences describing what a limit is and how it is found.
_____________________________________________________
_____________________________________________________
_____________________________________________________
9. Each of the following graphs has no limit at the indicated point. Use a graphing calculator and your knowledge of limits to determine why the limits do not exist.

10. Complete this statement: A limit does not exist if...
A. _____________________________________________________
B. _____________________________________________________
C. _____________________________________________________
SELECTED SOLUTIONS TO HANDS-ON ACTIVITY 3.1
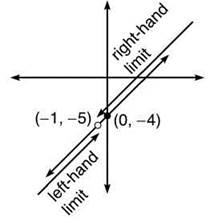
1. The domain is (—∞,—1) u (—1,∞), or all real numbers excluding x = —1, as this makes the denominator zero and the fraction undefined. The graph looks like y = x — 4 with a hole at point (—1, —5).
3. The graph seems to be heading toward a height of —5, so that is the left-hand limit.
5. The right-hand limit also appears to be —5.
6. The graph is identical toy — x — 4, except/(x) is undefined at the point (-1,-5). Even though the function is undefined there, the graph is still “headed” toward the height (or limit) of —5 from the left and right of the point.
7. The general limit does exist at x = —1, and ![]()
8. A general limit exists on f(x) at x = c (c is a constant) if the left- and right-hand limits exist and are equal at x = c. Mathematically, ![]() if and only if
if and only if ![]()
9. (a) ![]() Because
Because ![]() the general limit
the general limit ![]() does not exist, according to your conclusion from problem 8.
does not exist, according to your conclusion from problem 8.
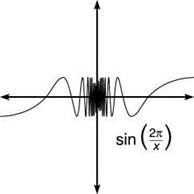
(b) As you get closer to x = 0 from the left or the right, the function does not approach any one height—it oscillates infinitely between heights, as demonstrated in its graph on the following page.
(c) As you approach x = 0 from the left or the right, the function grows infinitely large, never reaching any specified height. Functions that increase or decrease without bound, such as this one, have no general limit.
EXERCISE 1
Directions: Solve each of the following problems. Decide which is the best of the choices given and indicate your responses in the book.
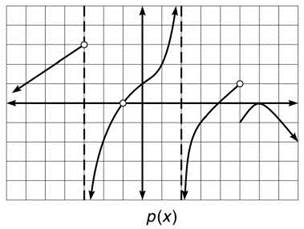
For problems 1 through 6, determine if the following limits exist, based on the graph below of p(x). If the limits do exist, state them.
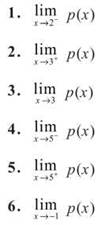
ALERT! You can write ![]() Technically, however, a limit of ∞ meanst here is no limit, since a limit must be a real number.
Technically, however, a limit of ∞ meanst here is no limit, since a limit must be a real number.
For problems 7 through 9, evaluate (if possible) the given limits, based on the graphs below of f(x) and g(x).
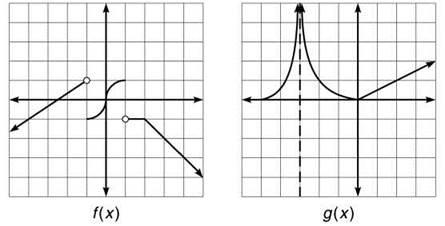
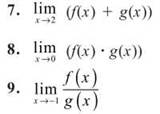
ANSWERS AND EXPLANATIONS
1. This limit does not exist, as p increases without bound as x→2+. You can also say ![]() which means there is no limit.
which means there is no limit.
2. You approach a height of —1. In this instance, ![]()
3. As stated in Number 2, the general limit exists at x = 3 and is equal to —1 (since the left- and right-hand limits are equal to —1).
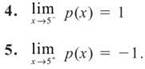 Notice that
Notice that ![]() cannot exist.
cannot exist.
![]() Thus, the general limit,
Thus, the general limit, ![]() Even though the function is undefined at x = —1, p is still headed for a height of 0, and that’s what’s important.
Even though the function is undefined at x = —1, p is still headed for a height of 0, and that’s what’s important.
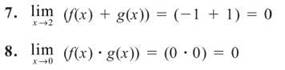
9. Although ![]() appears to be approximately 1/4,
appears to be approximately 1/4, ![]() does not exist. Therefore,
does not exist. Therefore, ![]() cannot exist.
cannot exist.