Master AP Calculus AB & BC

Part II. AP CALCULUS AB & BC REVIEW

CHAPTER 3. Limits and Continuity

LIMITS INVOLVING INFINITY

You can learn a lot about a function from its asymptotes, so it’s important that you can determine what kind of asymptotes shape a graph just by looking at a function. Remember that asymptotes are lines that a graph approaches but never reaches, as the graph stretches out forever. The two kinds of asymptotes with which you should concern yourself are vertical asymptotes and horizontal asymptotes; in the graph below of g(x), x = —2 is a vertical asymptote and y = 4 is a horizontal asymptote.

Some students are confused by this diagram, since g(x) actually intersects the horizontal asymptote. “I thought a graph can’t hit an asymptote,” they mutter, eyes filling with tears. A graph can intersect its asymptote, as long as it doesn’t make a habit of it. Even though g intersects y = 4 at (2,4), g only gets closer and closer toy = 4 after that (for x > 2), and g won’t intersect the line out there near infinity—it’s the infinite behavior of the function that concerns us. It’s the same with the criminal justice system—if you cross paths with the law a couple of times when you’re very young, it’s not that big a deal, but as you get much older, the police tend to frown upon your crossing them again.

Vertical asymptotes are discontinuities that force a function to increase or decrease without bound to avoid an x value. For example, consider the graph of

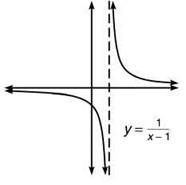
In this case, the function has an infinite discontinuity at x = 1. As you approach x = 1 from the left, the function decreases without bound, and from the right, you increase without bound. In general, if f(x) has a vertical asymptote at x = c, then or —∞. This is commonly called an infinite limit. This terminology is slightly confusing, because when/has an infinite limit at c, f has no limit at c!

Example 7: Determine which discontinuities of are caused by vertical asymptotes.

Solution: To begin, factor p(x) to get Because the denominator of a fraction cannot equal zero, p is discontinuous at x = —2 and —3. However, using the factoring method, exists and equals Therefore, the discontinuity at x = —2 is removable and not a vertical asymptote; however, x = —3 is a vertical asymptote. If you substitute x = —3 into the p, you will get —1/0. Remember, a constant divided by zero is the fingerprint of a vertical asymptote.

Horizontal asymptotes (or limits at infinity) are limiting heights that a graph approaches as x gets infinitely large or small. Consider the graph below of

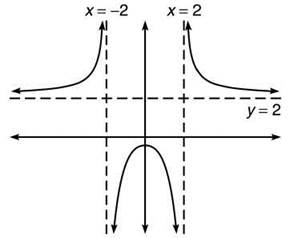
As x gets infinitely large (the extreme right side of the graph), the function is approaching a height of 2; in fact, the same is true as x gets infinitely negative (the left side of the graph). In this case, we write The AP Calculus test often features problems of the type and there is a handy trick to finding these limits at infinity of rational functions. We’ll begin with a generic example to learn the technique.

TIP. A rational function will always approach the same limit as x→∞ and x→—∞.

Example 8: Evaluate

Solution: Let A be the degree of f(x) and B be the degree of g(x).

• If A > B, then the limit is ∞.

• If B > A, then the limit is 0.

• If A = B, then the limit is the ratio of the leading coefficients of f(x) and g(x).

This technique only works for rational functions when you are finding the limit as x approaches infinity, and although it may sound tricky at first, the method is quite easy in practice.

NOTE. Remember that the degree of a polynomial is its highest exponent, and the coefficient of the term with the highest exponent is called the leading coefficient.

Example 9: Evaluate the following limits.

Because the degree of the numerator is greater than the degree of the denominator (4 > 2), the limit is ∞. In other words, the function does not approach a limiting height and will reach higher and higher as x increases.

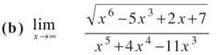
The degree of the numerator is 3, since and the degree of the denominator is 5. Since the denominator’s degree is higher, the limit is 0.

The degrees of the numerator and denominator are both 4, so take the ratio of those terms’ coefficients to get a limit of —7/5.

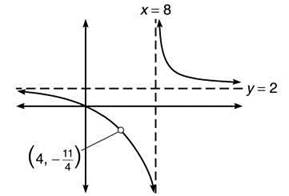
Example 10: Give the equations of the vertical and horizontal asymptotes of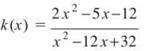

NOTE. Remember: If limit equals ∞, there technically is no limit, since ∞ is not a real number.

Solution: The horizontal asymptotes are easy to find using the technique of the previous two examples. The degrees of the numerator and denominator are equal, so Therefore, k has horizontal asymptote y = 2. In order to find any vertical asymptotes, begin by factoring k:

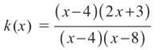
From this, you can see that k has discontinuities at x = 4 and 8. To determine which represents an asymptote, substitute them both into k. k(4) = 0/0 and k(8) = 76/0. Thus, x = 8 is an infinite discontinuity, and x = 4 is a point discontinuity, as verified by the graph.

EXERCISE 6

Directions: Solve each of the following problems. Decide which is the best of the choices given and indicate your responses in the book.

1. Explain how horizontal and vertical asymptotes are related to infinity.

2. If m is an even function with vertical asymptote x = 2 and draw a possible graph of m(x).

3. Evaluate:

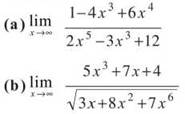
4. Given 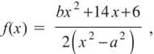a and b are positive integers, f has horizontal asymptote y = 2, and f has vertical asymptote x = 3:

(a) Find the correct values of a and b.

(b) Find the point of removable discontinuity on f.

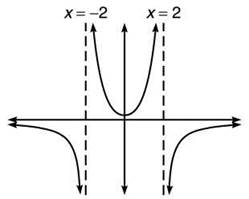
5. Draw a function g(x) that satisfies all of the following properties:

• Domain of g is (—∞,—2) ∪ (—2,—∞)

• g has a nonremovable discontinuity at x = —2

• Range of g is [—3,∞)

• g has one root: x = —4

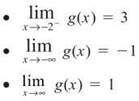
ANSWERS AND EXPLANATIONS

1. When a function approaches the vertical asymptote x = c, the function values either increase or decrease without bound (infinitely); for example: A horizontal asymptote occurs when a function approaches a fixed height forever as x approaches infinity (for example: ).

2. Because m is even, it must be y-symmetric, and, therefore, have a vertical asymptote at x = —2 as well. Furthermore, must also equal 0. Any solution must fit those characteristics; here is one:

3. (a) Because the degree of the denominator is greater than the degree of the numerator, the limit at infinity is zero.

(b) The numerator and denominator are both of degree 3 (since ), so take the corresponding coefficients to find the limit of . Note that the radical remains around the 7.

4. (a) If / has vertical asymptote x = 3, we can find a. Remember the vertical asymptote fingerprint: a zero in the denominator but not in the numerator. The denominator equals zero when 3² — a²= 0.

3² - a² = 0

a² = 9

a = 3 (since a has to be positive according to the problem)

Substitute a into the fraction to give you

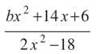
If f has horizontal asymptote y = 2, then The numerator and denominator have the same degree, so the limit is b/2 = 2. Thus, b = 4.

(b) Substituting both b and a gives you

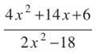
Factor completely to get

The (x + 3) factor can be eliminated, meaning that Since x = —3 is a discontinuity at which a limit exists, f is removably discontinuous at the point (—3,5/6).

5. This problem is pretty involved, and all solutions will look similar to the graph below.

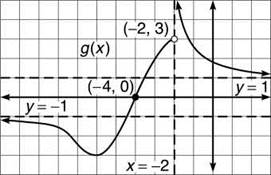
In order to get the range of [—3,∞), it’s important that The graph must also reach down to and include the height of —3, although it need not happen at (—6, —3) as on this graph.