Calculus AB and Calculus BC
CHAPTER 4 Applications of Differential Calculus
L. TANGENT-LINE APPROXIMATIONS
Local linear approximation
If f ′(a) exists, then the local linear approximation of f(x) at a is
f (a) + f ′(a)(x − a).
Since the equation of the tangent line to y = f (x) at x = a is
y − f (a) = f ′(a)(x − a),
we see that the y value on the tangent line is an approximation for the actual or true value of f. Local linear approximation is therefore also called tangent-line approximation.† For values of x close to a, we have
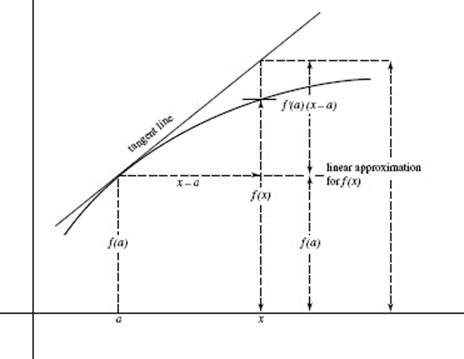
FIGURE N4–20
where f (a) + f ′(a)(x − a) is the linear or tangent-line approximation for f (x), and f ′(a)(x − a) is the approximate change in f as we move along the curve from a to x. See Figure N4–20.
In general, the closer x is to a, the better the approximation is to f (x).
EXAMPLE 30
Find tangent-line approximations for each of the following functions at the values indicated:
(a) sin x at a = 0 (b) cos x at a = 
(c) 2x3 − 3x at a = 1 (d) 
at a = 8
SOLUTIONS:
(a) At a = 0, sin x 
sin (0) + cos (0)(x − 0) 0 + 1 · x x
(b) 
(c) At a = 1, 2x3 − 3x − 1 + 3(x − 1) 3x − 4
(d) 
† Local linear approximation is also referred to as “local linearization” or even “best linear approximation” (the latter because it is better than any other linear approximation).
EXAMPLE 31
Using the tangent lines obtained in Example 30 and a calculator, we evaluate each function, then its linear approximation, at the indicated x-values:
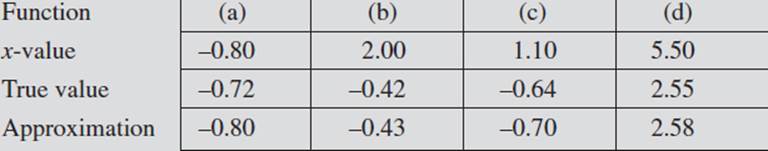
Example 31 shows how small the errors can be when tangent lines are used for approximations and x is near a.
EXAMPLE 32
A very useful and important local linearization enables us to approximate (1 + x) k by 1 + kx for k any real number and for x near 0. Equation (1) yields
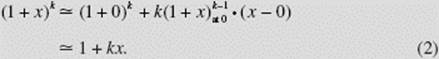
Then, near 0, for example,
EXAMPLE 33
Estimate the value of 
at x = 0.05.
SOLUTION: Use the line tangent to 
at x = 0; f (0) = 3.
so f ′(0) = 6 ; hence, the line is y = 6x + 3.
Our tangent-line approximation, then, is 
At x = 0.05, we have f (0.05) ≈ 6(0.05) + 3 = 3.3.
The true value, to three decimal places, of 
when x = 0.05 is 3.324; the tangent-line approximation yields 3.3. This tells us that the curve is concave up, lying above the tangent line to the curve near x = 0. Graph the curve and the tangent line on [−1, 1] × [−1, 6] to verify these statements.
Approximating the Change in a Function.
Equation (1) above for a local linear approximation also tells us by about how much f changes when we move along the curve from a to x: it is the quantity f ′(a)(x − a). (See Figure N4–20.)
EXAMPLE 34
By approximately how much does the area of a circle change when the radius increases from 3 to 3.01 inches?
SOLUTION: We use the formula A = πr2. Then Equation (1) tells us that the local linear approximation for A(r), when A is near 3, is
A(3) + A ′(3)(r − 3).
Here we want only the change in area; that is,
A ′(3)(r − 3) when r = 3.01.
Since A ′(r) = 2πr, therefore A ′(3) = 6π; also, (r − 3) = 0.01, so the approximate change is (6π)(0.01) 0.1885 in.2 The true increase in area, to four decimal places, is 0.1888 in.2
EXAMPLE 35
Suppose the diameter of a cylinder is 8 centimeters. If its circumference is increased by 2 centimeters, how much larger, approximately, are
(a) the diameter, and
(b) the area of a cross section?
SOLUTIONS:
(a) Let D and C be respectively the diameter and circumference of the cylinder. Here, D plays the role of f, and C that of x, in the linear approximation equation (1) a previous page. The approximate increase in diameter, when C = 8π, is therefore equal to D ′(C) times (the change in C). Since C = πD, 
and 
(which is constant for all C). The change in C is given as 2 cm; so the increase in diameter is equal approximately to 
2. 0.6366 cm.
(b) The approximate increase in the area of a (circular) cross section is equal to
A ′(C) · (change in C),
where the area 
Therefore,
Since the change in C is 2 cm, the area of a cross section increases by approximately 4 · 2 = 8 cm2.