Basic Math and Pre-Algebra
PART 3. The Shape of the World
CHAPTER 14. Quadrilaterals and Other Polygons
Polygons with More than Four Sides
Although triangles and quadrilaterals are the polygons you meet most often and the ones about which you have the most information, there are others. The other polygons have more sides, more vertices, more angles, and more diagonals, and there are a few rules they all follow. Even those rules will depend on the number of sides, but once you know that, you can figure out some things.
Remember that the name of a polygon is determined by how many sides it has. When there isn’t a particular name for a polygon with that number of sides, you just tack —gon on to the number of sides. A polygon with seventeen sides would be a seventeen-gon.
Naming Polygons by Sides
|
Sides |
5 |
6 |
7 |
8 |
9 |
10 |
|
Name |
Pentagon |
Hexagon |
Heptagon |
Octagon |
Nonagon |
Decagon |
Number of Diagonals
The more sides a polygon has, the more vertices it has, so the more diagonals you can draw. A triangle with three vertices has no diagonals, but a quadrilateral with four vertices has two diagonals. How many are there in a pentagon? Or a hexagon?
Let’s start with a pentagon, with five sides and five vertices. You can start a diagonal from any one of the five vertices, but once you pick the starting point, you have four vertices left. Of those four, you can only draw diagonals to two others. If you try to draw to the other two, you’ll be tracing over a side. So you have five places to start, but you can’t end where you started, and you can’t trace over a side, so you have only two places to end. You can draw two diagonals from that vertex, and there are five vertices, so there are 10 diagonals in a pentagon, right?
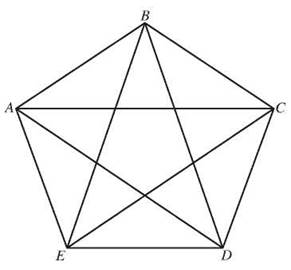
Not right. If your vertices were A, B, C, D, and E, the diagonal from C to A is the same diagonal you drew from A to C. In fact, there are only five diagonals in a pentagon because of that duplication.
The hexagon has six starting places and three ending places, but again you have to divide by two because of the duplicates. Six times three is 18, divided by two is nine diagonals in a hexagon. If a polygon has n sides, it will have ![]() diagonals.
diagonals.
Sum of the Angles
The sum of the measures of the three angles of a triangle is 180°. A quadrilateral can be split into two triangles by drawing one diagonal, and you can see that the total of the measurements of the four angles in a quadrilateral is 360°. What about polygons with more sides?

If you draw all the diagonals from one vertex, you break the polygon up into triangles. In this pentagon, drawing all the possible diagonals from one vertex divides the pentagon into three triangles. The angles of the pentagon are split up, but adding up the angles of all the triangles will make up the angles of the pentagon. The triangles each have a total of 180°, so the total for the three triangles is 540°. The total of the five interior angles of the pentagon is 540°.
The total number of degrees in the interior angles of a polygon with n sides is 180° times the number of triangles you create by drawing the diagonals from one vertex. The number of triangles is two less than the number of sides, so the total number of degrees is 180(n - 2).
CHECK POINT
21. Find the number of diagonals in an octagon (8 sides).
22. Find the total of the measures of all the interior angles in a nonagon (9 sides).
23. If a hexagon is regular, find the measure of any one of its interior angles.
24. If the interior angles of a polygon add up to 900°, how many sides does the polygon have?
25. If a polygon has a total of 119 possible diagonals, how many sides does it have?
Area of Regular Polygons
When it comes to the area of polygons with more than four sides, there aren’t a lot of rules you can follow. In most cases, you have to find a way to break the polygon up into smaller polygons, maybe triangles, find the area of those and add them all up. It’s something, but most times you won’t have the information you need to find all those areas.
There is one situation where you can follow a rule, and that’s when you’re looking for the area of a regular polygon. A regular polygon is one that is equilateral and equiangular. It has sides that are all the same length and angles that are all the same size.
DEFINITION
A polygon is regular if all sides are the same length and all angles are congruent.
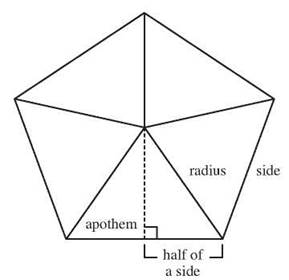
A regular polygon has a center point, and the radius, or distance from the center to a vertex, is the same no matter what vertex you go to. If you draw all the radii, you break the polygon into congruent triangles, one for each side of the polygon. If you can find the area of one of those triangles, you can multiply by the number of sides to find the area of the polygon.
Each of the triangles is isosceles, because all the radii are equal. The altitude from the center vertex to a side of the polygon is called the apothem, and it’s a super segment that is a median and angle bisector as well as an altitude. That means that if you know the length of a side and the radius, you can find the length of the apothem. Or if you know a side and the apothem, you can find the radius. Or if you know the radius and the apothem, you can find the side. How? The Pythagorean theorem, a2 + b2 = c2. When you draw the radii and an apothem, you create a right triangle whose legs are the apothem and half a side and whose diagonal is the radius. If you know any two of those, you can find the third.
DEFINITION
The apothem of a regular polygon is a line segment from the center of the polygon perpendicular to a side.
Suppose you have a regular pentagon with an apothem equal to 4 cm and a radius of 5 cm. You could use the Pythagorean theorem to find out that half the side is 3 cm, so the whole side is 6 cm. Once you have the measures of the apothem and a side, you can find the area of one triangle. ![]() square centimeters. There are five of those triangles, so the area of the pentagon is 5 x 12 = 60 square centimeters.
square centimeters. There are five of those triangles, so the area of the pentagon is 5 x 12 = 60 square centimeters.
The area of a regular polygon with n sides of length s and apothem a is given by the formula ![]() but since
but since ![]() and ns is the perimeter of the regular polygon, the formula is often given as
and ns is the perimeter of the regular polygon, the formula is often given as ![]() where P is the perimeter.
where P is the perimeter.
CHECK POINT
26. Find the area of a regular pentagon with sides 8 cm long and an apothem 5 cm long.
27. Find the area of an octagon with a perimeter of 40 inches and an apothem of 5 inches.
28. Find the area of a regular decagon if each of the 10 sides measures 2 meters and the apothem is 1.5 meters.
29. The perimeter of a regular hexagon is 42 inches and its area is 84 square inches. How long is its apothem?
30. A regular pentagon has an area of 1,080 square inches and an apothem of 18 inches. How long is each side?
The Least You Need to Know
• Parallelograms have both pairs of opposite sides parallel. Area formula: A = bh.
• Rhombuses are parallelograms with four equal sides. Area formula: A = the product of the diagonals divided by 2.
• Rectangles are parallelograms with four right angles. Area: A = lw. Squares are rectangles that are also rhombuses. Area formula: A = s2.
• Trapezoids are quadrilaterals with one pair of parallel sides. Isosceles trapezoids have nonparallel sides congruent and base angles congruent. Area formula: A = 1/2h multiplied by the sum of the bases.
• Regular polygons have congruent sides and congruent angles.
• Total of the interior angles of a polygon with n sides is 180(n - 2).