AP Physics C Exam

Part V

The Princeton Review AP Physics C Practice Tests

Chapter 18 Practice Test 1: Answers and Explanations

AP Physics C Answer Key Notes

Answer Key Physics C Test 1 Mechanics

1. E

2. A

3. A

4. E

5. C

6. A

7. E

8. D

9. B

10. D

11. A

12. D

13. C

14. D

15. C

16. A

17. C

18. C

19. D

20. B

21. C

22. C

23. C

24. C

25. D

26. B

27. D

28. D

29. A

30. B

31. D

32. E

33. E

34. B

35. E

Answer Key Physics C Test 1 Electricity and Magnetism

36. C

37. E

38. E

39. A

40. B

41. E

42. C

43. C

44. A

45. E

46. E

47. D

48. B

49. A

50. D

51. A

52. A

53. C

54. E

55. E

56. E

57. C

58. B

59. E

60. A

61. C

62. B

63. E

64. E

65. D

66. D

67. A

68. D

69. A

70. A

SECTION I, MECHANICS

1. E Let y denote the total distance that the rock falls and let T denote the total time of the fall. Then and , so

2. A The work-energy theorem says that the work done by friction is equal to the change in kinetic energy of the box. Therefore,

3. A Integrating a(t) gives v(t):

Since v₀ = 0, we have v(t) = t². Now integrating v(t) from t = 0 to t = 3 s gives the object’s displacement during this time interval:

4. E Angular momentum is conserved when no net external torque is applied (Item III). However, a body can experience a net nonzero torque even when the net external force is zero (which is the condition that guarantees conservation of linear momentum), so neither Item I nor Item II necessarily ensure conservation of angular momentum.

5. C The figure below shows that F_T sin θ = mg and F_T cos θ = mv²/R = mw²R:

We can eliminate θ from these equations by remembering that sin² θ + cos² θ is always equal to 1:

6. A Because the initial height of the object is comparable to the radius of the Moon, we cannot simply use mgh for its initial potential energy. Instead, we must use the more general expression U = –GMm/r, where r is the distance from the center of the Moon. Notice that since h = R, the object’s initial distance from the Moon’s center is h + R= R + R = 2R. Conservation of Energy then gives

7. E At equilibrium, the net force on the block is zero (by definition), so ky = mg, which gives y = mg/k. If m doubles, then so does y. This eliminates choices (A), (B), and (C). Since T = , T is proportional to . Therefore, if m increases by a factor of 2, then T increases by a factor of .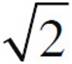

8. D Let F_T be the tension in each string. Then F_net = ma becomes mg – 2F_T = ma. Also, the total torque exerted by the tension forces on the cylinder is 2rF_T, so t_net = Ia becomes 2rF_T = (mr²)α. Because the cylinder doesn’t slip, α = a/r, so 2F_T = ma. These equations can be combined to give mg – ma = ma, which implies mg = ma. Therefore, a = g.

9. B The cylinder slides across the surface with acceleration a = F/m until time t = T, when a drops to zero (because F becomes zero). Therefore, from time t = 0 to t = T, the velocity is steadily increasing (because the acceleration is a positive constant), but, at t = T, the velocity remains constant. This is illustrated in graph (B).

10. D The speed of the object being lifted is v = (20 m)/(5 s) = 4 m/s. Since P = Fv, we find that F = P/v = 10 kW/(4 m/s) = 2.5 kN = 2500 N. Since the object is being lifted with constant velocity, the net force on it must be zero, so F must be equal to the object’s weight, mg. This gives m = F/g = 2500 N/(10 N/kg) = 250 kg.

11. A Let m and M denote the mass of the satellite and Earth, respectively. The work required to lift the satellite to height h above the surface of Earth is

Earth’s gravitational pull provides the necessary centripetal force on the satellite, so

Because we’re told that W = K, we find that

12. D First note that if L is the total length of the bar, then the distance of the block from Support 1 is L and its distance from Support 2 is L. Let P₁ denote the point at which Support 1 touches the bar. With respect to P₁, the upward force exerted by Support 1, F₁, produces no torque, but the upward force exerted by Support 2, F₂, does. Since the net torque must be zero if the system is in static equilibrium, the counterclockwise torque of F₂ must balance the total clockwise torque produced by the weight of the block and of the bar (which acts at the bar’s midpoint).

Now, with respect to P₂, the point at which Support 2 touches the bar,

These results give .

13. C Because the ball rebounded to the same height from which it was dropped, its takeoff speed from the floor must be the same as the impact speed. Calling up the positive direction, the change in linear momentum of the ball is p_f – p_i = mv – (–mv) = 2mv, where v = (this last equation comes from the equation mv² = mgh). The impulse–momentum theorem, J =p, now gives

14. D Using the Big Five equation θ = w₀t + αt², we find that

θ = (2 rad/s²)(4.0 s)² = 16 rad

Therefore, s= rθ = (0.1 m)(16 rad) = 1.6 m.

15. C The ball’s initial velocity from the slide is horizontal, so v_0y = 0, which implies that y = – gt². Since y = –R,

The (horizontal) speed with which the ball leaves the slide is found from the equation mgR = mv², which gives v = . Therefore,

Since the ball travels a horizontal distance of 2R from the end of the slide, the total horizontal distance from the ball’s starting point is R + 2R = 3R.

16. A From the diagram below,

if R cos q = –(0.5)R, then q = 240°. Therefore, y = R sin q = R sin 240° = –(0.866)R. Also, it is clear that the particle’s subsequent motion will cause the shadow on Screen 2 to continue moving in the –y direction.

17. C The center of mass of the system is at a distance of

below the mass m. With respect to this point, the clockwise torque produced by the force F has magnitude

Since the rotational inertia of the system about its center of mass is

the equation τ = Iα becomes

18. C By Newton’s Third Law, both vehicles experience the same magnitude of force and, therefore, the same impulse; so Statement I is false. Invoking Newton’s Second Law, in the form impulse = change in momentum, we see that Statement II is therefore also false. However, since the car has a smaller mass than the truck, its acceleration will be greater in magnitude than that of the truck, so Statement III is true.

19. D Since F_net = F₁ + F₂ = 0, the bar cannot accelerate translationally, so (C) is false. The net torque does not need to be zero, as the following diagram shows (eliminating choices (A) and (B)):

However, since F₂ = –F₁, choice D is true; one possible illustration of this is given below:

20. B The value of g near the surface of a planet depends on the planet’s mass and radius:

Therefore, calling this Planet X, we find that

Since g is half as much on the planet as it is on Earth, the astronaut’s weight (mg) will be half as much as well. The astronaut would weigh half of 800 N, which is 400 N or choice (B).

21. C The work done by the force F(x) is equal to the area under the given curve. The region under the graph can be broken into two triangles and a rectangle, and it is then easy to figure out that the total area is 50 N·m. Since work is equal to change in kinetic energy (and v_i = 0),

22. C Since no external torques act on the star as it collapses (just like a skater when she pulls in her arms), angular momentum, Iw, is conserved, and the star’s rotational speed increases. The moment of inertia of a sphere of mass m and radius r is given by the equation I = kmr² (with k = , but the actual value is irrelevant), so we have:

Therefore,

23. C The projectile’s horizontal speed is v₀ cos 60° = v₀ = 20 m/s, so it reaches the wall in 1.0 s.

24. C Impulse, J, is defined to be force × time, so

25. D The diagram given with the question shows that after the clay balls collide, they move in the –y direction, which means that the horizontal components of their linear momenta canceled. In other words, p_1x + p_2x = 0:

26. B The horizontal position of the blocks can be given by the equation

x = A sin(wt + ϕ₀)

where A is the amplitude, w is the angular frequency, and ϕ₀ is the initial phase. Differentiating this twice gives the acceleration:

This means that the maximum force on block m is F_max = ma_max = mAw². The static friction force must be able to provide this much force; otherwise, the block will slip. Therefore,

27. D The ball strikes the floor with speed v₁ = , and it leaves the floor with speed v₂ = . Therefore,

28. D The center of rotation is the center of mass of the plate, which is at the geometric center of the square because the plate is homogeneous. Since the line of action of the force coincides with one of the sides of the square, the lever arm of the force, ℓ, is simply equal to s. Therefore,

τ = ℓ F = sF = (0.40 m)(10 N) = 2.0 N·m

29. A Since gravity is a conservative force, the actual path taken is irrelevant. If the block rises a vertical distance of z = 3 m, then the work done by gravity is

W = –mgz = –(2.0 kg)(10 N/kg)(3 m) = –60 J

30. B The work done by the friction force as the block slides from x = A to x = 0 is

Now use Conservation of Energy, using U(x) = kx²:

31. D Consider an infinitesimal slice of width dx at position x; its mass is dm = λ dx = kx dx. Then, by definition of rotational inertia,

Because the total mass of the rod is

we see that I = ML².

32. E Points of equilibrium occur when dU/dx is equal to zero:

Points of stable equilibrium occur when dU/dx = 0 and d²U/dx² is positive (because then the equilibrium point is a relative minimum). Since

the point x = 4 is a point of stable equilibrium (x = 0 is unstable).

33. E The range of a projectile is given by the equation

and the maximum height attained by the projectile is

In order for R to equal H, it must be true that

34. B Differentiate the equation K = mv² with respect to time:

This tells us that

35. E The maximum net force on the object occurs when all three forces act in the same direction, giving F_net = 3F = 3(4 N) = 12 N, and a resulting acceleration of a = F_net/m = (12 N)/(2 kg) = 6 m/s². These three forces could not give the object an acceleration greater than this.

SECTION II, MECHANICS

1. (a) (i) Since the pulley is not accelerating, it can serve as the origin of an inertial reference frame. Applying Newton’s Second Law to the masses using the following free-body diagrams,

gives

F_T – mg = ma and Mg – F_T = Ma

Adding these equations eliminates F_T, and we find that

The acceleration of block m is (M – m)g/(M + m) upward, and the acceleration of block M is this value, (M – m)g/(M + m), downward.

(a) (ii) Substitute the value for a into either one of the equations containing F_T:

(b) (i) Because the center of the pulley is accelerating, it cannot serve as an inertial reference frame. Inertial frames are non-accelerating.

(b) (ii) Refer to the following diagram:

Since the second time derivative of displacement is acceleration,

Now since Y_m = z + y_m and Y_M = z + y_M, it is also true that

Because our positive direction is up for m and down for M, and is equal to b downward,

Therefore,

Adding these equations (and remembering that ), we find that

(b) (iii) Substitute the value for a into either one of the equations containing F_T:

(b) (iv) F_T will equal zero if b = g.

2. (a) The mass contained in a sphere of radius r < R is

(b) Since dU = –F(r) dr,

By applying the Pythagorean theorem to the two right triangles in the figure,

we see that

so the expression given above for v can be rewritten in the form

(d) (i) From expression (*) given in part (c) above, we can see that the value of y which will maximize v is

This is the midpoint of the tunnel.

(d) (ii) The maximum value for v is

3. (a) (i) Once the 3m puck sticks to the m puck, the center of mass of the system is

above the bottom pucks on the rod.

(a) (ii) Applying Conservation of Linear Momentum, we find that

(3m)v = (3m + m + 2m)v′_cm ⇒ v′_cm = v

(a) (iii) Now, applying Conservation of Angular Momentum, we find that

(b) The kinetic energy before the collision was the initial translational kinetic energy of the 3m puck:

K_i = (3m)v²

After the collision, the total kinetic energy is the sum of the system’s translational kinetic energy and its rotational kinetic energy:

Therefore, the fraction of the initial kinetic energy that is lost due to the collision is

SECTION I, ELECTRICITY AND MAGNETISM

36. C The proximity of the charged sphere will induce negative charge to move to the side of the uncharged sphere closer to the charged sphere. Since the induced negative charge is closer than the induced positive charge to the charged sphere, there will be a net electrostatic attraction between the spheres.

37. E The capacitance of a parallel-plate capacitor is C = Kε₀A/d, where K is the dielectric constant, A is the area of each plate, and d is their separation distance. Decreasing d will cause C to increase.

38. E When the particle enters the magnetic field, the magnetic force provides the centripetal force to cause the particle to execute uniform circular motion:

Since v and B are the same for all the particles, the largest r is found by maximizing the ratio m/|q|. Furthermore, since the ratio of u/e (atomic mass unit/magnitude of electric charge) is a constant, the answer depends only on the ratio of “factor of u” to “factor of charge.” The ratio 20/1 is largest, so it will have the largest r.

39. A Electric field lines are always perpendicular to the surface of a conductor, eliminating choices B and E. Excess charge on a conductor always resides on the surface (eliminating choice D), and there is a greater density of charge at points where the radius of curvature is smaller (eliminating choice C).

40. B The resistance of a wire made of a material with resistivity r and with length L and cross-sectional area A is given by the equation R = ρL/A. Since Wire B has the greatest length and smallest cross-sectional area, it has the greatest resistance.

41. E From the equation F = qvB, we see that 1 tesla is equal to 1 N·s/(C·m). Since

choices (A) and (C) are eliminated.

Furthermore,

eliminating choice (B). Finally, since

choice (D) is also eliminated.

42. C Gauss’s Law states that the total electric flux through a closed surface is equal to (1/e₀) times the net charge enclosed by the surface. Both the cube and the sphere contain the same net charge, so Φ_C must be equal to Φ_S.

43. C The electric field between the plates is uniform and equal to V/x, so the magnitude of the electric force on the charge is F_E = qE = qV/x. From the following free-body diagram

we see that

Dividing the first equation by the second one, we find that

44. A The Table of Information provides the following conversion factor: 1 e = 1.6 × 10^–19 C. Multiplying the magnitude of charge, 1 C, by this conversion factor gives the following:

There are 6.25 × 10¹⁸ electrons in 1 coulomb of negative charge.

45. E Resistors R₂, R₃, and R₄ are in parallel, so their equivalent resistance, R_2-3-4, satisfies

Since this is in series with R₁, the total resistance in the circuit is

The current provided by the source must therefore be

46. E The current through R₄ is the current through R₁, and R₄ = R₁, so

47. D Because the time constant for an RC circuit is equal to the product of resistance and capacitance, τ = RC, this product has the dimensions of time.

48. B Apply Faraday’s Law of Electromagnetic Induction:

Since r = 1 m, the value of E at t = 1 s is E = 1 N/C.

49. A The magnetic force, F_B, on the top horizontal wire of the loop must be directed upward and have magnitude mg. (The magnetic forces on the vertical portions of the wire will cancel.) By the right-hand rule, the current in the top horizontal wire must be directed to the right—because B is directed into the plane of the page—in order for F_Bto be directed upward; therefore, the current in the loop must be clockwise (eliminating choices B and D). Since F_B = IxB, we must have

50. D Construct a cylindrical Gaussian surface of radius r < R and length ℓ < L within the given cylinder. By symmetry, the electric field must be radial, so the electric flux through the lids of the Gaussian cylinder is zero. The lateral surface area of the Gaussian cylinder is 2πrℓ, so by Gauss’s Law,

51. A Call the top wire (the one carrying a current I to the right) Wire 1, and call the bottom wire (carrying a current 2I to the left) Wire 2. Then in the region between the wires, the individual magnetic field vectors due to the wires are both directed into the plane of the page, so they could not cancel in this region. Therefore, the total magnetic field could not be zero at either Point 2 or Point 3. This eliminates choices (B), (C), (D), and (E), so the answer must be (A). Since the magnetic field created by a current-carrying wire is proportional to the current and inversely proportional to the distance from the wire, the fact that Point 1 is in a region where the individual magnetic field vectors created by the wires point in opposite directions and that Point 1 is twice as far from Wire 2 as from Wire 1 implies that the total magnetic field there will be zero.

52. A If the mass of the +q charge is m, then its acceleration is

The graph in A best depicts an inverse-square relationship between a and r.

53. C Once the switch is closed, the capacitors are in parallel, which means the voltage across C₁ must equal the voltage across C₂. Since V = Q/C,

The total charge, 24 μC + 12 μC = 36 μC, must be redistributed so that Q′₂ = 2Q′₁. Therefore, we see that Q′₂ = 24 μC (and Q′₁ = 12 μC).

54. E Because v is parallel to B, the charges in the bar feel no magnetic force, so there will be no movement of charge in the bar and no motional emf.

55. E Along the line joining the two charges of an electric dipole, the individual electric field vectors point in the same direction, so they add constructively. At the point equidistant from both charges, the total electric field vector has magnitude

56. E Imagine placing equal but opposite charges on the spheres, say, +Q on the inner sphere and –Q on the outer sphere. Then the electric field between the spheres is radial and depends only on +Q, and its strength is

Therefore, the potential difference between the spheres is

Now, by definition, C = Q/V, so

57. C Since the magnetic force is always perpendicular to the object’s velocity, it does zero work on any charged particle. Zero work means zero change in kinetic energy, so the speed remains the same. Remember: The magnetic force can only change the direction of a charged particle’s velocity, not its speed.

58. B The presence of the inductor in the rightmost branch effectively removes that branch from the circuit at time t = 0 (the inductor produces a large back emf when the switch is closed and the current jumps abruptly). Initially, then, current only flows in the loop containing the resistors r and R. Since their total resistance is r + R, the initial current is ε/(r + R).

59. E After a long time, the current through the branch containing the inductor increases to its maximum value. With all three resistors in play, the total resistance is R_eq = r + , since is the resistance of the parallel branch.

The current provided by the source, , splits at the junction leading to the parallel combination. The amount which flows through the inductors is half the total.

so the energy stored in the inductor is

60. A Once the switch is opened, the resistor r is cut off from the circuit, so no current passes through it. (Current will flow around the loop containing the resistors labeled R, gradually decreasing until the energy stored in the inductor is exhausted.)

61. C If a conducting sphere contains a charge of +q within an inner cavity, a charge of –q will move to the wall of the cavity to “guard” the interior of the sphere from an electrostatic field, regardless of the size, shape, or location of the cavity. As a result, a charge of +q is left on the exterior of the sphere (and it will be uniform). So, at points outside the sphere, the sphere behaves as if this charge +q were concentrated at its center, so the electric field outside is simply kQ/r². Since points X and Y are at the same distance from the center of the sphere, the electric field strength at Y will be the same as at X.

62. B First determine the electric potential at Point P. Realize that Point P is 5 meters away from each charge because it is a 3-4-5 right triangle.

The work done by the electric field will be positive because a –Q charge would be attracted to point P by the two positive Q charges. Also the work done by the force is the negative of the change in potential energy.

63. E The current in an L–R series circuit—in which initially no current flows—increases with time t according to the equation

I(t) = I_max(1 – e^-t/t)

where I_max = ε/R and τ is the inductive time constant, L/R. The time t at which I = (99%)I_max is found as follows:

Alternatively, the solution to this question can be found by using process of elimination. Answer choice (B) can be eliminated because it does not incorporate the 99%. Answer choices (A) and (C) can be eliminated because they give negative results. Answer choice (D) can be eliminated because ln(100/99) is close to zero. This leaves only answer choice (E) satisfying the given conditions.

64. E First solve for the resistance of each light bulb as shown below:

P = V ²/R

R = V ²/P = (120 V)²/(40 W) = 360 Ω

Next solve for the equivalent resistance, R_eq, of the circuit as follows:

R_eq = V/I = (120 V)/(5 A) = 24 Ω

If you used a current less than 5A (the maximum allowable before the fuse blows), then the R_eq would increase.

Finally solve for the number of 360 Ω resistors (light bulbs) that can be placed in a parallel circuit with an equivalent resistance of 24 Ω.

1/R_eq = n/R

n = R/R_eq = (360 Ω)/(24 Ω) = 15

Thus, a maximum of 15 light bulbs can be connected in parallel with the 120 V source.

65. D Since the magnetic flux out of the page is decreasing, the induced current will oppose this change (as always), attempting to create more magnetic flux out of the page. In order to do this, the current must circulate in the counterclockwise direction (remember the right-hand rule). As B decreases uniformly (that is, while dB/dt is negative and constant), the induced emf,

is nonzero and constant, which implies that the induced current, I = ε/R, is also nonzero and constant.

66. D Treat the configuration as three capacitors in series, each with a distance between the plates of . The capacitance of the two vacuum capacitors will be , and the capacitance of the dielectric capacitor will be that value times K.

Now solve for the total capacitance for the three capacitors in series.

67. A The potential is zero at the point midway between the charges, but nowhere is the electric field equal to zero (except at infinity).

68. D Ampere’s Law states that

Because a total current of 2I + 4I = 6I passes through the interior of the loop in one direction, and a total current of I + 3I = 4I passes through in the opposite direction, the net current passing through the loop is 6I – 4I = 2I. Therefore, the absolute value of the integral of B around the loop WXYZ is equal to m₀(2I).

69. A Statement I is true: Since the line ℓ is the perpendicular bisector of the line segment joining the two charges, every point on ℓ is equidistant from the two charges. Therefore, the electric potential at any point on this line is

k(+Q)/r + k(–Q)/r = 0

so ℓ is an equipotential. By definition, then, a charge moving along ℓ would experience no change in electrical potential energy. Statement II is false: The individual field vectors created by each source charge do not point in opposite directions at any point on ℓ, so at no point on ℓ would the total electric field be zero, immediately implying that at no point on ℓ would the electrostatic force on a charge be zero. Statement III is also false: The equipotentials in the plane of the page are not ellipses with the source charges as foci. In fact, as we showed in the discussion of Statement I, the line ℓ itself is an equipotential (and it’s not an ellipse!).

70. A First, note that the distance between –q and each charge +Q is L. Now, refer to the following diagram, where we’ve invoked Coulomb’s Law to determine the two electrostatic forces:

We find that the magnitude of F is

so dividing this by m gives the initial acceleration of the charge –q.

SECTION II, ELECTRICITY AND MAGNETISM

1. (a) Consider a small arc on the ring, subtended by an angle dθ. The length of the arc is ds = R dθ, so the charge it carries is dQ = (Q/2πR)(R dθ) = (Q/2π) dθ, since λ = Q/(2πR) is the linear charge density.

The distance from this arc to an arbitrary Point P on the z-axis is (z² + R²)^1/2, so the contribution to the electric potential at P due to this arc is

Integrating this from θ = 0 to θ = 2π (i.e., all the way around the ring), we find that

(b) (i) From the expression derived in part (a), we see that V will be maximized when z = 0. That is, the point on the z-axis where the potential due to the ring is greatest is at the center of the ring.

(b) (ii) Putting z = 0 into the expression from part (a), we find that

To include the direction—and thereby find E—let’s use to denote the unit vector that defines the positive direction along the z-axis. Then

(d) The force on the positive charge is in the +z direction, so the charge will accelerate in that direction. As it moves along the z-axis, the force will decrease, so it will move with increasing speed and decreasing acceleration away from the origin.

2. (a) (i) The current decays exponentially according to the equation

where the initial current, I(0), is equal to ε/(r + R). To find the time t at which I(t) = I(0)/k, we solve the following equation:

(a) (ii) The charge on the capacitor increases according to the equation

The maximum value is Cε (when the capacitor is fully charged). To find the time t at which Q(t) = Cε/k, we solve the following equation:

(a) (iii) The field energy stored in the capacitor can be written in the form U_E(t) = [Q(t)]²/(2C), where Q(t) is the function of time given in part (ii) above. We are asked to find the time t at which

(b) (i) Once the switch is turned to position 2, the capacitor discharges through resistor R (only). Since the capacitor is fully charged (to V = ε) when the switch is moved to position 2, the current will decrease exponentially according to the equation

I(t) = I₀e^−t/RC

where I₀ = I_max = ε/R. To find the time at which I is equal to I₀/k, we solve the following equation:

(b) (ii) Since the charge on the capacitor obeys the same equation as the current—as given in (b) (i) above, simply replacing I by Q—the time t at which the charge drops to 1/k its initial value is the same as the time t at which the current drops to 1/k times its initial value: t = (ln k)RC.

3. (a) (i) As the strip XY slides down the rails, the magnetic flux through the rectangular region bounded by the strip increases. This change in magnetic flux induces an emf. If ℓ denotes the distance the strip has slid down the rails, then the magnetic flux through the rectangular region is

Φ_B = BA cos θ = BLℓ cos θ

The rate of change of Φ_B is

where v = dℓ/dt is the speed with which the strip slides. By Faraday’s Law, this is the (magnitude of the) induced emf.

(a) (ii) As the strip slides down, the area increases, so the magnetic flux upward increases. To oppose this change (in accordance with Lenz’s Law), the induced current must flow in such a way as to create some magnetic flux downward. By the right-hand rule, then, current must flow from Y to X.

(a) (iii) The induced current depends on the speed of the sliding strip and is equal to

(b) In order for the strip to slide with constant velocity, the net force on it must be zero. The force it feels parallel to the rails and downward is F_w sin θ = mg sin θ. The following diagram

which is a side view of the apparatus looking at the X end of the strip, shows that the force the strip feels parallel to the rails and upward is

The net force on the strip is zero when these two opposing forces balance; the speed at which this occurs will be denoted v_f:

where the last equality follows from the formula P = Fv for the power produced by a constant force F acting on an object whose velocity, v, is parallel to F.