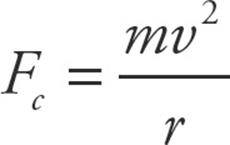AP Physics C Exam
Part IV
Content Review for the AP Physics C Exam
Chapter 5
Newton’s Laws
INTRODUCTION
In the previous chapter we studied the vocabulary and equations that describe motion. Now we will learn why things move the way they do; this is the subject of dynamics.
An interaction between two bodies—a push or a pull—is called a force. If you lift a book, you exert an upward force (created by your muscles) on it. If you pull on a rope that’s attached to a crate, you create a tension in the rope that pulls the crate. When a skydiver is falling through the air, Earth is exerting a downward pull called gravitational force, and the air exerts an upward force called air resistance. When you stand on the floor, the floor provides an upward, supporting force called the normal force. If you slide a book across a table, the table exerts a frictional force against the book, so the book slows down and then stops. Static cling provides a directly observable example of the electrostatic force. Protons and neutrons are held together in the nuclei of atoms by the strong nuclear force and radioactive nuclei decay through the action of theweak nuclear force.
The Englishman Sir Isaac Newton published a book in 1687 called Philosophiae Naturalis Principia Mathematica (The Mathematical Principles of Natural Philosophy)—referred to nowadays as simply The Principia—which began the modern study of physics as a scientific discipline. Three of the laws that Newton stated in The Principia form the basis for dynamics and are known simply as Newton’s Laws of Motion.
THE FIRST LAW
Newton’s First Law says that an object will continue in its state of motion unless compelled to change by a net force impressed upon it. That is, unless an unbalanced force acts on an object, the object’s velocity will not change: If the object is at rest, then it will stay at rest; and if it is moving, then it will continue to move at a constant speed in a straight line.
Basically, no net force means no change in velocity. This property of objects, their natural resistance to changes in their state of motion, is called inertia. In fact, the First Law is often referred to as the Law of Inertia.
THE SECOND LAW
Newton’s Second Law predicts what will happen when a net force does act on an object: The object’s velocity will change; the object will accelerate. More precisely, it says that its acceleration, a, will be directly proportional to the strength of the total—or net—force (Fnet) and inversely proportional to the object’s mass, m:
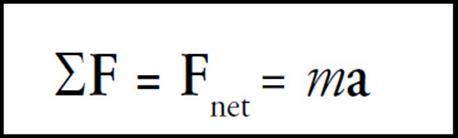
Always keep this force
equation in mind. This is
the most important equation
in mechanics!
The mass of an object is the quantitative measure of its inertia; intuitively, it measures how much matter is contained in an object. Two identical boxes, one empty and one full, have different masses. The box that is full has the greater mass, because it contains more stuff; more stuff, more mass. Mass is measured in kilograms, abbreviated as kg. (Note: An object whose mass is 1 kg weighs about 2.2 pounds.) It takes twice as much force to produce the same change in velocity of a 2 kg object than of a 1 kg object. Mass is a measure of an object’s inertia, its resistance to acceleration.
Forces are represented by vectors; they have magnitude and direction. If several different forces act on an object simultaneously, then the net force, Fnet, is the vector sum of all these forces. (The phrase resultant force is also used to mean net force.)
Since Fnet = ma, and m is a positive scalar, the direction of a always matches the direction of Fnet. Finally, since F = ma, the units for F equal the units of m times the units of a:
[F] = [m][a]
= kg m/s2
A force of 1 kg·m/s2 is renamed 1 newton (abbreviated as N). A medium-size apple weighs about 1 N.
THE THIRD LAW
This is the law that’s commonly remembered as, to every action, there is an equal, but opposite, reaction. More precisely, if Object 1 exerts a force on Object 2, then Object 2 exerts a force back on Object 1, equal in strength but opposite in direction. These two forces, F1-on-2 and F2-on-1, are called an action/reaction pair.
While the forces in an action/reaction are equal in magnitude, the ‘reaction’ (i.e., the resulting acceleration of the object) depends on the mass of each object. The greater the mass, the smaller the ‘reaction’ (acceleration); the smaller the mass, the greater the ‘reaction’ (acceleration).
Example 1 What net force is required to maintain a 5000 kg object moving at a constant velocity of magnitude 7500 m/s?
Solution. The First Law says that any object will continue in its state of motion unless a force acts on it. Therefore, no net force is required to maintain a 5000 kg object moving at a constant velocity of magnitude 7500 m/s. Here’s another way to look at it: Constant velocity means a = 0, so the equation Fnet = ma immediately gives Fnet = 0.
Example 2 How much force is required to cause an object of mass 2 kg to have an acceleration of 4 m/s2?
Solution. According to the Second Law, Fnet = ma = (2 kg)(4 m/s2) = 8 N.
Example 3 An object feels two forces; one of strength 8 N pulling to the left and one of strength 20 N pulling to the right. If the object’s mass is 4 kg, what is its acceleration?
Solution. Forces are represented by vectors and can be added and subtracted. Therefore, an 8 N force to the left added to a 20 N force to the right yields a net force of 20 – 8 = 12 N to the right. Then Newton’s Second Law gives a = Fnet/m = (12 N to the right)/(4 kg) = 3 m/s2 to the right.
WEIGHT
Mass and weight are not the same thing—there is a clear distinction between them in physics—but they are often used interchangeably in everyday life. The weight of an object is the gravitational force exerted on it by Earth (or by whatever planet it happens to be on). Mass, by contrast, is an intrinsic property of an object that measures its inertia. An object’s mass does not change with location. Put a baseball in a rocket and send it to the Moon. The baseball’s weight on the Moon is less than its weight here on Earth (because the Moon’s gravitational pull is weaker than Earth’s due to its much smaller mass), but the baseball’s mass would be the same.
Since weight is a force, we can use F = ma to compute it. What acceleration would the gravitational force impose on an object? The gravitational acceleration, of course! Therefore, setting a = g, the equation F = ma becomes
Fw = mg
This is the equation for the weight of an object of mass m. (Fg and Fw are both commonly used to represent the force of gravity. Weight is often symbolized merely by w, rather than Fw.) Notice that mass and weight are proportional but not identical. Furthermore, mass is measured in kilograms, while weight is measured in newtons.
Example 4 What is the mass of an object that weighs 500 N?
Solution. Since weight is m multiplied by g, mass is Fw (weight) divided by g. Therefore,
m = Fw/g = (500 N)/(10 m/s2) = 50 kg
Example 5 A person weighs 150 pounds. Given that a pound is a unit of weight equal to 4.45 N, what is this person’s mass?
Solution. This person’s weight in newtons is (150 lb)(4.45 N/lb) = 667.5 N, so his mass is
m = Fw/g = (667.5 N)/(10 m/s2) = 66.75 kg
m = Fw/g = (667.5 N)/(10 m/s2) = 67 kg
Example 6 A book whose mass is 2 kg rests on a table. Find the magnitude of the force exerted by the table on the book.
Solution. The book experiences two forces: The downward pull of Earth’s gravity and the upward, supporting force exerted by the table. Since the book is at rest on the table, its acceleration is zero, so the net force on the book must be zero. Therefore the magnitude of the support force must equal the magnitude of the book’s weight, which is Fw = mg = (2 kg)(10 m/s2) = 20 N.
Example 7 A can of paint with a mass of 6 kg hangs from a rope. If the can is to be pulled up to a rooftop with an acceleration of 1 m/s2, what must the tension in the rope be?
Solution. First draw a picture. Represent the object of interest (the can of paint) as a heavy dot, and draw the forces that act on the object as arrows connected to the dot. This is called a free-body (or force) diagram.
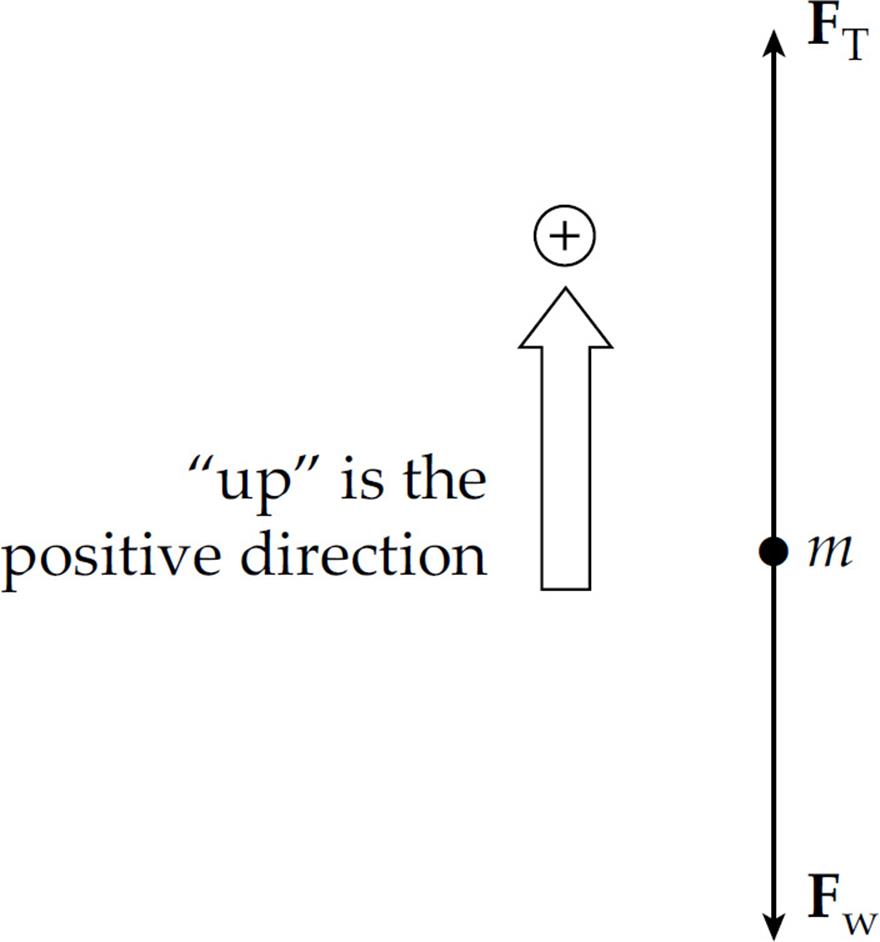
We have the tension force in the rope, FT (also symbolized merely by T), which is upward, and the weight, Fw, which is downward. Calling up the positive direction, the net force is FT – Fw. The Second Law, Fnet = ma, becomes FT – Fw = ma, so
FT = Fw + ma = mg + ma = m(g + a) = 6(10 + 1) = 66 N
Example 8 A can of paint with a mass of 6 kg hangs from a rope. If the can is to be pulled up to a rooftop with a constant velocity of 1 m/s, what must the tension in the rope be?
Solution. The phrase “constant velocity” automatically means a = 0 and, therefore, Fnet = 0. In the diagram above, FT would need to have the same magnitude as Fw in order to keep the can moving at a constant velocity. Thus, in this case, FT = Fw = mg = (6)(10) = 60 N.
Example 9 How much tension must a rope have to lift a 50 N object with an acceleration of 10 m/s2?
Solution. First draw a free-body diagram:
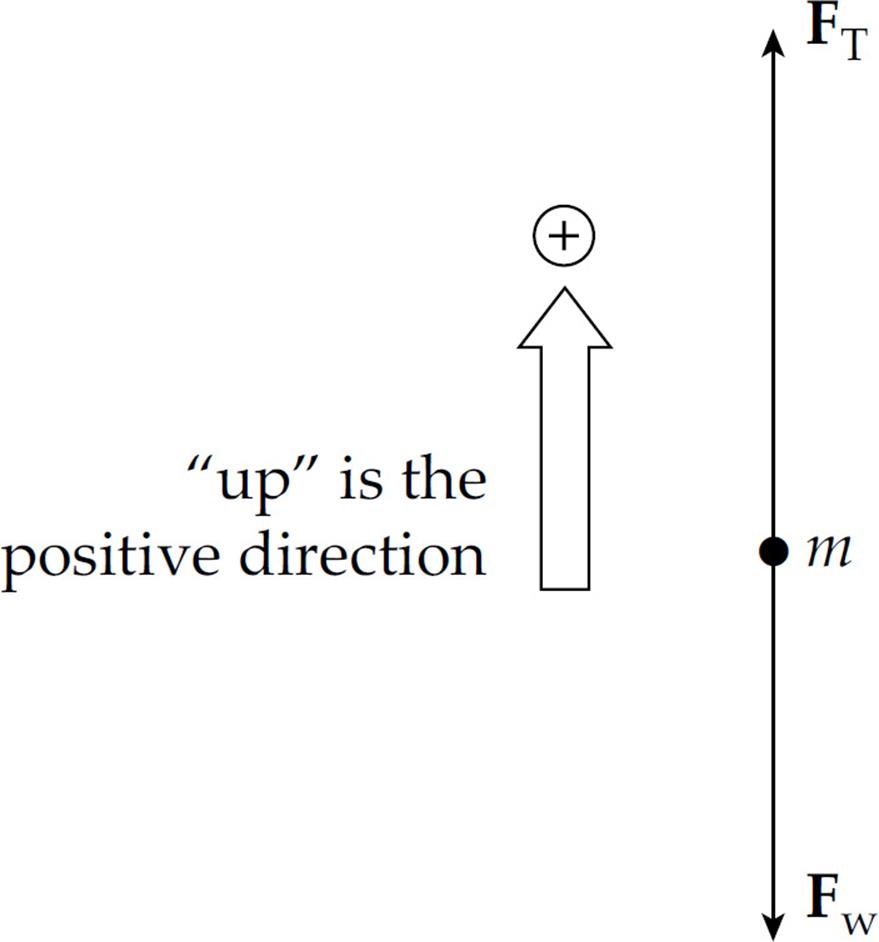
We have the tension force, FT, which is upward, and the weight, Fw, which is downward. Calling up the positive direction, the net force is FT – Fw. The Second Law, Fnet = ma, becomes FT – Fw = ma, so FT = Fw + ma. Remembering that m = Fw/g, we find that
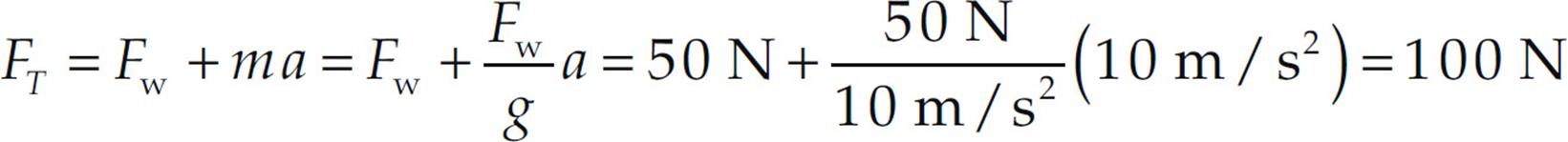
THE NORMAL FORCE
When an object is in contact with a surface, the surface exerts a contact force on the object. The component of the contact force that’s perpendicular to the surface is called the normal force on the object. The normal force comes from electric interactions among atoms. The normal force is what prevents objects from falling through tabletops or you from falling through the floor. The normal force is denoted by FN, or simply by N. (If you use the latter notation, be careful not to confuse it with N, the abbreviation for the newton.)
The word normal also
means perpendicular.
Example 10 A book whose mass is 2 kg rests on a table. Find the magnitude of the normal force exerted by the table on the book.
Solution. The book experiences two forces: The downward pull of Earth’s gravity and the upward, supporting force exerted by the table. Since the book is at rest on the table, its acceleration is zero, so the net force on the book must be zero. Therefore, the magnitude of the support force must equal the magnitude of the book’s weight, which is Fw = mg = (2)(10) = 20 N. This means the normal force must be 20 N as well: FN = 20 N. (Note that this is a repeat of Example 6, except now we have a name for the “upward, supporting force exerted by the table”; it’s called thenormal force.)
FRICTION
When an object is in contact with a surface, the surface exerts a contact force on the object. The component of the contact force that’s parallel to the surface is called the friction force on the object. Friction, like the normal force, arises from electrical interactions between atoms of which the object is composed and those of which the surface is composed.
Keep in mind that friction
comes from electric forces.
This explains why both
the normal force and the
static friction force can
change depending on how
much force is applied.
We’ll look at two main categories of friction: (1) static friction and (2) kinetic (sliding) friction. If you attempt to push a heavy crate across a floor, at first you meet with resistance, but then you push hard enough to get the crate moving. The force that acted on the crate to cancel out your initial pushes was static friction, and the force that acts on the crate as it slides across the floor is kinetic friction. Static friction occurs when there is no relative motion between the object and the surface (no sliding); kinetic friction occurs when there is relative motion (when there’s sliding).
The strength of the friction force depends, in general, on two things: The nature of the surfaces and the strength of the normal force. The nature of the surfaces is represented by the coefficient of friction, denoted by μ, which stands for the Greek letter mu. The greater this number is, the stronger the friction force will be. For example, the coefficient of friction between rubber-soled shoes and a wooden floor is 0.7, but between rubber-soled shoes and ice, it’s only 0.1. Also, since kinetic friction is generally weaker than static friction (it’s easier to keep an object sliding once it’s sliding than it is to start the object sliding in the first place), there are two coefficients of friction; one for static friction (μs) and one for kinetic friction (μk). For a given pair of surfaces, it’s virtually always true that μk < μs. The strengths of these two types of friction forces are given by the following equations:
Fstatic friction, max = μsFN or Fstatic friction, max ≤ μsFN
Fkinetic friction = μkFN
On the equation sheet for the free response section, this information is represented as follows:

Note that the equation for the strength of the static friction force is for the maximum or lesser value. This is because static friction can vary, precisely counteracting weaker forces that attempt to move an object. For example, suppose an object feels a normal force of FN = 100 N and the coefficient of static friction between it and the surface it’s on is 0.5. Then, the maximum force that static friction can exert is (0.5)(100 N) = 50 N. However, if you push on the object with a force of, say, 20 N, then the static friction force will be 20 N (in the opposite direction), not 50 N; the object won’t move. The net force on a stationary object must be zero. Static friction can take on all values, up to a certain maximum, and you must overcome the maximum static friction force to get the object to slide. The direction of Fkinetic friction = Ff (kinetic) is opposite to that of motion (sliding), and the direction of Fstatic friction = Ff (static) is opposite to that of the intended motion.
Example 11 A crate of mass 20 kg is sliding across a wooden floor. The coefficient of kinetic friction between the crate and the floor is 0.3.
(a) Determine the strength of the friction force acting on the crate.
(b) If the crate is being pulled by a force of 90 N (parallel to the floor), find the acceleration of the crate.
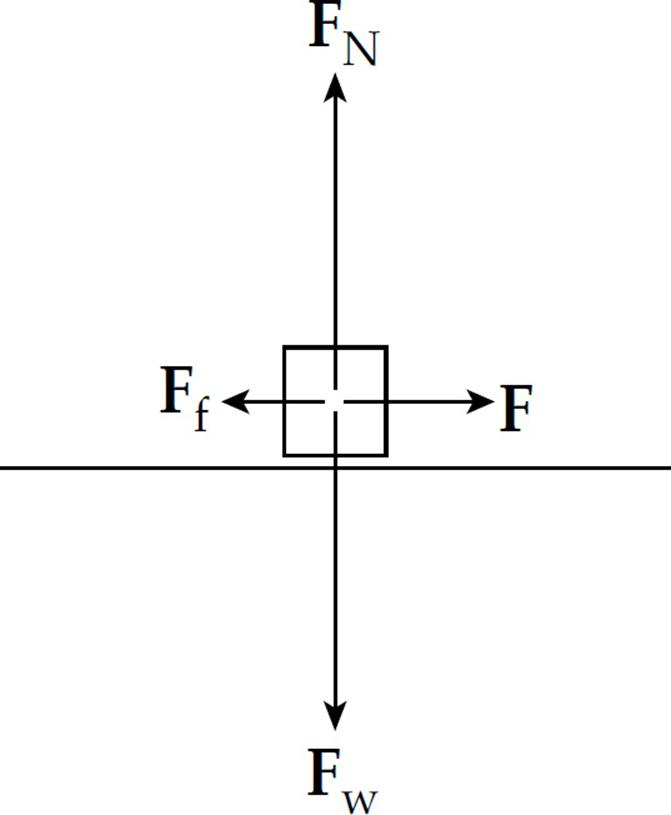
Solution. First draw a free-body diagram.
In part (a) F = 0, and in part (b) F = 90 N for our free-body diagram. Reminder: Separate the horizontal and vertical forces and use ΣFx = max and ΣFy = may.
(a) The normal force on the object balances the object’s weight, so FN = mg = (20 kg)(10 m/s2) = 200 N. Therefore, F(kinetic) = μkFN = (0.3)(200 N) = 60 N.
(b) The net horizontal force that acts on the crate is F – Ff = 90 N – 60 N = 30 N, so the acceleration of the crate is a = Fnet/m = (30 N)/(20 kg) = 1.5 m/s2.
Example 12 A crate of mass 100 kg rests on the floor. The coefficient of static friction is 0.4. If a force of 250 N (parallel to the floor) is applied to the crate, what’s the magnitude of the force of static friction on the crate?
Solution. The normal force on the object balances its weight, so FN = mg = (100 kg)(10 m/s2) = 1,000 N. Therefore, Fstatic friction, max = Ff (static), max = μsFN = (0.4)(1,000 N) = 400 N. This is the maximum force that static friction can exert, but in this case it’s not the actual value of the static friction force. Since the applied force on the crate is only 250 N, which is less than the Ff (static), max, the force of static friction will be less also: Ff (static) = 250 N, and the crate will not slide.
PULLEYS
Pulleys are devices that change the direction of the tension force in the cords that slide over them. Here we’ll consider each pulley to be frictionless and massless, which means that their masses are so much smaller than the objects of interest in the problem that they can be ignored.
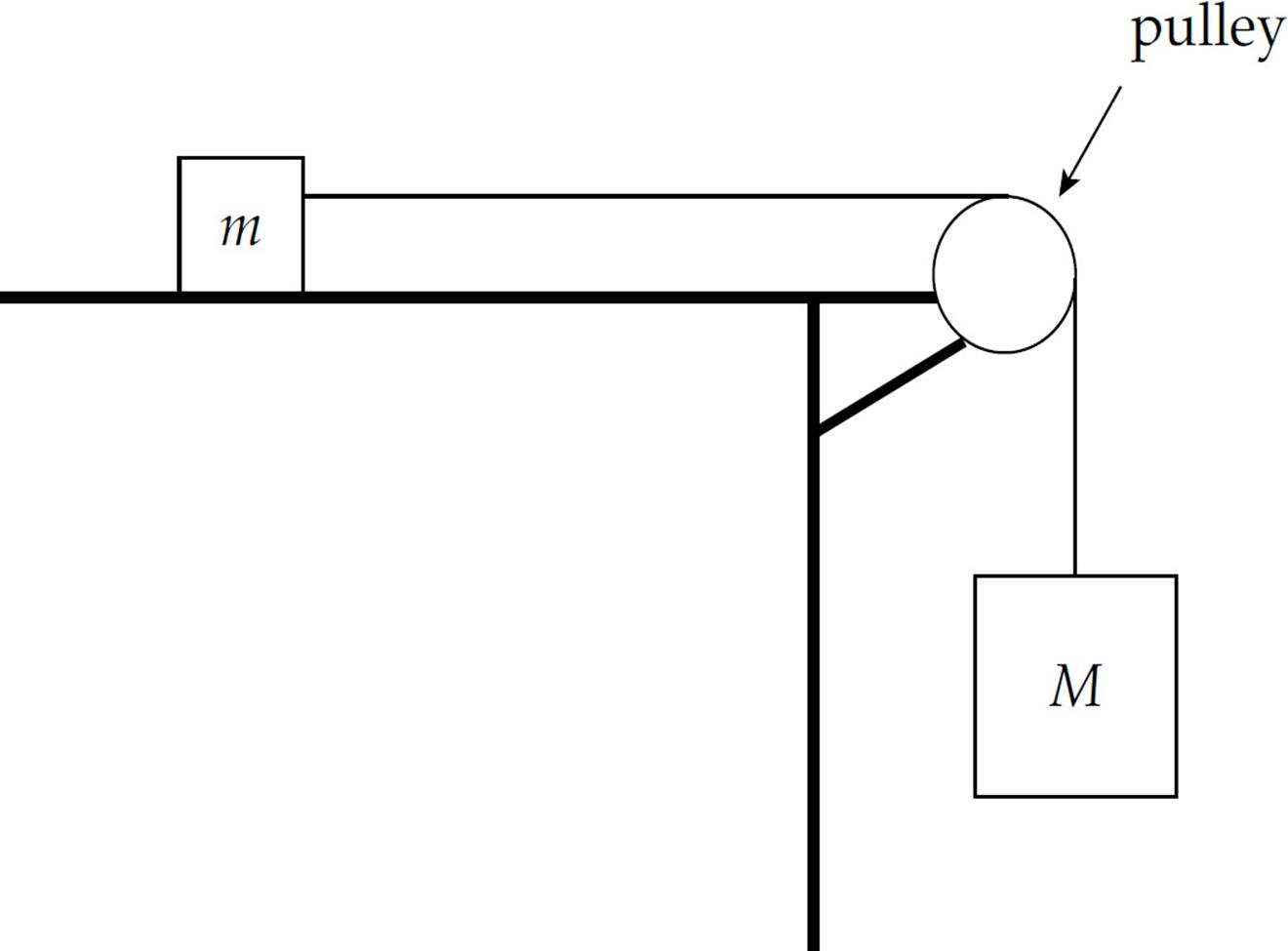
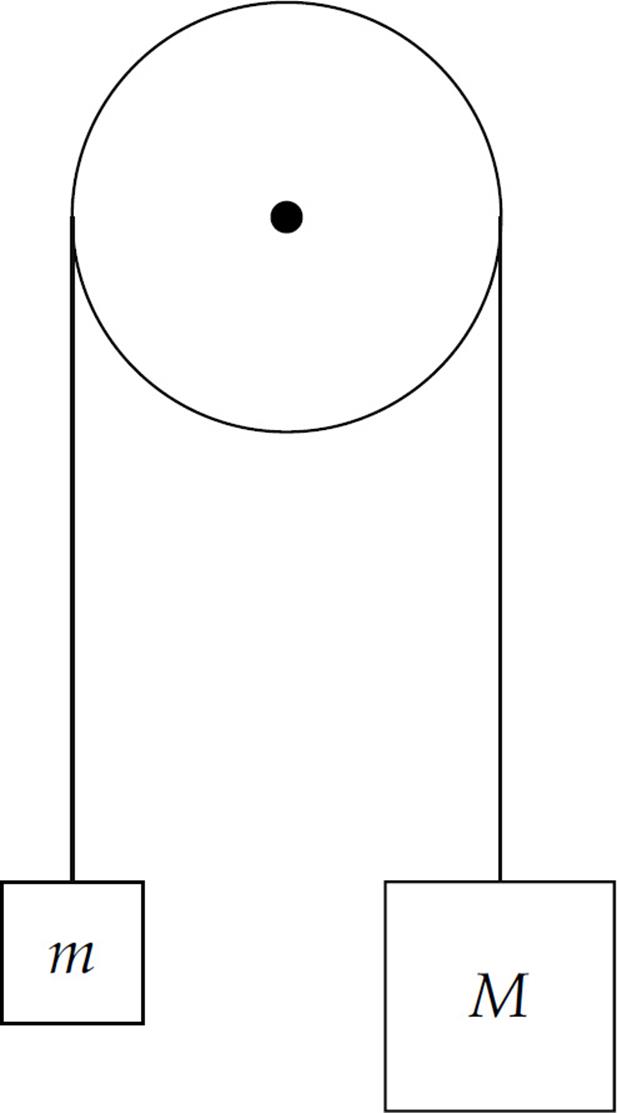
Example 13 In the diagram above, assume that the tabletop is frictionless. Determine the acceleration of the blocks once they’re released from rest.
Solution. There are two blocks, so we draw two free-body diagrams: The positive directions for each block must coincide. If the block on the table travels to the right then the hanging block travels down. This is why down is positive for the hanging block.

To get the acceleration of each one, we use Newton’s Second Law, Fnet = ma.
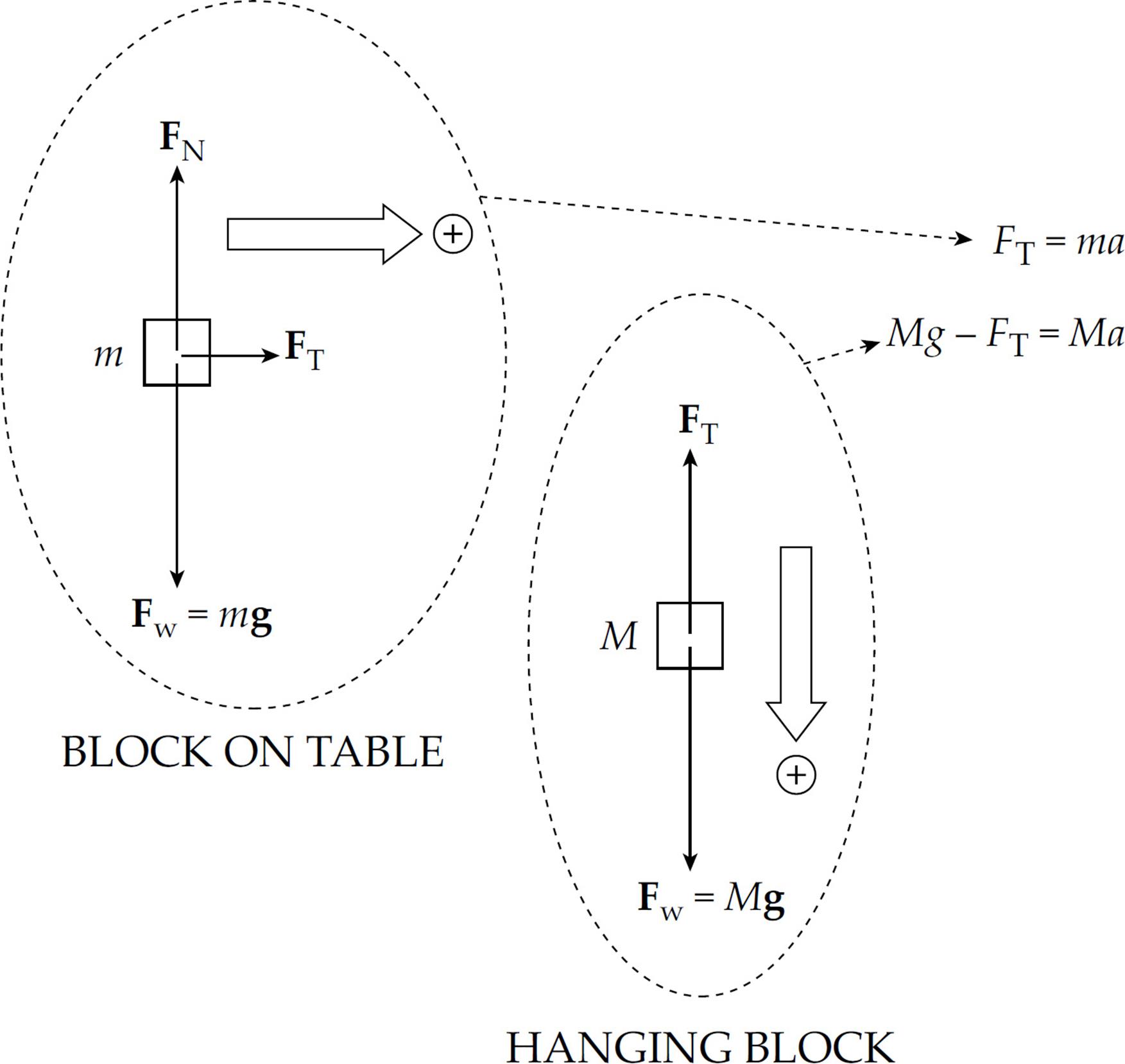
Note that there are two unknowns, FT and a, but we can eliminate FT by adding the two equations, and then we can solve for a.
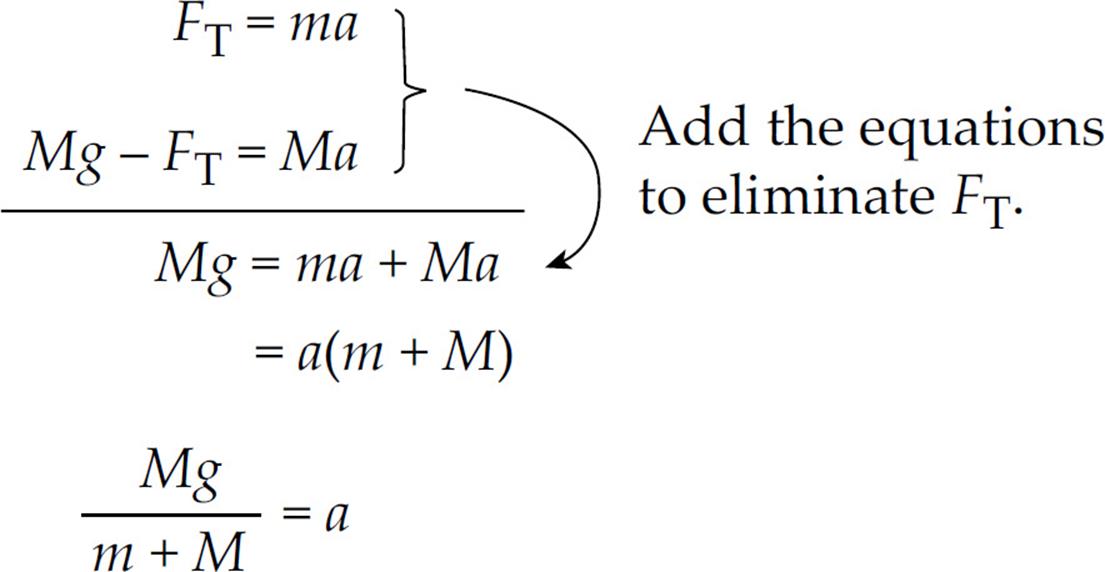
Example 14 Using the same diagram as in the previous example, assume that m = 2 kg, M = 10 kg, and the coefficient of kinetic friction between the small block and the tabletop is 0.5. Compute the acceleration of the blocks.
Solution. Once again, draw a free-body diagram for each object. Note that the only difference between these diagrams and the ones in the previous example is the inclusion of the force of (kinetic) friction, Ff, that acts on the block on the table.
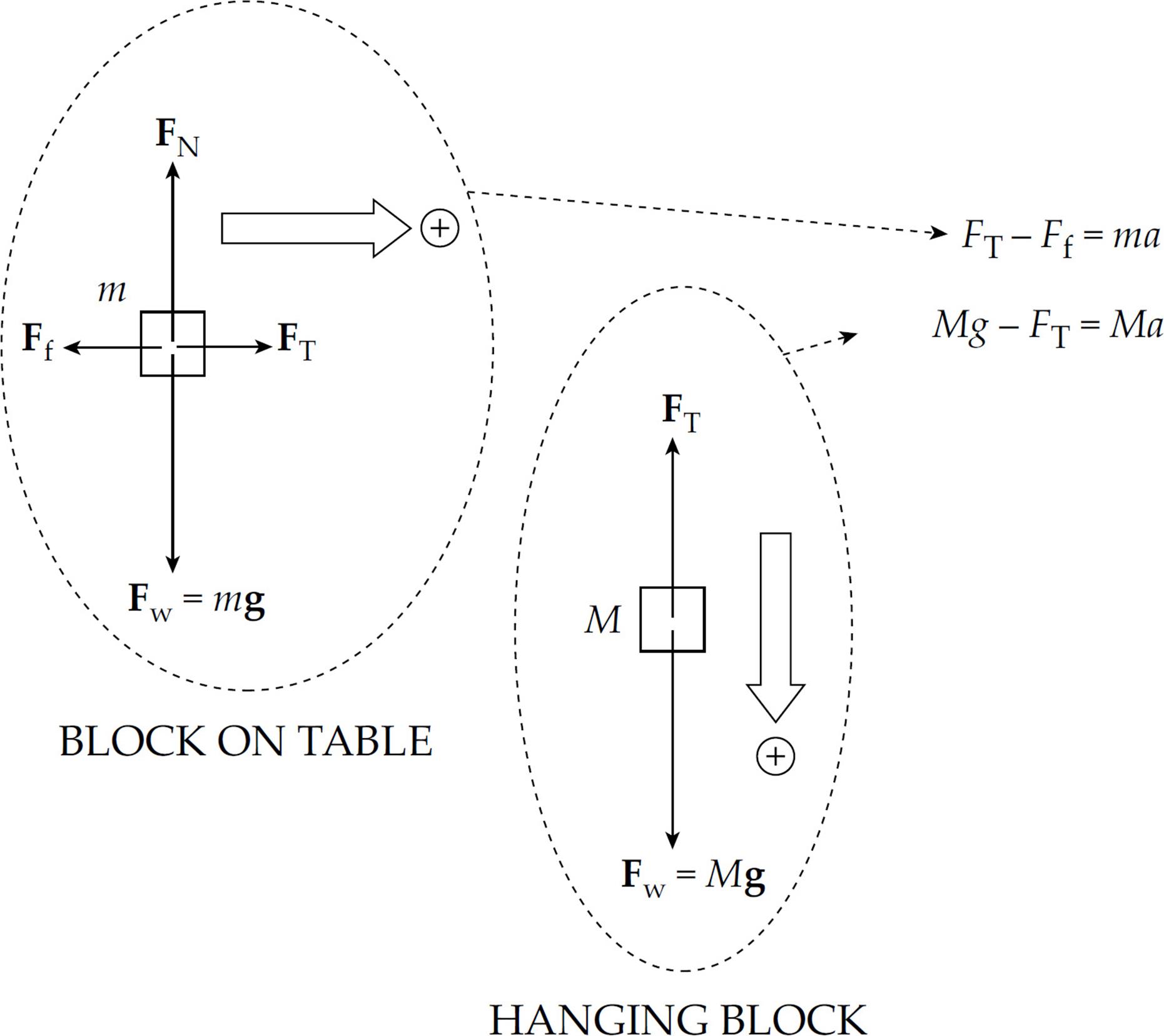
As before, we have two equations that contain two unknowns (a and FT):
FT – Ff = ma (1)
Mg – FT = Ma (2)
Add the equations (thereby eliminating FT) and solve for a. Note that, by definition, Ff = μ FN, and from the free-body diagram for m, we see that FN = mg, so Ff = μ mg:
Mg – Ff = ma + Ma
Mg – μmg = a(m + M)
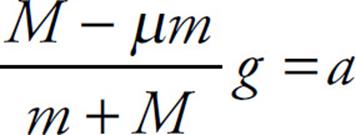
Substituting in the numerical values given for m, M, and μ, we find that a = ![]() g (or 7.5 m/s2).
g (or 7.5 m/s2).
Example 15 In the previous example, calculate the tension in the cord.
Solution. Since the value of a has been determined, we can use either of the two original equations to calculate FT. Using Equation (2), Mg – FT = Ma (because it’s simpler), we find

As you can see, we would have found the same answer if Equation (1) had been used:
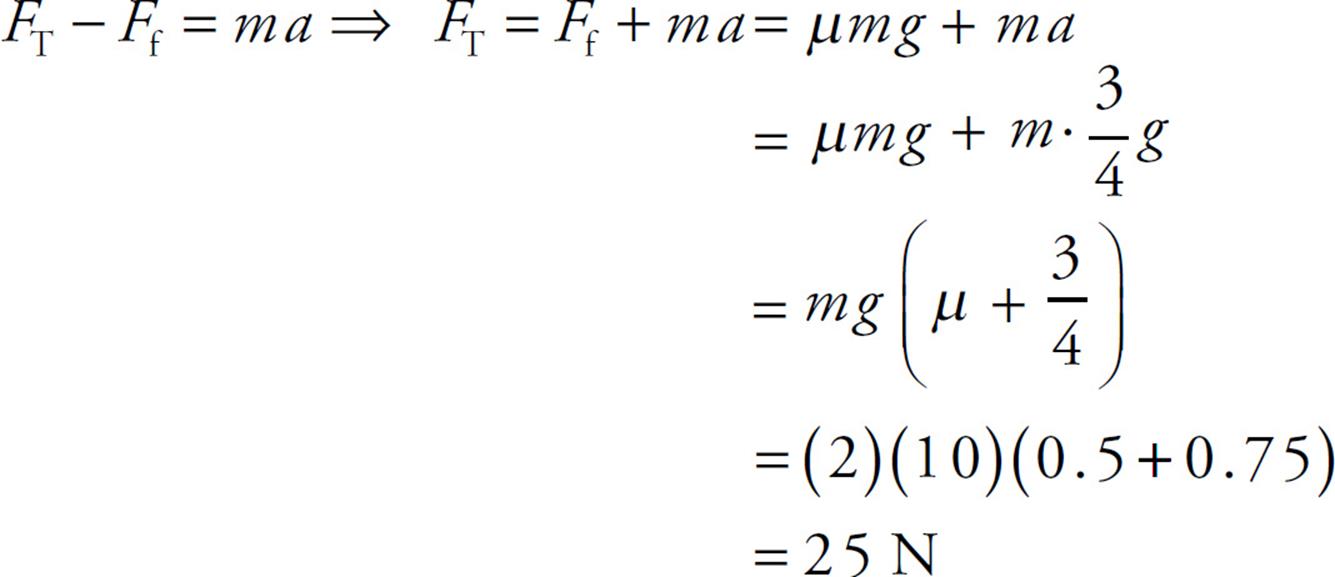
INCLINED PLANES
An inclined plane is basically a ramp. If an object of mass m is on the ramp, then the force of gravity on the object, Fw = mg, has two components: One that’s parallel to the ramp (mg sin θ) and one that’s normal to the ramp (mg cos θ), where θ is the incline angle. The force driving the block down the inclined plane is the component of the block’s weight that’s parallel to the ramp: mg sin θ.
When analyzing objects moving up or down inclined planes it is almost always easiest to rotate the coordinate axes such that the x-axis is parallel to the incline and the y-axis is perpendicular to the incline, as shown in the diagram. The object would accelerate in both the x- and y-directions as it moved down along the incline if you did not rotate the axis. However, with the rotated axes the acceleration in the y-direction is zero. Now we only have to worry about the acceleration in the x-direction.
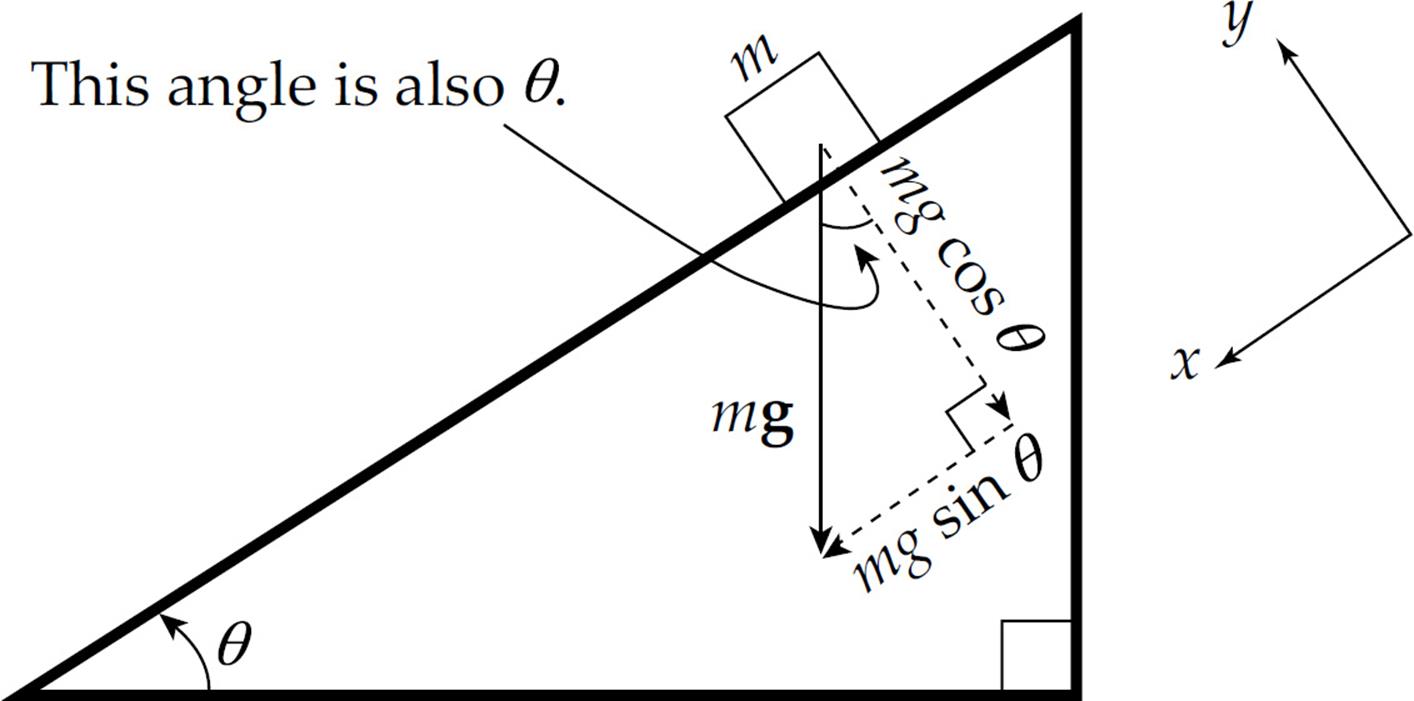
Example 16 A block slides down a frictionless, inclined plane that makes a 30° angle with the horizontal. Find the acceleration of this block.
Solution. Let m denote the mass of the block, so the force that pulls the block down the incline is mg sin θ, and the block’s acceleration down the plane is
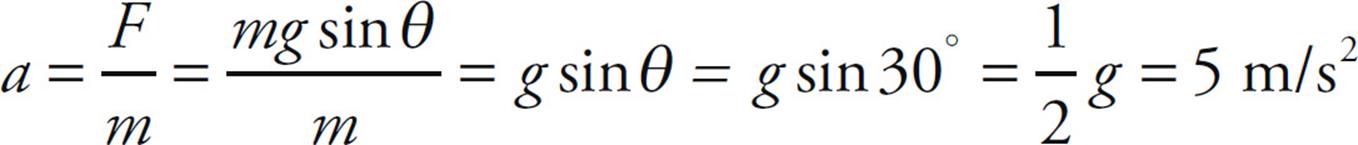
Example 17 A block slides down an inclined plane that makes a 30° angle with the horizontal. If the coefficient of kinetic friction is 0.3, find the acceleration of the block.
Solution. First draw a free-body diagram. Notice that, in the diagram shown below, the weight of the block, Fw = mg, has been written in terms of its scalar components: Fw sin θ parallel to the ramp and Fw cos θ normal to the ramp:
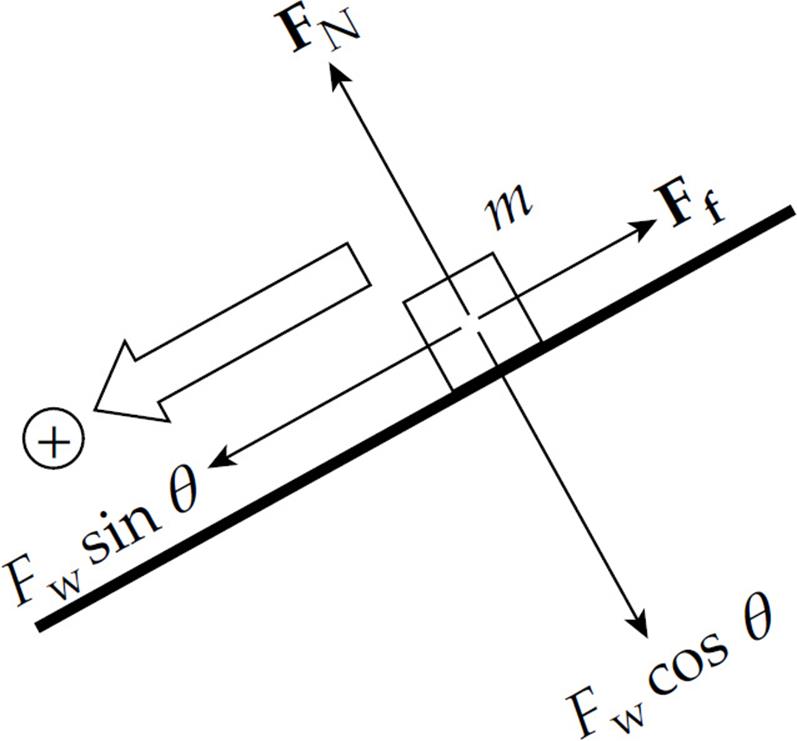
The force of friction, Ff, that acts up the ramp (opposite to the direction in which the block slides) has magnitude Ff = μFN. But the diagram shows that FN = Fw cos θ, so Ff = μ(mg cos θ). Therefore the net force down the ramp is
Fw sin θ – Ff = mg sin θ – μ mg cos θ = mg(sin θ – μ cos θ)
Then, setting Fnet equal to ma, we solve for a:
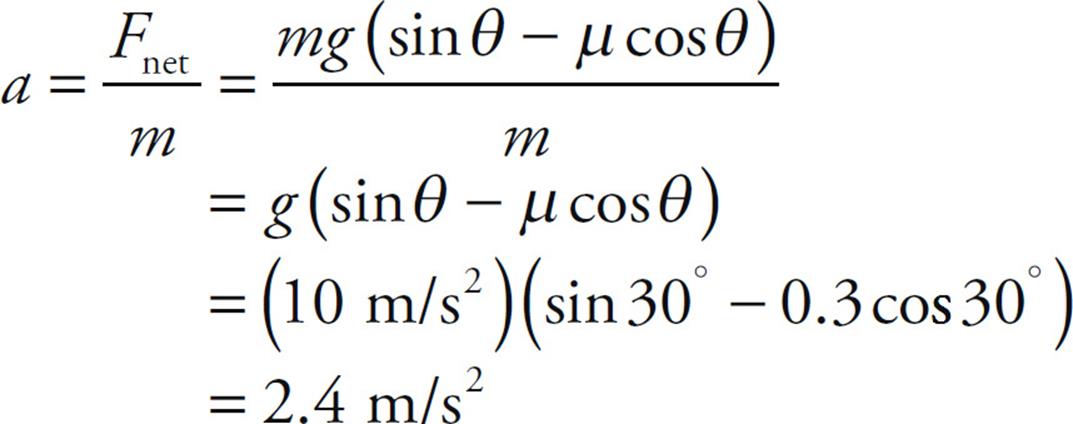
UNIFORM CIRCULAR MOTION
In Chapter 4, we considered two types of motion; straight-line motion and parabolic motion. We will now look at motion that follows a circular path, such as a rock on the end of a string, a horse on a merry-go-round, and (to a good approximation) the Moon around Earth and Earth around the Sun.
Let’s simplify matters and consider the object’s speed around its path to be constant. This is called uniform circular motion. You should remember that although the speed may be constant, the velocity is not, because the direction of the velocity is always changing. Since the velocity is changing, there must be acceleration. This acceleration does not change the speed of the object; it only changes the direction of the velocity to keep the object on its circular path. Also, in order to produce an acceleration, there must be a force; otherwise, the object would move off in a straight line (Newton’s First Law).
The figure on the left on the next page shows an object moving along a circular trajectory, along with its velocity vectors at two nearby points. The vector v1 is the object’s velocity at time t = t1, and v2 is the object’s velocity vector a short time later (at time t = t2). The velocity vector is always tangential to the object’s path (whatever the shape of the trajectory). Notice that since we are assuming constant speed, the lengths of v1 and v2 (their magnitudes) are the same.

Since  v = v2 – v1 points toward the center of the circle (see the figure on the right), so does the acceleration, since a =
v = v2 – v1 points toward the center of the circle (see the figure on the right), so does the acceleration, since a =  v/
v/ t. Because the acceleration vector points toward the center of the circle, it’s called centripetal acceleration, or ac. The centripetal acceleration is what turns the velocity vector to keep the object traveling in a circle. The magnitude of the centripetal acceleration depends on the object’s speed, v, and the radius, r, of the circular path according to the equation
t. Because the acceleration vector points toward the center of the circle, it’s called centripetal acceleration, or ac. The centripetal acceleration is what turns the velocity vector to keep the object traveling in a circle. The magnitude of the centripetal acceleration depends on the object’s speed, v, and the radius, r, of the circular path according to the equation
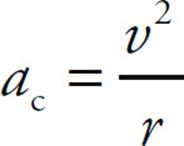
When this is given on the free response equation sheet, it is given in terms of angular velocity as well, which will be discussed later.
Example 18 An object of mass 5 kg moves at a constant speed of 6 m/s in a circular path of radius 2 m. Find the object’s acceleration and the net force responsible for its motion.
Solution. By definition, an object moving at constant speed in a circular path is undergoing uniform circular motion. Therefore, it experiences a centripetal acceleration of magnitude v2/r, always directed toward the center of the circle:
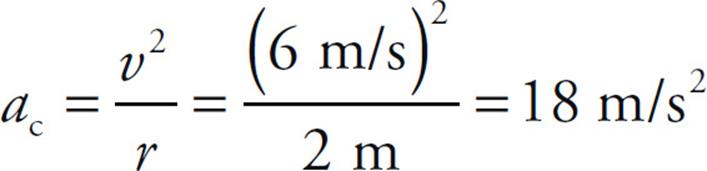
The force that produces the centripetal acceleration is given by Newton’s Second Law, coupled with the equation for centripetal acceleration:

This equation gives the magnitude of the force. As for the direction, recall that because F = ma, the directions of F and a are always the same. Since centripetal acceleration points toward the center of the circular path, so does the force that produces it. Therefore, it’s called centripetal force. Centripetal force is provided by everyday forces such as tension, friction, gravity, or normal forces. The centripetal force acting on this object has a magnitude of Fc = mac = (5 kg)(18 m/s2) = 90 N.
Two common types of circular motion are offered here. The diagrams on the next page show examples of a ball on a string traveling in a horizontal circle and a vertical circle.

Example 19 A 10 kg mass is attached to a string that has a breaking strength of 200 N. If the mass is whirled in a horizontal circle of radius 80 cm, what maximum speed can it have? Assume the string is horizontal.
Solution. The first thing to do in problems like this is to identify what force(s) provide the centripetal force. In this example, the tension in the string provides the centripetal force:
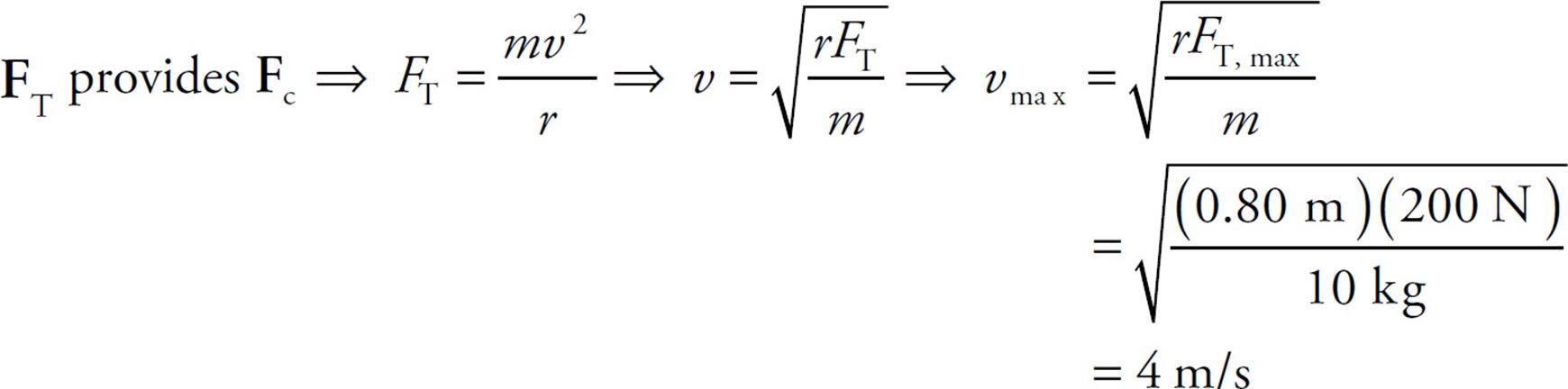
Example 20 An athlete who weighs 800 N is running around a curve at a speed of 5.0 m/s in an arc whose radius of curvature, r, is 5.0 m. Assuming his weight is equal to the force of static friction, find the centripetal force acting on him. What could happen to him if r were smaller?
Solution. Using the equation for the strength of the centripetal force, we find that
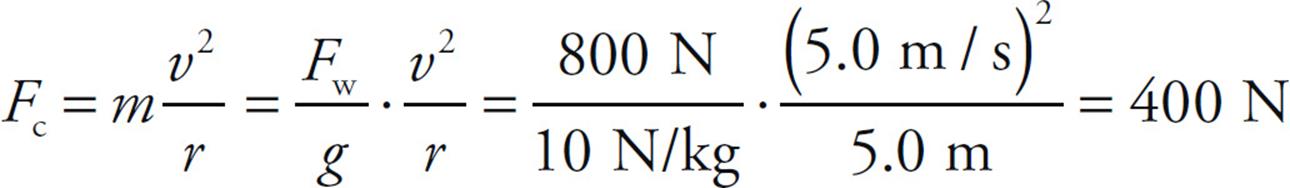
In this case, static friction provides the centripetal force. Since his weight is equal to the force of static friction, this means the coefficient of static friction between his shoes and the ground is 1, so the maximum force that static friction can exert is μsFN ≈ FN = Fw = 800 N. Fortunately, 800 N is greater than 400 N. But notice that if the radius of curvature of the arc were much smaller, then Fc would become greater than what static friction could handle, and he would slip.
Example 21 A roller-coaster car enters the circular-loop portion of the ride. At the very top of the circle (where the people in the car are upside down), the speed of the car is 25 m/s, and the acceleration points straight down. If the diameter of the loop is 50 m and the total mass of the car (plus passengers) is 1200 kg, find the magnitude of the normal force exerted by the track on the car at this point. Also find the normal force exerted by the track on the car when it is at the bottom of the loop. Assume it is still traveling 25 m/s at that location as well.
Solution. When analyzing circular motion, consider all forces pointing toward the center to be positive and all forces pointing away to be negative. There are two forces acting on the car at its topmost point: the normal force exerted by the track and the gravitational force, both of which point downward.
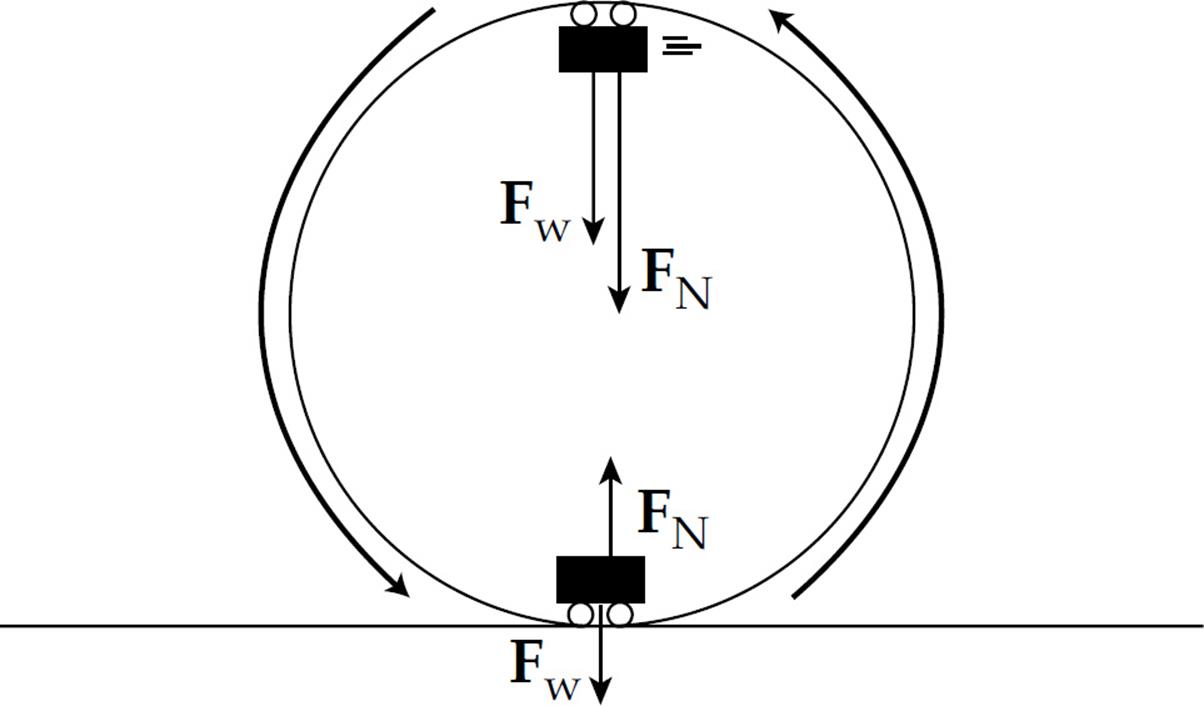
The combination of these two forces, FN + Fw, provides the centripetal force:
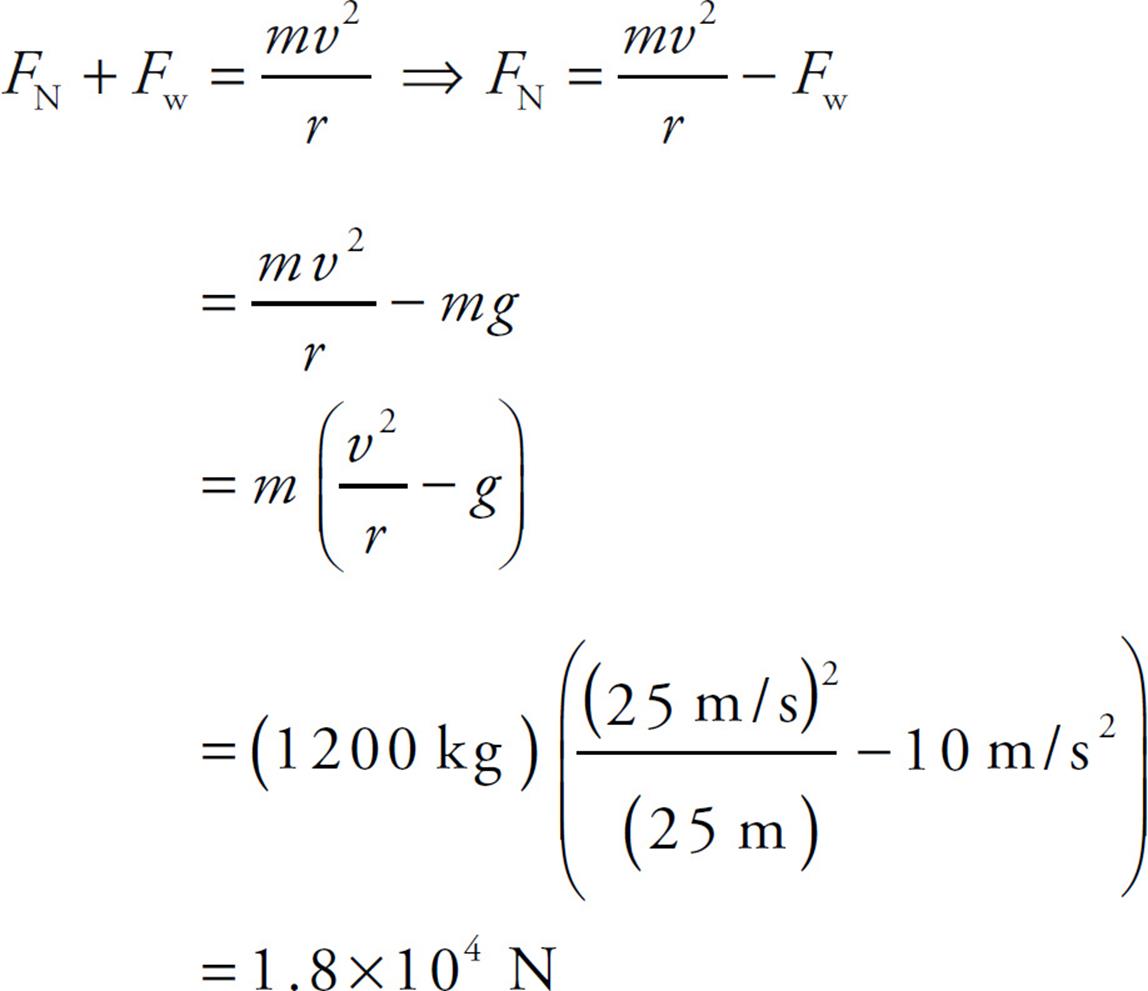
Now we will determine the normal force exerted by the track on the car at the bottom of the loop.
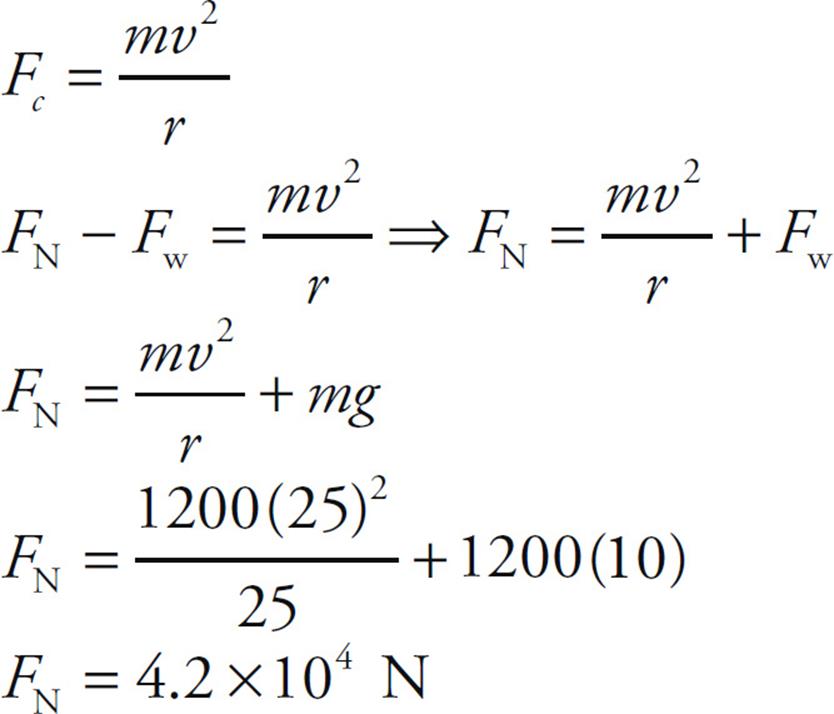
Notice that the normal force is much greater when the car is at the bottom of the track than when it is at the top. When it is at the top of the track, gravity is helping the car travel in a circle.
Example 22 In the previous example, if the net force on the car at its topmost point is straight down, why doesn’t the car fall straight down?
Solution. Remember that force tells an object how to accelerate. If the car had zero velocity at this point, then it would certainly fall straight down, but the car has a non-zero velocity (to the left) at this point. The fact that the acceleration is downward means that, at the next moment, vwill point down to the left at a slight angle, ensuring that the car remains on a circular path, in contact with the track. The minimum centripital acceleration of the car at the top of the track would be equal to the acceleration of gravity, g = 9.8 m/s2. If ac were less than g then the car would fall off its circular path.
CHAPTER 5 REVIEW QUESTIONS
The answers and explanations can be found in Chapter 16.
Section I: Multiple Choice
1. A person standing on a horizontal floor feels two forces: the downward pull of gravity and the upward supporting force from the floor. These two forces
(A) have equal magnitudes and form an action/reaction pair
(B) have equal magnitudes but do not form an action/reaction pair
(C) have unequal magnitudes and form an action/reaction pair
(D) have unequal magnitudes and do not form an action/reaction pair
(E) none of the above
2. A person who weighs 800 N steps onto a scale that is on the floor of an elevator car. If the elevator accelerates upward at a rate of 5 m/s2, what will the scale read?
(A) 400 N
(B) 800 N
(C) 1000 N
(D) 1200 N
(E) 1600 N
3. A frictionless inclined plane of length 20 m has a maximum vertical height of 5 m. If an object of mass 2 kg is placed on the plane, which is the net force it feels?
(A) 5 N
(B) 10 N
(C) 15 N
(D) 20 N
(E) 30 N
4. A 20 N block is being pushed across a horizontal table by an 18 N force. If the coefficient of kinetic friction between the block and the table is 0.4, find the acceleration of the block.
(A) 0.5 m/s2
(B) 1 m/s2
(C) 5 m/s2
(D) 7.5 m/s2
(E) 9 m/s2
5. The coefficient of static friction between a box and a ramp is 0.5. The ramp’s incline angle is 30°. If the box is placed at rest on the ramp, the box will do which of the following?
(A) Accelerate down the ramp.
(B) Accelerate briefly down the ramp but then slow down and stop.
(C) Move with constant velocity down the ramp.
(D) Not move.
(E) Cannot be determined from the information given.
6.
Assuming a frictionless, massless pulley, determine the acceleration of the blocks once they are released from rest.
(A) 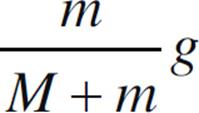
(B) 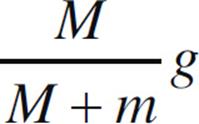
(C) 
(D) 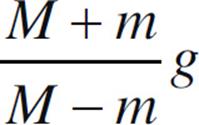
(E) 
7. If all of the forces acting on an object balance so that the net force is zero and the object’s mass remains constant, then
(A) the object must be at rest
(B) the object’s speed will decrease
(C) the object will follow a parabolic trajectory
(D) the object’s direction of motion can change, but not its speed
(E) none of the above
8. A block of mass m is at rest on a frictionless, horizontal table placed in a laboratory on the surface of Earth. An identical block is at rest on a frictionless, horizontal table placed on the surface of the Moon. Let F be the net force necessary to give Earth-bound block an acceleration of a across the table. Given that gMoon is one-sixth of gEarth, the force necessary to give the Moon-bound block the same acceleration a across the table is
(A) F/12
(B) F/6
(C) F/3
(D) F
(E) 6F
9. A crate of mass 100 kg is at rest on a horizontal floor. The coefficient of static friction between the crate and the floor is 0.4, and the coefficient of kinetic friction is 0.3. A force F of magnitude 344 N is then applied to the crate, parallel to the floor. Which of the following is true?
(A) The crate will accelerate across the floor at 0.5 m/s2.
(B) The static friction force, which is the reaction force to F as guaranteed by Newton’s Third Law, will also have a magnitude of 344 N.
(C) The crate will slide across the floor at a constant speed of 0.5 m/s.
(D) The crate will not move.
(E) None of the above is true.
10. An object moves at constant speed in a circular path. Which of the following statements is/are true?
I. The velocity is constant.
II. The acceleration is constant.
III. The net force on the object is zero.
(A) II only
(B) I and III only
(C) II and III only
(D) I and II only
(E) None of the above
Questions 11–13:
A 60 cm rope is tied to the handle of a bucket which is then whirled in a vertical circle. The mass of the bucket is 3 kg.
11. At the lowest point in its path, the tension in the rope is 50 N. What is the speed of the bucket?
(A) 1 m/s
(B) 2 m/s
(C) 3 m/s
(D) 4 m/s
(E) 5 m/s
12. What is the critical speed below which the rope would become slack when the bucket reaches the highest point in the circle?
(A) 0.6 m/s
(B) 1.8 m/s
(C) 2.4 m/s
(D) 3.2 m/s
(E) 4.8 m/s
13. An object moves at a constant speed in a circular path of radius r at a rate of 1 revolution per second. What is its acceleration?
(A) 0
(B) 2π2r
(C) 2π2r2
(D) 4π2r
(E) 4π2r2
Section II: Free Response
1. This question concerns the motion of a crate being pulled across a horizontal floor by a rope. In the diagram below, the mass of the crate is m, the coefficient of kinetic friction between the crate and the floor is μ, and the tension in the rope is FT.

(a) Draw and label all of the forces acting on the crate.
(b) Compute the normal force acting on the crate in terms of m, FT, θ, and g.
(c) Compute the acceleration of the crate in terms of m, FT, θ, μ, and g.
(d) Assume that the magnitude of the tension in the rope is fixed but that the angle may be varied. For what value of θ would the resulting horizontal acceleration of the crate be maximized?
2. In the diagram below, a massless string connects two blocks—of masses m1 and m2, respectively—on a flat, frictionless tabletop. A force F pulls on Block #2, as shown:
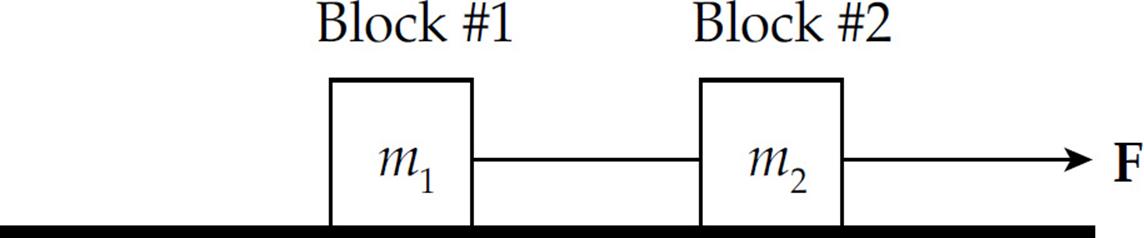
Solve for the following in terms of given quantities.
(a) Draw and label all of the forces acting on Block #1.
(b) Draw and label all of the forces acting on Block #2.
(c) What is the acceleration of Block #1?
(d) What is the tension in the string connecting the two blocks?
(e) If the string connecting the blocks were not massless, but instead had a mass of m, find
(i) the acceleration of Block #1, and
(ii) the difference between the strength of the force that the connecting string exerts on Block #2 and the strength of the force that the connecting string exerts on Block #1.
3. In the figure shown, assume that the pulley is frictionless and massless.
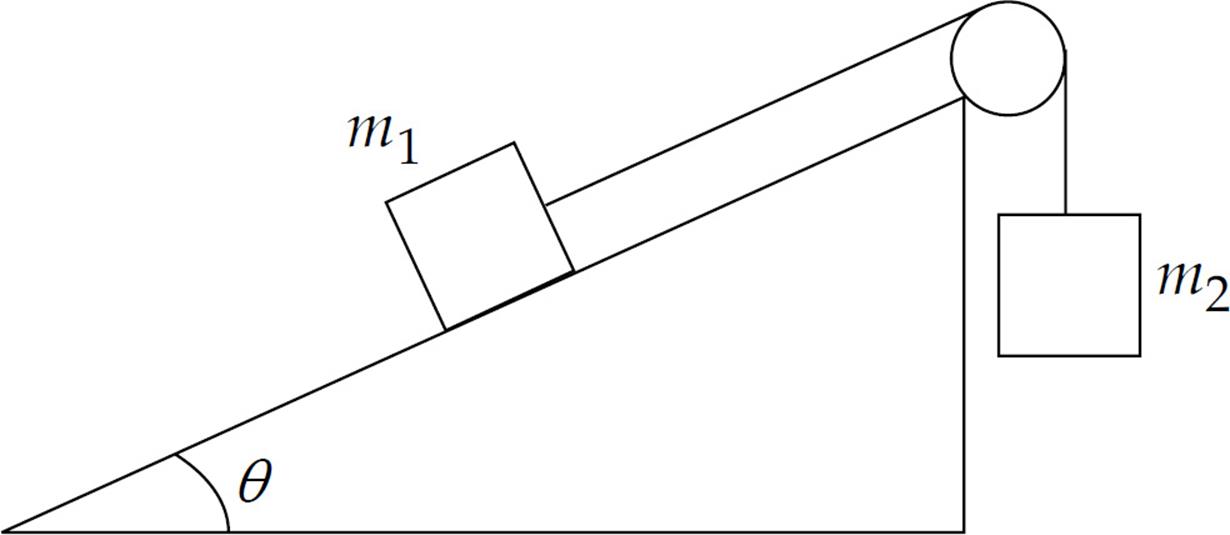
Solve for the following in terms of given quantities and the acceleration of gravity, g.
(a) If the surface of the inclined plane is frictionless, determine what value(s) of θ will cause the box of mass m1 to
(i) accelerate up the ramp
(ii) slide up the ramp at constant speed.
(b) If the coefficient of kinetic friction between the surface of the inclined plane and the box of mass m1 is μk, derive (but do not solve) an equation satisfied by the value of θ which will cause the box of mass m1 to slide up the ramp at constant speed.
4. A sky diver is falling with speed v0 through the air. At that moment (time t = 0), she opens her parachute and experiences the force of air resistance whose strength is given by the equation F = kv, where k is a proportionality constant and v is her descent speed. The total mass of the sky diver and equipment is m. Assume that g is constant throughout her descent.
(a) Draw and label all the forces acting on the sky diver after her parachute opens.
(b) Determine the sky diver’s acceleration in terms of m, v, k, and g.
(c) Determine the sky diver’s terminal speed (that is, the eventual constant speed of descent).
(d) Sketch a graph of v as a function of time, starting at t = 0 and going until she lands, being sure to label important values on the vertical axis.
(e) Derive an expression for her descent speed, v, as a function of time t since opening her parachute in terms of m, k, and g.
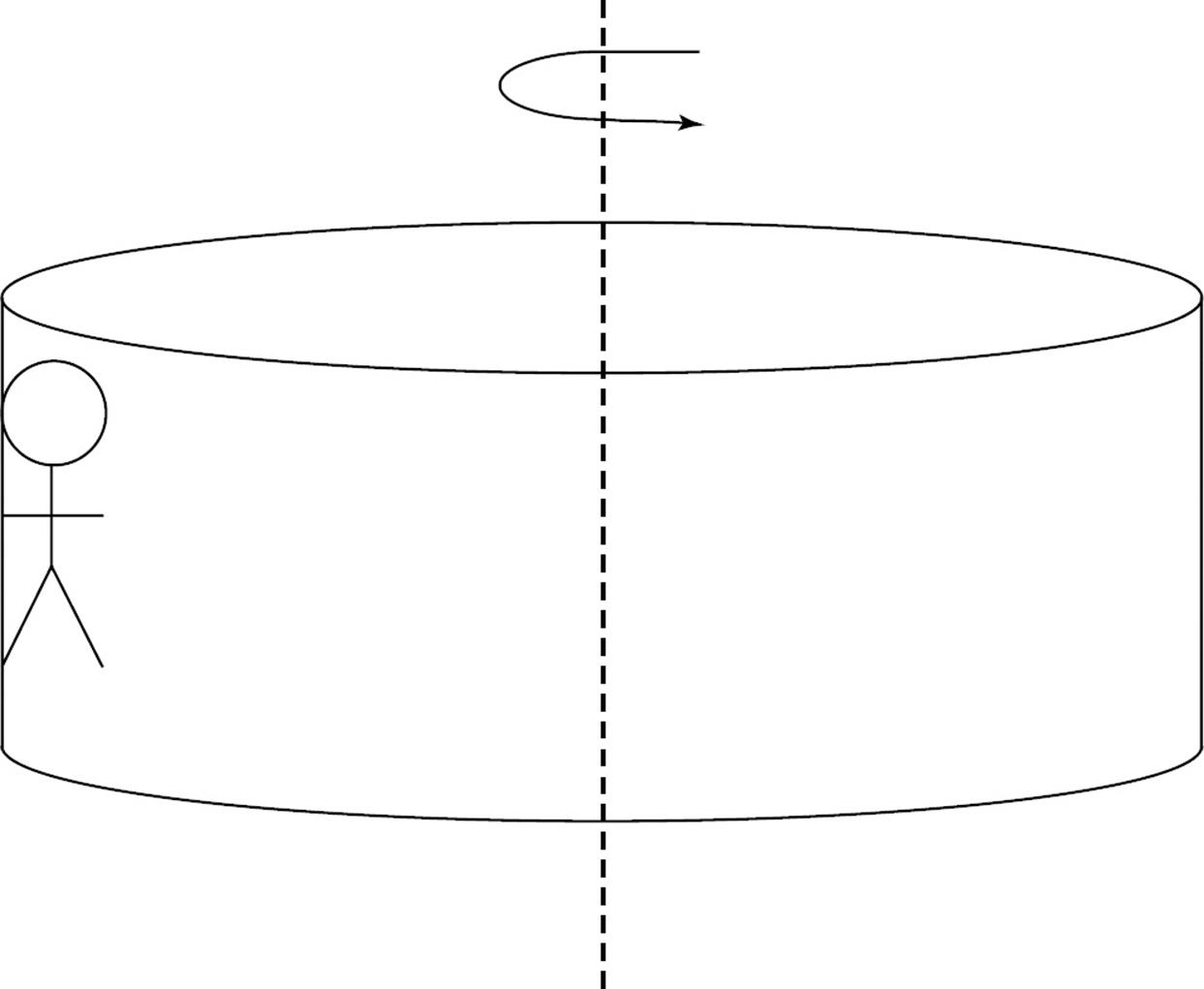
5. An amusement park ride consists of a large cylinder that rotates around its central axis as the passengers stand against the inner wall of the cylinder. Once the passengers are moving at a certain speed v, the floor on which they were standing is lowered. Each passenger feels pinned against the wall of the cylinder as it rotates. Let r be the inner radius of the cylinder.
Solve for the following in terms of given quantities and the acceleration of gravity, g.
(a) Draw and label all the forces acting on a passenger of mass m as the cylinder rotates with the floor lowered.
(b) Describe what conditions must hold to keep the passengers from sliding down the wall of the cylinder.
(c) Compare the conditions discussed in part (b) for an adult passenger of mass m and a child passenger of mass m/2.
6. A curved section of a highway has a radius of curvature of r. The coefficient of friction between standard automobile tires and the surface of the highway is μs.
(a) Draw and label all the forces acting on a car of mass m traveling along this curved part of the highway.
(b) Compute the maximum speed with which a car of mass m could make it around the turn without skidding in terms of μs, r, g, and m.
City engineers are planning on banking this curved section of highway at an angle of θ to the horizontal.
(c) Draw and label all of the forces acting on a car of mass m traveling along this banked turn. Do not include friction.
(d) The engineers want to be sure that a car of mass m traveling at a constant speed v (the posted speed limit) could make it safely around the banked turn even if the road were covered with ice (that is, essentially frictionless). Compute this banking angle θ in terms of r, v, g, and m.
Summary
Newton’s Laws
- Newton’s First Law (Law of Inertia) states that objects will continue in their state of motion unless acted upon by an unbalanced force.
- Newton’s Second Law: Fnet = ma
- Newton’s Third Law states that whenever two objects interact, the force the first object exerts on the second object is equal to, but in the opposite direction of, the force the second object exerts on the first object.
Weight
- The weight of an object is given by Fw = mg.
The Normal Force
- The normal force is the component of the contact force exerted on an object in contact with a surface and is perpendicular to the surface.
- The normal force can be represented by FN or N.
Friction
- Friction is the component of the contact force exerted on an object in contact with a surface and is parallel to the surface.
- Static friction occurs when there is no relative motion between the object and the surface. Its strength is given by the following equation: Fstatic friction, max = μsFN
- Kinetic friction occurs when there is relative motion between the two surfaces. Its strength is given by the following equation: Fkinetic friction = μkFN
Inclined Planes
- There are two components to the force of gravity on an object on an inclined plane: the force parallel to the ramp (mg sin θ) and the force normal to the ramp (mg cos θ).
- To simplify analysis of an object moving up or down a ramp, rotate the coordinate axes so that the x-axis is parallel to the incline and the y-axis is perpendicular to the incline.
Uniform Circular Motion
- The velocity vector is tangent to the circle.
- The centripetal acceleration points toward the center of the circle, and therefore the centripetal force must also point to the center of the circle.
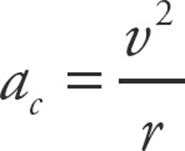
- Any force, or component of a force, that points toward the center of the circle is positive and any force, or component of a force, that points away from the center is negative when using the following equation: