Homework Helpers: Physics
1 Kinematics
We begin our study of physics with the branch called mechanics. Mechanics is the study of the motion of objects. Classical, or Newtonian, mechanics deals with the motion of macroscopic, or relatively large, objects. Quantum mechanics deals with the motion of microscopic particles.Kinematics is the study of the motion of objects without regard for the forces that influence the objects. In this chapter, we will concern ourselves solely with analyzing the motion of objects, not the forces that can change motion. We will begin to study forces in Chapter 2. The formulas for kinematics will allow you to analyze the motion of objects, such as baseballs and cars, which you encounter in your day-to-day life. As you learn the formulas, you might want to look for opportunities to apply them to real life. They will not be hard to find.
Lesson 1–1: Distance and Displacement
When studying any type of motion, perhaps the first thing that a person is likely to notice is a change in the object”s position. If we looked out a window and noticed a squirrel, we would have an opportunity to observe its motion. Of course, even a “stationary” squirrel is in constant motion from some point of view. The squirrel is on a planet that revolves and rotates in space. Furthermore, even when it appears to be standing still it may move its legs or tail, but in order to exhibit the type of motion that we are concerned with here, it would need to change its position relative to some “fixed” object, or reference point.
When we first noticed the squirrel, it might have been a certain distance away from a tree. Over time, it may move closer or farther away from the tree. Such a movement is referred to as a change in position and will be represented in this book by the symbol ΔX. Let”s suppose we first observed the squirrel when it was 1.0 m away from the tree (our reference point). After a burst of speed in a straight line, the squirrel stops at a point that is 2.5 m away from the same tree. The change in the squirrel”s position (ΔX) would be:
ΔX = Xfinal – Xinitial = 2.5 m – 1.0 m = 1.5 m.
We might also call this quantity the distance that the squirrel traveled, so distance represents how far an object moves. In physics we are often concerned with a similar quantity called displacement, which we will represent with the symbol d. Displacement represents the change in an object”s position in a particular direction. If we noted that the squirrel moved 1.5 m away from the tree in a direction of 25° east of south, we would be talking about the displacement of the squirrel. If we are comparing the distance and displacement of the squirrel in this example, it would be correct to describe the displacement as “the distance that the squirrel traveled in a particular direction.” However, it is much better to think of displacement as the change in an object”s position in a particular direction. The real distinction between distance and displacement will be made more apparent when we watch the squirrel for a bit longer.
Suppose we observed the squirrel for several more minutes in which it traveled a total of another 4.5 m, ultimately ending up in the same location (1.0 m away from the tree) as when we first observed it. Now, in the time that we had been observing it, the squirrel would have covered a total distance of 6.0 m (1.5 m + 4.5 m). What would be the overall displacement of the squirrel in this amount of time? Zero! Zero? Yes, zero. Because the squirrel ended up on its original starting point, the overall change in the squirrel”s position, measured from the tree or origin, is
d = ΔX = Xfinal – Xinitial = 1.0 m – 1.0 m = 0.0 m.
Distance, and other quantities that can be represented completely with numbers and units, are called scalars or scalar quantities. Displacement, on the other hand, is the first of several vector quantities that you will encounter in physics. A vector quantity, or just vector for short, must be represented by both a magnitude (number and units) and a direction. When our displacement was equal to zero, there was no need to specify a direction because there was no overall change in position. However, when there is a vector quantity that is not equal to zero, a direction must be stated to describe the quantity completely.
In physics, vector quantities are often represented by arrows. The length of the arrow can indicate the relative magnitude or size of the vector. The direction of the arrow indicates the direction of the vector. Using arrows to represent vectors allows us to solve some problems graphically. These arrows also help us visualize what is going on in a particular problem.
To illustrate the use of arrows to represent vectors, and to reinforce the distinction between scalar and vector quantities, let”s try a problem together.
Example 1
A squirrel starts from a reference point (origin) and travels 3.0 meters north to fetch an acorn. The squirrel then travels 5.0 meters east, and then another 3.0 m south. Find both the total distance the squirrel traveled, and its overall displacement.
Determining the distance that the squirrel traveled is quite easy, requiring neither arrows nor a class in physics to find. We simply add each of the distances together.
Total Distance = 3.0 m + 5.0 m + 3.0 m = 11.0 m
Notice that we can represent the total distance with numbers (11.0) and units (m) without mentioning direction, because distance is a scalar quantity. To illustrate the displacement of the squirrel we will represent each of the individual changes in position with vector arrows. The length of the arrows will relate to the distances the squirrel covered and the directions of the arrows will indicate the directions of the individual displacements. According to the standard convention, we will treat the top of the page as north, the bottom as south, the right as east, and the left as west.
Because each of these arrows represents a portion of the squirrel”s overall displacement, they are called component vectors. When we add them all together to find the overall displacement, we will find the resultant vector. We graphically add the arrows together using what is called the “tip-to-tail” method. As the name of this method implies, component vectors are added together in such a way that the tip of one component is made to touch the tail of the next component. The order of adding the component vectors does not matter.
The component vectors are added “tip-to-tail.”
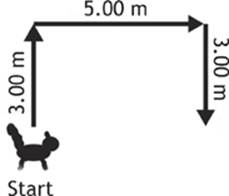
Figure 1.1
The next step in this method involves drawing the resultant vector. The resultant vector is the vector sum of all of the component vectors. Unlike the component vectors, the resultant vector is drawn from the tail of the first component vector to the tip of the last, as shown in Figure 1.2.
The resultant vector is drawn from “tail-to-tip.”

Figure 1.2
As with the component vectors, the magnitude of the resultant vector is indicated by the length of the arrow, and the direction is also shown. The advantage of this graphical method of vector addition is that if you draw the component vectors to scale, you can measure the magnitude of the resultant vector with a ruler. Even without a ruler, you can see that the resultant vector is the same length and in the same direction as the component vector for 5.00 m to the east. So then 5.00 m east is our resultant vector, or overall displacement.
Answer:
Total Distance = 3.00 m + 5.00 m + 3.00 m = 11.0 m
Total Displacement = 5.00 m east
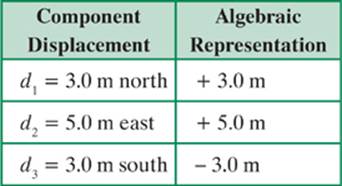
You may have noticed that the graphical method wasn”t entirely necessary for solving the problem in Example 1. Many of these problems can be solved with simple algebraic addition. The key to solving these problems involves assigning algebraic signs to the different directions. For example, we can consider the direction forward as a positive displacement, and reverse as a negative displacement. For Example 1, let”s consider north positive and south negative. Let”s also designate east as positive and west as negative. Using these conventions, we can find the resultant displacement for the problem in Example 1 as follows:
Now, we only add the vectors that lie along the same axis, so we add vectors d1 and d3, and find that they add up to zero, as shown here:
d 1+ d3 = +3.0 m + (–3.0 m) = 0 m
Because these two component vectors cancel each other out, the resultant vector is equal to the remaining component vector, 5.0 m to the east.
Which method you use to solve a particular problem will often depend on the specifics of the problem, and how much time you have to solve it. One thing that you will notice in physics is that the problems often seem easier to solve if you draw a sketch of what is going on in the problem. Often, the most successful students are the ones that get in the habit of making a visual representation of the problem.
Let”s try a couple more example problems. First, let”s try a problem that you may want to solve algebraically. If the answer comes to you right away, you are ready to move on to harder problems.
Example 2
A football quarterback backpedals for 1.4 m before seeing an opening, and then runs forward for 3.5 m. Find his overall displacement.
Given: d1 = –1.4 m d2 = 3.5 m
Find: dnet
Solution: dnet = d1 + d2 = –1.4 m + (+3.5 m) = +2.1 m forward
Example 2 represents the simplest of these types of problems, because all of the displacement occurred along a single access, or a straight line. The only “trick” to this type of problem is to be sure to designate one direction as positive and the other as negative.
For our third example, let”s try something a bit more difficult. Read the question first and then try to solve it on your own. I recommend making a diagram of the action. Some people get this type of example right away; others need to have it explained to them at first. The difference might have to do with how long it has been since you have studied geometry and trigonometry. If you have trouble with this next example, be sure to read the review of skills in the introduction of this book.
Example 3
A dog walks 3.0 m east and sniffs a fire hydrant. It then turns and walks 4.0 m north to sniff a tree. What is the overall displacement of the dog?
Notice that the component vectors do not lie along the same axis, so you can”t just add them algebraically. The solution to this will only present itself if you visualize the actual component displacements in your mind or on paper. Try the problem before looking at my following explanation.
The first thing that we want to do is sketch the problem using the “tip-to-tail” method that I described earlier in this lesson. We draw the component vectors in such a way that the tip of one vector touches the tail of the other. It doesn”t matter which vector you draw first, as I will show you shortly. Remember: If you use a ruler and draw these component vectors to scale, you will be able to find the magnitude of the resultant vector with the same ruler. For example, your scale may set each meter equal to a centimeter. You would then draw the first displacement vector (3.0 m east) 3.0 cm long, and the second displacement vector (4.0 m north) 4.0 cm long.
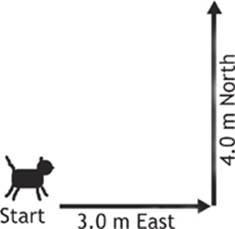
Figure 1.3
Now we are ready to draw the resultant vector. Remember that, unlike the component vectors, the resultant vector is drawn from the tail of the first component vector to the tip of the last, or “tail-to-tip.”
Figure 1.3 is not drawn to scale. If it were, we could find the magnitude of the resultant vector by measuring the resultant vector and apply it to our original scale. If you were careful with your own diagram, you would find it to be 5.0 cm long, representing a resultant vector of 5.0 m. However, you would still need to determine the direction of the resultant vector.
If you didn”t draw your diagram to scale, or you are not confident in your ability to find the magnitude of the resultant vector with the graphical method, you could also use the Pythagorean theorem.
The Pythagorean Theorem
a2 + b2 = c2
Given: d1 = 3.0 m d2 = 4.0 m
Find: dr (resultant displacement)
Isolate:
Let”s rewrite the Pythagorean theorem using our symbols: ![]()
Now, take the square root of both sides of the equation:
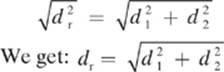
Solution:
![]()
Whichever method we use to find the magnitude of the resultant vector, we will still need to find the direction. In some situations, it may be enough to indicate that the direction of the resultant vector is “north-east,” but in other situations you will need to be more specific. If you carefully measured the lengths of the vectors involved, you can find the angle of the displacement vector using a protractor. If you are familiar with the type of triangle formed by our vectors, you may know the angles between each of the sides. If not, you can make use of one of the trigonometry functions that we went over in the Introduction.
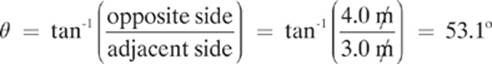
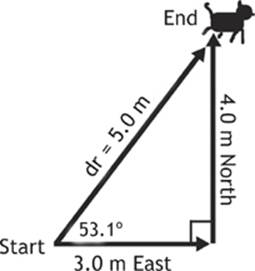
Figure 1.4
We find that the overall displacement of the dog is 5.0 m at 53.1° north of east.
I wanted to mention one last thing before we move on to the practice questions for this section. Remember that we said it doesn”t matter in what order you add component vectors, provided that you add them from tip-to-tail? Try the problem again, this time adding the tip of the 4.0 m vector to the tail of the 3.0 m vector. You will find the same resultant vector. In other words, it wouldn”t matter if the dog went 4.0 m north and then 3.0 m east, or if it went 3.0 m east and then 4.0 m north. It still ends up in the same place.
Try the following practice problems. Check your answers in the Answer Key (page 67) at the end of the chapter before moving on to the next lesson.
Lesson 1–1 Review
1. _______________ is a vector quantity that describes the change in an object”s position in terms of direction and distance.
2. A girl walks 33 m north, then 42 m east, and then 23 m west. Find the distance that she traveled.
3. A boy rides his bike 15 m due east and then 33 m due north. Find his displacement.